prove that:lim_(n→∞) ∫_(−1) ^1 (1+(t/n))^n dt = e−(1/e).


Question and Answers Forum

All Questions Topic List
Integration Questions
Previous in All Question Next in All Question
Previous in Integration Next in Integration
Question Number 47098 by rahul 19 last updated on 04/Nov/18

$p r o v e t h a t : \lim_{n \to \infty} \int_{- 1}^{1} {(1 + \frac{t}{n})}^{n} d t = e - \frac{1}{e} .$
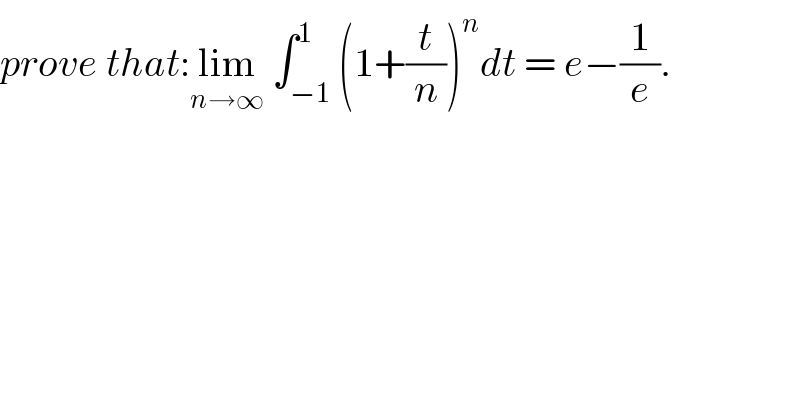
Commented by rahul 19 last updated on 04/Nov/18

$M y d o u b t i s w h y w e a r e t a k i n g l i m i t$ $f i r s t a n d t h e n a p p l y i n g i n t e g r a t i o n ? ?$ $I s t h i s c o r r e c t ? ?$
Commented by maxmathsup by imad last updated on 05/Nov/18

$s i r y o u m u s t t a k e a l o o k a n d r e v i s e d o m i n e n t c n v e r g e n c e t h e o r e m b e c a u s e$ $t h e r i s a c o n d i t o n s t o a p p l y t h i s t h e o r e m a n d i t s e a s y t o u s e i t t h a n o t h e r$ $m e t h o d .$
Commented by maxmathsup by imad last updated on 04/Nov/18

$l e t A_{n} = \int_{- 1}^{1} {(1 + \frac{t}{n})}^{n} d t = \int_{R} {(1 + \frac{t}{n})}^{n} χ_{[- 1, 1]} (t) d t l e t f_{n} (t) = {(1 + \frac{t}{n})}^{n} χ_{[- 1, 1]} (t)$ $w e h a v e {l i m}_{n \to + \infty} f_{n} (t) = e^{t} χ_{[- 1, 1]} (t) (s i m p l e c o n v e r g e n c e) a n d$ $∣ f_{n} (t) ∣ ⩽ e^{t} \forall t \in [- 1, 1] d o m i n e n t c o n v e r g e n c e g i v e$ ${l i m}_{n \to + \infty} A_{n} = \int_{R} {l i m}_{n \to} f_{n} (t) d t = \int_{R} e^{t} χ_{[- 1, 1]} (t) d t = \int_{- 1}^{1} e^{t} d t = {[e^{t}]}_{- 1}^{1}$ $= e - \frac{1}{e} .$
Commented by maxmathsup by imad last updated on 04/Nov/18
$another method let A_n = ∫_(−1) ^1 (1+(t/n))^n dt changement (t/n)=x give A_n =∫_(−(1/n)) ^(1/n) (1+x)^n ndx =n ∫_(−(1/n)) ^(1/n) (1+x)^n dx =(n/(n+1)) [(1+x)^(n+1) ]_(−(1/n)) ^(1/n) =(n/(n+1)){(1+(1/n))^(n+1) −(1−(1/n))^(n+1) } but (1+(1/n))^(n+1) =e^((n+1)ln( 1+(1/n))) but we have ln(1+(1/n))=(1/n)+o((1/n)) ⇒ (n+1)ln(1+(1/n))=((n+1)/n) +o(1) →1 (n→+∞) also (n+1)ln(1−(1/n)) =−((n+1)/n) →−1 (n→+∞) ⇒lim_(n→+∞) A_n =lim_(n→+∞) (n/(n+1))(e−e^(−1) ) ⇒ lim_(n→+∞) A_n =e −e^(−1) .$
$a n o t h e r m e t h o d l e t A_{n} = \int_{- 1}^{1} {(1 + \frac{t}{n})}^{n} d t c h a n g e m e n t \frac{t}{n} = x g i v e$ $A_{n} = \int_{- \frac{1}{n}}^{\frac{1}{n}} {(1 + x)}^{n} n d x = n \int_{- \frac{1}{n}}^{\frac{1}{n}} {(1 + x)}^{n} d x$ $= \frac{n}{n + 1} {[{(1 + x)}^{n + 1}]}_{- \frac{1}{n}}^{\frac{1}{n}} = \frac{n}{n + 1} {{(1 + \frac{1}{n})}^{n + 1} - {(1 - \frac{1}{n})}^{n + 1}} b u t$ ${(1 + \frac{1}{n})}^{n + 1} = e^{(n + 1) l n (1 + \frac{1}{n})} b u t w e h a v e l n (1 + \frac{1}{n}) = \frac{1}{n} + o (\frac{1}{n}) \Rightarrow$ $(n + 1) l n (1 + \frac{1}{n}) = \frac{n + 1}{n} + o (1) \to 1 (n \to + \infty) a l s o$ $(n + 1) l n (1 - \frac{1}{n}) = - \frac{n + 1}{n} \to - 1 (n \to + \infty) \Rightarrow {l i m}_{n \to + \infty} A_{n} = {l i m}_{n \to + \infty} \frac{n}{n + 1} (e - e^{- 1}) \Rightarrow$ ${l i m}_{n \to + \infty} A_{n} = e - e^{- 1} .$
Commented by peter frank last updated on 04/Nov/18

$pls help QN 46827$
Commented by rahul 19 last updated on 05/Nov/18

$P r o f A b d o,$ $p l s c l e a r m y d o u b t :$ $I n t h i s p r o b l e m w e a r e f i r s t g i v e n t o$ $i n t e g r a t e a n d t h e n t o a p p l y l i m i t s . . .$ $B u t i f w e f i r s t a p p l y l i m i t s a n d t h e n$ $i n t e g r a t e w e a r e g e t t i n g s a m e r e s u l t$ $a n d w h i c h i s s h o r t e r m e t h o d t o o!$ $S o, i s t h i s m e t h o d c o r r e c t o r i s i t j u s t a$ $c o i n c i d e n c e ?$
Commented by rahul 19 last updated on 05/Nov/18

$o k a y t h a n k y o u p r o f!$ $I^{'} m n e w t o d o m i n e n t c o n v e r g e n c e!!$
Commented by maxmathsup by imad last updated on 06/Nov/18

$n e v e r m i n d s i r m a t h s i s l i k e a s e a w t h o u t b o r d e r s . . . .$
	Answered by tanmay.chaudhury50@gmail.com last updated on 04/Nov/18

	$I = \int {(1 + \frac{t}{n})}^{n} d t$ $k = 1 + \frac{t}{n} d k = \frac{d t}{n}$ $\int k^{n} \times n d k$ $n \times \frac{k^{n + 1}}{n + 1} + c_{1}$ $s o t h e i n t r e g s l i s$ $\frac{n}{n + 1} \times ∣ {(1 + \frac{t}{n})}^{n + 1} ∣_{- 1}^{1}$ $\frac{n}{n + 1} [{(1 + \frac{1}{n})}^{n + 1} - {(1 - \frac{1}{n})}^{n + 1}$ $n o w a p p l y l i m i t$ $\lim_{n \to \infty} \frac{1}{1 + \frac{1}{n}} [{(1 + \frac{1}{n})}^{n + 1} - {(1 - \frac{1}{n})}^{n + 1}]$ $= \lim_{n \to \infty} \frac{1}{1 + \frac{1}{n}} \times [\lim_{n \to \infty} (1 + \frac{1}{n}) \times \lim_{n \to \infty} {(1 + \frac{1}{n})}^{n} - \lim_{n \to \infty [} (1 - \frac{1}{n}) \times \lim_{n \to \infty} {(1 - \frac{1}{n})}^{n}]$ $= \frac{1}{1 + 0} \times [(1 + 0) \times e - (1 - 0) \times e^{- 1}]$ $= (e - \frac{1}{e})$ $f i r s t i n t r e g a t i o n t h e n l i m i t d o n e$ $p l s c h e c k . . .$ $n o w f o r m u l a$ $\underset{n \to \infty}{li p}$ $= \underset{n \to \infty}{li l} \times \underset{n \to \infty}{li}$ $n z = \underset{n \to \infty}{li}$ $= \underset{y \to 0}{li}$ $z = e$ $\underset{n \to \infty}{li}$ $l n p = \underset{n \to \infty}{li}$ $w = \frac{1}{n} \underset{w \to 0}{li}$ $= \underset{w \to 0}{li}$
	Commented by rahul 19 last updated on 05/Nov/18
	thanks sir ��
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum