Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 47540 by maxmathsup by imad last updated on 11/Nov/18

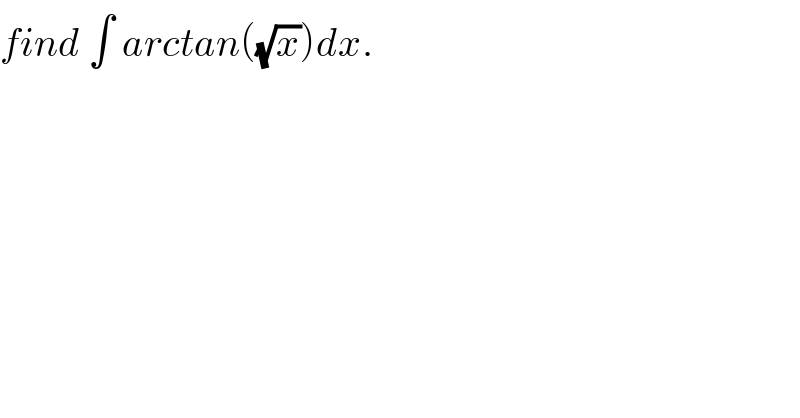
find ∫ arctan((√x))dx.

$${find}\:\int\:{arctan}\left(\sqrt{{x}}\right){dx}. \\ $$

Commented by maxmathsup by imad last updated on 16/Nov/18

let I=∫ arctan((√x))dx changement (√x)=t give I = ∫ arctan(t)(2t)dt =2 ∫ t arctan(t)dt =_(by parts) 2 { (t^2 /2)arctan(t)−∫ (t^2 /2) (dt/(1+t^2 ))}=t^2 arctan(t) −∫ (t^2 /(1+t^2 ))dt but ∫ (t^2 /(1+t^2 ))dt =∫ ((1+t^2 −1)/(1+t^2 ))dt=t−arctan(t)+c ⇒ I =t^2 arctan(t)−t +arctan(t) +c =x arctan((√x))−(√x)+arctan((√x)) +c ⇒ I =(x+1)arctan((√x)) −(√x)+c .

$${let}\:{I}=\int\:{arctan}\left(\sqrt{{x}}\right){dx}\:{changement}\:\sqrt{{x}}={t}\:{give} \\ $$$${I}\:=\:\int\:\:{arctan}\left({t}\right)\left(\mathrm{2}{t}\right){dt}\:=\mathrm{2}\:\int\:\:{t}\:{arctan}\left({t}\right){dt} \\ $$$$=_{{by}\:{parts}} \:\:\:\mathrm{2}\:\left\{\:\frac{{t}^{\mathrm{2}} }{\mathrm{2}}{arctan}\left({t}\right)−\int\:\:\frac{{t}^{\mathrm{2}} }{\mathrm{2}}\:\frac{{dt}}{\mathrm{1}+{t}^{\mathrm{2}} }\right\}={t}^{\mathrm{2}} {arctan}\left({t}\right)\:−\int\:\frac{{t}^{\mathrm{2}} }{\mathrm{1}+{t}^{\mathrm{2}} }{dt}\:{but} \\ $$$$\int\:\:\frac{{t}^{\mathrm{2}} }{\mathrm{1}+{t}^{\mathrm{2}} }{dt}\:=\int\:\frac{\mathrm{1}+{t}^{\mathrm{2}} −\mathrm{1}}{\mathrm{1}+{t}^{\mathrm{2}} }{dt}={t}−{arctan}\left({t}\right)+{c}\:\Rightarrow \\ $$$${I}\:={t}^{\mathrm{2}} {arctan}\left({t}\right)−{t}\:+{arctan}\left({t}\right)\:+{c}\:={x}\:{arctan}\left(\sqrt{{x}}\right)−\sqrt{{x}}+{arctan}\left(\sqrt{{x}}\right)\:+{c}\:\Rightarrow \\ $$$${I}\:=\left({x}+\mathrm{1}\right){arctan}\left(\sqrt{{x}}\right)\:−\sqrt{{x}}+{c}\:. \\ $$

Answered by arcana last updated on 11/Nov/18

w=(√x) dw=(1/(2(√x)))dx=(1/(2w))dx ∫2wtan^(−1) (w)dw u=tan^(−1) (w) dv=2wdw du=(1/(1+w^2 ))dw v=w^2 ∫2wtan^(−1) (w)dw=w^2 tan^(−1) (w)−∫(w^2 /(1+w^2 ))dw ∫((1+w^2 −1)/(1+w^2 ))dw =w−∫(1/(1+w^2 ))dw =w−tan^(−1) (w)+C ∫2wtan^(−1) (w)dw=w^2 tan^(−1) (w)−w+tan^(−1) (w)+C =(1+w^2 )tan^(−1) (w)−w+C =(1+x)tan^(−1) ((√x))−(√x)+C

$${w}=\sqrt{{x}} \\ $$$${dw}=\frac{\mathrm{1}}{\mathrm{2}\sqrt{{x}}}{dx}=\frac{\mathrm{1}}{\mathrm{2}{w}}{dx} \\ $$$$\int\mathrm{2}{wtan}^{−\mathrm{1}} \left({w}\right){dw} \\ $$$${u}={tan}^{−\mathrm{1}} \left({w}\right)\:\:\:\:\:{dv}=\mathrm{2}{wdw} \\ $$$${du}=\frac{\mathrm{1}}{\mathrm{1}+{w}^{\mathrm{2}} }{dw}\:\:\:\:\:\:\:{v}={w}^{\mathrm{2}} \\ $$$$\int\mathrm{2}{wtan}^{−\mathrm{1}} \left({w}\right){dw}={w}^{\mathrm{2}} {tan}^{−\mathrm{1}} \left({w}\right)−\int\frac{{w}^{\mathrm{2}} }{\mathrm{1}+{w}^{\mathrm{2}} }{dw} \\ $$$$\int\frac{\mathrm{1}+{w}^{\mathrm{2}} −\mathrm{1}}{\mathrm{1}+{w}^{\mathrm{2}} }{dw}\:={w}−\int\frac{\mathrm{1}}{\mathrm{1}+{w}^{\mathrm{2}} }{dw} \\ $$$$={w}−{tan}^{−\mathrm{1}} \left({w}\right)+\mathrm{C} \\ $$$$\int\mathrm{2}{wtan}^{−\mathrm{1}} \left({w}\right){dw}={w}^{\mathrm{2}} {tan}^{−\mathrm{1}} \left({w}\right)−{w}+{tan}^{−\mathrm{1}} \left({w}\right)+\mathrm{C} \\ $$$$=\left(\mathrm{1}+{w}^{\mathrm{2}} \right){tan}^{−\mathrm{1}} \left({w}\right)−{w}+\mathrm{C} \\ $$$$=\left(\mathrm{1}+{x}\right){tan}^{−\mathrm{1}} \left(\sqrt{{x}}\right)−\sqrt{{x}}+\mathrm{C} \\ $$$$ \\ $$

Commented by maxmathsup by imad last updated on 16/Nov/18

correct answer thanks Arcana.

$${correct}\:{answer}\:{thanks}\:{Arcana}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com