Math Question and Answers


Question and Answers Forum

All Questions Topic List
Geometry Questions
Previous in All Question Next in All Question
Previous in Geometry Next in Geometry
Question Number 47657 by behi83417@gmail.com last updated on 12/Nov/18

Commented by mr W last updated on 13/Nov/18

$d e a r f a t h e r f r o m B e h i :$ $i t t o o k m e s o m e t i m e, b u t I c o u l d f i n a l l y$ $You can't use 'macro parameter character #' in math mode$ $t h i s i n t e r e s t i n g q u e s t i o n w a s o n c e$ $t r e a t e d . I g a v e t h e r e a m e t h o d h o w$ $t o d r a w t h e r e q u e s t e d e q u i l a t e r a l$ $t r i a n g l e a n d t h e f o r m u l a t o c a l c u l a t e$ $i t s s i d e l e n g t h w h i c h i s$ $l = \sqrt{\frac{a^{2} + b^{2} + c^{2} \pm \sqrt{6 (a^{2} b^{2} + b^{2} c^{2} + c^{2} a^{2}) - 3 (a^{4} + b^{4} + c^{4})}}{2}}$ $w i t h a, b, c = d i s t a n c e s f r o m a p o i n t$ $t o t h e v e r t e x e s o f t h e t r i a n g l e .$ $i n o u r c a s e w e h a v e$ $a = 5$ $b = 7$ $c = 11$ $\Rightarrow l \approx 11.9342 o r \approx 7.2509$
Commented by MJS last updated on 13/Nov/18

$great!$ $but how to decide if the given point is inside$ $our outside the triangle (s) ?$
Commented by behi83417@gmail.com last updated on 13/Nov/18

$i h a v e n o w o r d s t o s a y m a s t e r .$ $i j u s t s a y t h a n k s i n a d v a n c e s i r .$ $. . . . . . a n d m e m o r i e s a t t a c k!$
Commented by mr W last updated on 11/Feb/20

$t o s e e i f t h e p o i n t p i s i n s i d e o r o u t s i d e$ $t h e t r i a n g l e, w e c a l c u l a t e t h e a n g l e s$ $b e t w e e n a a n d b, b a n d c a s w e l l a s$ $c a n d a . i f a l l o f t h e s e a n g l e s a r e$ $o b t u s e, t h e n p o i n t p l i e s i n s i d e .$ $i f a t l e a s t o n e o f t h e s e a n g l e s i s a c u t e,$ $t h e n p o i n t p l i e s o u t s i d e .$ $i n o u r e x a m p l e :$ $w i t h l = 11.9342$ $\cos α = \frac{b^{2} + c^{2} - l^{2}}{2 b c} \Rightarrow α = 79.7 ° < 90 °$ $\cos β = \frac{c^{2} + a^{2} - l^{2}}{2 c a} \Rightarrow β = 88.1 ° < 90 °$ $\cos γ = \frac{a^{2} + b^{2} - l^{2}}{2 a b} \Rightarrow γ = 167.8 °$ $\Rightarrow p i s o u t s i d e .$ $w i t h l = 7.2509$ $\cos α = \frac{b^{2} + c^{2} - l^{2}}{2 b c} \Rightarrow α = 40.3 ° < 90 °$ $\cos β = \frac{c^{2} + a^{2} - l^{2}}{2 c a} \Rightarrow β = 31.9 ° < 90 °$ $\cos γ = \frac{a^{2} + b^{2} - l^{2}}{2 a b} \Rightarrow γ = 72.2 ° < 90 °$ $\Rightarrow p i s o u t s i d e .$ $[c o n c l u s i o n n o t c o r r e c t, n e e d s t o b e$ $a m e n d e d . . .]$
Commented by MJS last updated on 13/Nov/18

$thank you$
Commented by MJS last updated on 14/Nov/18

$l = \sqrt{\frac{a^{2} + b^{2} + c^{2} \pm \sqrt{6 (a^{2} b^{2} + b^{2} c^{2} + c^{2} a^{2}) - 3 (a^{4} + b^{4} + c^{4})}}{2}} =$ $= \sqrt{\frac{a^{2} + b^{2} + c^{2} \pm \sqrt{3 δ}}{2}}; δ = (a + b + c) (a + b - c) (a - b + c) (- a + b + c)$
Commented by mr W last updated on 14/Nov/18

$t h a n k s f o r t h i s e a s y t o r e m e m b e r$ $f o r m!$
	Answered by MJS last updated on 13/Nov/18

	$A = (\begin{matrix} 0 \\ 0 \end{matrix}) B = (\begin{matrix} s \\ 0 \end{matrix}) C = (\begin{matrix} \frac{1}{2} s \\ \frac{\sqrt{3}}{2} s \end{matrix})$ $P = (\begin{matrix} p \\ q \end{matrix})$ $∣ A P ∣^{2} = 25 \Rightarrow p^{2} + q^{2} - 25 = 0$ $∣ B P ∣^{2} = 49 \Rightarrow p^{2} + q^{2} + s^{2} - 2 p s - 49 = 0$ $∣ C P ∣^{2} = 121 \Rightarrow p^{2} + q^{2} + s^{2} - p s - \sqrt{3} q s - 121 = 0$ $Solution 1$ $p \approx 4.96 q \approx - . 62 s \approx 11.93$ $A = (\begin{matrix} 0 \\ 0 \end{matrix}) B = (\begin{matrix} 11.93 \\ 0 \end{matrix}) C = (\begin{matrix} 5.97 \\ 10.34 \end{matrix}) P = (\begin{matrix} 4.96 \\ - . 62 \end{matrix})$ $Solution 2$ $p \approx 1.97 q \approx - 4.60 s \approx 7.25$ $A = (\begin{matrix} 0 \\ 0 \end{matrix}) B = (\begin{matrix} 7.25 \\ 0 \end{matrix}) C = (\begin{matrix} 3.63 \\ 6.28 \end{matrix}) P = (\begin{matrix} 1.97 \\ - 4.60 \end{matrix})$ $\Rightarrow no solution within the triangle$ $these are symmetrically the same :$ $Solution 3$ $p \approx - 4.96 q \approx . 62 s \approx - 11.93$ $Solution 4$ $p \approx - 1.97 q \approx 4.60 s \approx - 7.25$
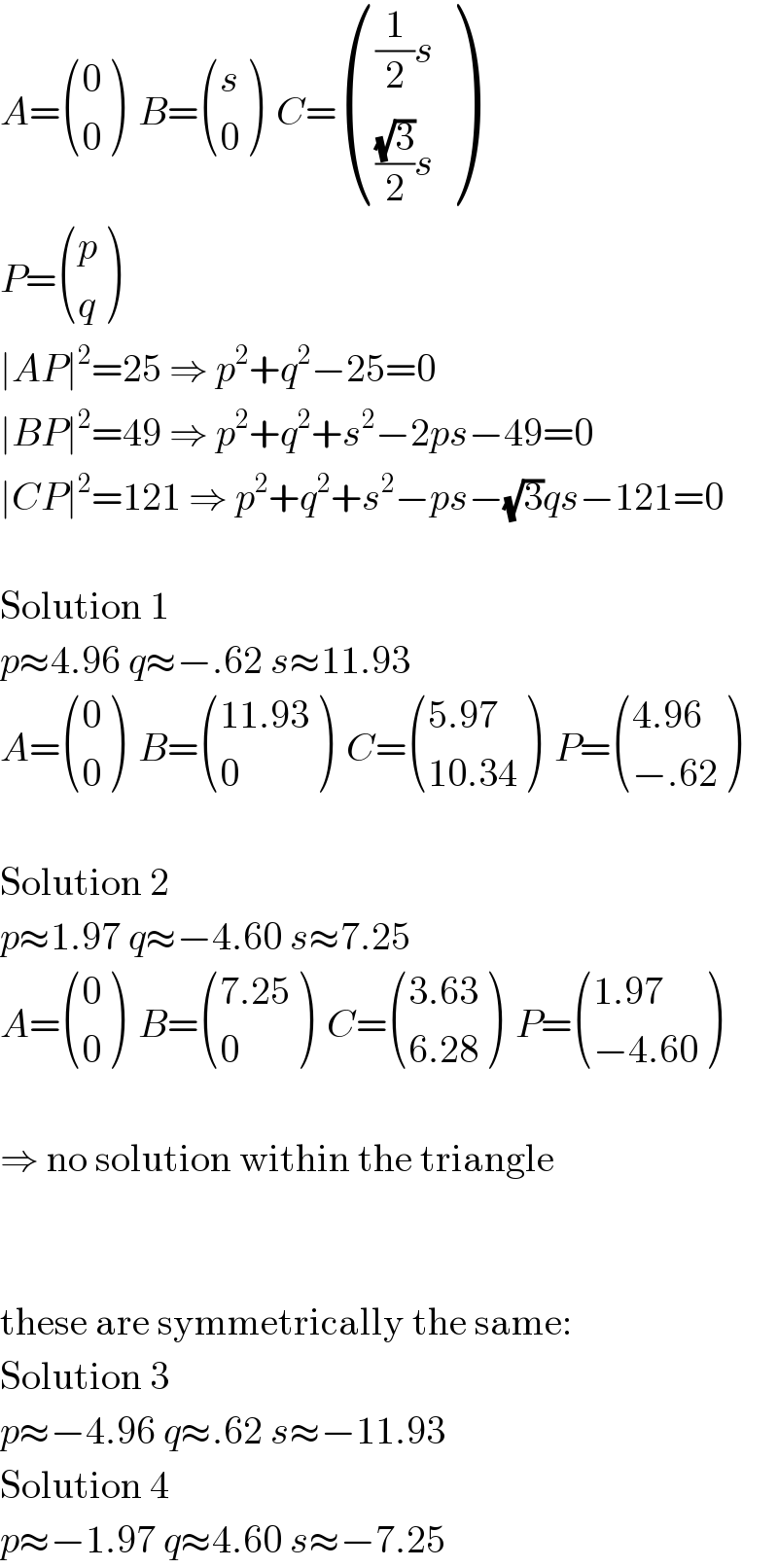
	Commented by behi83417@gmail.com last updated on 13/Nov/18

	$t h a n k y o u s o m u c h d e a r M J S .$ $t h i s i s n i c e a n d s i m p l e .$ $s = 11.93, 7.25 i s t h e c o r r e c t a n s w e r .$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum