Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 48497 by maxmathsup by imad last updated on 24/Nov/18

let f(x)=∫_0 ^1 ((ln(1+xt^2 ))/(1+t^2 ))dt 1) find a xplicit form of f(x) 2) developp f at integr serie 3)find the value of ∫_0 ^1 ((ln(1+t^2 ))/(1+t^2 ))dt 4)find the value of ∫_0 ^1 ((ln(1+2t^2 ))/(1+t^2 ))dt

$${let}\:{f}\left({x}\right)=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{ln}\left(\mathrm{1}+{xt}^{\mathrm{2}} \right)}{\mathrm{1}+{t}^{\mathrm{2}} }{dt} \\ $$$$\left.\mathrm{1}\right)\:{find}\:{a}\:{xplicit}\:{form}\:{of}\:{f}\left({x}\right) \\ $$$$\left.\mathrm{2}\right)\:{developp}\:{f}\:{at}\:{integr}\:{serie}\: \\ $$$$\left.\mathrm{3}\right){find}\:{the}\:{value}\:{of}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{ln}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)}{\mathrm{1}+{t}^{\mathrm{2}} }{dt} \\ $$$$\left.\mathrm{4}\right){find}\:{the}\:{value}\:{of}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{ln}\left(\mathrm{1}+\mathrm{2}{t}^{\mathrm{2}} \right)}{\mathrm{1}+{t}^{\mathrm{2}} }{dt} \\ $$$$ \\ $$

Commented by maxmathsup by imad last updated on 26/Nov/18

1) we have f^′ (x)=∫_0 ^1 (t^2 /((1+xt^2 )(1+t^2 )))dt let decompose F(t)=(t^2 /((1+xt^2 )(1+t^2 ))) case 1 if x>0 ⇒F(x)=((at +b)/((1+xt^2 ))) +((ct +d)/(1+t^2 )) F(−x) =F(x)⇒((−at +b)/(1+xt^2 )) +((−ct +d)/(1+t^2 )) =((at +b)/(1+xt^2 )) +((ct +d)/(1+t^2 )) ⇒ a=0 and c =0 ⇒F(x)=(b/(1+xt^2 )) +(d/(1+t^2 )) lim_(t→+∞) t^2 F(t)=(1/x) =(b/x) +d ⇒1 =b +xd F(0)=0 =b+d ⇒d =−b ⇒1=b−xb =(1−x)b ⇒b=(1/(1−x)) and d=−(1/(1−x)) F(t) = (1/((1−x))){(1/(1+xt^2 )) −(1/(1+t^2 ))} ⇒f(x)=(1/(1−x))∫_0 ^1 (dt/(1+xt^2 )) −(1/(1−x)) ∫_0 ^1 (dt/(1+t^2 )) but ∫_0 ^1 (dt/(1+xt^2 )) =_((√x)t =u) ∫_0 ^(√x) (du/((√x)(1+u^2 ))) =(1/(√x)) arctan((√x)) ⇒ f^′ (x) =((arctan((√x)))/((1−x)(√x))) −(π/(4(1−x))) ⇒f(x)=∫_0 ^x ((arctan((√t)))/((1−t)(√t)))dt+(π/4)ln∣1−x∣ changement (√t)=u give ∫_0 ^x ((arctan((√t)))/((√t)(1−t))) dt =∫_0 ^(√x) ((arctan(u))/(u(1−u^2 )))(2u)du =2 ∫_0 ^(√x) ((arctan(u))/(1−u^2 )) du ⇒f(x)=2 ∫_0 ^(√x) ((arctan(u))/(1−u^2 ))du +(π/4)ln∣1−x∣ (x≠1)

$$\left.\mathrm{1}\right)\:{we}\:{have}\:{f}^{'} \left({x}\right)=\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{t}^{\mathrm{2}} }{\left(\mathrm{1}+{xt}^{\mathrm{2}} \right)\left(\mathrm{1}+{t}^{\mathrm{2}} \right)}{dt}\:\:{let}\:{decompose}\:{F}\left({t}\right)=\frac{{t}^{\mathrm{2}} }{\left(\mathrm{1}+{xt}^{\mathrm{2}} \right)\left(\mathrm{1}+{t}^{\mathrm{2}} \right)} \\ $$$${case}\:\mathrm{1}\:\:\:{if}\:{x}>\mathrm{0}\:\Rightarrow{F}\left({x}\right)=\frac{{at}\:+{b}}{\left(\mathrm{1}+{xt}^{\mathrm{2}} \right)}\:+\frac{{ct}\:+{d}}{\mathrm{1}+{t}^{\mathrm{2}} } \\ $$$${F}\left(−{x}\right)\:={F}\left({x}\right)\Rightarrow\frac{−{at}\:\:+{b}}{\mathrm{1}+{xt}^{\mathrm{2}} }\:\:+\frac{−{ct}\:+{d}}{\mathrm{1}+{t}^{\mathrm{2}} }\:=\frac{{at}\:+{b}}{\mathrm{1}+{xt}^{\mathrm{2}} }\:+\frac{{ct}\:+{d}}{\mathrm{1}+{t}^{\mathrm{2}} }\:\Rightarrow \\ $$$${a}=\mathrm{0}\:{and}\:{c}\:=\mathrm{0}\:\Rightarrow{F}\left({x}\right)=\frac{{b}}{\mathrm{1}+{xt}^{\mathrm{2}} }\:+\frac{{d}}{\mathrm{1}+{t}^{\mathrm{2}} } \\ $$$${lim}_{{t}\rightarrow+\infty} \:{t}^{\mathrm{2}} \:{F}\left({t}\right)=\frac{\mathrm{1}}{{x}}\:=\frac{{b}}{{x}}\:+{d}\:\Rightarrow\mathrm{1}\:={b}\:+{xd}\: \\ $$$${F}\left(\mathrm{0}\right)=\mathrm{0}\:={b}+{d}\:\Rightarrow{d}\:=−{b}\:\Rightarrow\mathrm{1}={b}−{xb}\:=\left(\mathrm{1}−{x}\right){b}\:\Rightarrow{b}=\frac{\mathrm{1}}{\mathrm{1}−{x}}\:{and}\:{d}=−\frac{\mathrm{1}}{\mathrm{1}−{x}} \\ $$$${F}\left({t}\right)\:=\:\frac{\mathrm{1}}{\left(\mathrm{1}−{x}\right)}\left\{\frac{\mathrm{1}}{\mathrm{1}+{xt}^{\mathrm{2}} }\:−\frac{\mathrm{1}}{\mathrm{1}+{t}^{\mathrm{2}} }\right\}\:\Rightarrow{f}\left({x}\right)=\frac{\mathrm{1}}{\mathrm{1}−{x}}\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{dt}}{\mathrm{1}+{xt}^{\mathrm{2}} }\:−\frac{\mathrm{1}}{\mathrm{1}−{x}}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{dt}}{\mathrm{1}+{t}^{\mathrm{2}} } \\ $$$${but}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\frac{{dt}}{\mathrm{1}+{xt}^{\mathrm{2}} }\:=_{\sqrt{{x}}{t}\:={u}} \:\:\:\:\:\int_{\mathrm{0}} ^{\sqrt{{x}}} \:\:\:\:\:\frac{{du}}{\sqrt{{x}}\left(\mathrm{1}+{u}^{\mathrm{2}} \right)}\:=\frac{\mathrm{1}}{\sqrt{{x}}}\:{arctan}\left(\sqrt{{x}}\right)\:\Rightarrow \\ $$$${f}^{'} \left({x}\right)\:=\frac{{arctan}\left(\sqrt{{x}}\right)}{\left(\mathrm{1}−{x}\right)\sqrt{{x}}}\:\:−\frac{\pi}{\mathrm{4}\left(\mathrm{1}−{x}\right)}\:\Rightarrow{f}\left({x}\right)=\int_{\mathrm{0}} ^{{x}} \:\:\frac{{arctan}\left(\sqrt{{t}}\right)}{\left(\mathrm{1}−{t}\right)\sqrt{{t}}}{dt}+\frac{\pi}{\mathrm{4}}{ln}\mid\mathrm{1}−{x}\mid \\ $$$${changement}\:\sqrt{{t}}={u}\:{give}\:\int_{\mathrm{0}} ^{{x}} \:\frac{{arctan}\left(\sqrt{{t}}\right)}{\sqrt{{t}}\left(\mathrm{1}−{t}\right)}\:{dt}\:=\int_{\mathrm{0}} ^{\sqrt{{x}}} \:\:\frac{{arctan}\left({u}\right)}{{u}\left(\mathrm{1}−{u}^{\mathrm{2}} \right)}\left(\mathrm{2}{u}\right){du} \\ $$$$=\mathrm{2}\:\int_{\mathrm{0}} ^{\sqrt{{x}}} \:\:\:\frac{{arctan}\left({u}\right)}{\mathrm{1}−{u}^{\mathrm{2}} }\:{du}\:\Rightarrow{f}\left({x}\right)=\mathrm{2}\:\int_{\mathrm{0}} ^{\sqrt{{x}}} \:\:\frac{{arctan}\left({u}\right)}{\mathrm{1}−{u}^{\mathrm{2}} }{du}\:+\frac{\pi}{\mathrm{4}}{ln}\mid\mathrm{1}−{x}\mid\:\:\:\left({x}\neq\mathrm{1}\right) \\ $$

Commented by maxmathsup by imad last updated on 26/Nov/18

case 2 x<0 ⇒F(x)=(t^2 /(1−((√(−x))t)^2 (1+t^2 ))) =−(t^2 /(((√(−x))t−1)((√(−x))t +1)(t^2 +1))) =(a/(((√(−x))t−1))) +(b/((√(−x))t +1)) +((ct +d)/(t^2 +1)) ⇒f(x)=(a/(√(−x)))ln∣(√(−x))t+1∣+(b/(√(−x)))ln∣(√(−x))t+1) +(c/2)ln(t^2 +1) +d arctant +c....

$${case}\:\mathrm{2}\:{x}<\mathrm{0}\:\Rightarrow{F}\left({x}\right)=\frac{{t}^{\mathrm{2}} }{\mathrm{1}−\left(\sqrt{−{x}}{t}\right)^{\mathrm{2}} \left(\mathrm{1}+{t}^{\mathrm{2}} \right)}\:=−\frac{{t}^{\mathrm{2}} }{\left(\sqrt{−{x}}{t}−\mathrm{1}\right)\left(\sqrt{−{x}}{t}\:+\mathrm{1}\right)\left({t}^{\mathrm{2}} \:+\mathrm{1}\right)} \\ $$$$\left.=\frac{{a}}{\left(\sqrt{−{x}}{t}−\mathrm{1}\right)}\:+\frac{{b}}{\sqrt{−{x}}{t}\:+\mathrm{1}}\:+\frac{{ct}\:+{d}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:\:\:\Rightarrow{f}\left({x}\right)=\frac{{a}}{\sqrt{−{x}}}{ln}\mid\sqrt{−{x}}{t}+\mathrm{1}\mid+\frac{{b}}{\sqrt{−{x}}}{ln}\mid\sqrt{−{x}}{t}+\mathrm{1}\right) \\ $$$$+\frac{{c}}{\mathrm{2}}{ln}\left({t}^{\mathrm{2}} \:+\mathrm{1}\right)\:+{d}\:{arctant}\:\:+{c}.... \\ $$

Commented by maxmathsup by imad last updated on 26/Nov/18

2) we have f(x)=∫_0 ^1 ln(1+xt^2 )(Σ_(n=0) ^∞ (−1)^n t^(2n) )dt =Σ_(n=0) ^∞ (−1)^n ∫_0 ^1 t^(2n) ln(1+xt^2 )dt =Σ_(n=0) ^∞ (−1)^n A_n by parts A_n =∫_0 ^1 t^(2n) ln(1+xt^2 )dt =[(1/(2n+1))t^(2n+1) ln(1+xt^2 )]_(t=0) ^1 −∫_0 ^1 (1/(2n+1))t^(2n+1) ((2xt)/(1+xt^2 ))dt =((ln(1+x))/(2n+1)) −((2x)/(2n+1)) ∫_0 ^1 (t^(2n+2) /(1+xt^2 )) dt but ∫_0 ^1 (t^(2n+2) /(1+xt^2 )) dt =_((√x)t=u) ∫_0 ^(√x) (u^(2n+2) /(x^(n+1) (1+u^2 ))) (du/(√x)) =(1/(x^(n+1) (√x))) ∫_0 ^(√x) (u^(2n+2) /(u^2 +1)) du and changement u=tanθ give ∫_0 ^(√x) (u^(2n+2) /(u^2 +1)) du =∫_0 ^(arctan((√x))) ((tan^(2n+2) θ)/(1+tan^2 θ)) (1+tan^2 θ) =∫_0 ^(arctan((√x))) tan^(2n+2) θ dθ be continued...

$$\left.\mathrm{2}\right)\:{we}\:{have}\:{f}\left({x}\right)=\int_{\mathrm{0}} ^{\mathrm{1}} \:{ln}\left(\mathrm{1}+{xt}^{\mathrm{2}} \right)\left(\sum_{{n}=\mathrm{0}} ^{\infty} \left(−\mathrm{1}\right)^{{n}} {t}^{\mathrm{2}{n}} \right){dt} \\ $$$$=\sum_{{n}=\mathrm{0}} ^{\infty} \:\left(−\mathrm{1}\right)^{{n}} \:\int_{\mathrm{0}} ^{\mathrm{1}} {t}^{\mathrm{2}{n}} {ln}\left(\mathrm{1}+{xt}^{\mathrm{2}} \right){dt}\:=\sum_{{n}=\mathrm{0}} ^{\infty} \left(−\mathrm{1}\right)^{{n}} \:{A}_{{n}} \:\:\:{by}\:{parts} \\ $$$${A}_{{n}} =\int_{\mathrm{0}} ^{\mathrm{1}} \:{t}^{\mathrm{2}{n}} {ln}\left(\mathrm{1}+{xt}^{\mathrm{2}} \right){dt}\:=\left[\frac{\mathrm{1}}{\mathrm{2}{n}+\mathrm{1}}{t}^{\mathrm{2}{n}+\mathrm{1}} {ln}\left(\mathrm{1}+{xt}^{\mathrm{2}} \right)\right]_{{t}=\mathrm{0}} ^{\mathrm{1}} \:−\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{1}}{\mathrm{2}{n}+\mathrm{1}}{t}^{\mathrm{2}{n}+\mathrm{1}} \:\frac{\mathrm{2}{xt}}{\mathrm{1}+{xt}^{\mathrm{2}} }{dt} \\ $$$$=\frac{{ln}\left(\mathrm{1}+{x}\right)}{\mathrm{2}{n}+\mathrm{1}}\:−\frac{\mathrm{2}{x}}{\mathrm{2}{n}+\mathrm{1}}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{t}^{\mathrm{2}{n}+\mathrm{2}} }{\mathrm{1}+{xt}^{\mathrm{2}} }\:{dt}\:{but}\: \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{t}^{\mathrm{2}{n}+\mathrm{2}} }{\mathrm{1}+{xt}^{\mathrm{2}} }\:{dt}\:=_{\sqrt{{x}}{t}={u}} \:\:\:\int_{\mathrm{0}} ^{\sqrt{{x}}} \:\:\:\:\:\frac{{u}^{\mathrm{2}{n}+\mathrm{2}} }{{x}^{{n}+\mathrm{1}} \left(\mathrm{1}+{u}^{\mathrm{2}} \right)}\:\frac{{du}}{\sqrt{{x}}} \\ $$$$=\frac{\mathrm{1}}{{x}^{{n}+\mathrm{1}} \sqrt{{x}}}\:\int_{\mathrm{0}} ^{\sqrt{{x}}} \:\:\:\frac{{u}^{\mathrm{2}{n}+\mathrm{2}} }{{u}^{\mathrm{2}} \:+\mathrm{1}}\:{du}\:\:{and}\:{changement}\:{u}={tan}\theta\:{give} \\ $$$$\int_{\mathrm{0}} ^{\sqrt{{x}}} \:\:\frac{{u}^{\mathrm{2}{n}+\mathrm{2}} }{{u}^{\mathrm{2}} \:+\mathrm{1}}\:{du}\:=\int_{\mathrm{0}} ^{{arctan}\left(\sqrt{{x}}\right)} \:\:\frac{{tan}^{\mathrm{2}{n}+\mathrm{2}} \theta}{\mathrm{1}+{tan}^{\mathrm{2}} \theta}\:\left(\mathrm{1}+{tan}^{\mathrm{2}} \theta\right)\:=\int_{\mathrm{0}} ^{{arctan}\left(\sqrt{{x}}\right)} \:{tan}^{\mathrm{2}{n}+\mathrm{2}} \theta\:{d}\theta \\ $$$${be}\:{continued}... \\ $$

Commented by maxmathsup by imad last updated on 26/Nov/18

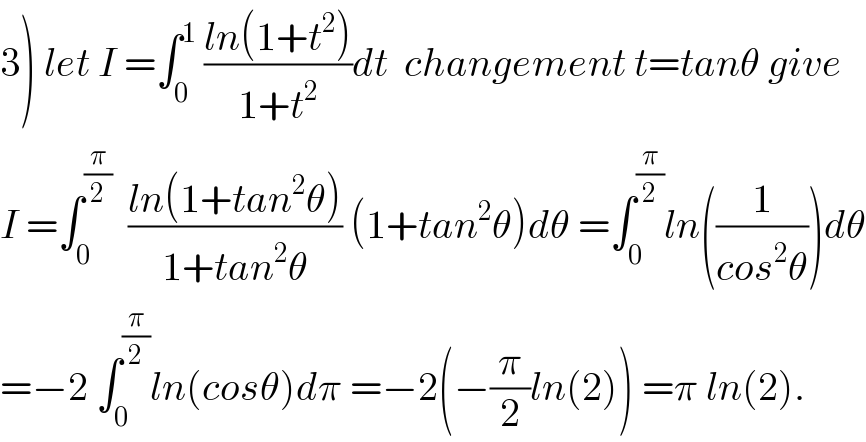
3) let I =∫_0 ^1 ((ln(1+t^2 ))/(1+t^2 ))dt changement t=tanθ give I =∫_0 ^(π/2) ((ln(1+tan^2 θ))/(1+tan^2 θ)) (1+tan^2 θ)dθ =∫_0 ^(π/2) ln((1/(cos^2 θ)))dθ =−2 ∫_0 ^(π/2) ln(cosθ)dπ =−2(−(π/2)ln(2)) =π ln(2).

$$\left.\mathrm{3}\right)\:{let}\:{I}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{ln}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)}{\mathrm{1}+{t}^{\mathrm{2}} }{dt}\:\:{changement}\:{t}={tan}\theta\:{give} \\ $$$${I}\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:\frac{{ln}\left(\mathrm{1}+{tan}^{\mathrm{2}} \theta\right)}{\mathrm{1}+{tan}^{\mathrm{2}} \theta}\:\left(\mathrm{1}+{tan}^{\mathrm{2}} \theta\right){d}\theta\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {ln}\left(\frac{\mathrm{1}}{{cos}^{\mathrm{2}} \theta}\right){d}\theta \\ $$$$=−\mathrm{2}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {ln}\left({cos}\theta\right){d}\pi\:=−\mathrm{2}\left(−\frac{\pi}{\mathrm{2}}{ln}\left(\mathrm{2}\right)\right)\:=\pi\:{ln}\left(\mathrm{2}\right). \\ $$

Commented by maxmathsup by imad last updated on 26/Nov/18

4) ∫_0 ^1 ((ln(1+2t^2 ))/(1+t^2 )) dt =f(2) =2 ∫_0 ^(√2) ((arctan(u))/(1−u^2 )) du...be continued...

$$\left.\mathrm{4}\right)\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{ln}\left(\mathrm{1}+\mathrm{2}{t}^{\mathrm{2}} \right)}{\mathrm{1}+{t}^{\mathrm{2}} }\:{dt}\:={f}\left(\mathrm{2}\right)\:=\mathrm{2}\:\int_{\mathrm{0}} ^{\sqrt{\mathrm{2}}} \:\:\frac{{arctan}\left({u}\right)}{\mathrm{1}−{u}^{\mathrm{2}} }\:{du}...{be}\:{continued}... \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com