Math Question and Answers


Question and Answers Forum

All Questions Topic List
Geometry Questions
Previous in All Question Next in All Question
Previous in Geometry Next in Geometry
Question Number 48522 by ajfour last updated on 25/Nov/18

Commented by ajfour last updated on 25/Nov/18

$A r e g u l a r h e x a g o n a l p y r a m i d$ $w i t h b a s e e d g e a a n d a l t i t u d e h .$ $F i n d t h e a r e a o f a s e c t i o n t h a t$ $p a s s e s t h r o u g h m i d p o i n t s P, Q$ $o f s i d e s A B a n d C D a n d a l s o$ $t h r o u g h M, t h e m i d p o i n t o f$ $a l t i t u d e .$
	Answered by mr W last updated on 25/Nov/18

	Commented by mr W last updated on 25/Nov/18

	$A D = 2 a$ $b_{1} = P Q = (a + 2 a) / 2 = 3 a / 2$ $b_{2} = U R = A D / 2 = a$ $c = \sqrt{{(\sqrt{3} a / 4)}^{2} + {(h / 2)}^{2}} = (\sqrt{3 a^{2} + 4 h^{2}}) / 4$ $\frac{e}{f - h / 2} = \frac{\sqrt{3} a / 4}{h / 2}$ $\frac{e}{h - f} = \frac{\sqrt{3} a / 2}{h}$ $\Rightarrow f - h / 2 = h - f$ $\Rightarrow f = 3 h / 4$ $b_{3} = S T$ $\frac{b_{3}}{a} = \frac{h - f}{h} = 1 / 4$ $\Rightarrow b_{3} = a / 4$ $\frac{d + c}{c} = \frac{f}{h / 2} = \frac{3}{2}$ $\frac{d}{c} = \frac{1}{2}$ $\Rightarrow d = c / 2 = (\sqrt{3 a^{2} + 4 h^{2}}) / 8$ $A r e a o f P Q R S T U P = A$ $A = (b_{1} + b_{2}) c / 2 + (b_{2} + b_{3}) d / 2 = [b_{1} c + b_{2} (c + d) + b_{3} d] / 2$ $= \frac{1}{2} [\frac{3 a}{2} \times \frac{\sqrt{3 a^{2} + 4 h^{2}}}{4} + a \times \frac{3 \sqrt{3 a^{2} + 4 h^{2}}}{8} + \frac{a}{4} \times \frac{\sqrt{3 a^{2} + 4 h^{2}}}{8}]$ $\Rightarrow A = \frac{25 a \sqrt{3 a^{2} + 4 h^{2}}}{64}$
	Commented by ajfour last updated on 25/Nov/18

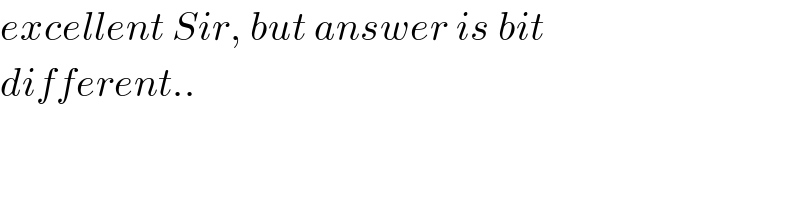
	$e x c e l l e n t S i r, b u t a n s w e r i s b i t$ $d i f f e r e n t . .$
	Commented by mr W last updated on 25/Nov/18

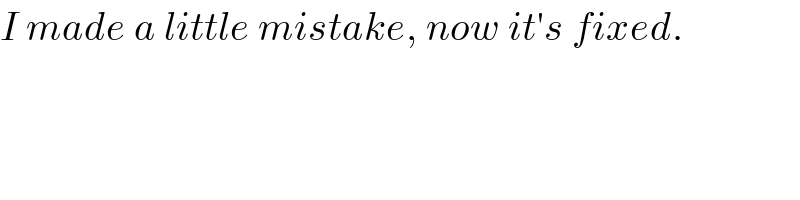
	$I m a d e a l i t t l e m i s t a k e, n o w {i t}^{'} s f i x e d .$
	Commented by ajfour last updated on 25/Nov/18

	$R e a l l y C o r r e c t n o w, a n d b e y o n d$ $a l l a d m i r a t i o n a n d p r a i s e S i r;$ $\frac{b_{3}}{a} = \frac{h - f}{h} (p l e a s e h e l p; h o w ?)$
	Commented by mr W last updated on 26/Nov/18

	$S^{'} = m i d p o i n t o f S a n d T$ $E^{'} = m i d p o i n t o f E a n d F$ $\frac{S T}{E F} = \frac{{V S}^{'}}{{V E}^{'}} = \frac{h - f}{h}$ $\Rightarrow \frac{b_{3}}{a} = \frac{h - f}{h}$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum