1) find f(λ) =∫_0 ^1 (dx/(2+e^(−λx) )) with λ>0 2)calculate ∫_0 ^1 (x/((2+e^(−λx) )^2 ))dx 3) find the value of ∫_0 ^1 (dx/(2 +e^(−x(√3)) ))dx and ∫


Question and Answers Forum

All Questions Topic List
Integration Questions
Previous in All Question Next in All Question
Previous in Integration Next in Integration
Question Number 48715 by Abdo msup. last updated on 27/Nov/18

$1) f i n d f (λ) = \int_{0}^{1} \frac{d x}{2 + e^{- λ x}} w i t h λ > 0$ $2) c a l c u l a t e \int_{0}^{1} \frac{x}{{(2 + e^{- λ x})}^{2}} d x$ $3) f i n d t h e v a l u e o f \int_{0}^{1} \frac{d x}{2 + e^{- x \sqrt{3}}} d x a n d \int_{0}^{1} \frac{x}{{(2 + e^{- x \sqrt{3}})}^{2}} d x$
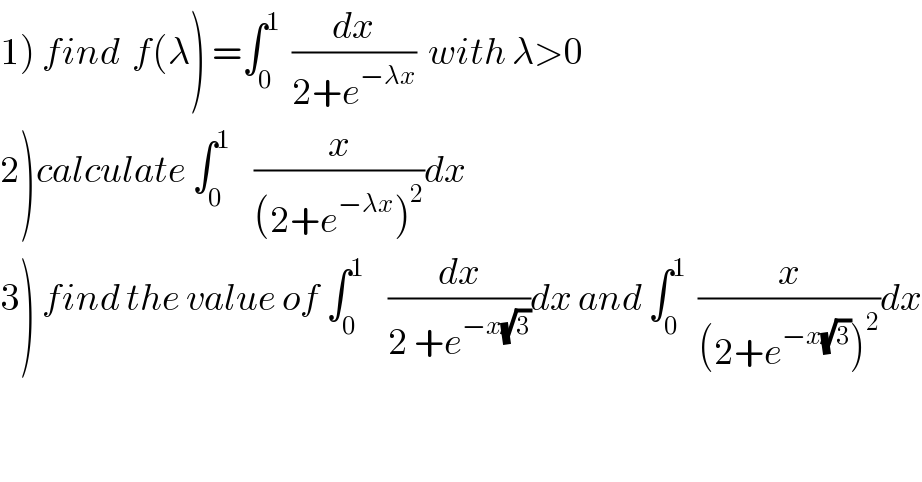
Commented bymaxmathsup by imad last updated on 01/Dec/18
$1) we have f(λ)=∫_0 ^1 (dx/(2+e^(−λx) )) changement e^(λx ) =t give λx=ln(t) ⇒ x=(1/λ)ln(t) ⇒f(λ)=∫_1 ^e^λ (1/(λ(2+t^(−1) ))) (dt/t) =(1/λ) ∫_1 ^e^λ (dt/(2t +1)) =(1/(2λ))[ln(2t+1)]_1 ^e^λ = (1/(2λ)){ln(2e^λ +1)−ln(3)} ⇒ f(λ)=(1/(2λ)){ln(2 e^λ +1)−ln(3)} .$
$1) w e h a v e f (λ) = \int_{0}^{1} \frac{d x}{2 + e^{- λ x}} c h a n g e m e n t e^{λ x} = t g i v e λ x = l n (t) \Rightarrow$ $x = \frac{1}{λ} l n (t) \Rightarrow f (λ) = \int_{1}^{e^{λ}} \frac{1}{λ (2 + t^{- 1})} \frac{d t}{t} = \frac{1}{λ} \int_{1}^{e^{λ}} \frac{d t}{2 t + 1}$ $= \frac{1}{2 λ} {[l n (2 t + 1)]}_{1}^{e^{λ}} = \frac{1}{2 λ} {l n (2 e^{λ} + 1) - l n (3)} \Rightarrow$ $f (λ) = \frac{1}{2 λ} {l n (2 e^{λ} + 1) - l n (3)} .$
Commented bymaxmathsup by imad last updated on 01/Dec/18
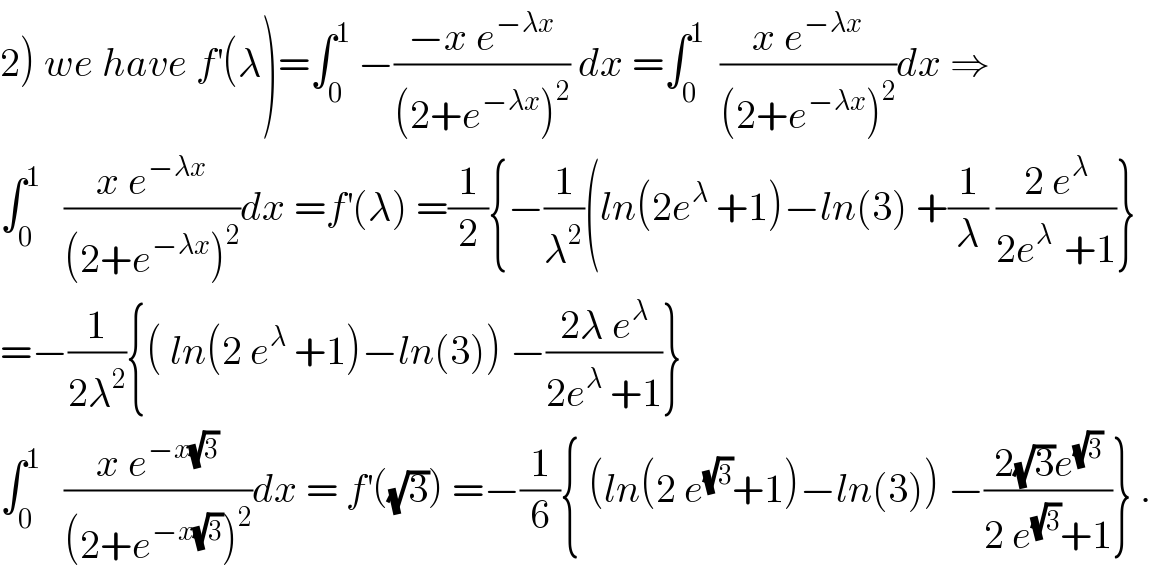
$2) we have f^′ (λ)=∫_0 ^1 −((−x e^(−λx) )/((2+e^(−λx) )^2 )) dx =∫_0 ^1 ((x e^(−λx) )/((2+e^(−λx) )^2 ))dx ⇒ ∫_0 ^1 ((x e^(−λx) )/((2+e^(−λx) )^2 ))dx =f^′ (λ) =(1/2){−(1/λ^2 )(ln(2e^λ +1)−ln(3) +(1/λ) ((2 e^λ )/(2e^(λ ) +1))} =−(1/(2λ^2 )){( ln(2 e^λ +1)−ln(3)) −((2λ e^λ )/(2e^λ +1))} ∫_0 ^1 ((x e^(−x(√3)) )/((2+e^(−x(√3)) )^2 ))dx = f^′ ((√3)) =−(1/6){ (ln(2 e^(√3) +1)−ln(3)) −((2(√3)e^(√3) )/(2 e^(√3) +1))} .$
$2) w e h a v e f^{^{'}} (λ) = \int_{0}^{1} - \frac{- x e^{- λ x}}{{(2 + e^{- λ x})}^{2}} d x = \int_{0}^{1} \frac{x e^{- λ x}}{{(2 + e^{- λ x})}^{2}} d x \Rightarrow$ $\int_{0}^{1} \frac{x e^{- λ x}}{{(2 + e^{- λ x})}^{2}} d x = f^{^{'}} (λ) = \frac{1}{2} {- \frac{1}{λ^{2}} (l n (2 e^{λ} + 1) - l n (3) + \frac{1}{λ} \frac{2 e^{λ}}{2 e^{λ} + 1}}$ $= - \frac{1}{2 λ^{2}} {(l n (2 e^{λ} + 1) - l n (3)) - \frac{2 λ e^{λ}}{2 e^{λ} + 1}}$ $\int_{0}^{1} \frac{x e^{- x \sqrt{3}}}{{(2 + e^{- x \sqrt{3}})}^{2}} d x = f^{^{'}} (\sqrt{3}) = - \frac{1}{6} {(l n (2 e^{\sqrt{3}} + 1) - l n (3)) - \frac{2 \sqrt{3} e^{\sqrt{3}}}{2 e^{\sqrt{3}} + 1}} .$
Commented bymaxmathsup by imad last updated on 01/Dec/18

$2) t h e Q . i s c a l c u l a t e \int_{0}^{1} \frac{x e^{- λ x}}{{(2 + e^{- λ x})}^{2}} d x$
Commented bymaxmathsup by imad last updated on 01/Dec/18
$) we have ∫_0 ^1 (dx/(2+e^(−x(√3)) )) =f((√3)) =(1/(2(√3))){ln(2 e^(√3) +1)−ln(3)}$
$) w e h a v e \int_{0}^{1} \frac{d x}{2 + e^{- x \sqrt{3}}} = f (\sqrt{3}) = \frac{1}{2 \sqrt{3}} {l n (2 e^{\sqrt{3}} + 1) - l n (3)}$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum