
Question Number 48717 by Abdo msup. last updated on 27/Nov/18
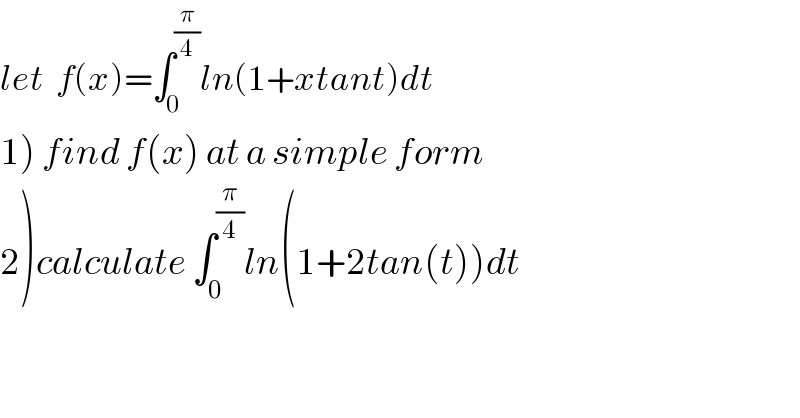
$${let}\:\:{f}\left({x}\right)=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} {ln}\left(\mathrm{1}+{xtant}\right){dt} \\ $$$$\left.\mathrm{1}\right)\:{find}\:{f}\left({x}\right)\:{at}\:{a}\:{simple}\:{form} \\ $$$$\left.\mathrm{2}\right){calculate}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} {ln}\left(\mathrm{1}+\mathrm{2}{tan}\left({t}\right)\right){dt} \\ $$
Commented by maxmathsup by imad last updated on 01/Dec/18
![1) we hsve f^′ (x)= ∫_0 ^(π/4) ((tant)/(1+xtant))dt =(1/x) ∫_0 ^(π/4) ((1+xtant −1)/(1+xtan(t))) dt ( x≠o) =(π/(4x)) −(1/x) ∫_0 ^(π/4) (dt/(1+xtant)) but ∫_0 ^(π/4) (dt/(1+xtant)) =_(tant=u) ∫_0 ^1 (1/(1+xu)) (du/(1+u^2 )) = ∫_0 ^1 (du/((1+u^2 )(1+xu))) let decompose F(u)= (1/((1+xu)(1+u^2 ))) F(u)= (a/(xu +1)) +((bu +c)/(u^2 +1)) a =lim_(u→−(1/x)) (xu+1)F(u) =(1/(1+(1/x^2 ))) =(x^2 /(1+x^2 )) lim_(u→+∞) u F(u) =(a/x) +b =0 ⇒b =−(a/x) =−(x/(1+x^2 )) ⇒ F(u)=(x^2 /((x^2 +1)(xu+1))) +((−(x/(1+x^2 ))u+ c)/(u^2 +1)) F(0)=1 = (x^2 /(x^2 +1)) + c ⇒c=1−(x^2 /(x^2 +1)) =(1/(x^2 +1)) ⇒ F(u)= (x^2 /((x^2 +1)(xu+1))) −(1/(x^2 +1)) ((xu−1)/(u^2 +1)) ⇒ ∫_0 ^1 (du/((1+u^2 )(1+xu))) =(x^2 /((x^2 +1))) ∫_0 ^1 (du/(xu +1)) −(x/(2(x^2 +1))) ∫_0 ^1 ((2u)/(u^2 +1))du +(1/(1+x^2 )) ∫_0 ^1 (du/(1+u^2 )) =(x/(x^2 +1))[ln∣xu+1∣]_(u=0) ^1 −(x/(2(x^2 +1)))[ln(u^2 +1)]_(u=0) ^1 +(1/(1+x^2 ))[arctanu]_(u=0) ^1 =(x/(x^2 +1))ln∣x+1∣ −((xln(2))/(2(x^2 +1))) +(π/(4(1+x^2 ))) ⇒f^′ (x)=(x/(x^2 +1))ln∣x+1∣−((xln(2))/(2(x^2 +1))) +(π/(4(1+x^2 ))) ⇒f(x)= ∫ ((xln∣x+1∣)/(x^2 +1))dx−((ln(2))/4)ln(x^2 +1) +(π/4) arctanx +c if we suppose x>−1 by parts u^′ =(x/(x^2 +1)) and v=ln(x+1) we get ∫ ((x ln(x+1))/(x^2 +1))dx =((xln(x+1))/(2(x^2 +1))) −∫ ((ln(x^2 +1))/(1+x)) dx ....be continued...](Q49050.png)
$$\left.\mathrm{1}\right)\:{we}\:{hsve}\:{f}^{'} \left({x}\right)=\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\frac{{tant}}{\mathrm{1}+{xtant}}{dt}\:=\frac{\mathrm{1}}{{x}}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\frac{\mathrm{1}+{xtant}\:−\mathrm{1}}{\mathrm{1}+{xtan}\left({t}\right)}\:{dt}\:\:\:\left(\:{x}\neq{o}\right) \\ $$$$=\frac{\pi}{\mathrm{4}{x}}\:−\frac{\mathrm{1}}{{x}}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\frac{{dt}}{\mathrm{1}+{xtant}}\:\:{but}\:\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\:\frac{{dt}}{\mathrm{1}+{xtant}}\:=_{{tant}={u}} \:\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\frac{\mathrm{1}}{\mathrm{1}+{xu}}\:\frac{{du}}{\mathrm{1}+{u}^{\mathrm{2}} } \\ $$$$=\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\frac{{du}}{\left(\mathrm{1}+{u}^{\mathrm{2}} \right)\left(\mathrm{1}+{xu}\right)}\:\:{let}\:{decompose}\:{F}\left({u}\right)=\:\frac{\mathrm{1}}{\left(\mathrm{1}+{xu}\right)\left(\mathrm{1}+{u}^{\mathrm{2}} \right)} \\ $$$${F}\left({u}\right)=\:\frac{{a}}{{xu}\:+\mathrm{1}}\:+\frac{{bu}\:+{c}}{{u}^{\mathrm{2}} \:+\mathrm{1}} \\ $$$${a}\:={lim}_{{u}\rightarrow−\frac{\mathrm{1}}{{x}}} \:\:\:\left({xu}+\mathrm{1}\right){F}\left({u}\right)\:=\frac{\mathrm{1}}{\mathrm{1}+\frac{\mathrm{1}}{{x}^{\mathrm{2}} }}\:=\frac{{x}^{\mathrm{2}} }{\mathrm{1}+{x}^{\mathrm{2}} } \\ $$$${lim}_{{u}\rightarrow+\infty} {u}\:{F}\left({u}\right)\:=\frac{{a}}{{x}}\:+{b}\:\:=\mathrm{0}\:\Rightarrow{b}\:=−\frac{{a}}{{x}}\:=−\frac{{x}}{\mathrm{1}+{x}^{\mathrm{2}} }\:\Rightarrow \\ $$$${F}\left({u}\right)=\frac{{x}^{\mathrm{2}} }{\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)\left({xu}+\mathrm{1}\right)}\:+\frac{−\frac{{x}}{\mathrm{1}+{x}^{\mathrm{2}} }{u}+\:{c}}{{u}^{\mathrm{2}} \:+\mathrm{1}} \\ $$$${F}\left(\mathrm{0}\right)=\mathrm{1}\:=\:\frac{{x}^{\mathrm{2}} }{{x}^{\mathrm{2}} \:+\mathrm{1}}\:+\:{c}\:\Rightarrow{c}=\mathrm{1}−\frac{{x}^{\mathrm{2}} }{{x}^{\mathrm{2}} \:+\mathrm{1}}\:=\frac{\mathrm{1}}{{x}^{\mathrm{2}} \:+\mathrm{1}}\:\Rightarrow \\ $$$${F}\left({u}\right)=\:\frac{{x}^{\mathrm{2}} }{\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)\left({xu}+\mathrm{1}\right)}\:−\frac{\mathrm{1}}{{x}^{\mathrm{2}} \:+\mathrm{1}}\:\frac{{xu}−\mathrm{1}}{{u}^{\mathrm{2}} \:+\mathrm{1}}\:\Rightarrow \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\frac{{du}}{\left(\mathrm{1}+{u}^{\mathrm{2}} \right)\left(\mathrm{1}+{xu}\right)}\:=\frac{{x}^{\mathrm{2}} }{\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{du}}{{xu}\:+\mathrm{1}}\:−\frac{{x}}{\mathrm{2}\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{\mathrm{2}{u}}{{u}^{\mathrm{2}} \:+\mathrm{1}}{du}\:+\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{2}} }\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{du}}{\mathrm{1}+{u}^{\mathrm{2}} } \\ $$$$=\frac{{x}}{{x}^{\mathrm{2}} +\mathrm{1}}\left[{ln}\mid{xu}+\mathrm{1}\mid\right]_{{u}=\mathrm{0}} ^{\mathrm{1}} \:−\frac{{x}}{\mathrm{2}\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)}\left[{ln}\left({u}^{\mathrm{2}} \:+\mathrm{1}\right)\right]_{{u}=\mathrm{0}} ^{\mathrm{1}} \:+\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{2}} }\left[{arctanu}\right]_{{u}=\mathrm{0}} ^{\mathrm{1}} \\ $$$$=\frac{{x}}{{x}^{\mathrm{2}} \:+\mathrm{1}}{ln}\mid{x}+\mathrm{1}\mid\:−\frac{{xln}\left(\mathrm{2}\right)}{\mathrm{2}\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)}\:+\frac{\pi}{\mathrm{4}\left(\mathrm{1}+{x}^{\mathrm{2}} \right)}\:\Rightarrow{f}^{'} \left({x}\right)=\frac{{x}}{{x}^{\mathrm{2}} \:+\mathrm{1}}{ln}\mid{x}+\mathrm{1}\mid−\frac{{xln}\left(\mathrm{2}\right)}{\mathrm{2}\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)} \\ $$$$+\frac{\pi}{\mathrm{4}\left(\mathrm{1}+{x}^{\mathrm{2}} \right)}\:\Rightarrow{f}\left({x}\right)=\:\int\:\:\frac{{xln}\mid{x}+\mathrm{1}\mid}{{x}^{\mathrm{2}} \:+\mathrm{1}}{dx}−\frac{{ln}\left(\mathrm{2}\right)}{\mathrm{4}}{ln}\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)\:+\frac{\pi}{\mathrm{4}}\:{arctanx}\:\:+{c}\: \\ $$$${if}\:\:{we}\:{suppose}\:{x}>−\mathrm{1}\:\:\:\:{by}\:{parts}\:{u}^{'} =\frac{{x}}{{x}^{\mathrm{2}} \:+\mathrm{1}}\:\:{and}\:{v}={ln}\left({x}+\mathrm{1}\right)\:{we}\:{get} \\ $$$$\int\:\:\frac{{x}\:{ln}\left({x}+\mathrm{1}\right)}{{x}^{\mathrm{2}} \:+\mathrm{1}}{dx}\:=\frac{{xln}\left({x}+\mathrm{1}\right)}{\mathrm{2}\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)}\:−\int\:\:\frac{{ln}\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)}{\mathrm{1}+{x}}\:{dx}\:....{be}\:{continued}... \\ $$
