Question and Answers Forum

All Questions      Topic List

Others Questions

Previous in All Question      Next in All Question      

Previous in Others      Next in Others      

Question Number 4897 by 123456 last updated on 19/Mar/16

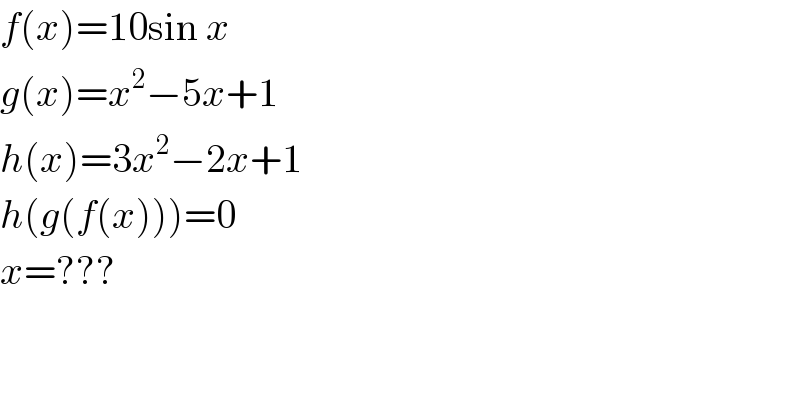
f(x)=10sin x g(x)=x^2 −5x+1 h(x)=3x^2 −2x+1 h(g(f(x)))=0 x=???

$${f}\left({x}\right)=\mathrm{10sin}\:{x} \\ $$$${g}\left({x}\right)={x}^{\mathrm{2}} −\mathrm{5}{x}+\mathrm{1} \\ $$$${h}\left({x}\right)=\mathrm{3}{x}^{\mathrm{2}} −\mathrm{2}{x}+\mathrm{1} \\ $$$${h}\left({g}\left({f}\left({x}\right)\right)\right)=\mathrm{0} \\ $$$${x}=??? \\ $$

Commented by prakash jain last updated on 19/Mar/16

h(x)=3x^2 −2x+1=3(x^2 −(2/3)x+(1/3^2 )−(1/3^2 )+(1/3)) =3((x−(1/3))^2 +(2/9))≥(2/3) for all x∈R h(x)≥(2/3) so no real solution exists

$${h}\left({x}\right)=\mathrm{3}{x}^{\mathrm{2}} −\mathrm{2}{x}+\mathrm{1}=\mathrm{3}\left({x}^{\mathrm{2}} −\frac{\mathrm{2}}{\mathrm{3}}{x}+\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{2}} }−\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{3}}\right) \\ $$$$=\mathrm{3}\left(\left({x}−\frac{\mathrm{1}}{\mathrm{3}}\right)^{\mathrm{2}} +\frac{\mathrm{2}}{\mathrm{9}}\right)\geqslant\frac{\mathrm{2}}{\mathrm{3}}\:\mathrm{for}\:\mathrm{all}\:{x}\in\mathbb{R} \\ $$$${h}\left({x}\right)\geqslant\frac{\mathrm{2}}{\mathrm{3}}\:\mathrm{so}\:\mathrm{no}\:\mathrm{real}\:\mathrm{solution}\:\mathrm{exists} \\ $$

Commented by Yozzii last updated on 23/Mar/16

h(u)=0⇒u=u_r =((2+(−1)^r (√(2^2 −4×3×1)))/6) u_r =((2+(−1)^r 2(√2)i)/6)=(1/3)(1+(−1)^r i(√2)) (r=1,2) ∴ u=g(k)=u_r ∴ k^2 −5k+1−u_r =0 k=k_q =((5+(−1)^q (√(25−4(1−u_r ))))/2) k_q =((5+(−1)^q (√(21+4u_r )))/2) (q=1,2) ∴ f(x)=k_q ⇒sinx=(k_q /(10)) ⇒x=jπ+(−1)^j {sin^(−1) ((k_q /(10)))} x=jπ+(−1)^j {sin^(−1) (((5+(−1)^q (√(21+(4/3)(1+(−1)^r i(√2)))))/(20)))} (q,r=1,2,j∈Z,i=(√(−1))) a+bi=sin^(−1) (c+di) (a,b,c,d∈R) sin(a+bi)=c+di sinacosib+sinbicosa=c+di sinacoshb+icosasinhb=c+di ⇒c=sinacoshb and d=sinhbcosa. (c^2 /(cosh^2 b))+(d^2 /(sinh^2 b))=1 c^2 sinh^2 b+d^2 cosh^2 b=sinh^2 bcosh^2 b c^2 sinh^2 b+d^2 (1+sinh^2 b)=sinh^2 b(1+sinh^2 b) sinh^4 b+sinh^2 b−d^2 sinh^2 b−c^2 sinh^2 b−d^2 =0 sinh^4 b+(1−d^2 −c^2 )sinh^2 b−d^2 =0 ⇒sinh^2 b=((d^2 +c^2 −1±(√((d^2 +c^2 −1)^2 +4d^2 )))/2) ∵ b∈R and d^2 +c^2 −1<(√((d^2 +c^2 −1)^2 +4d^2 )) ⇒sinh^2 b≥0 & ⇒sinh^2 b=((d^2 +c^2 −1+(√((d^2 +c^2 −1)^2 +4d^2 )))/2). b=±sinh^(−1) ((√((d^2 +c^2 −1+(√((d^2 +c^2 −1)^2 +4d^2 )))/2))) cosa=(d/(sinhb))=±(d(√2)/(√(d^2 +c^2 −1+(√((d^2 +c^2 −1)^2 +4d^2 ))))) ⇒a=2nπ±cos^(−1) {((±d(√2))/(√(d^2 +c^2 −1+(√((d^2 +c^2 −1)^2 +4d^2 )))))} (n∈Z) But, ∣cosa∣≤1. ⇒∣d∣(√2)≤(√(d^2 +c^2 −1+(√((d^2 +c^2 −1)^2 +4d^2 )))) 2d^2 ≤d^2 +c^2 −1+(√((d^2 +c^2 −1)^2 +4d^2 )) (d^2 +1−c^2 )^2 ≤(d^2 +c^2 −1)^2 +4d^2 (d^2 +1−c^2 +d^2 +c^2 −1)(d^2 +1−c^2 +1−d^2 −c^2 )≤4d^2 2d^2 ×2(1−c^2 )≤4d^2 d^2 (1−c^2 )−d^2 ≤0 d^2 (1−c^2 −1)≤0 −(cd)^2 ≤0⇒(cd)^2 ≥0 which is true for all c,d∈R. So, a and b∈R always exists for a+bi=sin^(−1) (c+di) if given c,d∈R. This shows that the arcsine of a complex number gives infinitely many complex solutions who share an imaginary part which takes one of two values, but whose real parts have ∣Z∣ many values. The original question has infinitely many complex solutions as Prakash said.

$${h}\left({u}\right)=\mathrm{0}\Rightarrow{u}={u}_{{r}} =\frac{\mathrm{2}+\left(−\mathrm{1}\right)^{{r}} \sqrt{\mathrm{2}^{\mathrm{2}} −\mathrm{4}×\mathrm{3}×\mathrm{1}}}{\mathrm{6}} \\ $$$${u}_{{r}} =\frac{\mathrm{2}+\left(−\mathrm{1}\right)^{{r}} \mathrm{2}\sqrt{\mathrm{2}}{i}}{\mathrm{6}}=\frac{\mathrm{1}}{\mathrm{3}}\left(\mathrm{1}+\left(−\mathrm{1}\right)^{{r}} {i}\sqrt{\mathrm{2}}\right)\:\left({r}=\mathrm{1},\mathrm{2}\right) \\ $$$$\therefore\:{u}={g}\left({k}\right)={u}_{{r}} \\ $$$$\therefore\:{k}^{\mathrm{2}} −\mathrm{5}{k}+\mathrm{1}−{u}_{{r}} =\mathrm{0} \\ $$$${k}={k}_{{q}} =\frac{\mathrm{5}+\left(−\mathrm{1}\right)^{{q}} \sqrt{\mathrm{25}−\mathrm{4}\left(\mathrm{1}−{u}_{{r}} \right)}}{\mathrm{2}} \\ $$$${k}_{{q}} =\frac{\mathrm{5}+\left(−\mathrm{1}\right)^{{q}} \sqrt{\mathrm{21}+\mathrm{4}{u}_{{r}} }}{\mathrm{2}}\:\:\:\:\left({q}=\mathrm{1},\mathrm{2}\right) \\ $$$$\therefore\:{f}\left({x}\right)={k}_{{q}} \Rightarrow{sinx}=\frac{{k}_{{q}} }{\mathrm{10}} \\ $$$$\Rightarrow{x}={j}\pi+\left(−\mathrm{1}\right)^{{j}} \left\{{sin}^{−\mathrm{1}} \left(\frac{{k}_{{q}} }{\mathrm{10}}\right)\right\}\:\: \\ $$$${x}={j}\pi+\left(−\mathrm{1}\right)^{{j}} \left\{{sin}^{−\mathrm{1}} \left(\frac{\mathrm{5}+\left(−\mathrm{1}\right)^{{q}} \sqrt{\mathrm{21}+\frac{\mathrm{4}}{\mathrm{3}}\left(\mathrm{1}+\left(−\mathrm{1}\right)^{{r}} {i}\sqrt{\mathrm{2}}\right)}}{\mathrm{20}}\right)\right\} \\ $$$$\:\:\:\:\:\:\:\:\left({q},{r}=\mathrm{1},\mathrm{2},{j}\in\mathbb{Z},{i}=\sqrt{−\mathrm{1}}\right) \\ $$$${a}+{bi}={sin}^{−\mathrm{1}} \left({c}+{di}\right)\:\:\left({a},{b},{c},{d}\in\mathbb{R}\right) \\ $$$${sin}\left({a}+{bi}\right)={c}+{di} \\ $$$${sinacosib}+{sinbicosa}={c}+{di} \\ $$$${sinacoshb}+{icosasinhb}={c}+{di} \\ $$$$\Rightarrow{c}={sinacoshb}\:{and}\:{d}={sinhbcosa}. \\ $$$$\frac{{c}^{\mathrm{2}} }{{cosh}^{\mathrm{2}} {b}}+\frac{{d}^{\mathrm{2}} }{{sinh}^{\mathrm{2}} {b}}=\mathrm{1} \\ $$$${c}^{\mathrm{2}} {sinh}^{\mathrm{2}} {b}+{d}^{\mathrm{2}} {cosh}^{\mathrm{2}} {b}={sinh}^{\mathrm{2}} {bcosh}^{\mathrm{2}} {b} \\ $$$${c}^{\mathrm{2}} {sinh}^{\mathrm{2}} {b}+{d}^{\mathrm{2}} \left(\mathrm{1}+{sinh}^{\mathrm{2}} {b}\right)={sinh}^{\mathrm{2}} {b}\left(\mathrm{1}+{sinh}^{\mathrm{2}} {b}\right) \\ $$$${sinh}^{\mathrm{4}} {b}+{sinh}^{\mathrm{2}} {b}−{d}^{\mathrm{2}} {sinh}^{\mathrm{2}} {b}−{c}^{\mathrm{2}} {sinh}^{\mathrm{2}} {b}−{d}^{\mathrm{2}} =\mathrm{0} \\ $$$${sinh}^{\mathrm{4}} {b}+\left(\mathrm{1}−{d}^{\mathrm{2}} −{c}^{\mathrm{2}} \right){sinh}^{\mathrm{2}} {b}−{d}^{\mathrm{2}} =\mathrm{0} \\ $$$$\Rightarrow{sinh}^{\mathrm{2}} {b}=\frac{{d}^{\mathrm{2}} +{c}^{\mathrm{2}} −\mathrm{1}\pm\sqrt{\left({d}^{\mathrm{2}} +{c}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} +\mathrm{4}{d}^{\mathrm{2}} }}{\mathrm{2}} \\ $$$$\because\:{b}\in\mathbb{R}\:{and}\:{d}^{\mathrm{2}} +{c}^{\mathrm{2}} −\mathrm{1}<\sqrt{\left({d}^{\mathrm{2}} +{c}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} +\mathrm{4}{d}^{\mathrm{2}} }\:\Rightarrow{sinh}^{\mathrm{2}} {b}\geqslant\mathrm{0} \\ $$$$\&\:\Rightarrow{sinh}^{\mathrm{2}} {b}=\frac{{d}^{\mathrm{2}} +{c}^{\mathrm{2}} −\mathrm{1}+\sqrt{\left({d}^{\mathrm{2}} +{c}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} +\mathrm{4}{d}^{\mathrm{2}} }}{\mathrm{2}}. \\ $$$${b}=\pm{sinh}^{−\mathrm{1}} \left(\sqrt{\frac{{d}^{\mathrm{2}} +{c}^{\mathrm{2}} −\mathrm{1}+\sqrt{\left({d}^{\mathrm{2}} +{c}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} +\mathrm{4}{d}^{\mathrm{2}} }}{\mathrm{2}}}\right) \\ $$$${cosa}=\frac{{d}}{{sinhb}}=\pm\frac{{d}\sqrt{\mathrm{2}}}{\sqrt{{d}^{\mathrm{2}} +{c}^{\mathrm{2}} −\mathrm{1}+\sqrt{\left({d}^{\mathrm{2}} +{c}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} +\mathrm{4}{d}^{\mathrm{2}} }}} \\ $$$$\Rightarrow{a}=\mathrm{2}{n}\pi\pm{cos}^{−\mathrm{1}} \left\{\frac{\pm{d}\sqrt{\mathrm{2}}}{\sqrt{{d}^{\mathrm{2}} +{c}^{\mathrm{2}} −\mathrm{1}+\sqrt{\left({d}^{\mathrm{2}} +{c}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} +\mathrm{4}{d}^{\mathrm{2}} }}}\right\}\:\left({n}\in\mathbb{Z}\right) \\ $$$${But},\:\mid{cosa}\mid\leqslant\mathrm{1}. \\ $$$$\Rightarrow\mid{d}\mid\sqrt{\mathrm{2}}\leqslant\sqrt{{d}^{\mathrm{2}} +{c}^{\mathrm{2}} −\mathrm{1}+\sqrt{\left({d}^{\mathrm{2}} +{c}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} +\mathrm{4}{d}^{\mathrm{2}} }} \\ $$$$\mathrm{2}{d}^{\mathrm{2}} \leqslant{d}^{\mathrm{2}} +{c}^{\mathrm{2}} −\mathrm{1}+\sqrt{\left({d}^{\mathrm{2}} +{c}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} +\mathrm{4}{d}^{\mathrm{2}} } \\ $$$$\left({d}^{\mathrm{2}} +\mathrm{1}−{c}^{\mathrm{2}} \right)^{\mathrm{2}} \leqslant\left({d}^{\mathrm{2}} +{c}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} +\mathrm{4}{d}^{\mathrm{2}} \\ $$$$\left({d}^{\mathrm{2}} +\mathrm{1}−{c}^{\mathrm{2}} +{d}^{\mathrm{2}} +{c}^{\mathrm{2}} −\mathrm{1}\right)\left({d}^{\mathrm{2}} +\mathrm{1}−{c}^{\mathrm{2}} +\mathrm{1}−{d}^{\mathrm{2}} −{c}^{\mathrm{2}} \right)\leqslant\mathrm{4}{d}^{\mathrm{2}} \\ $$$$\mathrm{2}{d}^{\mathrm{2}} ×\mathrm{2}\left(\mathrm{1}−{c}^{\mathrm{2}} \right)\leqslant\mathrm{4}{d}^{\mathrm{2}} \\ $$$${d}^{\mathrm{2}} \left(\mathrm{1}−{c}^{\mathrm{2}} \right)−{d}^{\mathrm{2}} \leqslant\mathrm{0} \\ $$$${d}^{\mathrm{2}} \left(\mathrm{1}−{c}^{\mathrm{2}} −\mathrm{1}\right)\leqslant\mathrm{0} \\ $$$$−\left({cd}\right)^{\mathrm{2}} \leqslant\mathrm{0}\Rightarrow\left({cd}\right)^{\mathrm{2}} \geqslant\mathrm{0}\:{which}\:{is}\:{true}\:{for} \\ $$$${all}\:{c},{d}\in\mathbb{R}.\:{So},\:{a}\:{and}\:{b}\in\mathbb{R}\:{always}\:{exists}\:{for} \\ $$$${a}+{bi}={sin}^{−\mathrm{1}} \left({c}+{di}\right)\:{if}\:{given}\:{c},{d}\in\mathbb{R}. \\ $$$${This}\:{shows}\:{that}\:{the}\:{arcsine}\:{of}\:{a}\: \\ $$$${complex}\:{number}\:{gives}\:{infinitely} \\ $$$${many}\:{complex}\:{solutions}\:{who}\:{share} \\ $$$${an}\:{imaginary}\:{part}\:{which}\:{takes}\:{one} \\ $$$${of}\:{two}\:{values},\:{but}\:{whose}\:{real}\:{parts} \\ $$$${have}\:\mid\mathbb{Z}\mid\:{many}\:{values}.\:{The}\:{original} \\ $$$${question}\:{has}\:{infinitely}\:{many}\:{complex} \\ $$$${solutions}\:{as}\:{Prakash}\:{said}. \\ $$

Answered by prakash jain last updated on 19/Mar/16

h(x)=0⇒x=((2±(√(4−16)))/6)=((2±(√(−12)))/6)=(1/3)(1±i(√3)) g(x)=1±i(√3) for all x∈R, g(x)∈R so no real solution exists.

$${h}\left({x}\right)=\mathrm{0}\Rightarrow{x}=\frac{\mathrm{2}\pm\sqrt{\mathrm{4}−\mathrm{16}}}{\mathrm{6}}=\frac{\mathrm{2}\pm\sqrt{−\mathrm{12}}}{\mathrm{6}}=\frac{\mathrm{1}}{\mathrm{3}}\left(\mathrm{1}\pm{i}\sqrt{\mathrm{3}}\right) \\ $$$${g}\left({x}\right)=\mathrm{1}\pm{i}\sqrt{\mathrm{3}} \\ $$$${for}\:{all}\:{x}\in\mathbb{R},\:{g}\left({x}\right)\in\mathbb{R} \\ $$$${so}\:{no}\:{real}\:{solution}\:{exists}. \\ $$

Commented by prakash jain last updated on 19/Mar/16

Infinite number of complex solution will exists solved by Yozzi for earlier question. In this case sin x is periodic function.

$$\mathrm{Infinite}\:\mathrm{number}\:\mathrm{of}\:\mathrm{complex}\:\mathrm{solution}\:\mathrm{will} \\ $$$$\mathrm{exists}\:\mathrm{solved}\:\mathrm{by}\:\mathrm{Yozzi}\:\mathrm{for}\:\mathrm{earlier}\:\mathrm{question}. \\ $$$$\mathrm{In}\:\mathrm{this}\:\mathrm{case}\:\mathrm{sin}\:{x}\:\mathrm{is}\:\mathrm{periodic}\:\mathrm{function}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com