
Question and Answers Forum
Question Number 49061 by Pk1167156@gmail.com last updated on 02/Dec/18
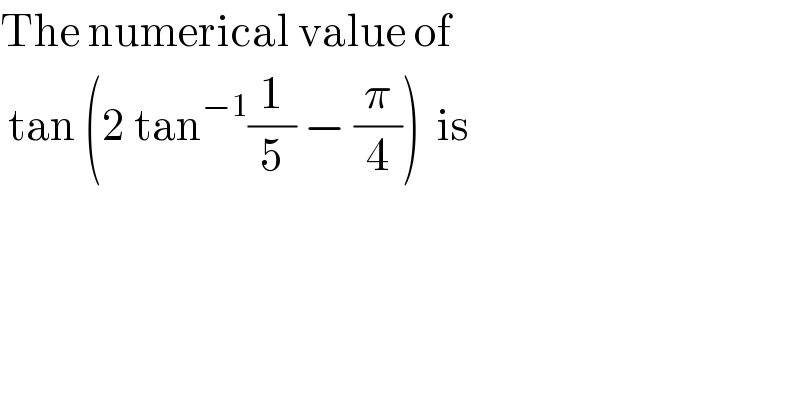
Answered by tanmay.chaudhury50@gmail.com last updated on 02/Dec/18
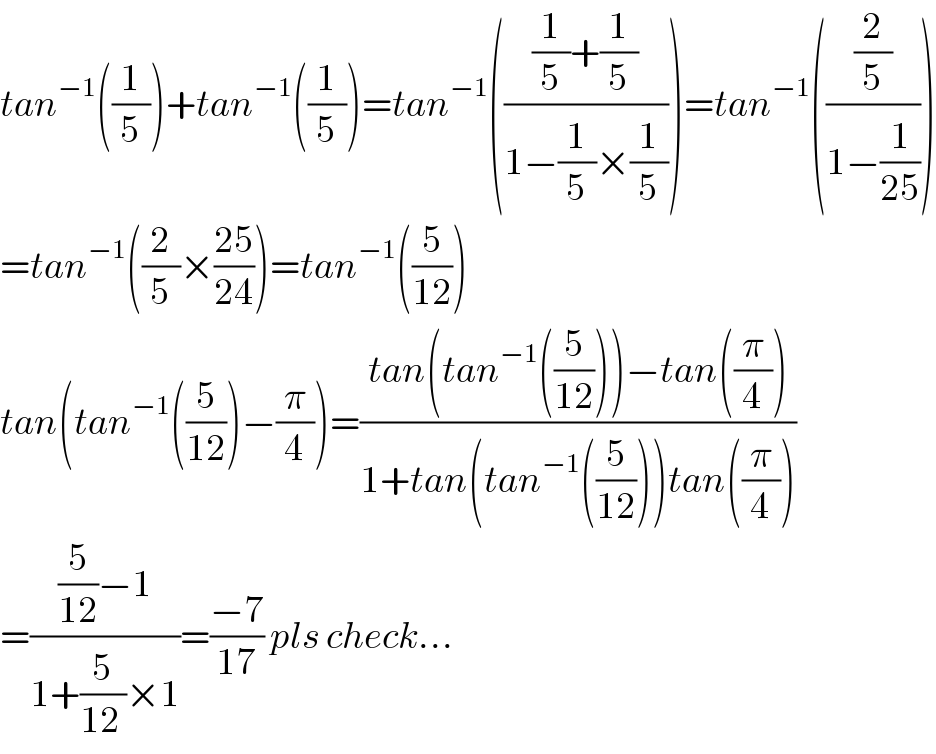
Answered by hknkrc46 last updated on 02/Dec/18
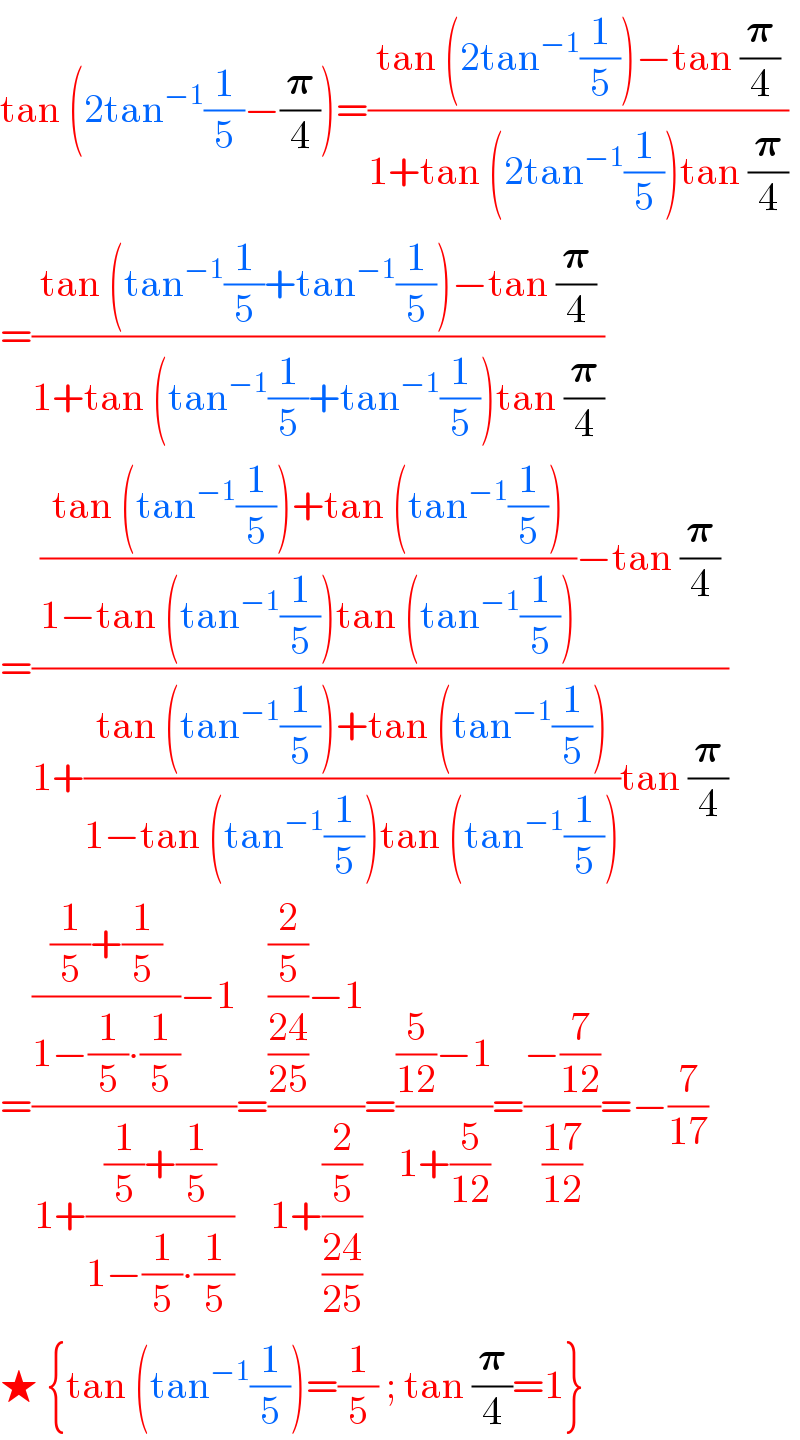
| ||
Question and Answers Forum | ||
Question Number 49061 by Pk1167156@gmail.com last updated on 02/Dec/18 | ||
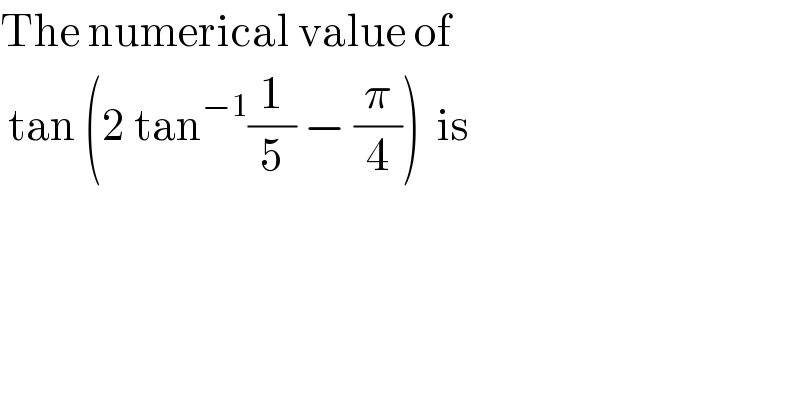 | ||
Answered by tanmay.chaudhury50@gmail.com last updated on 02/Dec/18 | ||
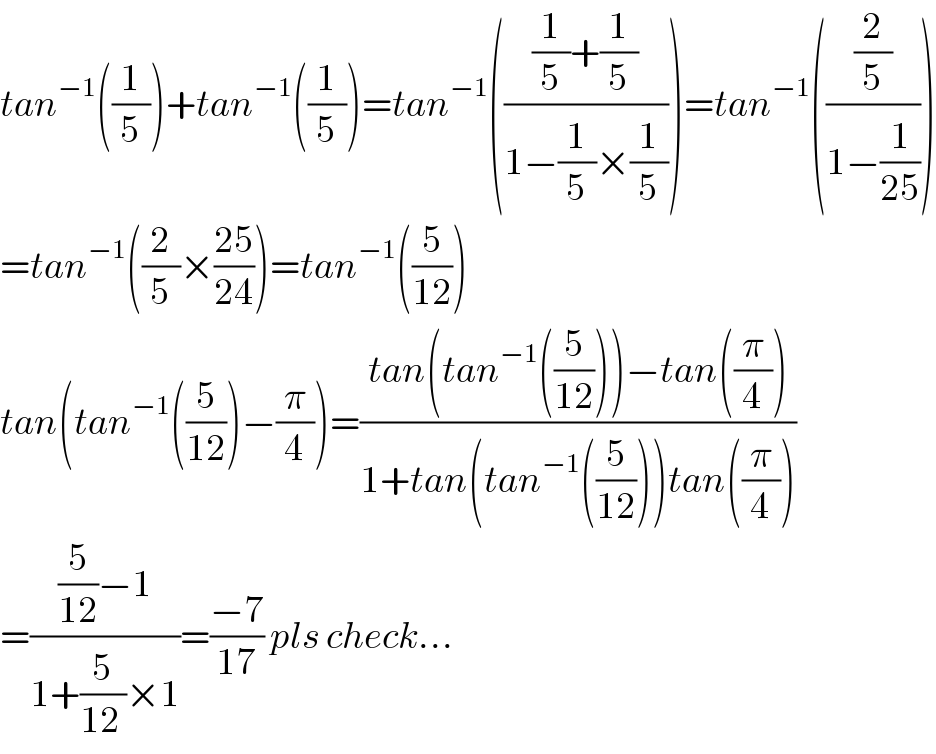 | ||
| ||
Answered by hknkrc46 last updated on 02/Dec/18 | ||
 | ||
| ||