Question Number 49357 by peter frank last updated on 06/Dec/18
Commented by maxmathsup by imad last updated on 01/Jan/19
$$\left.\mathrm{2}\right)\:{let}\:{prove}\:{that}\:{y}^{\left({n}\right)} \left({x}\right)=\frac{\left(−\mathrm{1}\right)^{{n}−\mathrm{1}} \left({n}−\mathrm{1}\right)!}{\left(\mathrm{1}+{x}\right)^{{n}} }\:\:{with}\:{y}\left({x}\right)={ln}\left(\mathrm{1}+{x}\right) \\ $$$${by}\:{recurrence}\:{on}\:{n}\:\:{for}\:{n}=\mathrm{1}\:\:\:{y}^{\left(\mathrm{1}\right)} \left({x}\right)=\frac{\mathrm{1}}{\mathrm{1}+{x}}\:=\:\frac{\left(−\mathrm{1}\right)^{\mathrm{1}−\mathrm{1}} \mathrm{0}!}{\left(\mathrm{1}+{x}\right)^{\mathrm{1}} }\:\:\left({true}\right)\:{let}\:{suppose} \\ $$$${y}^{\left({n}\right)} \left({x}\right)\:=\frac{\left(−\mathrm{1}\right)^{{n}−\mathrm{1}} \left({n}−\mathrm{1}\right)!}{\left(\mathrm{1}+{x}\right)^{{n}} }\:\:{we}\:{have} \\ $$$${y}^{\left({n}+\mathrm{1}\right)} \left({x}\right)\:=\frac{{d}}{{dx}}\left({y}^{\left({n}\right)} \left({x}\right)\right)=\left(−\mathrm{1}\right)^{{n}−\mathrm{1}} \left({n}−\mathrm{1}\right)!×\frac{−{n}\left(\mathrm{1}+{x}\right)^{{n}−\mathrm{1}} }{\left(\mathrm{1}+{x}\right)^{\mathrm{2}{n}} } \\ $$$$=\left(−\mathrm{1}\right)^{{n}} {n}!\:\:\:\frac{\mathrm{1}}{\left(\mathrm{1}+{x}\right)^{{n}+\mathrm{1}} }\:=\frac{\left(−\mathrm{1}\right)^{{n}+\mathrm{1}−\mathrm{1}} \left({n}+\mathrm{1}−\mathrm{1}\right)!}{\left(\mathrm{1}+{x}\right)^{{n}+\mathrm{1}} }\:\:{so}\:{the}\:{result}\:{is}\:{true}\:{at}\:{term} \\ $$$$\left({n}+\mathrm{1}\right). \\ $$
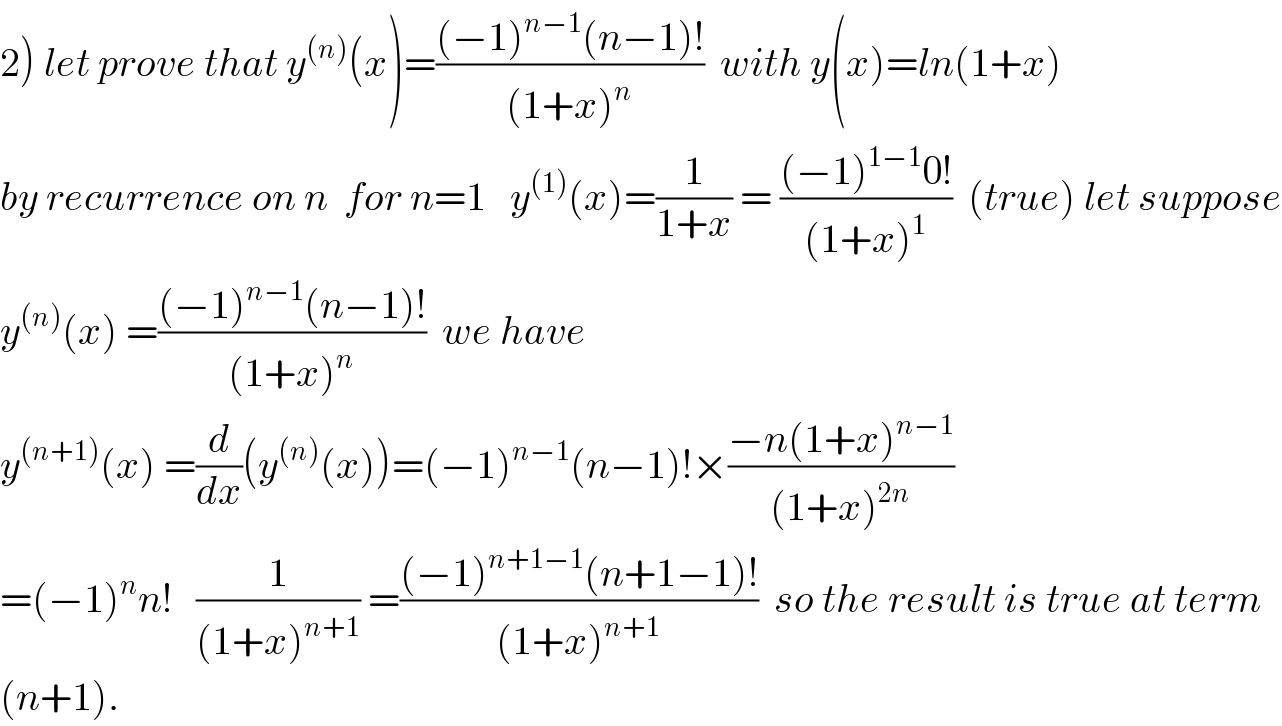
Answered by tanmay.chaudhury50@gmail.com last updated on 06/Dec/18
![1)(d^2 x/dt^2 )+k(dx/dt)+n^2 x=pcoswt step1 (d^2 x/dt^2 )+k(dx/dt)+n^2 x=0 let x=e^(αt) so (dx/dt)=αe^(αt) and (d^2 x/dt^2 )=α^2 e^(αt) e^(αt) (α^2 +kα+n^2 )=0 e^(αt) ≠0 α^2 +kα+n^2 =0 α=((−k±(√(k^2 −4n^2 )))/2) Complementary function x=Ae^((((−k+(√(k^2 −4n^2 )))/2))t) +Be^((((−k−(√(k^2 −4n^2 )) )/2))^ t) step 2 determination of particular intregal (d^2 x/dt^2 )+k(dx/dt)+n^2 x=pcoswt let (d/dt)=D and (d^2 /dx^2 )=D^2 so (D^2 +kD+n^2 )x=pcoswt x=(1/(D^2 +n^2 +kD))×pcoswt =(((D^2 +n^2 −kD))/((D^2 +n^2 )^2 −k^2 D^2 ))pcoswt D(pcoswt)=−wpsinwt D^2 (pcoswt)=−w^2 pcoswt =(((−w^2 pcoswt+n^2 pcoswt+kpwsinwt))/((−w^2 +n^2 )^2 −k^2 (−w^2 ))) =p×[(((n^2 −w^2 )coswt+kwsinwt)/((n^2 −w^2 )^2 +k^2 w^2 ))]](Q49403.png)
$$\left.\mathrm{1}\right)\frac{{d}^{\mathrm{2}} {x}}{{dt}^{\mathrm{2}} }+{k}\frac{{dx}}{{dt}}+{n}^{\mathrm{2}} {x}={pcoswt} \\ $$$${step}\mathrm{1} \\ $$$$\frac{{d}^{\mathrm{2}} {x}}{{dt}^{\mathrm{2}} }+{k}\frac{{dx}}{{dt}}+{n}^{\mathrm{2}} {x}=\mathrm{0} \\ $$$${let}\:{x}={e}^{\alpha{t}} \:\:{so}\:\frac{{dx}}{{dt}}=\alpha{e}^{\alpha{t}} \:\:\:{and}\:\:\frac{{d}^{\mathrm{2}} {x}}{{dt}^{\mathrm{2}} }=\alpha^{\mathrm{2}} {e}^{\alpha{t}} \\ $$$${e}^{\alpha{t}} \left(\alpha^{\mathrm{2}} +{k}\alpha+{n}^{\mathrm{2}} \right)=\mathrm{0}\:\:{e}^{\alpha{t}} \neq\mathrm{0} \\ $$$$\alpha^{\mathrm{2}} +{k}\alpha+{n}^{\mathrm{2}} =\mathrm{0} \\ $$$$\alpha=\frac{−{k}\pm\sqrt{{k}^{\mathrm{2}} −\mathrm{4}{n}^{\mathrm{2}} }}{\mathrm{2}} \\ $$$${Complementary}\:{function} \\ $$$${x}={Ae}^{\left(\frac{−{k}+\sqrt{{k}^{\mathrm{2}} −\mathrm{4}{n}^{\mathrm{2}} }}{\mathrm{2}}\right){t}} +{Be}^{\left(\frac{−{k}−\sqrt{{k}^{\mathrm{2}} −\mathrm{4}{n}^{\mathrm{2}} }\:}{\mathrm{2}}\right)^{} {t}} \\ $$$${step}\:\mathrm{2} \\ $$$${determination}\:{of}\:{particular}\:{intregal} \\ $$$$\frac{{d}^{\mathrm{2}} {x}}{{dt}^{\mathrm{2}} }+{k}\frac{{dx}}{{dt}}+{n}^{\mathrm{2}} {x}={pcoswt} \\ $$$${let}\:\frac{{d}}{{dt}}={D}\:\:{and}\:\frac{{d}^{\mathrm{2}} }{{dx}^{\mathrm{2}} }={D}^{\mathrm{2}} \\ $$$${so}\:\left({D}^{\mathrm{2}} +{kD}+{n}^{\mathrm{2}} \right){x}={pcoswt} \\ $$$${x}=\frac{\mathrm{1}}{{D}^{\mathrm{2}} +{n}^{\mathrm{2}} +{kD}}×{pcoswt} \\ $$$$=\frac{\left({D}^{\mathrm{2}} +{n}^{\mathrm{2}} −{kD}\right)}{\left({D}^{\mathrm{2}} +{n}^{\mathrm{2}} \right)^{\mathrm{2}} −{k}^{\mathrm{2}} {D}^{\mathrm{2}} }{pcoswt} \\ $$$${D}\left({pcoswt}\right)=−{wpsinwt} \\ $$$${D}^{\mathrm{2}} \left({pcoswt}\right)=−{w}^{\mathrm{2}} {pcoswt} \\ $$$$=\frac{\left(−{w}^{\mathrm{2}} {pcoswt}+{n}^{\mathrm{2}} {pcoswt}+{kpwsinwt}\right)}{\left(−{w}^{\mathrm{2}} +{n}^{\mathrm{2}} \right)^{\mathrm{2}} −{k}^{\mathrm{2}} \left(−{w}^{\mathrm{2}} \right)} \\ $$$$={p}×\left[\frac{\left({n}^{\mathrm{2}} −{w}^{\mathrm{2}} \right){coswt}+{kwsinwt}}{\left({n}^{\mathrm{2}} −{w}^{\mathrm{2}} \right)^{\mathrm{2}} +{k}^{\mathrm{2}} {w}^{\mathrm{2}} }\right] \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Answered by tanmay.chaudhury50@gmail.com last updated on 06/Dec/18
![2)y=ln(1+x) (dy/dx)=(1/((1+x))) (d^2 y/dx^2 )=((−1)/((1+x)^2 )) so when n=2 LHS=((−1)/((1+x)^2 )) and RHS=(((−1)^(2−1) (2−1)!)/((1+x)^2 ))=((−1)/((1+x)^2 )) so for n=2 the statement is true now let assume for n=k the statement is true we have to prove that it is true for n=k+1 (d^k y/dx^k )=(((−1)^(k−1) (k−1)!)/((1+x)^k )) so (d/dx)((d^k y/dx^k ))=(d^(k+1) y/dx^(k+1) )←LHS (d/dx)[(((−1)^(k−1) (k−1)!)/((1+x)^k ))] =(((−1)^(k−1) (k−1)!)/1)×(d/dx){(1/((1+x)^k ))} =(−1)^(k−1) (k−1)!×(−k)×(1+x)^(−k−1) =(−1)^(k−1) (k−1)!(−1)k×(1/((1+x)^(k+1) )) =(−1)^(k−1+1) ×k(k−1)!×(1/((1+x)^(k+1) )) =(((−1)^k ×k!)/((1+x)^(k+1) ))←this expression is same if you put n=k+1 in expression (((−1)^(n−1) ×(n−1)!)/((1+x)^n )) hence proved...](Q49404.png)
$$\left.\mathrm{2}\right){y}={ln}\left(\mathrm{1}+{x}\right) \\ $$$$\frac{{dy}}{{dx}}=\frac{\mathrm{1}}{\left(\mathrm{1}+{x}\right)}\:\:\:\:\frac{{d}^{\mathrm{2}} {y}}{{dx}^{\mathrm{2}} }=\frac{−\mathrm{1}}{\left(\mathrm{1}+{x}\right)^{\mathrm{2}} } \\ $$$${so}\:\:{when}\:{n}=\mathrm{2} \\ $$$${LHS}=\frac{−\mathrm{1}}{\left(\mathrm{1}+{x}\right)^{\mathrm{2}} }\:\:\:{and}\:{RHS}=\frac{\left(−\mathrm{1}\right)^{\mathrm{2}−\mathrm{1}} \left(\mathrm{2}−\mathrm{1}\right)!}{\left(\mathrm{1}+{x}\right)^{\mathrm{2}} }=\frac{−\mathrm{1}}{\left(\mathrm{1}+{x}\right)^{\mathrm{2}} } \\ $$$${so}\:{for}\:{n}=\mathrm{2}\:{the}\:{statement}\:{is}\:{true}\: \\ $$$${now}\:{let}\:{assume}\:{for}\:{n}={k}\:{the}\:{statement}\:{is}\:{true} \\ $$$${we}\:{have}\:{to}\:{prove}\:{that}\:{it}\:{is}\:{true}\:{for}\:{n}={k}+\mathrm{1} \\ $$$$\frac{{d}^{{k}} {y}}{{dx}^{{k}} }=\frac{\left(−\mathrm{1}\right)^{{k}−\mathrm{1}} \left({k}−\mathrm{1}\right)!}{\left(\mathrm{1}+{x}\right)^{{k}} } \\ $$$${so}\:\frac{{d}}{{dx}}\left(\frac{{d}^{{k}} {y}}{{dx}^{{k}} }\right)=\frac{{d}^{{k}+\mathrm{1}} {y}}{{dx}^{{k}+\mathrm{1}} }\leftarrow{LHS} \\ $$$$\frac{{d}}{{dx}}\left[\frac{\left(−\mathrm{1}\right)^{{k}−\mathrm{1}} \left({k}−\mathrm{1}\right)!}{\left(\mathrm{1}+{x}\right)^{{k}} }\right] \\ $$$$=\frac{\left(−\mathrm{1}\right)^{{k}−\mathrm{1}} \left({k}−\mathrm{1}\right)!}{\mathrm{1}}×\frac{{d}}{{dx}}\left\{\frac{\mathrm{1}}{\left(\mathrm{1}+{x}\right)^{{k}} }\right\} \\ $$$$=\left(−\mathrm{1}\right)^{{k}−\mathrm{1}} \left({k}−\mathrm{1}\right)!×\left(−{k}\right)×\left(\mathrm{1}+{x}\right)^{−{k}−\mathrm{1}} \\ $$$$=\left(−\mathrm{1}\right)^{{k}−\mathrm{1}} \left({k}−\mathrm{1}\right)!\left(−\mathrm{1}\right){k}×\frac{\mathrm{1}}{\left(\mathrm{1}+{x}\right)^{{k}+\mathrm{1}} } \\ $$$$=\left(−\mathrm{1}\right)^{{k}−\mathrm{1}+\mathrm{1}} ×{k}\left({k}−\mathrm{1}\right)!×\frac{\mathrm{1}}{\left(\mathrm{1}+{x}\right)^{{k}+\mathrm{1}} } \\ $$$$=\frac{\left(−\mathrm{1}\right)^{{k}} ×{k}!}{\left(\mathrm{1}+{x}\right)^{{k}+\mathrm{1}} }\leftarrow{this}\:{expression}\:{is}\:{same}\:{if}\:{you} \\ $$$${put}\:{n}={k}+\mathrm{1}\:{in}\:{expression} \\ $$$$\:\frac{\left(−\mathrm{1}\right)^{{n}−\mathrm{1}} ×\left({n}−\mathrm{1}\right)!}{\left(\mathrm{1}+{x}\right)^{{n}} } \\ $$$${hence}\:{proved}... \\ $$