
Question and Answers Forum
Question Number 49367 by behi83417@gmail.com last updated on 06/Dec/18
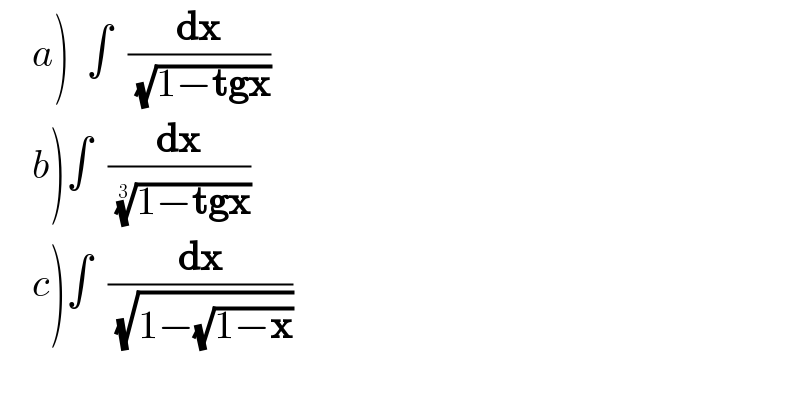
Commented by behi83417@gmail.com last updated on 06/Dec/18

Commented by maxmathsup by imad last updated on 06/Dec/18
Commented by maxmathsup by imad last updated on 06/Dec/18
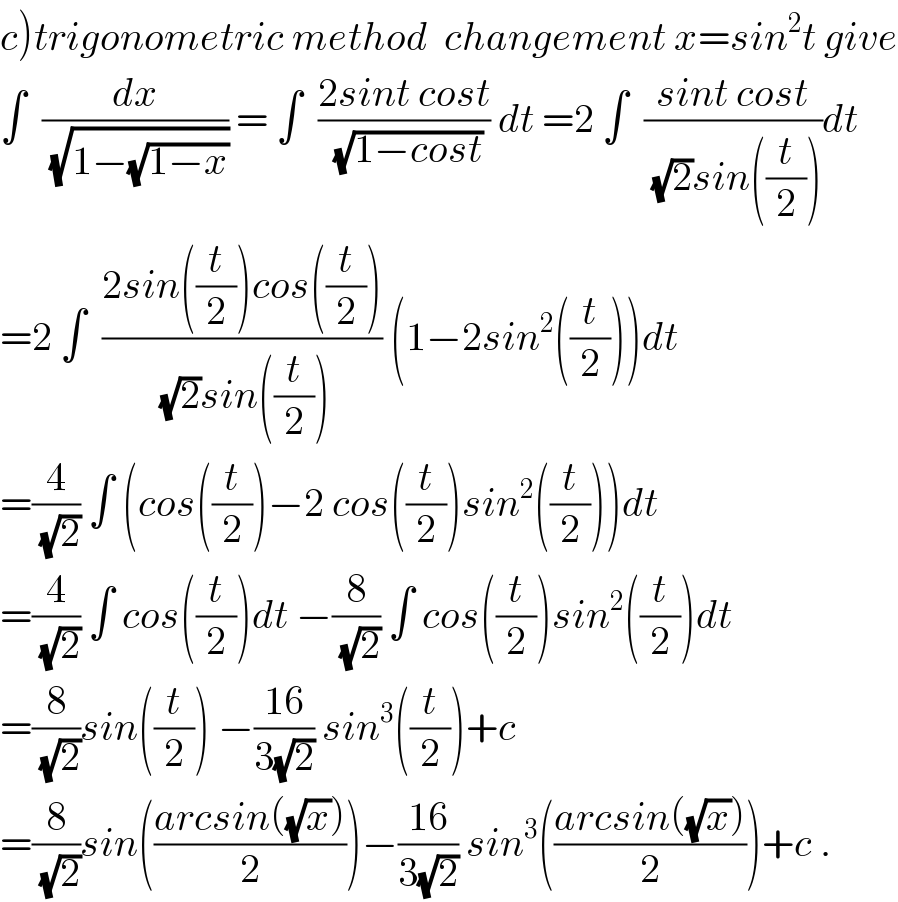
Commented by maxmathsup by imad last updated on 06/Dec/18
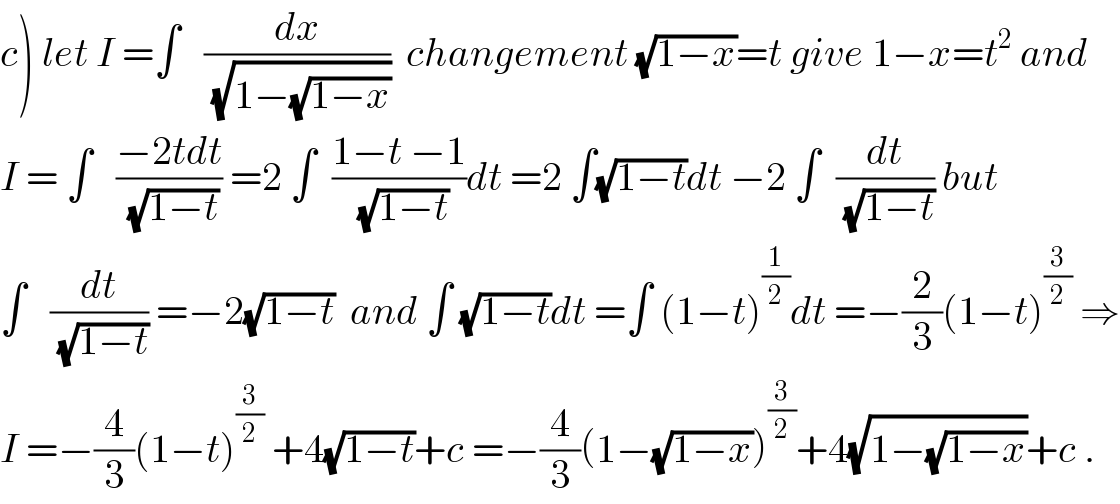
Commented by behi83417@gmail.com last updated on 06/Dec/18
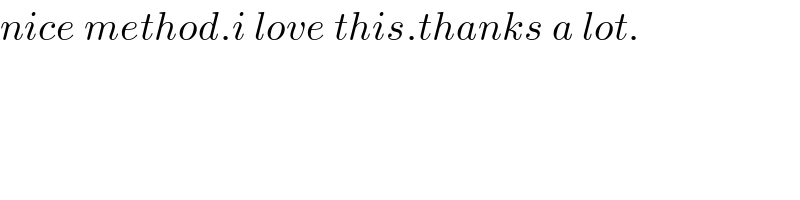
Answered by MJS last updated on 06/Dec/18
![(a) ∫(dx/(√(1−tan x)))= [t=(√(1−tan x)) → dx=−((2tdt)/(t^4 −2t^2 +2))] =−2∫(dt/(t^4 −2t^2 +2))=−2∫(dt/((t^2 −(√(2+2(√2)))t+(√2))(t^2 +(√(2+2(√2)))t+(√2))))= =−2∫(dt/((t^2 −pt+q)(t^2 +pt+q)))= =(1/(pq))∫((t−p)/(t^2 −pt+q))dt−(1/(pq))∫((t+p)/(t^2 +pt+q))dt and these can be solved](Q49377.png)
Answered by tanmay.chaudhury50@gmail.com last updated on 06/Dec/18
![a)∫(dx/(√(1−tanx))) t^2 =1−tanx 2tdt=−sec^2 xdx dx=((−2tdt)/(1+(1−t^2 )^2 )) ∫((−2tdt)/(t×{1+(1−t^2 )^2 })) =−2∫(dt/(t^4 −2t^2 +2)) =−2∫((1/t^2 )/(t^2 −2+(2/t^2 )))dt now t^2 +(2/t^2 )=(t+((√2)/t))^2 −2(√2) =(t−((√2)/t))^2 +2(√2) d(t+((√2)/t))=(1−((√2)/t^2 ))dt d(t−((√2)/t))=(1+((√2)/t^2 ))dt =((−1)/(√2))∫(((2(√2))/t^2 )/(t^2 −2+(2/t^2 )))dt =((−1)/(√2))∫(((1+((√2)/t^2 ))−(1−((√2)/t^2 )))/(t^2 −2+(2/t^2 )))dt =((−1)/(√2))[∫((1+((√2)/t^2 ))/((t−((√2)/t))^2 +2(√2)))dt−∫((1−((√2)/t^2 ))/((t+((√2)/t))^2 −2(√2)))dt] =((−1)/(√2))[(1/(√(2(√2) )))tan^(−1) (((t−((√2)/t))/(√(2(√2)))))−(1/(2(√(2(√2)))))ln{(((t+((√2)/t))−(√(2(√2))))/((t+((√2)/t))+(√(2(√2)))))}+c now pls put t=(√(1−tanx))](Q49378.png)
Commented by behi83417@gmail.com last updated on 06/Dec/18
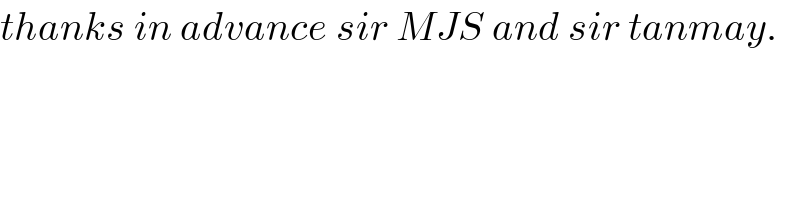
Answered by tanmay.chaudhury50@gmail.com last updated on 06/Dec/18
Commented by tanmay.chaudhury50@gmail.com last updated on 06/Dec/18
Commented by behi83417@gmail.com last updated on 06/Dec/18

Commented by behi83417@gmail.com last updated on 06/Dec/18

Answered by MJS last updated on 06/Dec/18
![(b) ∫(dx/((1−tan x)^(1/3) ))= [t=(1−tan x)^(1/3) → dx=−((3t^2 )/(t^6 −2t^3 +2))] =−3∫(t/(t^6 −2t^3 +2))dt t^6 −2t^3 +2=(t^2 +(4)^(1/3) t+(2)^(1/3) )(t^2 −((1−(√3))/(2)^(1/3) )t+(2)^(1/3) )(t^2 −((1+(√3))/(2)^(1/3) )t+(2)^(1/3) )= =(t^2 +pt+q)(t^2 +rt+q)(t^2 +st+q) ⇒ ⇒ (3/(q(p−r)(p−s)))∫((t+p)/(t^2 +pt+q))dt+(3/(q(r−p)(r−s)))∫((t+r)/(t^2 +rt+q))dt+(3/(q(s−p)(s−r)))∫((t+s)/(t^2 +st+q))dt ...and again we can solve these](Q49411.png)
Commented by behi83417@gmail.com last updated on 06/Dec/18
Answered by tanmay.chaudhury50@gmail.com last updated on 07/Dec/18
![b)t^3 =1−tanx 3t^2 dt=−sec^2 xdx dx=((−3t^2 )/(1+(1−t^3 )^2 ))dt=((−3t^2 )/(t^6 −2t^3 +2))dt ∫((−3t^2 )/(t^6 −2t^3 +2))×(1/t)dt =−3∫((tdt)/(t^6 −2t^3 +2)) t^6 −2t^3 +2 =(t^3 )^2 −2×t^3 ×1+1+1 =−3∫((tdt)/((t^3 −1)^2 −i^2 )) =−3∫((tdt)/((t^3 −1−i)(t^3 −1+i))) =−3∫((tdt)/((t^3 +a)(t^3 +b))) [a=−1−i b=−1+i] =((−3)/(a−b))∫[((t(t^3 +a)−t(t^3 +b))/((t^3 +a)(t^3 +b)))]dt =(3/(b−a))[∫((tdt)/(t^3 +b))−∫((tdt)/(t^3 +a))] now solving ∫((tdt)/(t^3 +a)) ∫((tdt)/((t+^3 (√a) )(t^2 −t×^3 (√a) +^(2/3) (√a) ))) ∫((tdt)/((t+p)(t^2 −tp+p^2 ))) (t/((t+p)(t^2 −tp+p^2 )))=(A/(t+p))+((Bt+C)/(t^2 −tp+p^2 )) t=A(t^2 −tp+p^2 )+(Bt+C)(t+p) t=t^2 (A+B)+t(−Ap+Bp+C)+Ap^2 +Cp A+B=0 Ap^2 +Cp=0 −Ap+Bp+C=1 C=−Ap −Ap−Ap−Ap=1 A=((−1)/(3p)) B=(1/(3p)) C=((−1)/3) ∫(A/(t+p))dt+∫((Bt+C)/(t^2 −tp+p^2 ))dt ((−1)/(3p))∫(dt/(t+p))+∫(((1/(3p))t+((−1)/3))/(t^2 −tp+p^2 ))dt ((−1)/(3p))∫(dt/(t+p))+(1/3)∫((t−1)/(t^2 −tp+p^2 ))dt ((−1)/(3p))∫(dt/(t+p))+(1/6)∫((2t−p+p−2)/(t^2 −tp+p^2 )) ((−1)/(3p))∫(dt/(t+p))+(1/6)∫((d(t^2 −tp+p^2 ))/(t^2 −tp+p^2 ))+((p−2)/6)∫(dt/(t^2 −2.t.(p/2)+(p^2 /4)+((3p^2 )/4))) ((−1)/(3p))ln(t+p)+(1/6)ln(t^2 −tp+p^2 )+((p−2)/6)×(1/(((((√3) p)/2))^ ))×tan^(−1) (((t−(p/2))/(((√3) p)/2)))+c_1 now put p=(a)^(1/3) next put a=(−1−i) similarly we can find ∫((tdt)/(t^3 +b)) in place of p put (b)^(1/3) next put b=(−1+i) finaly algebric addition...](Q49458.png)
Commented by behi83417@gmail.com last updated on 07/Dec/18
Commented by tanmay.chaudhury50@gmail.com last updated on 07/Dec/18

