
Question and Answers Forum
Question Number 49460 by Pk1167156@gmail.com last updated on 07/Dec/18
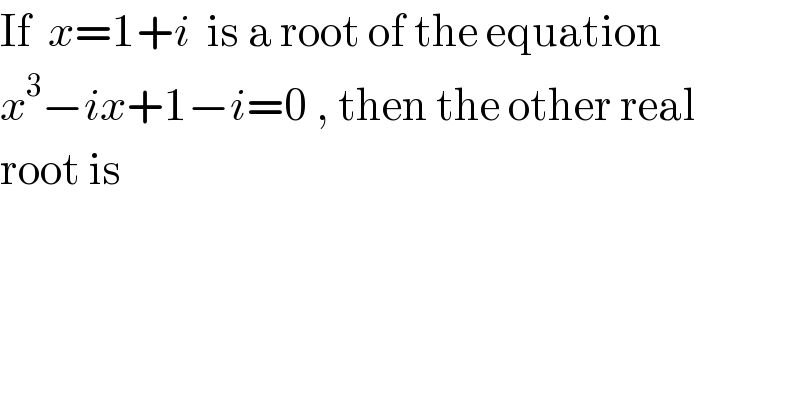
Commented by Kunal12588 last updated on 07/Dec/18
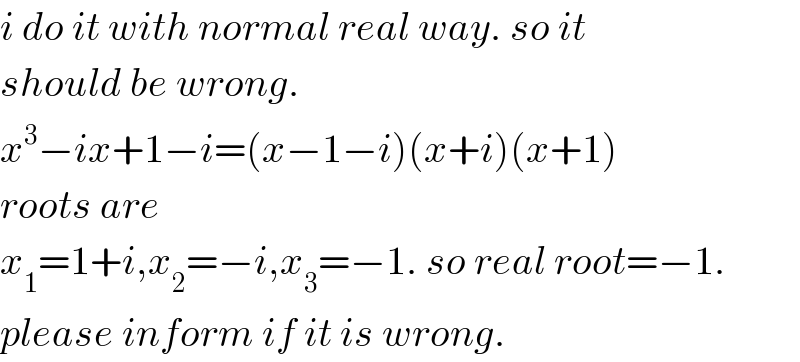
Commented by Abdo msup. last updated on 08/Dec/18
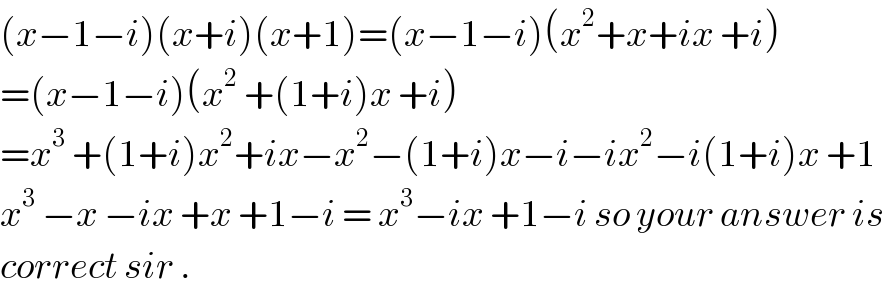
| ||
Question and Answers Forum | ||
Question Number 49460 by Pk1167156@gmail.com last updated on 07/Dec/18 | ||
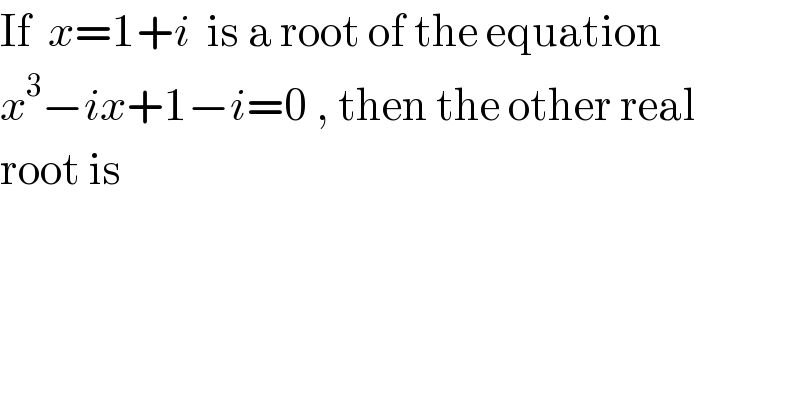 | ||
Commented by Kunal12588 last updated on 07/Dec/18 | ||
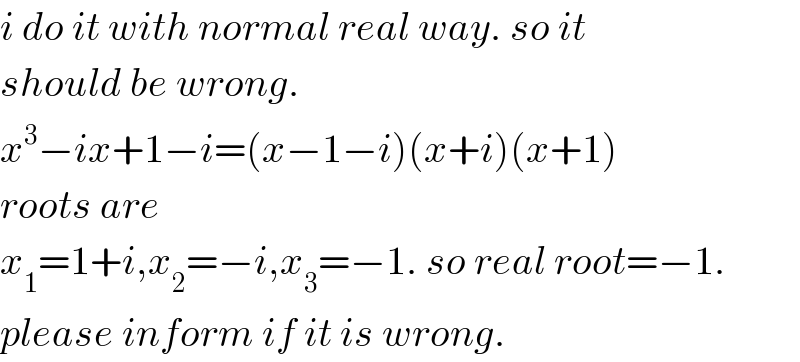 | ||
Commented by Abdo msup. last updated on 08/Dec/18 | ||
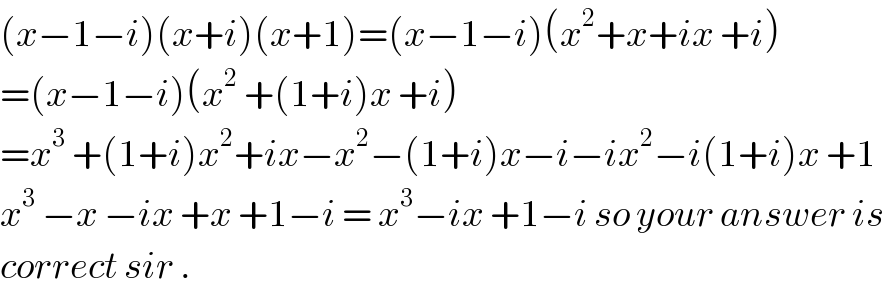 | ||