Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 50415 by Abdo msup. last updated on 16/Dec/18

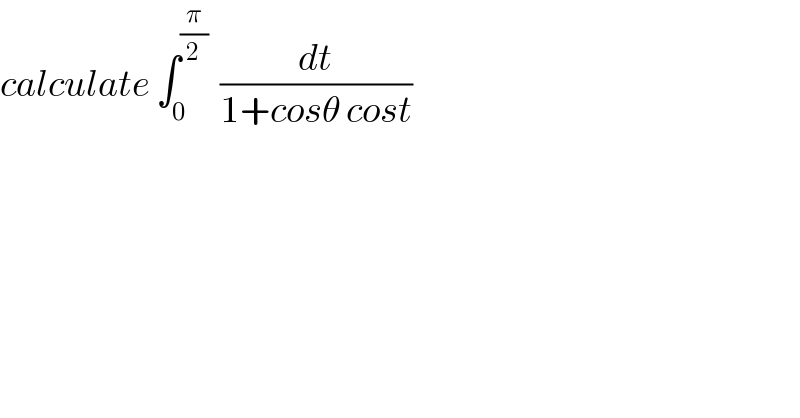
calculate ∫_0 ^(π/2) (dt/(1+cosθ cost))

$${calculate}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:\frac{{dt}}{\mathrm{1}+{cos}\theta\:{cost}} \\ $$

Commented by Abdo msup. last updated on 18/Dec/18

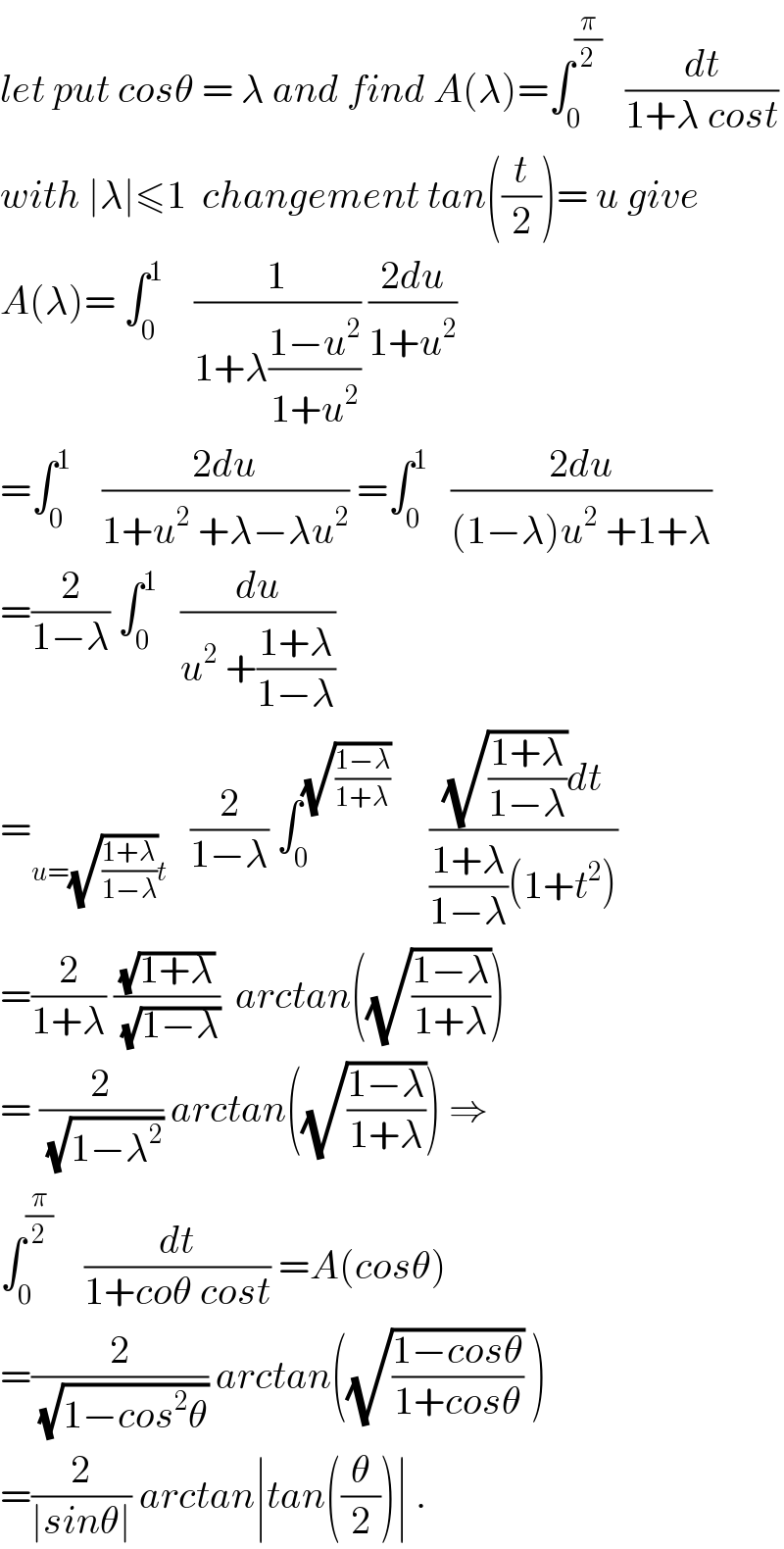
let put cosθ = λ and find A(λ)=∫_0 ^(π/2) (dt/(1+λ cost)) with ∣λ∣≤1 changement tan((t/2))= u give A(λ)= ∫_0 ^1 (1/(1+λ((1−u^2 )/(1+u^2 )))) ((2du)/(1+u^2 )) =∫_0 ^1 ((2du)/(1+u^2 +λ−λu^2 )) =∫_0 ^1 ((2du)/((1−λ)u^2 +1+λ)) =(2/(1−λ)) ∫_0 ^1 (du/(u^2 +((1+λ)/(1−λ)))) =_(u=(√((1+λ)/(1−λ)))t) (2/(1−λ)) ∫_0 ^(√((1−λ)/(1+λ))) (((√((1+λ)/(1−λ)))dt)/(((1+λ)/(1−λ))(1+t^2 ))) =(2/(1+λ)) ((√(1+λ))/(√(1−λ))) arctan((√((1−λ)/(1+λ)))) = (2/(√(1−λ^2 ))) arctan((√((1−λ)/(1+λ)))) ⇒ ∫_0 ^(π/2) (dt/(1+coθ cost)) =A(cosθ) =(2/(√(1−cos^2 θ))) arctan((√((1−cosθ)/(1+cosθ))) ) =(2/(∣sinθ∣)) arctan∣tan((θ/2))∣ .

$${let}\:{put}\:{cos}\theta\:=\:\lambda\:{and}\:{find}\:{A}\left(\lambda\right)=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\frac{{dt}}{\mathrm{1}+\lambda\:{cost}} \\ $$$${with}\:\mid\lambda\mid\leqslant\mathrm{1}\:\:{changement}\:{tan}\left(\frac{{t}}{\mathrm{2}}\right)=\:{u}\:{give} \\ $$$${A}\left(\lambda\right)=\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\:\frac{\mathrm{1}}{\mathrm{1}+\lambda\frac{\mathrm{1}−{u}^{\mathrm{2}} }{\mathrm{1}+{u}^{\mathrm{2}} }}\:\frac{\mathrm{2}{du}}{\mathrm{1}+{u}^{\mathrm{2}} } \\ $$$$=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\:\frac{\mathrm{2}{du}}{\mathrm{1}+{u}^{\mathrm{2}} \:+\lambda−\lambda{u}^{\mathrm{2}} }\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\frac{\mathrm{2}{du}}{\left(\mathrm{1}−\lambda\right){u}^{\mathrm{2}} \:+\mathrm{1}+\lambda} \\ $$$$=\frac{\mathrm{2}}{\mathrm{1}−\lambda}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\frac{{du}}{{u}^{\mathrm{2}} \:+\frac{\mathrm{1}+\lambda}{\mathrm{1}−\lambda}}\: \\ $$$$=_{{u}=\sqrt{\frac{\mathrm{1}+\lambda}{\mathrm{1}−\lambda}}{t}} \:\:\:\frac{\mathrm{2}}{\mathrm{1}−\lambda}\:\int_{\mathrm{0}} ^{\sqrt{\frac{\mathrm{1}−\lambda}{\mathrm{1}+\lambda}}} \:\:\:\:\:\frac{\sqrt{\frac{\mathrm{1}+\lambda}{\mathrm{1}−\lambda}}{dt}}{\frac{\mathrm{1}+\lambda}{\mathrm{1}−\lambda}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)} \\ $$$$=\frac{\mathrm{2}}{\mathrm{1}+\lambda}\:\frac{\sqrt{\mathrm{1}+\lambda}}{\sqrt{\mathrm{1}−\lambda}}\:\:{arctan}\left(\sqrt{\frac{\mathrm{1}−\lambda}{\mathrm{1}+\lambda}}\right) \\ $$$$=\:\frac{\mathrm{2}}{\sqrt{\mathrm{1}−\lambda^{\mathrm{2}} }}\:{arctan}\left(\sqrt{\frac{\mathrm{1}−\lambda}{\mathrm{1}+\lambda}}\right)\:\Rightarrow \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\:\frac{{dt}}{\mathrm{1}+{co}\theta\:{cost}}\:={A}\left({cos}\theta\right) \\ $$$$=\frac{\mathrm{2}}{\sqrt{\mathrm{1}−{cos}^{\mathrm{2}} \theta}}\:{arctan}\left(\sqrt{\frac{\mathrm{1}−{cos}\theta}{\mathrm{1}+{cos}\theta}}\:\right) \\ $$$$=\frac{\mathrm{2}}{\mid{sin}\theta\mid}\:{arctan}\mid{tan}\left(\frac{\theta}{\mathrm{2}}\right)\mid\:. \\ $$

Answered by tanmay.chaudhury50@gmail.com last updated on 17/Dec/18

∫(dt/(1+cosθcost)) (1/(cosθ))∫(dt/(secθ+cost)) (1/(cosθ))∫(dt/(secθ+((1−tan^2 (t/2))/(1+tan^2 (t/2))))) (1/(cosθ))∫((sec^2 (t/2)dt)/(secθ+secθtan^2 (t/2)+1−tan^2 (t/2))) =secθ∫((sec^2 (t/2)×dt)/((secθ+1)+tan^2 (t/2)(secθ−1))) =secθ∫((sec^2 (t/2))/((secθ−1){((secθ+1)/(secθ−1))+tan^2 (t/2)}))dt =((secθ)/(secθ−1))∫((sec^2 (t/2))/(((1+cosθ)/(1−cosθ))+tan^2 (t/2)))dt =(1/(1−cosθ))∫((sec^2 (t/2)dt)/(cot^2 (θ/2)+tan^2 (t/2))) k=tan(t/2) dk=(1/2)sec^2 (t/2)dt =(1/(2sin^2 (θ/2)))∫((2dk)/(cot^2 (θ/2)+k^2 )) =(1/(sin^2 (θ/2)))∫(dk/(cot^2 (θ/2)+k^2 )) =(1/(sin^2 (θ/2)))×(1/(cot(θ/2)))tan^(−1) ((k/(cot(θ/2)))) =(2/(sinθ))tan^(−1) (((tan(t/2))/(cot(θ/2))))+c required answer iz (2/(sinθ))∣tan^(−1) (((tan(t/2))/(cot(θ/2))))∣_0 ^(π/2) =(2/(sinθ))[tan^(−1) (((tan(π/4))/(cot(θ/2))))−tan^(−1) (((tan0)/(cot(θ/2))))] =(2/(sinθ))[tan^(−1) (tan(θ/2))] =(2/(sinθ))×(θ/2)=(θ/(sinθ))

$$\int\frac{{dt}}{\mathrm{1}+{cos}\theta{cost}} \\ $$$$\frac{\mathrm{1}}{{cos}\theta}\int\frac{{dt}}{{sec}\theta+{cost}} \\ $$$$\frac{\mathrm{1}}{{cos}\theta}\int\frac{{dt}}{{sec}\theta+\frac{\mathrm{1}−{tan}^{\mathrm{2}} \frac{{t}}{\mathrm{2}}}{\mathrm{1}+{tan}^{\mathrm{2}} \frac{{t}}{\mathrm{2}}}} \\ $$$$\frac{\mathrm{1}}{{cos}\theta}\int\frac{{sec}^{\mathrm{2}} \frac{{t}}{\mathrm{2}}{dt}}{{sec}\theta+{sec}\theta{tan}^{\mathrm{2}} \frac{{t}}{\mathrm{2}}+\mathrm{1}−{tan}^{\mathrm{2}} \frac{{t}}{\mathrm{2}}} \\ $$$$={sec}\theta\int\frac{{sec}^{\mathrm{2}} \frac{{t}}{\mathrm{2}}×{dt}}{\left({sec}\theta+\mathrm{1}\right)+{tan}^{\mathrm{2}} \frac{{t}}{\mathrm{2}}\left({sec}\theta−\mathrm{1}\right)} \\ $$$$={sec}\theta\int\frac{{sec}^{\mathrm{2}} \frac{{t}}{\mathrm{2}}}{\left({sec}\theta−\mathrm{1}\right)\left\{\frac{{sec}\theta+\mathrm{1}}{{sec}\theta−\mathrm{1}}+{tan}^{\mathrm{2}} \frac{{t}}{\mathrm{2}}\right\}}{dt} \\ $$$$=\frac{{sec}\theta}{{sec}\theta−\mathrm{1}}\int\frac{{sec}^{\mathrm{2}} \frac{{t}}{\mathrm{2}}}{\frac{\mathrm{1}+{cos}\theta}{\mathrm{1}−{cos}\theta}+{tan}^{\mathrm{2}} \frac{{t}}{\mathrm{2}}}{dt} \\ $$$$=\frac{\mathrm{1}}{\mathrm{1}−{cos}\theta}\int\frac{{sec}^{\mathrm{2}} \frac{{t}}{\mathrm{2}}{dt}}{{cot}^{\mathrm{2}} \frac{\theta}{\mathrm{2}}+{tan}^{\mathrm{2}} \frac{{t}}{\mathrm{2}}} \\ $$$${k}={tan}\frac{{t}}{\mathrm{2}}\:\:{dk}=\frac{\mathrm{1}}{\mathrm{2}}{sec}^{\mathrm{2}} \frac{{t}}{\mathrm{2}}{dt} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}{sin}^{\mathrm{2}} \frac{\theta}{\mathrm{2}}}\int\frac{\mathrm{2}{dk}}{{cot}^{\mathrm{2}} \frac{\theta}{\mathrm{2}}+{k}^{\mathrm{2}} } \\ $$$$=\frac{\mathrm{1}}{{sin}^{\mathrm{2}} \frac{\theta}{\mathrm{2}}}\int\frac{{dk}}{{cot}^{\mathrm{2}} \frac{\theta}{\mathrm{2}}+{k}^{\mathrm{2}} } \\ $$$$=\frac{\mathrm{1}}{{sin}^{\mathrm{2}} \frac{\theta}{\mathrm{2}}}×\frac{\mathrm{1}}{{cot}\frac{\theta}{\mathrm{2}}}{tan}^{−\mathrm{1}} \left(\frac{{k}}{{cot}\frac{\theta}{\mathrm{2}}}\right) \\ $$$$=\frac{\mathrm{2}}{{sin}\theta}{tan}^{−\mathrm{1}} \left(\frac{{tan}\frac{{t}}{\mathrm{2}}}{{cot}\frac{\theta}{\mathrm{2}}}\right)+{c} \\ $$$${required}\:{answer}\:{iz} \\ $$$$\frac{\mathrm{2}}{{sin}\theta}\mid{tan}^{−\mathrm{1}} \left(\frac{{tan}\frac{{t}}{\mathrm{2}}}{{cot}\frac{\theta}{\mathrm{2}}}\right)\mid_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \\ $$$$=\frac{\mathrm{2}}{\boldsymbol{{sin}}\theta}\left[{tan}^{−\mathrm{1}} \left(\frac{{tan}\frac{\pi}{\mathrm{4}}}{{cot}\frac{\theta}{\mathrm{2}}}\right)−{tan}^{−\mathrm{1}} \left(\frac{{tan}\mathrm{0}}{{cot}\frac{\theta}{\mathrm{2}}}\right)\right] \\ $$$$=\frac{\mathrm{2}}{{sin}\theta}\left[{tan}^{−\mathrm{1}} \left({tan}\frac{\theta}{\mathrm{2}}\right)\right] \\ $$$$=\frac{\mathrm{2}}{{sin}\theta}×\frac{\theta}{\mathrm{2}}=\frac{\theta}{{sin}\theta} \\ $$$$ \\ $$$$ \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com