
Question Number 50417 by Abdo msup. last updated on 16/Dec/18
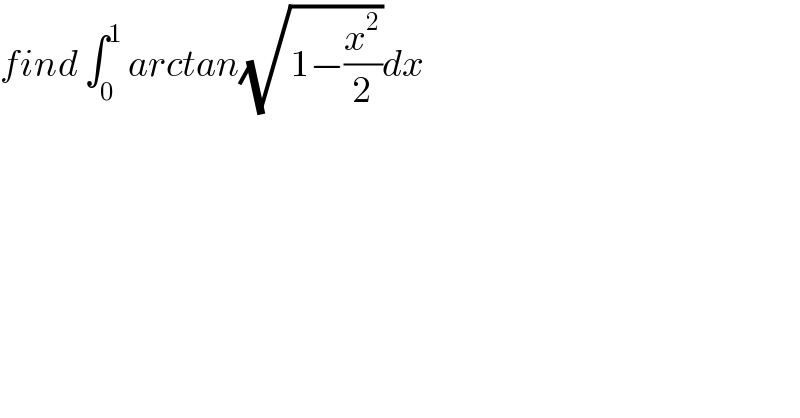
$${find}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:{arctan}\sqrt{\mathrm{1}−\frac{{x}^{\mathrm{2}} }{\mathrm{2}}}{dx} \\ $$
Commented by Abdo msup. last updated on 19/Dec/18
![changement x=(√2)sinθ give I = ∫_0 ^(π/4) arctan(cosθ)(√2)cosθ dθ =(√2)∫_0 ^(π/4) cosθ arctan(cosθ)dθ by psrts u^′ =cosθ and v=arctan(cosθ) ⇒ I = (√2){ [sinθ arctan(cosθ)]_0 ^(π/4) −∫_0 ^(π/4) sinθ((−sinθ)/(1+cos^2 θ))dθ =(√2){(1/(√2)) arctan((1/(√2))) +∫_0 ^(π/4) ((1−cos^2 θ)/(1+cos^2 θ))dθ} but ∫_0 ^(π/4) ((1−cos^2 θ)/(1+cos^2 θ)) dθ =∫_0 ^(π/4) ((1−(1/(1+tan^2 θ)))/(1+(1/(1+tan^2 θ))))dθ =∫_0 ^(π/4) ((tan^2 θ)/(2+tan^2 θ))dθ =_(tanθ =x) ∫_0 ^1 (x^2 /(2+x^2 )) (dx/(1+x^2 )) =∫_0 ^1 (x^2 /((x^2 +2)(x^2 +1)))dx = ∫_0 ^1 ((x^2 +1−1)/((x^2 +1)(x^2 +2)))dx =∫_0 ^1 (dx/(x^2 +2)) −∫_0 ^1 (dx/((x^2 +1)(x^2 +2))) =∫_0 ^1 (dx/(x^2 +2)) −∫_0 ^1 ((1/(x^2 +1)) −(1/(x^2 +2)))dx =2 ∫_0 ^1 (dx/(x^(2 ) +2)) −(π/4) ∫_0 ^1 (dx/(x^2 +2)) =_(x=(√2)u) ∫_0 ^(1/(√2)) (((√2)du)/(2(1+u^2 ))) =((√2)/2) arctan((1/(√2))) ⇒ ∫_0 ^(π/4) ((1−cos^2 θ)/(1+cos^2 θ))dθ =(√2)arctan((1/(√2)))−(π/4) ⇒ I = arctan((1/(√2))) +2 arctan((1/(√2)))−((π(√2))/4) =3arctan((1/(√2)))−((π(√2))/4)](Q50693.png)
$${changement}\:{x}=\sqrt{\mathrm{2}}{sin}\theta\:{give} \\ $$$${I}\:=\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:{arctan}\left({cos}\theta\right)\sqrt{\mathrm{2}}{cos}\theta\:{d}\theta \\ $$$$=\sqrt{\mathrm{2}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:{cos}\theta\:{arctan}\left({cos}\theta\right){d}\theta\:\:{by}\:{psrts}\:{u}^{'} ={cos}\theta\:{and} \\ $$$${v}={arctan}\left({cos}\theta\right)\:\Rightarrow \\ $$$${I}\:=\:\sqrt{\mathrm{2}}\left\{\:\left[{sin}\theta\:{arctan}\left({cos}\theta\right)\right]_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:−\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:{sin}\theta\frac{−{sin}\theta}{\mathrm{1}+{cos}^{\mathrm{2}} \theta}{d}\theta\right. \\ $$$$=\sqrt{\mathrm{2}}\left\{\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\:{arctan}\left(\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\right)\:+\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\frac{\mathrm{1}−{cos}^{\mathrm{2}} \theta}{\mathrm{1}+{cos}^{\mathrm{2}} \theta}{d}\theta\right\}\:{but} \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\:\frac{\mathrm{1}−{cos}^{\mathrm{2}} \theta}{\mathrm{1}+{cos}^{\mathrm{2}} \theta}\:{d}\theta\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\frac{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{1}+{tan}^{\mathrm{2}} \theta}}{\mathrm{1}+\frac{\mathrm{1}}{\mathrm{1}+{tan}^{\mathrm{2}} \theta}}{d}\theta \\ $$$$=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\frac{{tan}^{\mathrm{2}} \theta}{\mathrm{2}+{tan}^{\mathrm{2}} \theta}{d}\theta\:=_{{tan}\theta\:={x}} \:\:\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{x}^{\mathrm{2}} }{\mathrm{2}+{x}^{\mathrm{2}} }\:\frac{{dx}}{\mathrm{1}+{x}^{\mathrm{2}} } \\ $$$$=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\frac{{x}^{\mathrm{2}} }{\left({x}^{\mathrm{2}} +\mathrm{2}\right)\left({x}^{\mathrm{2}} +\mathrm{1}\right)}{dx}\: \\ $$$$=\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{x}^{\mathrm{2}} +\mathrm{1}−\mathrm{1}}{\left({x}^{\mathrm{2}} +\mathrm{1}\right)\left({x}^{\mathrm{2}} +\mathrm{2}\right)}{dx} \\ $$$$=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\frac{{dx}}{{x}^{\mathrm{2}} \:+\mathrm{2}}\:−\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\frac{{dx}}{\left({x}^{\mathrm{2}} +\mathrm{1}\right)\left({x}^{\mathrm{2}} +\mathrm{2}\right)} \\ $$$$=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{dx}}{{x}^{\mathrm{2}} \:+\mathrm{2}}\:−\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\left(\frac{\mathrm{1}}{{x}^{\mathrm{2}} +\mathrm{1}}\:−\frac{\mathrm{1}}{{x}^{\mathrm{2}} \:+\mathrm{2}}\right){dx} \\ $$$$=\mathrm{2}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{dx}}{{x}^{\mathrm{2}\:} +\mathrm{2}}\:−\frac{\pi}{\mathrm{4}} \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\frac{{dx}}{{x}^{\mathrm{2}} \:+\mathrm{2}}\:=_{{x}=\sqrt{\mathrm{2}}{u}} \int_{\mathrm{0}} ^{\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}} \:\:\:\:\frac{\sqrt{\mathrm{2}}{du}}{\mathrm{2}\left(\mathrm{1}+{u}^{\mathrm{2}} \right)}\:=\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\:{arctan}\left(\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\right)\:\Rightarrow \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\frac{\mathrm{1}−{cos}^{\mathrm{2}} \theta}{\mathrm{1}+{cos}^{\mathrm{2}} \theta}{d}\theta\:=\sqrt{\mathrm{2}}{arctan}\left(\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\right)−\frac{\pi}{\mathrm{4}}\:\Rightarrow \\ $$$${I}\:=\:{arctan}\left(\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\right)\:+\mathrm{2}\:{arctan}\left(\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\right)−\frac{\pi\sqrt{\mathrm{2}}}{\mathrm{4}} \\ $$$$=\mathrm{3}{arctan}\left(\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\right)−\frac{\pi\sqrt{\mathrm{2}}}{\mathrm{4}} \\ $$$$ \\ $$
