
Question Number 50421 by Abdo msup. last updated on 16/Dec/18
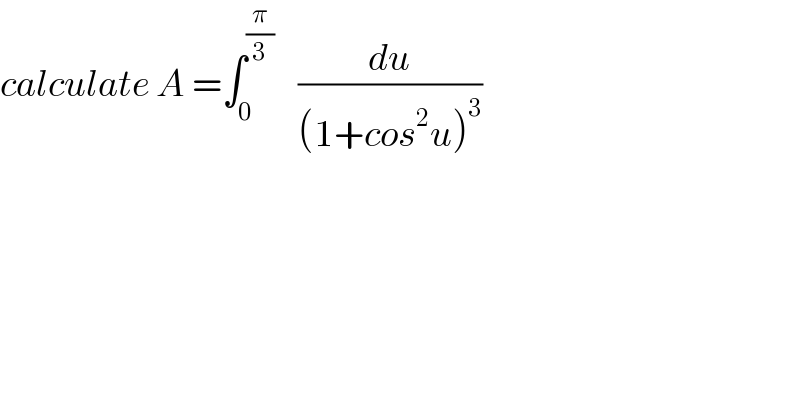
$${calculate}\:{A}\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{3}}} \:\:\:\:\frac{{du}}{\left(\mathrm{1}+{cos}^{\mathrm{2}} {u}\right)^{\mathrm{3}} } \\ $$
Commented by maxmathsup by imad last updated on 18/Dec/18
![we have 1+cos^2 u =1+(1/(1+tan^2 u)) =((2+tan^2 u)/(1+tan^2 u)) ⇒ A =∫_0 ^(π/3) (du/((((2+tan^2 u)/(1+tan^2 u)))^3 )) =∫_0 ^(π/3) (((1+tan^2 u)^3 )/((2+tan^2 u)^3 )) changement tan(u)=x give A =∫_0 ^(√3) (((1+x^2 )^3 )/((2+x^2 )^3 )) (dx/((1+x^2 ))) =∫_0 ^(√3) ((x^4 +2x^2 +1)/((x^2 +2)^3 )) dx let decompose F(x) =((x^4 +2x^2 +1)/((x^2 +2)^3 )) F(x)=G(u) =((u^2 +2u +1)/((u+2)^3 )) =(a/(u+2)) +(b/((u+2)^2 )) +(c/((u+2)^3 )) c =lim_(u→−2) (u+2)^2 G(u) =1 lim_(u→+∞) uG(u) =1 =a ⇒G(u) =(1/(u+2)) +(b/((u+2)^2 )) +(1/((u+2)^3 )) G(−1) =0 =1 +b +1 =b+2 ⇒b =−2 ⇒G(u)=(1/(u+2)) −(2/((u+2)^2 )) +(1/((u+2)^3 )) ⇒ F(x) = (1/(x^2 +2)) −(2/((x^2 +2)^2 )) +(1/((x^2 +2)^3 )) ⇒ ∫_0 ^(√3) F(x)dx =∫_0 ^(√3) (dx/(x^2 +2)) −2 ∫_0 ^(√3) (dx/((x^2 +2)^2 )) +∫_0 ^(√3) (dx/((x^2 +2)^3 )) ∫_0 ^(√3) (dx/(x^2 +2)) =_(x=(√2)u) ∫_0 ^((√3)/(√2)) (((√2)du)/(2(1+u^2 ))) =(1/(√2)) arctan(((√3)/(√2))). ∫_0 ^(√3) (dx/((x^2 +2)^2 )) =_(x=(√2)tanθ) ∫_0 ^(arctan(((√3)/(√2)))) (((√2)(1+tan^2 θ)dθ)/(4(1+tan^2 θ)^2 )) =((√2)/4)∫_0 ^(arctan(((√3)/(√2)))) cos^2 θ dθ =((√2)/4) ∫_0 ^(arctan(((√3)/(√2)))) ((1+cos(2θ))/2) dθ =((√2)/8) arctan(((√3)/(√2))) +((√2)/(16)) [sin(2θ)]_0 ^(arctan(((√3)/(√2)))) =((√2)/8) arctan(((√3)/(√2))) +((√2)/(16)) sin(2arctan(((√3)/(√2)))). ...be continued...](Q50686.png)
$$\:{we}\:{have}\:\mathrm{1}+{cos}^{\mathrm{2}} {u}\:=\mathrm{1}+\frac{\mathrm{1}}{\mathrm{1}+{tan}^{\mathrm{2}} {u}}\:=\frac{\mathrm{2}+{tan}^{\mathrm{2}} {u}}{\mathrm{1}+{tan}^{\mathrm{2}} {u}}\:\Rightarrow \\ $$$${A}\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{3}}} \:\:\:\frac{{du}}{\left(\frac{\mathrm{2}+{tan}^{\mathrm{2}} {u}}{\mathrm{1}+{tan}^{\mathrm{2}} {u}}\right)^{\mathrm{3}} }\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{3}}} \:\:\frac{\left(\mathrm{1}+{tan}^{\mathrm{2}} {u}\right)^{\mathrm{3}} }{\left(\mathrm{2}+{tan}^{\mathrm{2}} {u}\right)^{\mathrm{3}} }\:\:{changement}\:{tan}\left({u}\right)={x}\:{give} \\ $$$${A}\:=\int_{\mathrm{0}} ^{\sqrt{\mathrm{3}}} \:\:\:\frac{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{\mathrm{3}} }{\left(\mathrm{2}+{x}^{\mathrm{2}} \right)^{\mathrm{3}} }\:\frac{{dx}}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)}\:=\int_{\mathrm{0}} ^{\sqrt{\mathrm{3}}} \:\:\:\frac{{x}^{\mathrm{4}} \:+\mathrm{2}{x}^{\mathrm{2}} \:+\mathrm{1}}{\left({x}^{\mathrm{2}} \:+\mathrm{2}\right)^{\mathrm{3}} }\:{dx}\:{let}\:{decompose} \\ $$$${F}\left({x}\right)\:=\frac{{x}^{\mathrm{4}} \:+\mathrm{2}{x}^{\mathrm{2}} \:+\mathrm{1}}{\left({x}^{\mathrm{2}} \:+\mathrm{2}\right)^{\mathrm{3}} } \\ $$$${F}\left({x}\right)={G}\left({u}\right)\:=\frac{{u}^{\mathrm{2}} \:+\mathrm{2}{u}\:+\mathrm{1}}{\left({u}+\mathrm{2}\right)^{\mathrm{3}} }\:=\frac{{a}}{{u}+\mathrm{2}}\:+\frac{{b}}{\left({u}+\mathrm{2}\right)^{\mathrm{2}} }\:+\frac{{c}}{\left({u}+\mathrm{2}\right)^{\mathrm{3}} } \\ $$$${c}\:={lim}_{{u}\rightarrow−\mathrm{2}} \left({u}+\mathrm{2}\right)^{\mathrm{2}} {G}\left({u}\right)\:=\mathrm{1} \\ $$$${lim}_{{u}\rightarrow+\infty} {uG}\left({u}\right)\:=\mathrm{1}\:={a}\:\Rightarrow{G}\left({u}\right)\:=\frac{\mathrm{1}}{{u}+\mathrm{2}}\:+\frac{{b}}{\left({u}+\mathrm{2}\right)^{\mathrm{2}} }\:+\frac{\mathrm{1}}{\left({u}+\mathrm{2}\right)^{\mathrm{3}} } \\ $$$${G}\left(−\mathrm{1}\right)\:=\mathrm{0}\:=\mathrm{1}\:+{b}\:+\mathrm{1}\:={b}+\mathrm{2}\:\Rightarrow{b}\:=−\mathrm{2}\:\Rightarrow{G}\left({u}\right)=\frac{\mathrm{1}}{{u}+\mathrm{2}}\:−\frac{\mathrm{2}}{\left({u}+\mathrm{2}\right)^{\mathrm{2}} }\:+\frac{\mathrm{1}}{\left({u}+\mathrm{2}\right)^{\mathrm{3}} }\:\Rightarrow \\ $$$${F}\left({x}\right)\:=\:\frac{\mathrm{1}}{{x}^{\mathrm{2}} \:+\mathrm{2}}\:−\frac{\mathrm{2}}{\left({x}^{\mathrm{2}} \:+\mathrm{2}\right)^{\mathrm{2}} }\:+\frac{\mathrm{1}}{\left({x}^{\mathrm{2}} \:+\mathrm{2}\right)^{\mathrm{3}} }\:\Rightarrow \\ $$$$\int_{\mathrm{0}} ^{\sqrt{\mathrm{3}}} \:{F}\left({x}\right){dx}\:=\int_{\mathrm{0}} ^{\sqrt{\mathrm{3}}} \:\:\frac{{dx}}{{x}^{\mathrm{2}} \:+\mathrm{2}}\:−\mathrm{2}\:\int_{\mathrm{0}} ^{\sqrt{\mathrm{3}}} \:\:\frac{{dx}}{\left({x}^{\mathrm{2}} \:+\mathrm{2}\right)^{\mathrm{2}} }\:+\int_{\mathrm{0}} ^{\sqrt{\mathrm{3}}} \:\:\frac{{dx}}{\left({x}^{\mathrm{2}} \:+\mathrm{2}\right)^{\mathrm{3}} } \\ $$$$\int_{\mathrm{0}} ^{\sqrt{\mathrm{3}}} \:\:\:\frac{{dx}}{{x}^{\mathrm{2}} \:+\mathrm{2}}\:=_{{x}=\sqrt{\mathrm{2}}{u}} \:\:\:\:\int_{\mathrm{0}} ^{\frac{\sqrt{\mathrm{3}}}{\sqrt{\mathrm{2}}}} \:\:\:\frac{\sqrt{\mathrm{2}}{du}}{\mathrm{2}\left(\mathrm{1}+{u}^{\mathrm{2}} \right)}\:=\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\:{arctan}\left(\frac{\sqrt{\mathrm{3}}}{\sqrt{\mathrm{2}}}\right). \\ $$$$\int_{\mathrm{0}} ^{\sqrt{\mathrm{3}}} \:\:\frac{{dx}}{\left({x}^{\mathrm{2}} \:+\mathrm{2}\right)^{\mathrm{2}} }\:=_{{x}=\sqrt{\mathrm{2}}{tan}\theta} \:\:\:\:\int_{\mathrm{0}} ^{{arctan}\left(\frac{\sqrt{\mathrm{3}}}{\sqrt{\mathrm{2}}}\right)} \:\:\:\:\frac{\sqrt{\mathrm{2}}\left(\mathrm{1}+{tan}^{\mathrm{2}} \theta\right){d}\theta}{\mathrm{4}\left(\mathrm{1}+{tan}^{\mathrm{2}} \theta\right)^{\mathrm{2}} } \\ $$$$=\frac{\sqrt{\mathrm{2}}}{\mathrm{4}}\int_{\mathrm{0}} ^{{arctan}\left(\frac{\sqrt{\mathrm{3}}}{\sqrt{\mathrm{2}}}\right)} \:\:\:\:{cos}^{\mathrm{2}} \theta\:{d}\theta\:=\frac{\sqrt{\mathrm{2}}}{\mathrm{4}}\:\:\int_{\mathrm{0}} ^{{arctan}\left(\frac{\sqrt{\mathrm{3}}}{\sqrt{\mathrm{2}}}\right)} \frac{\mathrm{1}+{cos}\left(\mathrm{2}\theta\right)}{\mathrm{2}}\:{d}\theta \\ $$$$=\frac{\sqrt{\mathrm{2}}}{\mathrm{8}}\:{arctan}\left(\frac{\sqrt{\mathrm{3}}}{\sqrt{\mathrm{2}}}\right)\:+\frac{\sqrt{\mathrm{2}}}{\mathrm{16}}\:\left[{sin}\left(\mathrm{2}\theta\right)\right]_{\mathrm{0}} ^{{arctan}\left(\frac{\sqrt{\mathrm{3}}}{\sqrt{\mathrm{2}}}\right)} \\ $$$$=\frac{\sqrt{\mathrm{2}}}{\mathrm{8}}\:{arctan}\left(\frac{\sqrt{\mathrm{3}}}{\sqrt{\mathrm{2}}}\right)\:+\frac{\sqrt{\mathrm{2}}}{\mathrm{16}}\:{sin}\left(\mathrm{2}{arctan}\left(\frac{\sqrt{\mathrm{3}}}{\sqrt{\mathrm{2}}}\right)\right).\:\:\:...{be}\:{continued}... \\ $$
