
Question and Answers Forum
Question Number 50825 by behi83417@gmail.com last updated on 20/Dec/18
![x^4 =ax^2 +by^2 y^4 =bx^2 +ay^2 solve for x, y. [a ,b∈ R; a, b≠0]](Q50825.png)
Answered by mr W last updated on 21/Dec/18
![x=y=0 is a solution. let X=x^2 , Y=y^2 X^2 =aX+bY Y^2 =bX+aY (X+Y)^2 −2XY=(a+b)(X+Y) (XY)^2 =ab(X^2 +Y^2 )+(a^2 +b^2 )XY (XY)^2 =ab(X^2 +Y^2 +2XY)+(a^2 +b^2 −2ab)XY (XY)^2 =ab(X+Y)^2 +(a−b)^2 XY letu=X+Y, v=XY u^2 −(a+b)u−2v=0 ⇒v=(([u−(a+b)]u)/2) abu^2 −v^2 +(a−b)^2 v=0 abu^2 −[((u^2 −(a+b)u)/2)]^2 +(a−b)^2 ((u^2 −(a+b)u)/2)=0 4abu^2 −[u^2 −(a+b)u]^2 +2(a−b)^2 [u^2 −(a+b)u]=0 u≠0 (u=0 ⇒x=y=0) 4abu−u^2 +2(a+b)u−(a+b)^2 +2(a−b)^2 u−2(a−b)^2 (a+b)=0 u^2 −2(a+b+a^2 +b^2 )u+[a+b+2(a−b)^2 ](a+b)=0 ⇒u=(a+b+a^2 +b^2 )±(√((a+b+a^2 +b^2 )^2 −(a+b)[a+b+2(a−b)^2 ])) ⇒v=(([u−(a+b)]u)/2) X+Y=u XY=v ⇒X and Y are roots of t^2 −ut+v=0 ⇒X,Y=((u±(√(u^2 −4v)))/2) ⇒x,y=±(√((u±(√(u^2 −4v)))/2))](Q50828.png)
Commented by behi83417@gmail.com last updated on 21/Dec/18
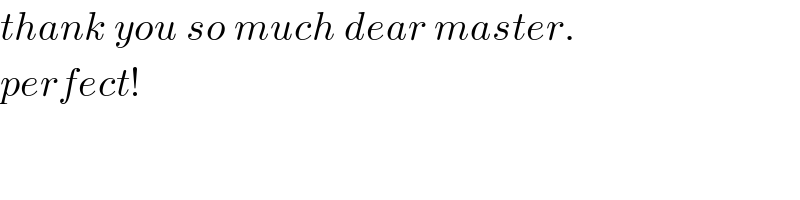
| ||
Question and Answers Forum | ||
Question Number 50825 by behi83417@gmail.com last updated on 20/Dec/18 | ||
![x^4 =ax^2 +by^2 y^4 =bx^2 +ay^2 solve for x, y. [a ,b∈ R; a, b≠0]](Q50825.png) | ||
Answered by mr W last updated on 21/Dec/18 | ||
![x=y=0 is a solution. let X=x^2 , Y=y^2 X^2 =aX+bY Y^2 =bX+aY (X+Y)^2 −2XY=(a+b)(X+Y) (XY)^2 =ab(X^2 +Y^2 )+(a^2 +b^2 )XY (XY)^2 =ab(X^2 +Y^2 +2XY)+(a^2 +b^2 −2ab)XY (XY)^2 =ab(X+Y)^2 +(a−b)^2 XY letu=X+Y, v=XY u^2 −(a+b)u−2v=0 ⇒v=(([u−(a+b)]u)/2) abu^2 −v^2 +(a−b)^2 v=0 abu^2 −[((u^2 −(a+b)u)/2)]^2 +(a−b)^2 ((u^2 −(a+b)u)/2)=0 4abu^2 −[u^2 −(a+b)u]^2 +2(a−b)^2 [u^2 −(a+b)u]=0 u≠0 (u=0 ⇒x=y=0) 4abu−u^2 +2(a+b)u−(a+b)^2 +2(a−b)^2 u−2(a−b)^2 (a+b)=0 u^2 −2(a+b+a^2 +b^2 )u+[a+b+2(a−b)^2 ](a+b)=0 ⇒u=(a+b+a^2 +b^2 )±(√((a+b+a^2 +b^2 )^2 −(a+b)[a+b+2(a−b)^2 ])) ⇒v=(([u−(a+b)]u)/2) X+Y=u XY=v ⇒X and Y are roots of t^2 −ut+v=0 ⇒X,Y=((u±(√(u^2 −4v)))/2) ⇒x,y=±(√((u±(√(u^2 −4v)))/2))](Q50828.png) | ||
| ||
Commented by behi83417@gmail.com last updated on 21/Dec/18 | ||
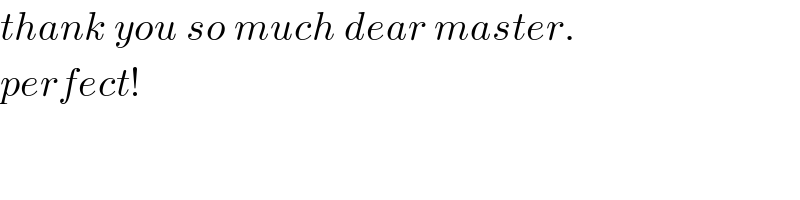 | ||