Question Number 50898 by ajfour last updated on 21/Dec/18
Commented by ajfour last updated on 21/Dec/18
$${If}\:{length}\:{of}\:{BP}\:\:{is}\:{maximum} \\ $$$${and}\:{equal}\:{to}\:\boldsymbol{{l}},\:{and}\:{the}\:{two}\:{coloured} \\ $$$${areas}\:{equal},\:{find}\:\boldsymbol{{a}}\:{and}\:\boldsymbol{{b}}\:{of}\:{ellipse}. \\ $$
Answered by ajfour last updated on 21/Dec/18
$${i}\:{got}\:\:{a}\:=\:{l}\sqrt{\mathrm{2}−\sqrt{\mathrm{2}}}\:\:\:,\:\:{b}\:=\:{l}\sqrt{\mathrm{3}\sqrt{\mathrm{2}}−\mathrm{4}}\:\:. \\ $$
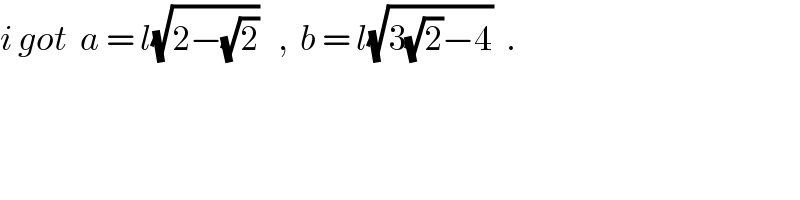
Commented by mr W last updated on 22/Dec/18
$${i}\:{got}\:{the}\:{same}\:{sir}. \\ $$
Answered by tanmay.chaudhury50@gmail.com last updated on 22/Dec/18
![area sky=(1/2)×acosθ×bsinθ+∫_(bsinθ) ^b (a/b)(√(b^2 −y^2 )) dy =(a/b)[∣(y/2)(√(b^2 −y^2 )) +(b^2 /2)sin^(−1) ((y/b))∣_(bsinθ) ^b ]+(1/4)sin2θ =(a/b)[((b^2 /2)×(π/2))−(((bsinθ×bcosθ)/2)+(b^2 /2)θ)]+((sin2θ)/4) =((πab)/4)−((absin2θ)/4)+((abθ)/2)+((sin2θ)/4) given A_s =A_p =((πab)/8) at θ=θ_0 ((πab)/8)=((πab)/4)−((absin2θ_0 )/4)+((abθ_0 )/2)+((sin2θ_0 )/4) sin2θ_0 ×(1/4)(ab−1)−((ab)/2)sin^(−1) ((b^2 /(a^2 −b^2 )))=((πab)/8) length BP=(√((acosθ−0)^2 +(bsinθ+b)^2 )) =s s_(max) =l given s^2 =a^2 cos^2 θ+b^2 (1+sinθ)^2 2s×(ds/dθ)=a^2 ×−sin2θ+b^2 ×2(1+sinθ)(cosθ) 2b^2 cosθ+b^2 sin2θ−a^2 sin2θ=0 cosθ[2b^2 +2b^2 sinθ−2a^2 sinθ]=0 cosθ=0 θ=(π/2)←ignored sinθ_0 =(b^2 /(a^2 −b^2 )) cosθ_0 =((√((a^2 −b^2 )^2 −b^4 ))/((a^2 −b^2 )))=((√(a^4 −2a^2 b^2 ))/((a^2 −b^2 ))) wait...](Q50916.png)
$${area}\:{sky}=\frac{\mathrm{1}}{\mathrm{2}}×{acos}\theta×{bsin}\theta+\int_{{bsin}\theta} ^{{b}} \frac{{a}}{{b}}\sqrt{{b}^{\mathrm{2}} −{y}^{\mathrm{2}} }\:{dy} \\ $$$$=\frac{{a}}{{b}}\left[\mid\frac{{y}}{\mathrm{2}}\sqrt{{b}^{\mathrm{2}} −{y}^{\mathrm{2}} }\:+\frac{{b}^{\mathrm{2}} }{\mathrm{2}}{sin}^{−\mathrm{1}} \left(\frac{{y}}{{b}}\right)\mid_{{bsin}\theta} ^{{b}} \right]+\frac{\mathrm{1}}{\mathrm{4}}{sin}\mathrm{2}\theta \\ $$$$=\frac{{a}}{{b}}\left[\left(\frac{{b}^{\mathrm{2}} }{\mathrm{2}}×\frac{\pi}{\mathrm{2}}\right)−\left(\frac{{bsin}\theta×{bcos}\theta}{\mathrm{2}}+\frac{{b}^{\mathrm{2}} }{\mathrm{2}}\theta\right)\right]+\frac{{sin}\mathrm{2}\theta}{\mathrm{4}} \\ $$$$=\frac{\pi{ab}}{\mathrm{4}}−\frac{{absin}\mathrm{2}\theta}{\mathrm{4}}+\frac{{ab}\theta}{\mathrm{2}}+\frac{{sin}\mathrm{2}\theta}{\mathrm{4}} \\ $$$${given}\:{A}_{{s}} ={A}_{{p}} =\frac{\pi{ab}}{\mathrm{8}}\:{at}\:\theta=\theta_{\mathrm{0}} \\ $$$$\frac{\pi{ab}}{\mathrm{8}}=\frac{\pi{ab}}{\mathrm{4}}−\frac{{absin}\mathrm{2}\theta_{\mathrm{0}} }{\mathrm{4}}+\frac{{ab}\theta_{\mathrm{0}} }{\mathrm{2}}+\frac{{sin}\mathrm{2}\theta_{\mathrm{0}} }{\mathrm{4}} \\ $$$${sin}\mathrm{2}\theta_{\mathrm{0}} ×\frac{\mathrm{1}}{\mathrm{4}}\left({ab}−\mathrm{1}\right)−\frac{{ab}}{\mathrm{2}}{sin}^{−\mathrm{1}} \left(\frac{{b}^{\mathrm{2}} }{{a}^{\mathrm{2}} −{b}^{\mathrm{2}} }\right)=\frac{\pi{ab}}{\mathrm{8}} \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$${length}\:{BP}=\sqrt{\left({acos}\theta−\mathrm{0}\right)^{\mathrm{2}} +\left({bsin}\theta+{b}\right)^{\mathrm{2}} }\:={s} \\ $$$${s}_{{max}} ={l}\:{given} \\ $$$${s}^{\mathrm{2}} ={a}^{\mathrm{2}} {cos}^{\mathrm{2}} \theta+{b}^{\mathrm{2}} \left(\mathrm{1}+{sin}\theta\right)^{\mathrm{2}} \\ $$$$\mathrm{2}{s}×\frac{{ds}}{{d}\theta}={a}^{\mathrm{2}} ×−{sin}\mathrm{2}\theta+{b}^{\mathrm{2}} ×\mathrm{2}\left(\mathrm{1}+{sin}\theta\right)\left({cos}\theta\right) \\ $$$$\mathrm{2}{b}^{\mathrm{2}} {cos}\theta+{b}^{\mathrm{2}} {sin}\mathrm{2}\theta−{a}^{\mathrm{2}} {sin}\mathrm{2}\theta=\mathrm{0} \\ $$$${cos}\theta\left[\mathrm{2}{b}^{\mathrm{2}} +\mathrm{2}{b}^{\mathrm{2}} {sin}\theta−\mathrm{2}{a}^{\mathrm{2}} {sin}\theta\right]=\mathrm{0}\:\: \\ $$$${cos}\theta=\mathrm{0}\:\:\theta=\frac{\pi}{\mathrm{2}}\leftarrow{ignored} \\ $$$${sin}\theta_{\mathrm{0}} =\frac{{b}^{\mathrm{2}} }{{a}^{\mathrm{2}} −{b}^{\mathrm{2}} }\:\:{cos}\theta_{\mathrm{0}} =\frac{\sqrt{\left({a}^{\mathrm{2}} −{b}^{\mathrm{2}} \right)^{\mathrm{2}} −{b}^{\mathrm{4}} }}{\left({a}^{\mathrm{2}} −{b}^{\mathrm{2}} \right)}=\frac{\sqrt{{a}^{\mathrm{4}} −\mathrm{2}{a}^{\mathrm{2}} {b}^{\mathrm{2}} }}{\left({a}^{\mathrm{2}} −{b}^{\mathrm{2}} \right)} \\ $$$${wait}... \\ $$$$\: \\ $$$$\:\: \\ $$$$ \\ $$$$ \\ $$
Answered by mr W last updated on 22/Dec/18
Commented by OTCHRRE ABDULLAI last updated on 22/Dec/18
$${My}\:\:{man}\:{you}\:{are}\:{too}\:{good} \\ $$$${please}\:{you}\:{arefrom}\:{which}\:{country}? \\ $$
Commented by mr W last updated on 22/Dec/18
![r^2 =((a^2 b^2 )/(a^2 sin^2 θ+b^2 cos^2 θ)) A_(red) =A_(blue) =((πab)/8) A_(red) =∫_0 ^θ ((r^2 dθ)/2)=((a^2 b^2 )/2)∫_0 ^θ (dθ/(a^2 sin^2 θ+b^2 cos^2 θ)) =((a^2 b^2 )/2)×((tan^(−1) ((a/b)tan θ))/(ab)) =((ab)/2)×tan^(−1) ((a/b)tan θ)=((πab)/8) ⇒tan^(−1) ((a/b)tan θ)=(π/4) ⇒(a/b)tan θ=1 ⇒tan θ=(b/a) ⇒ sin θ=(b/(√(a^2 +b^2 ))) and cos θ=(a/(√(a^2 +b^2 ))) P(r,θ) r^2 =((a^2 b^2 )/(a^2 sin^2 θ+b^2 cos^2 θ))=((a^2 b^2 )/((2a^2 b^2 )/(a^2 +b^2 )))=((a^2 +b^2 )/2) (x/a^2 )+(y/b^2 )y′=0 ((r cos θ)/a^2 )+((r sin θ)/b^2 )y′=0 ⇒y′(at P)=−(b^2 /(a^2 tan θ))=−(b/a)=−tan α=−tan θ ⇒α=θ ϕ=(π/2)−α=(π/2)−θ r cos θ=l cos ϕ=l sin θ ⇒r=l tan θ=((lb)/a) r sin θ=l sin ϕ−b=l cos θ−b ⇒r=(l/(tan θ))−(b/(sin θ)) ⇒(l/(tan θ))−(b/(sin θ))=l tan θ ⇒b=l((1/(tan θ))−tan θ)sin θ=l×((a^2 −b^2 )/(ab))×(b/(√(a^2 +b^2 ))) ⇒l=((ab(√(a^2 +b^2 )))/(a^2 −b^2 )) r^2 =((l^2 b^2 )/a^2 )=(b^2 /a^2 )×((a^2 b^2 (a^2 +b^2 ))/((a^2 −b^2 )^2 ))=((b^4 (a^2 +b^2 ))/((a^2 −b^2 )^2 ))=((a^2 +b^2 )/2) ⇒(b^4 /((a^2 −b^2 )^2 ))=(1/2) ((√2)b^2 )^2 −(a^2 −b^2 )^2 =0 [((√2)+1)b^2 −a^2 ][((√2)−1)b^2 +a^2 ]=0 ⇒a^2 =((√2)+1)b^2 ⇒l=((ab(√(a^2 +b^2 )))/(a^2 −b^2 ))=((ab(√((2+(√2))b^2 )))/((√2)b^2 ))=a(√((2+(√2))/2)) ⇒a=(√(2/(2+(√2)))) l=(√(2−(√2))) l ⇒b=(a/(√((√2)+1)))=(√((2−(√2))/((√2)+1))) l=(√(3(√2)−4)) l](Q50945.png)
$${r}^{\mathrm{2}} =\frac{{a}^{\mathrm{2}} {b}^{\mathrm{2}} }{{a}^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \:\theta+{b}^{\mathrm{2}} \mathrm{cos}^{\mathrm{2}} \:\theta} \\ $$$${A}_{{red}} ={A}_{{blue}} =\frac{\pi{ab}}{\mathrm{8}} \\ $$$${A}_{{red}} =\int_{\mathrm{0}} ^{\theta} \frac{{r}^{\mathrm{2}} {d}\theta}{\mathrm{2}}=\frac{{a}^{\mathrm{2}} {b}^{\mathrm{2}} }{\mathrm{2}}\int_{\mathrm{0}} ^{\theta} \frac{{d}\theta}{{a}^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \:\theta+{b}^{\mathrm{2}} \mathrm{cos}^{\mathrm{2}} \:\theta} \\ $$$$=\frac{{a}^{\mathrm{2}} {b}^{\mathrm{2}} }{\mathrm{2}}×\frac{\mathrm{tan}^{−\mathrm{1}} \left(\frac{{a}}{{b}}\mathrm{tan}\:\theta\right)}{{ab}} \\ $$$$=\frac{{ab}}{\mathrm{2}}×\mathrm{tan}^{−\mathrm{1}} \left(\frac{{a}}{{b}}\mathrm{tan}\:\theta\right)=\frac{\pi{ab}}{\mathrm{8}} \\ $$$$\Rightarrow\mathrm{tan}^{−\mathrm{1}} \left(\frac{{a}}{{b}}\mathrm{tan}\:\theta\right)=\frac{\pi}{\mathrm{4}} \\ $$$$\Rightarrow\frac{{a}}{{b}}\mathrm{tan}\:\theta=\mathrm{1} \\ $$$$\Rightarrow\mathrm{tan}\:\theta=\frac{{b}}{{a}}\:\Rightarrow\:\mathrm{sin}\:\theta=\frac{{b}}{\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }}\:{and}\:\mathrm{cos}\:\theta=\frac{{a}}{\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }} \\ $$$${P}\left({r},\theta\right) \\ $$$${r}^{\mathrm{2}} =\frac{{a}^{\mathrm{2}} {b}^{\mathrm{2}} }{{a}^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \:\theta+{b}^{\mathrm{2}} \mathrm{cos}^{\mathrm{2}} \:\theta}=\frac{{a}^{\mathrm{2}} {b}^{\mathrm{2}} }{\frac{\mathrm{2}{a}^{\mathrm{2}} {b}^{\mathrm{2}} }{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }}=\frac{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }{\mathrm{2}} \\ $$$$\frac{{x}}{{a}^{\mathrm{2}} }+\frac{{y}}{{b}^{\mathrm{2}} }{y}'=\mathrm{0} \\ $$$$\frac{{r}\:\mathrm{cos}\:\theta}{{a}^{\mathrm{2}} }+\frac{{r}\:\mathrm{sin}\:\theta}{{b}^{\mathrm{2}} }{y}'=\mathrm{0} \\ $$$$\Rightarrow{y}'\left({at}\:{P}\right)=−\frac{{b}^{\mathrm{2}} }{{a}^{\mathrm{2}} \:\mathrm{tan}\:\theta}=−\frac{{b}}{{a}}=−\mathrm{tan}\:\alpha=−\mathrm{tan}\:\theta \\ $$$$\Rightarrow\alpha=\theta \\ $$$$\varphi=\frac{\pi}{\mathrm{2}}−\alpha=\frac{\pi}{\mathrm{2}}−\theta \\ $$$${r}\:\mathrm{cos}\:\theta={l}\:\mathrm{cos}\:\varphi={l}\:\mathrm{sin}\:\theta \\ $$$$\Rightarrow{r}={l}\:\mathrm{tan}\:\theta=\frac{{lb}}{{a}} \\ $$$${r}\:\mathrm{sin}\:\theta={l}\:\mathrm{sin}\:\varphi−{b}={l}\:\mathrm{cos}\:\theta−{b} \\ $$$$\Rightarrow{r}=\frac{{l}}{\mathrm{tan}\:\theta}−\frac{{b}}{\mathrm{sin}\:\theta} \\ $$$$\Rightarrow\frac{{l}}{\mathrm{tan}\:\theta}−\frac{{b}}{\mathrm{sin}\:\theta}={l}\:\mathrm{tan}\:\theta \\ $$$$\Rightarrow{b}={l}\left(\frac{\mathrm{1}}{\mathrm{tan}\:\theta}−\mathrm{tan}\:\theta\right)\mathrm{sin}\:\theta={l}×\frac{{a}^{\mathrm{2}} −{b}^{\mathrm{2}} }{{ab}}×\frac{{b}}{\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }} \\ $$$$\Rightarrow{l}=\frac{{ab}\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }}{{a}^{\mathrm{2}} −{b}^{\mathrm{2}} } \\ $$$${r}^{\mathrm{2}} =\frac{{l}^{\mathrm{2}} {b}^{\mathrm{2}} }{{a}^{\mathrm{2}} }=\frac{{b}^{\mathrm{2}} }{{a}^{\mathrm{2}} }×\frac{{a}^{\mathrm{2}} {b}^{\mathrm{2}} \left({a}^{\mathrm{2}} +{b}^{\mathrm{2}} \right)}{\left({a}^{\mathrm{2}} −{b}^{\mathrm{2}} \right)^{\mathrm{2}} }=\frac{{b}^{\mathrm{4}} \left({a}^{\mathrm{2}} +{b}^{\mathrm{2}} \right)}{\left({a}^{\mathrm{2}} −{b}^{\mathrm{2}} \right)^{\mathrm{2}} }=\frac{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }{\mathrm{2}} \\ $$$$\Rightarrow\frac{{b}^{\mathrm{4}} }{\left({a}^{\mathrm{2}} −{b}^{\mathrm{2}} \right)^{\mathrm{2}} }=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\left(\sqrt{\mathrm{2}}{b}^{\mathrm{2}} \right)^{\mathrm{2}} −\left({a}^{\mathrm{2}} −{b}^{\mathrm{2}} \right)^{\mathrm{2}} =\mathrm{0} \\ $$$$\left[\left(\sqrt{\mathrm{2}}+\mathrm{1}\right){b}^{\mathrm{2}} −{a}^{\mathrm{2}} \right]\left[\left(\sqrt{\mathrm{2}}−\mathrm{1}\right){b}^{\mathrm{2}} +{a}^{\mathrm{2}} \right]=\mathrm{0} \\ $$$$\Rightarrow{a}^{\mathrm{2}} =\left(\sqrt{\mathrm{2}}+\mathrm{1}\right){b}^{\mathrm{2}} \\ $$$$\Rightarrow{l}=\frac{{ab}\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }}{{a}^{\mathrm{2}} −{b}^{\mathrm{2}} }=\frac{{ab}\sqrt{\left(\mathrm{2}+\sqrt{\mathrm{2}}\right){b}^{\mathrm{2}} }}{\sqrt{\mathrm{2}}{b}^{\mathrm{2}} }={a}\sqrt{\frac{\mathrm{2}+\sqrt{\mathrm{2}}}{\mathrm{2}}} \\ $$$$\Rightarrow{a}=\sqrt{\frac{\mathrm{2}}{\mathrm{2}+\sqrt{\mathrm{2}}}}\:{l}=\sqrt{\mathrm{2}−\sqrt{\mathrm{2}}}\:{l} \\ $$$$\Rightarrow{b}=\frac{{a}}{\sqrt{\sqrt{\mathrm{2}}+\mathrm{1}}}=\sqrt{\frac{\mathrm{2}−\sqrt{\mathrm{2}}}{\sqrt{\mathrm{2}}+\mathrm{1}}}\:{l}=\sqrt{\mathrm{3}\sqrt{\mathrm{2}}−\mathrm{4}}\:{l} \\ $$
Commented by ajfour last updated on 22/Dec/18
$$\mathscr{W}\mathcal{O}\mathscr{W}\:! \\ $$
Commented by mr W last updated on 22/Dec/18
Commented by mr W last updated on 22/Dec/18
$${diagram}\:{shows}\:{the}\:{case}\:{l}=\mathrm{5}. \\ $$
Commented by ajfour last updated on 22/Dec/18
$${Too}\:{Good}\:{Sir},\:{Thanks}\:{for}\:{confirming}. \\ $$
Commented by OTCHRRE ABDULLAI last updated on 23/Dec/18
$${You}\:{are}\:{world}\:{best}\:{my}\:{man}\: \\ $$$${if}\:{mathematics}\:{is}\:{football}\:{you}\:{will}\: \\ $$$${be}\:{messi}\:{or}\:{Ronaldo}\:{i}\:{really}\:{like}\: \\ $$$${you}\:{infact}\:{you}\:{are}\:{now}\:{my}\:{best}\: \\ $$$${friend} \\ $$
Commented by peter frank last updated on 22/Dec/18
$${and}\:{physics}\:{too} \\ $$