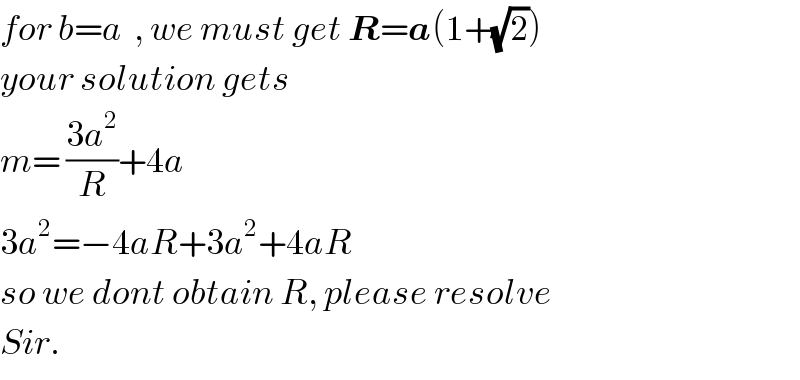Math Question and Answers


Question and Answers Forum

All Questions Topic List
Geometry Questions
Previous in All Question Next in All Question
Previous in Geometry Next in Geometry
Question Number 51422 by ajfour last updated on 26/Dec/18

Commented by ajfour last updated on 26/Dec/18

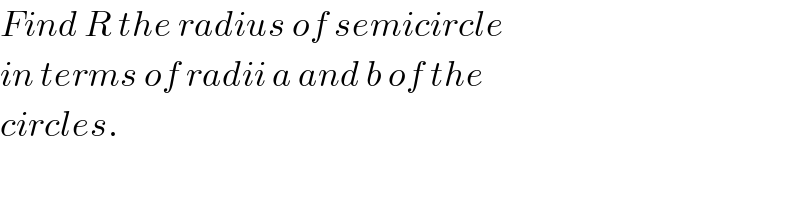
$F i n d R t h e r a d i u s o f s e m i c i r c l e$ $i n t e r m s o f r a d i i a a n d b o f t h e$ $c i r c l e s .$
	Answered by ajfour last updated on 26/Dec/18
	$let sin θ = (a/(R−a)) , sin φ = (b/(R−b)) (R−a)cos θ+(R−b)cos φ = (√(4ab)) ⇒ (√(R(R−2a)))+(√(R(R−2b))) = (√(4ab)) squaring 2R^2 −2(a+b)R +2(√(R^2 {R^2 −2(a+b)R+4ab})) = 4ab rearranging and squaring R^2 {R^2 −2(a+b)R+4ab} = {2ab−R^2 +(a+b)R}^2 ⇒ 4abR^2 = 4a^2 b^2 +(a+b)^2 R^2 −4abR^2 +4ab(a+b)R ⇒ (a^2 +b^2 −6ab)R^2 +4ab(a+b)R +4a^2 b^2 = 0 ⇒ R = ((−2ab(a+b)−(√(4a^2 b^2 (8ab))))/(a^2 +b^2 −6ab)) or R = [(√(8ab))+(a+b)](((2ab)/(6ab−a^2 −b^2 ))) .$
	$l e t \sin θ = \frac{a}{R - a}, \sin ϕ = \frac{b}{R - b}$ $(R - a) \cos θ + (R - b) \cos ϕ = \sqrt{4 a b}$ $\Rightarrow \sqrt{R (R - 2 a)} + \sqrt{R (R - 2 b)} = \sqrt{4 a b}$ $s q u a r i n g$ $2 R^{2} - 2 (a + b) R + 2 \sqrt{R^{2} {R^{2} - 2 (a + b) R + 4 a b}} = 4 a b$ $r e a r r a n g i n g a n d s q u a r i n g$ $R^{2} {R^{2} - 2 (a + b) R + 4 a b}$ $= {2 a b - R^{2} + (a + b) R}^{2}$ $\Rightarrow 4 {a b R}^{2} = 4 a^{2} b^{2} + {(a + b)}^{2} R^{2}$ $- 4 {a b R}^{2} + 4 a b (a + b) R$ $\Rightarrow (a^{2} + b^{2} - 6 a b) R^{2} + 4 a b (a + b) R$ $+ 4 a^{2} b^{2} = 0$ $\Rightarrow R = \frac{- 2 a b (a + b) - \sqrt{4 a^{2} b^{2} (8 a b)}}{a^{2} + b^{2} - 6 a b}$ $o r R = [\sqrt{8 a b} + (a + b)] (\frac{2 a b}{6 a b - a^{2} - b^{2}}) .$
	Answered by behi83417@gmail.com last updated on 27/Dec/18

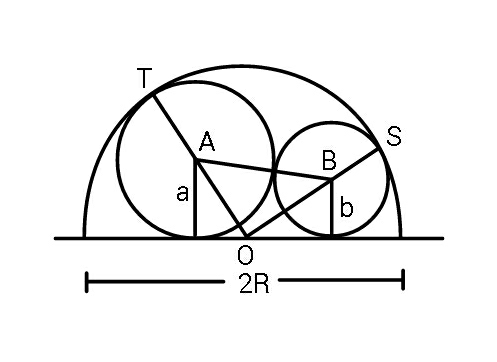
	$\Rightarrow A B = \sqrt{2 (a^{2} + b^{2})}$ $T O = O S = R$ $O A = R - a, O B = R - b$ $s i n α = \frac{a}{R - a}, s i n β = \frac{b}{R - b}$ $c o s α = \frac{\sqrt{{(R - a)}^{2} - a^{2}}}{R - a}, c o s β = \frac{\sqrt{{(R - b)}^{2} - b^{2}}}{R - b}$ $c o s φ = c o s (π - α - β) = - c o s (α + β) =$ $= \frac{a b - \sqrt{R^{2} - 2 R a} . \sqrt{R^{2} - 2 R b}}{(R - a) (R - b)} (i)$ $c o s φ = \frac{{O A}^{2} + {O B}^{2} - {A B}^{2}}{2 O A . O B} = \frac{{(R - a)}^{2} + {(R - b)}^{2} - 2 (a^{2} + b^{2})}{2 (R - a) (R - b)} =$ $= \frac{2 R^{2} - 2 R a - 2 R b - a^{2} - b^{2}}{2 (R - a) (R - b)} (i i)$ $i = i i \Rightarrow$ $2 R^{2} - 2 R a - 2 R b - a^{2} - b^{2} =$ $= a b - \sqrt{R^{2} - 2 R a} . \sqrt{R^{2} - 2 R b}$ $a^{2} + b^{2} + a b + 2 R a + 2 R b - 2 R^{2} = \sqrt{R^{2} - 2 R a} . \sqrt{R^{2} - 2 R b}$ $R^{2} - 2 R a = p, R^{2} - 2 R b = q, a^{2} + b^{2} + a b = t$ $a^{2} + b^{2} + a b - (p + q) = \sqrt{p q}$ $t - (p + q) = \sqrt{p q} \Rightarrow t^{2} - 2 t (p + q) + {(p + q)}^{2} = p q$ $p + q = 2 R^{2} - 2 R a - 2 R b$ ${(p + q)}^{2} = 4 R^{4} + 4 R^{2} a^{2} + 4 R^{2} b^{2} - 8 R^{3} a - 8 R^{3} b + 8 R^{2} a b$ $p q = (R^{2} - 2 R a) (R^{2} - 2 R b) = R^{4} - 2 R^{3} b - 2 R^{3} a + 4 R^{2} a b$ $t^{2} - 4 R (R - a - b) t + 3 R^{4} + 4 R^{2} (a^{2} + b^{2} + a b)$ $- 6 R^{3} a - 6 R^{3} b = 0$ $t^{2} + 4 R (a + b) t + 3 R^{3} (R - 2 a - 2 b) = 0$ $\Rightarrow △^{'} = 4 R^{2} {(a + b)}^{2} - 3 R^{3} (R - 2 a - 2 b) =$ $= R^{2} [4 {(a + b)}^{2} - 3 R^{2} + 6 R (a + b)] = R^{2} m^{2}$ $a^{2} + b^{2} + a b = - 2 R (a + b) + R m$ $m = \frac{a^{2} + b^{2} + a b}{R} + 2 (a + b)$
	Commented by ajfour last updated on 27/Dec/18

	$f o r b = a, w e m u s t g e t R = a (1 + \sqrt{2})$ $y o u r s o l u t i o n g e t s$ $m = \frac{3 a^{2}}{R} + 4 a$ $3 a^{2} = - 4 a R + 3 a^{2} + 4 a R$ $s o w e d o n t o b t a i n R, p l e a s e r e s o l v e$ $S i r .$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum