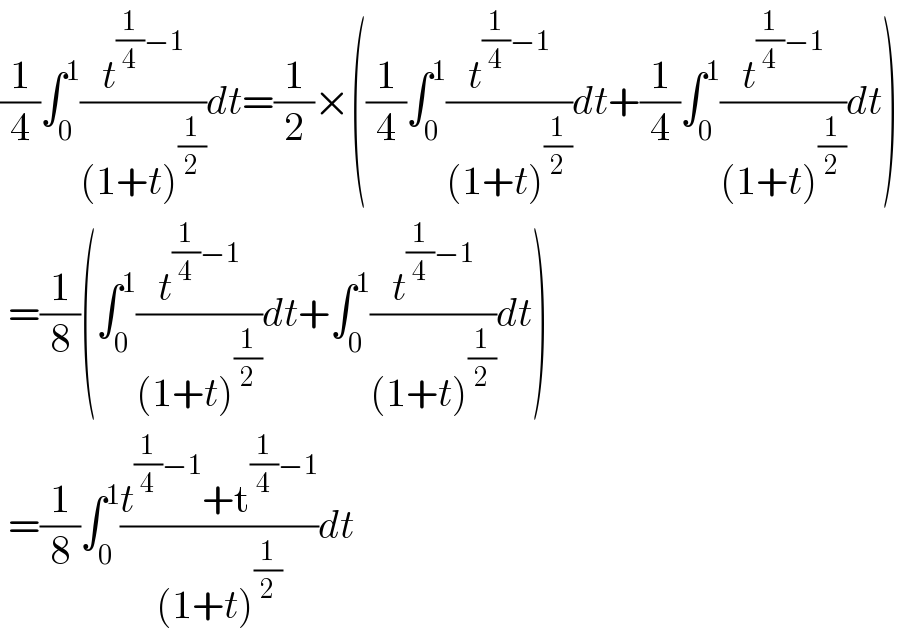Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 109616 by mathmax by abdo last updated on 24/Aug/20

$$\mathrm{find}{\int }_0^1\sqrt{1+{\mathrm{x}}^4}\mathrm{dx}$$

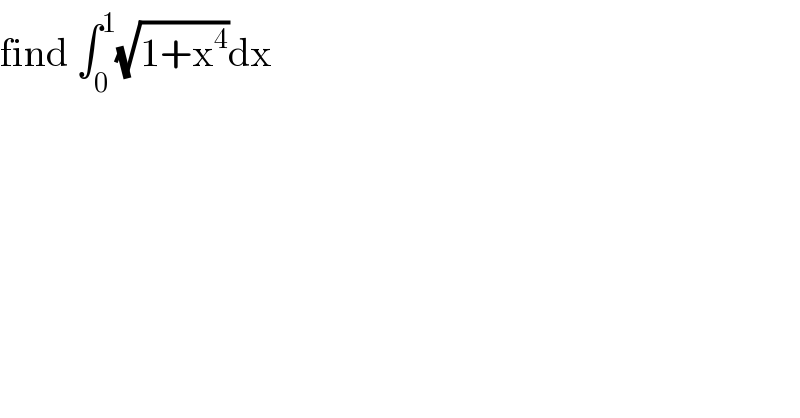
Commented by mathdave last updated on 24/Aug/20

$$thisishypergeometricquestionorquestion$$

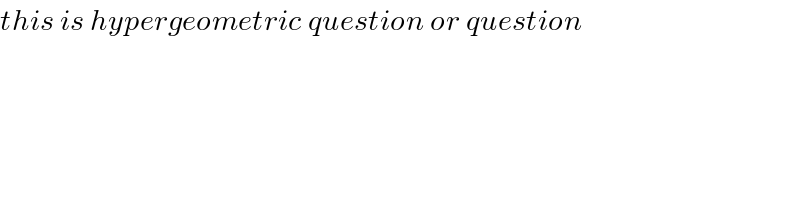
Answered by mathmax by abdo last updated on 25/Aug/20

$$\mathrm{A}={\int }_0^1\sqrt{1+{\mathrm{x}}^4}\mathrm{dx}\mathrm{by}\mathrm{parts}\mathrm{A}={\left[\mathrm{x}\sqrt{1+{\mathrm{x}}^4}\right]}_0^1-{\int }_0^1\mathrm{x}.\frac{{4\mathrm{x}}^3}{2\sqrt{1+{\mathrm{x}}^4}}\mathrm{dx}$$$$=\sqrt{2}-2{\int }_0^1\frac{{\mathrm{x}}^4}{\sqrt{1+{\mathrm{x}}^4}}\mathrm{dx}=\sqrt{2}-2{\int }_0^1\frac{{\mathrm{x}}^4+1-1}{\sqrt{1+{\mathrm{x}}^4}}\mathrm{dx}$$$$=\sqrt{2}-2{\int }_0^1\sqrt{1+{\mathrm{x}}^4}\mathrm{dx}+2{\int }_0^1\frac{\mathrm{dx}}{{(1+{\mathrm{x}}^4)}^{\frac{1}{2}}}\Rightarrow$$$$3\mathrm{A}=\sqrt{2}+2{\int }_0^1\frac{\mathrm{dx}}{{(1+{\mathrm{x}}^4)}^{\frac{1}{2}}}\mathrm{changement}\mathrm{x}={\mathrm{t}}^{\frac{1}{4}}\mathrm{give}$$$${\int }_0^1\frac{\mathrm{dx}}{{(1+{\mathrm{x}}^4)}^{\frac{1}{2}}}=\frac{1}{4}{\int }_0^1\frac{{\mathrm{t}}^{\frac{1}{4}-1}}{{(1+\mathrm{t})}^{\frac{1}{2}}}\mathrm{dt}\mathrm{we}\mathrm{know}\mathrm{B}(\mathrm{p},\mathrm{q})={\int }_0^1\frac{{\mathrm{t}}^{\mathrm{p}-1}+{\mathrm{t}}^{\mathrm{q}-1}}{{(1+\mathrm{t})}^{\mathrm{p}+\mathrm{q}}}\mathrm{dt}$$$$=\frac{1}{8}{\int }_0^1\frac{{\mathrm{t}}^{\frac{1}{4}-1}+{\mathrm{t}}^{\frac{1}{4}-1}}{{(1+\mathrm{t})}^{\frac{1}{2}}}(\mathrm{p}=\mathrm{q}=\frac{1}{4})=\frac{1}{8}\mathrm{B}(\frac{1}{4},\frac{1}{4})=\frac{1}{8}.\frac{\mathrm{\Gamma }\left(\frac{1}{4}\right)\mathrm{\Gamma }\left(\frac{1}{4}\right)}{\mathrm{\Gamma }\left(\frac{1}{2}\right)}$$$$=\frac{{\mathrm{\Gamma }}^2\left(\frac{1}{4}\right)}{8\sqrt{\pi }}\Rightarrow 3\mathrm{A}=\sqrt{2}+2\times \frac{{\mathrm{\Gamma }}^2\left(\frac{1}{4}\right)}{8\sqrt{\pi }}=\sqrt{2}+\frac{{\mathrm{\Gamma }}^2\left(\frac{1}{4}\right)}{4\sqrt{\pi }}\Rightarrow$$$$\mathrm{A}=\frac{\sqrt{2}}{3}+\frac{{\mathrm{\Gamma }}^2\left(\frac{1}{4}\right)}{12\sqrt{\pi }}$$

Commented by mathdave last updated on 25/Aug/20

$$howdidyougetthe\frac{1}{8}whenitwas\frac{1}{4}{\int }_0^1\frac{t^{\frac{1}{4}-1}}{{(1+t)}^{\frac{1}{2}}}dt$$$$has\frac{1}{2}didntfollowbetaformularyoustated$$

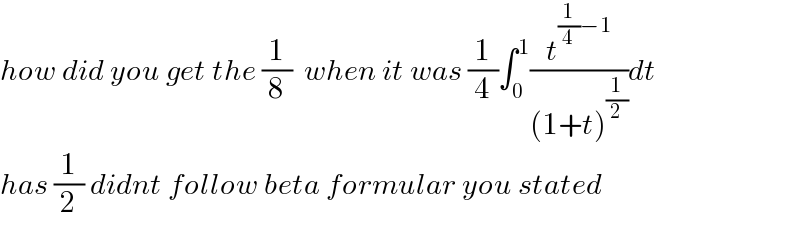
Commented by Aziztisffola last updated on 25/Aug/20

$$\frac{1}{4}{\int }_0^1\frac{t^{\frac{1}{4}-1}}{{(1+t)}^{\frac{1}{2}}}dt=\frac{1}{2}\times (\frac{1}{4}{\int }_0^1\frac{t^{\frac{1}{4}-1}}{{(1+t)}^{\frac{1}{2}}}dt+\frac{1}{4}{\int }_0^1\frac{t^{\frac{1}{4}-1}}{{(1+t)}^{\frac{1}{2}}}dt)$$$$=\frac{1}{8}({\int }_0^1\frac{t^{\frac{1}{4}-1}}{{(1+t)}^{\frac{1}{2}}}dt+{\int }_0^1\frac{t^{\frac{1}{4}-1}}{{(1+t)}^{\frac{1}{2}}}dt)$$$$=\frac{1}{8}{\int }_0^1\frac{t^{\frac{1}{4}-1}+{\mathrm{t}}^{\frac{1}{4}-1}}{{(1+t)}^{\frac{1}{2}}}dt$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com