Question Number 52214 by Tawa1 last updated on 04/Jan/19
Commented by Tawa1 last updated on 04/Jan/19
$$\mathrm{Evaluate} \\ $$
Commented by mr W last updated on 05/Jan/19
$${i}\:{know}\:{it}'{s}\:\frac{\mathrm{9}}{\mathrm{32}} \\ $$$${but}\:{i}\:{can}'{t}\:{prove}. \\ $$
Commented by rahul 19 last updated on 05/Jan/19
Yes Sir! U r right . Just interchange the summation (as they are independent) , call them as EQ. (1) & (2) and add them...��
Commented by Tawa1 last updated on 05/Jan/19
$$\mathrm{Please}\:\mathrm{show}\:\mathrm{it}\:\mathrm{sir} \\ $$
Answered by tanmay.chaudhury50@gmail.com last updated on 05/Jan/19
$${T}_{{m},{n}} =\frac{\frac{{m}}{\mathrm{3}^{{m}} }}{\left(\frac{\mathrm{3}^{{m}} }{{m}}+\frac{\mathrm{3}^{{n}} }{{n}}\right)} \\ $$$${since}\:{both}\:{m}\rightarrow\infty \\ $$$${and}\:{n}\rightarrow\infty \\ $$$${and}\:\:{value}\:{of}\:\frac{\mathrm{3}^{{m}} }{{m}}=\frac{\mathrm{3}^{{n}} }{{n}}\:{when}\:{we}\:{put}\:{m}=\mathrm{1},\mathrm{2},\mathrm{3}... \\ $$$${n}=\mathrm{1},\mathrm{2},\mathrm{3}...{simutaneously} \\ $$$${so}\: \\ $$$${T}_{{m},{n}} ={T}_{{r}\:} =\frac{\frac{{r}}{\mathrm{3}^{{r}} }}{\mathrm{2}×\frac{\mathrm{3}^{{r}} }{{r}}}=\frac{\mathrm{1}}{\mathrm{2}}×\left(\frac{{r}}{\mathrm{3}^{{r}} }\right)^{\mathrm{2}} \\ $$$$\boldsymbol{{s}}=\boldsymbol{{T}}_{\mathrm{1}} +{T}_{\mathrm{2}} +{T}_{\mathrm{3}} +...\infty \\ $$$${S}=\frac{\mathrm{1}}{\mathrm{2}}\left[\left(\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{1}} }\right)^{\mathrm{2}} +\left(\frac{\mathrm{2}}{\mathrm{3}^{\mathrm{2}} }\right)^{\mathrm{2}} +\left(\frac{\mathrm{3}}{\mathrm{3}^{\mathrm{3}} }\right)^{\mathrm{2}} +...\infty\right. \\ $$$${wait}... \\ $$
Commented by mr W last updated on 05/Jan/19
$${it}'{s}\:{not}\:{correct}\:{sir}. \\ $$$${you}\:{can}\:{not}\:{transfer}\:{T}_{\infty,\infty} \:{to}\:{T}_{{m},{n}} . \\ $$$$\underset{{m}=\mathrm{1}} {\overset{\infty} {\sum}}\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}{a}_{{m},{n}} =\left({a}_{\mathrm{1},\mathrm{1}} +{a}_{\mathrm{1},\mathrm{2}} +...\right)+\left({a}_{\mathrm{2},\mathrm{1}} +{a}_{\mathrm{2},\mathrm{3}} +...\right)+\left(.....\right)+ \\ $$$$=\left(\infty\:{numbers}\right)+\left(\infty\:{numbers}\right)+\left(...\right)+ \\ $$$$=\infty×\infty\:{numbers} \\ $$$$ \\ $$$${e}.{g}. \\ $$$$\underset{{m}=\mathrm{1}} {\overset{\infty} {\sum}}\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{m}^{\mathrm{2}} +{n}^{\mathrm{2}} }\neq\frac{\mathrm{1}}{\mathrm{2}}\underset{{r}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{r}^{\mathrm{2}} } \\ $$$$ \\ $$$${to}\:{check}: \\ $$$${S}=\frac{\mathrm{1}}{\mathrm{2}}\left[\left(\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{1}} }\right)^{\mathrm{2}} +\left(\frac{\mathrm{2}}{\mathrm{3}^{\mathrm{2}} }\right)^{\mathrm{2}} +\left(\frac{\mathrm{3}}{\mathrm{3}^{\mathrm{3}} }\right)^{\mathrm{2}} +...\infty=\frac{\mathrm{45}}{\mathrm{512}}\neq\frac{\mathrm{9}}{\mathrm{32}}\right. \\ $$
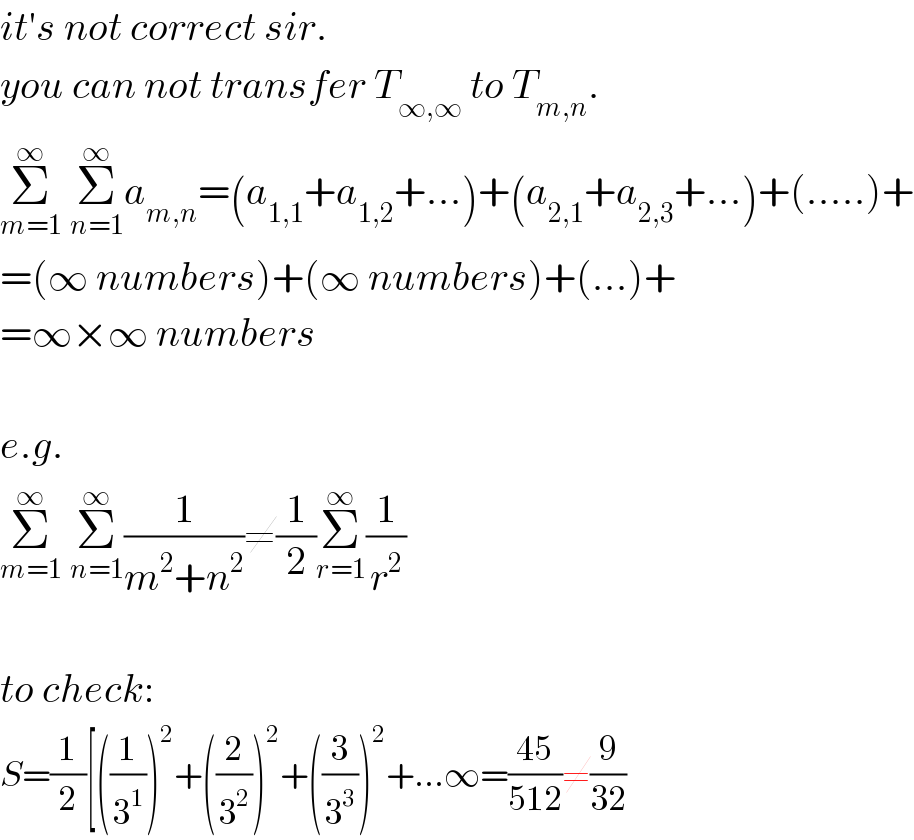
Commented by tanmay.chaudhury50@gmail.com last updated on 05/Jan/19
$${thank}\:{you}\:{sir}...{i}\:{viewed}\:{it}\:{diffdrently}... \\ $$
Answered by rahul 19 last updated on 05/Jan/19
![S = Σ_(m=1) ^∞ Σ_(n=1) ^∞ ((m^2 n)/(3^m (n.3^m +m.3^n ))) S = Σ_(n=1) ^∞ Σ_(m=1) ^∞ ((n^2 m)/(3^n (m.3^n +n.3^m ))) ⇒ 2S = ΣΣ((mn)/(n.3^m +m.3^n ))[(m/3^m )+(n/3^n )]. ⇒2S= Σ_(m=1) ^∞ (m/3^m ) . Σ_(n=1) ^∞ (n/3^n ) ⇒2S= (3/4) × (3/4) ⇒ S = (9/(32 )) .](Q52265.png)
$${S}\:=\:\underset{{m}=\mathrm{1}} {\overset{\infty} {\sum}}\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\:\frac{{m}^{\mathrm{2}} {n}}{\mathrm{3}^{{m}} \left({n}.\mathrm{3}^{{m}} +{m}.\mathrm{3}^{{n}} \right)} \\ $$$${S}\:=\:\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\underset{{m}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{n}^{\mathrm{2}} {m}}{\mathrm{3}^{{n}} \left({m}.\mathrm{3}^{{n}} +{n}.\mathrm{3}^{{m}} \right)} \\ $$$$\Rightarrow\:\mathrm{2}{S}\:=\:\Sigma\Sigma\frac{{mn}}{{n}.\mathrm{3}^{{m}} +{m}.\mathrm{3}^{{n}} }\left[\frac{{m}}{\mathrm{3}^{{m}} }+\frac{{n}}{\mathrm{3}^{{n}} }\right]. \\ $$$$\Rightarrow\mathrm{2}{S}=\:\underset{{m}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{m}}{\mathrm{3}^{{m}} }\:\:.\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{n}}{\mathrm{3}^{{n}} } \\ $$$$\Rightarrow\mathrm{2}{S}=\:\frac{\mathrm{3}}{\mathrm{4}}\:×\:\frac{\mathrm{3}}{\mathrm{4}} \\ $$$$\Rightarrow\:{S}\:=\:\frac{\mathrm{9}}{\mathrm{32}\:}\:. \\ $$
Commented by mr W last updated on 05/Jan/19
$${very}\:{tricky}\:{sir}! \\ $$