
Question Number 52356 by Tawa1 last updated on 06/Jan/19
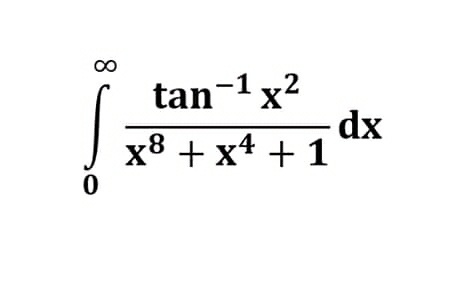
Commented by tanmay.chaudhury50@gmail.com last updated on 06/Jan/19
Commented by Tawa1 last updated on 07/Jan/19

$$\mathrm{Sir}\:\mathrm{please}\:\mathrm{help}. \\ $$
Commented by MJS last updated on 07/Jan/19
![this can′t be solved using elementary calculus not sure if these help: tan^(−1) θ =(i/2)(ln (1−iθ) −ln (1+iθ)) ⇒ ⇒ tan^(−1) x^2 =(i/2)(ln (1−ix^2 ) −ln (1+ix^2 )) ⇒ ⇒ tan^(−1) x^2 =(i/2)ln ((1−ix^2 )/(1+ix^2 )) =(i/2)ln ((1−x^4 +2ix^2 )/(x^4 +1)) = [ln (a+bi) =(1/2)ln (a^2 +b^2 ) +i(sign(b)(π/2)−tan^(−1) (a/b))] =(π/4)+(1/2)tan^(−1) ((x^4 −1)/(2x^2 )) x^8 +x^4 +1=(x^4 +x^2 +1)(x^4 −x^2 +1)= =(x^2 +x+1)(x^2 −x+1)(x^2 +(√3)x+1)(x^2 −(√3)x+1 =(x+((1+i(√3))/2))(x−((1+i(√3))/2))(x+((1−i(√3))/2))(x−((1−i(√3))/2))(x+(((√3)+i)/2))(x−(((√3)+i)/2))(x+(((√3)−i)/2))(x−(((√3)−i)/2))](Q52400.png)
$$\mathrm{this}\:\mathrm{can}'\mathrm{t}\:\mathrm{be}\:\mathrm{solved}\:\mathrm{using}\:\mathrm{elementary}\:\mathrm{calculus} \\ $$$$\mathrm{not}\:\mathrm{sure}\:\mathrm{if}\:\mathrm{these}\:\mathrm{help}: \\ $$$$\mathrm{tan}^{−\mathrm{1}} \:\theta\:=\frac{\mathrm{i}}{\mathrm{2}}\left(\mathrm{ln}\:\left(\mathrm{1}−\mathrm{i}\theta\right)\:−\mathrm{ln}\:\left(\mathrm{1}+\mathrm{i}\theta\right)\right)\:\Rightarrow \\ $$$$\Rightarrow\:\mathrm{tan}^{−\mathrm{1}} \:{x}^{\mathrm{2}} \:=\frac{\mathrm{i}}{\mathrm{2}}\left(\mathrm{ln}\:\left(\mathrm{1}−\mathrm{i}{x}^{\mathrm{2}} \right)\:−\mathrm{ln}\:\left(\mathrm{1}+\mathrm{i}{x}^{\mathrm{2}} \right)\right)\:\Rightarrow \\ $$$$\Rightarrow\:\mathrm{tan}^{−\mathrm{1}} \:{x}^{\mathrm{2}} =\frac{\mathrm{i}}{\mathrm{2}}\mathrm{ln}\:\frac{\mathrm{1}−\mathrm{i}{x}^{\mathrm{2}} }{\mathrm{1}+\mathrm{i}{x}^{\mathrm{2}} }\:=\frac{\mathrm{i}}{\mathrm{2}}\mathrm{ln}\:\frac{\mathrm{1}−{x}^{\mathrm{4}} +\mathrm{2i}{x}^{\mathrm{2}} }{{x}^{\mathrm{4}} +\mathrm{1}}\:= \\ $$$$\:\:\:\:\:\left[\mathrm{ln}\:\left({a}+{b}\mathrm{i}\right)\:=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\:\left({a}^{\mathrm{2}} +{b}^{\mathrm{2}} \right)\:+\mathrm{i}\left(\mathrm{sign}\left({b}\right)\frac{\pi}{\mathrm{2}}−\mathrm{tan}^{−\mathrm{1}} \:\frac{{a}}{{b}}\right)\right] \\ $$$$=\frac{\pi}{\mathrm{4}}+\frac{\mathrm{1}}{\mathrm{2}}\mathrm{tan}^{−\mathrm{1}} \:\frac{{x}^{\mathrm{4}} −\mathrm{1}}{\mathrm{2}{x}^{\mathrm{2}} } \\ $$$${x}^{\mathrm{8}} +{x}^{\mathrm{4}} +\mathrm{1}=\left({x}^{\mathrm{4}} +{x}^{\mathrm{2}} +\mathrm{1}\right)\left({x}^{\mathrm{4}} −{x}^{\mathrm{2}} +\mathrm{1}\right)= \\ $$$$=\left({x}^{\mathrm{2}} +{x}+\mathrm{1}\right)\left({x}^{\mathrm{2}} −{x}+\mathrm{1}\right)\left({x}^{\mathrm{2}} +\sqrt{\mathrm{3}}{x}+\mathrm{1}\right)\left({x}^{\mathrm{2}} −\sqrt{\mathrm{3}}{x}+\mathrm{1}\right. \\ $$$$=\left({x}+\frac{\mathrm{1}+\mathrm{i}\sqrt{\mathrm{3}}}{\mathrm{2}}\right)\left({x}−\frac{\mathrm{1}+\mathrm{i}\sqrt{\mathrm{3}}}{\mathrm{2}}\right)\left({x}+\frac{\mathrm{1}−\mathrm{i}\sqrt{\mathrm{3}}}{\mathrm{2}}\right)\left({x}−\frac{\mathrm{1}−\mathrm{i}\sqrt{\mathrm{3}}}{\mathrm{2}}\right)\left({x}+\frac{\sqrt{\mathrm{3}}+\mathrm{i}}{\mathrm{2}}\right)\left({x}−\frac{\sqrt{\mathrm{3}}+\mathrm{i}}{\mathrm{2}}\right)\left({x}+\frac{\sqrt{\mathrm{3}}−\mathrm{i}}{\mathrm{2}}\right)\left({x}−\frac{\sqrt{\mathrm{3}}−\mathrm{i}}{\mathrm{2}}\right) \\ $$
Commented by Tawa1 last updated on 07/Jan/19

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$
Commented by Abdo msup. last updated on 07/Jan/19
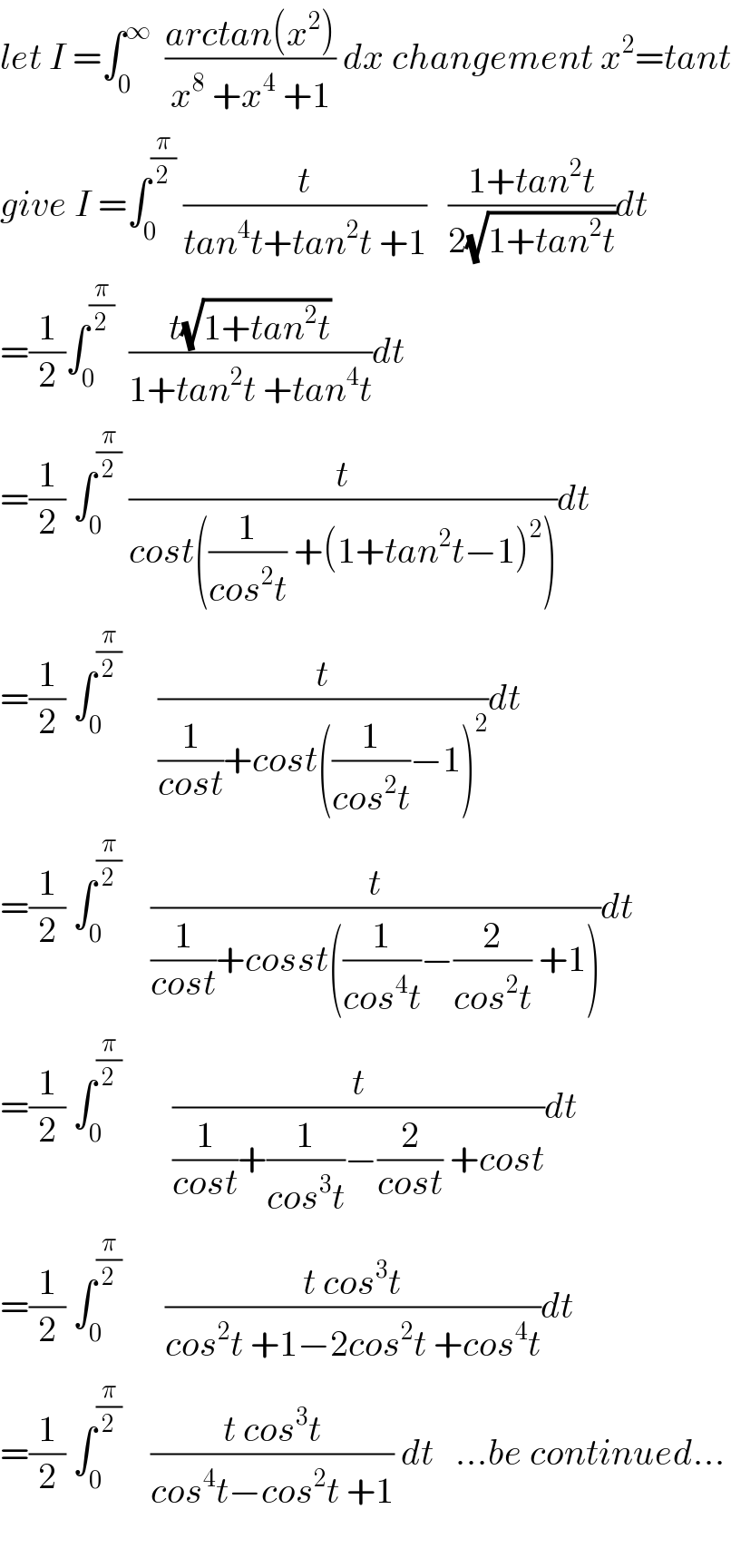
$${let}\:{I}\:=\int_{\mathrm{0}} ^{\infty} \:\:\frac{{arctan}\left({x}^{\mathrm{2}} \right)}{{x}^{\mathrm{8}} \:+{x}^{\mathrm{4}} \:+\mathrm{1}}\:{dx}\:{changement}\:{x}^{\mathrm{2}} ={tant} \\ $$$${give}\:{I}\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\frac{{t}}{{tan}^{\mathrm{4}} {t}+{tan}^{\mathrm{2}} {t}\:+\mathrm{1}}\:\:\:\frac{\mathrm{1}+{tan}^{\mathrm{2}} {t}}{\mathrm{2}\sqrt{\mathrm{1}+{tan}^{\mathrm{2}} {t}}}{dt} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:\frac{{t}\sqrt{\mathrm{1}+{tan}^{\mathrm{2}} {t}}}{\mathrm{1}+{tan}^{\mathrm{2}} {t}\:+{tan}^{\mathrm{4}} {t}}{dt} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\frac{{t}}{{cost}\left(\frac{\mathrm{1}}{{cos}^{\mathrm{2}} {t}}\:+\left(\mathrm{1}+{tan}^{\mathrm{2}} {t}−\mathrm{1}\right)^{\mathrm{2}} \right)}{dt} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\:\:\frac{{t}}{\frac{\mathrm{1}}{{cost}}+{cost}\left(\frac{\mathrm{1}}{{cos}^{\mathrm{2}} {t}}−\mathrm{1}\right)^{\mathrm{2}} }{dt} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\:\frac{{t}}{\frac{\mathrm{1}}{{cost}}+{cosst}\left(\frac{\mathrm{1}}{{cos}^{\mathrm{4}} {t}}−\frac{\mathrm{2}}{{cos}^{\mathrm{2}} {t}}\:+\mathrm{1}\right)}{dt} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\:\:\:\:\frac{{t}}{\frac{\mathrm{1}}{{cost}}+\frac{\mathrm{1}}{{cos}^{\mathrm{3}} {t}}−\frac{\mathrm{2}}{{cost}}\:+{cost}}{dt} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\:\:\:\frac{{t}\:{cos}^{\mathrm{3}} {t}}{{cos}^{\mathrm{2}} {t}\:+\mathrm{1}−\mathrm{2}{cos}^{\mathrm{2}} {t}\:+{cos}^{\mathrm{4}} {t}}{dt} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\:\frac{{t}\:{cos}^{\mathrm{3}} {t}}{{cos}^{\mathrm{4}} {t}−{cos}^{\mathrm{2}} {t}\:+\mathrm{1}}\:{dt}\:\:\:...{be}\:{continued}... \\ $$$$ \\ $$
Commented by Abdo msup. last updated on 07/Jan/19
![let F(α)=∫_0 ^∞ ((arctan(αx^2 ))/(1+x^4 +x^8 )) dx with α>0 we have F^′ (α) =∫_0 ^∞ (x^2 /((1+α^2 x^4 )(x^8 +x^4 +1)))dx ⇒2F^′ (α) =∫_(−∞) ^(+∞) (x^2 /((x^8 +x^4 +1)(α^2 x^4 +1)))dx let consider the complex function ϕ(z)=(z^2 /((z^8 +z^4 +1)(α^2 z^4 +1))) poles of ϕ? z^8 +z^4 +1=0 ⇔1+z^4 +(z^4 )^2 =0 ⇔((1−z^(12) )/(1−z^4 )) =0 ⇔ z^(12) =1 and z^4 ≠ 1 the roots of z^(12) =1 are z_k =e^(i((kπ)/6)) and k∈[[0,11]] but only we must take 8 roots let search.. z_0 =1 (to eliminate) z_1 =e^((iπ)/6) and z_1 ^4 ≠1 z_2 =e^((iπ)/3) and z_2 ^4 ≠1 z_3 =e^((iπ)/2) and z_3 ^4 =1 (to eliminate) z_4 =e^(i((2π)/3)) and z_4 ^4 ≠1 z_5 =e^((i5π)/6) and z_5 ^4 ≠1 z_6 =e^(iπ) and z_6 ^4 =1 (to eliminate) z_7 =e^((i7.π)/6) and z_7 ^4 ≠1 z_8 = e^((i4π)/3) and z_8 ^4 ≠1 z_9 = e^((i3π)/2) and z_9 ^4 =1 (to eliminate) z_(10) =e^((i5π)/3) and z_(10) ^4 ≠1 z_(11) =e^((i11π)/6) ⇒ z^8 +z^4 +1 =(z−z_1 )(z−z_2 )(z−z_4 )(z−z_5 )(z−z_7 )(z−z_8 )(z−z_(10) )(z−z_(11) ) also we have α^2 z^4 +1 =α^2 (z^4 +(1/α^2 )) =α^2 (z^4 −((i/α))^2 )=α^2 (z^2 −(i/α))(z^2 +(i/α)) =α^2 (z−(1/(√α)) e^((iπ)/4) )(z+(1/(√α)) e^((iπ)/4) )(z−(1/(√α)) e^(−((iπ)/4)) )(z+(1/(√α))e^(−((iπ)/4)) ) so the poles of ϕ are +^− (1/(√α)) e^((iπ)/4) and +^− (1/(√α)) e^(−((iπ)/4)) also tbe complex z_k with k ∈{1,2,4,5,7,8,10,11} after we use Residus theorem ∫_(−∞) ^(+∞) ϕ(z)dz =2iπ Σ_(im(z_k )>0) Res(ϕ ,z_k ) ...be continued ...](Q52417.png)
$${let}\:{F}\left(\alpha\right)=\int_{\mathrm{0}} ^{\infty} \:\:\:\:\frac{{arctan}\left(\alpha{x}^{\mathrm{2}} \right)}{\mathrm{1}+{x}^{\mathrm{4}} \:+{x}^{\mathrm{8}} }\:{dx}\:\:{with}\:\alpha>\mathrm{0}\:{we} \\ $$$${have}\:{F}^{'} \left(\alpha\right)\:=\int_{\mathrm{0}} ^{\infty} \:\:\:\:\frac{{x}^{\mathrm{2}} }{\left(\mathrm{1}+\alpha^{\mathrm{2}} {x}^{\mathrm{4}} \right)\left({x}^{\mathrm{8}} \:+{x}^{\mathrm{4}} \:+\mathrm{1}\right)}{dx} \\ $$$$\Rightarrow\mathrm{2}{F}^{'} \left(\alpha\right)\:=\int_{−\infty} ^{+\infty} \:\:\:\frac{{x}^{\mathrm{2}} }{\left({x}^{\mathrm{8}} \:+{x}^{\mathrm{4}} \:+\mathrm{1}\right)\left(\alpha^{\mathrm{2}} {x}^{\mathrm{4}} \:+\mathrm{1}\right)}{dx} \\ $$$${let}\:{consider}\:{the}\:{complex}\:{function} \\ $$$$\varphi\left({z}\right)=\frac{{z}^{\mathrm{2}} }{\left({z}^{\mathrm{8}} \:+{z}^{\mathrm{4}} \:+\mathrm{1}\right)\left(\alpha^{\mathrm{2}} {z}^{\mathrm{4}} \:+\mathrm{1}\right)}\:{poles}\:{of}\:\varphi? \\ $$$${z}^{\mathrm{8}} \:+{z}^{\mathrm{4}} \:+\mathrm{1}=\mathrm{0}\:\Leftrightarrow\mathrm{1}+{z}^{\mathrm{4}} \:+\left({z}^{\mathrm{4}} \right)^{\mathrm{2}} =\mathrm{0}\:\Leftrightarrow\frac{\mathrm{1}−{z}^{\mathrm{12}} }{\mathrm{1}−{z}^{\mathrm{4}} }\:=\mathrm{0}\:\Leftrightarrow \\ $$$${z}^{\mathrm{12}} =\mathrm{1}\:{and}\:{z}^{\mathrm{4}} \neq\:\mathrm{1}\:{the}\:{roots}\:{of}\:{z}^{\mathrm{12}} =\mathrm{1}\:{are} \\ $$$${z}_{{k}} ={e}^{{i}\frac{{k}\pi}{\mathrm{6}}} \:\:\:{and}\:{k}\in\left[\left[\mathrm{0},\mathrm{11}\right]\right]\:{but}\:{only}\:{we}\:{must}\:{take}\: \\ $$$$\mathrm{8}\:{roots}\:{let}\:{search}.. \\ $$$${z}_{\mathrm{0}} =\mathrm{1}\:\left({to}\:{eliminate}\right) \\ $$$${z}_{\mathrm{1}} ={e}^{\frac{{i}\pi}{\mathrm{6}}} \:\:{and}\:{z}_{\mathrm{1}} ^{\mathrm{4}} \neq\mathrm{1} \\ $$$${z}_{\mathrm{2}} ={e}^{\frac{{i}\pi}{\mathrm{3}}} \:\:{and}\:{z}_{\mathrm{2}} ^{\mathrm{4}} \:\neq\mathrm{1} \\ $$$${z}_{\mathrm{3}} ={e}^{\frac{{i}\pi}{\mathrm{2}}} \:\:{and}\:{z}_{\mathrm{3}} ^{\mathrm{4}} \:=\mathrm{1}\:\left({to}\:{eliminate}\right) \\ $$$${z}_{\mathrm{4}} ={e}^{{i}\frac{\mathrm{2}\pi}{\mathrm{3}}} \:\:{and}\:{z}_{\mathrm{4}} ^{\mathrm{4}} \:\neq\mathrm{1} \\ $$$${z}_{\mathrm{5}} \:={e}^{\frac{{i}\mathrm{5}\pi}{\mathrm{6}}} \:\:{and}\:{z}_{\mathrm{5}} ^{\mathrm{4}} \:\neq\mathrm{1} \\ $$$${z}_{\mathrm{6}} ={e}^{{i}\pi} \:{and}\:{z}_{\mathrm{6}} ^{\mathrm{4}} =\mathrm{1}\:\left({to}\:{eliminate}\right) \\ $$$${z}_{\mathrm{7}} ={e}^{\frac{{i}\mathrm{7}.\pi}{\mathrm{6}}} \:\:{and}\:{z}_{\mathrm{7}} ^{\mathrm{4}} \:\neq\mathrm{1} \\ $$$${z}_{\mathrm{8}} =\:{e}^{\frac{{i}\mathrm{4}\pi}{\mathrm{3}}} \:\:{and}\:{z}_{\mathrm{8}} ^{\mathrm{4}} \:\neq\mathrm{1} \\ $$$${z}_{\mathrm{9}} =\:{e}^{\frac{{i}\mathrm{3}\pi}{\mathrm{2}}} \:\:{and}\:{z}_{\mathrm{9}} ^{\mathrm{4}} =\mathrm{1}\:\left({to}\:{eliminate}\right) \\ $$$${z}_{\mathrm{10}} ={e}^{\frac{{i}\mathrm{5}\pi}{\mathrm{3}}} \:\:{and}\:{z}_{\mathrm{10}} ^{\mathrm{4}} \:\neq\mathrm{1} \\ $$$${z}_{\mathrm{11}} ={e}^{\frac{{i}\mathrm{11}\pi}{\mathrm{6}}} \:\:\Rightarrow \\ $$$${z}^{\mathrm{8}} \:+{z}^{\mathrm{4}} \:+\mathrm{1}\:=\left({z}−{z}_{\mathrm{1}} \right)\left({z}−{z}_{\mathrm{2}} \right)\left({z}−{z}_{\mathrm{4}} \right)\left({z}−{z}_{\mathrm{5}} \right)\left({z}−{z}_{\mathrm{7}} \right)\left({z}−{z}_{\mathrm{8}} \right)\left({z}−{z}_{\mathrm{10}} \right)\left({z}−{z}_{\mathrm{11}} \right) \\ $$$${also}\:{we}\:{have}\:\alpha^{\mathrm{2}} {z}^{\mathrm{4}} +\mathrm{1}\:=\alpha^{\mathrm{2}} \left({z}^{\mathrm{4}} +\frac{\mathrm{1}}{\alpha^{\mathrm{2}} }\right) \\ $$$$=\alpha^{\mathrm{2}} \left({z}^{\mathrm{4}} −\left(\frac{{i}}{\alpha}\right)^{\mathrm{2}} \right)=\alpha^{\mathrm{2}} \left({z}^{\mathrm{2}} −\frac{{i}}{\alpha}\right)\left({z}^{\mathrm{2}} +\frac{{i}}{\alpha}\right) \\ $$$$=\alpha^{\mathrm{2}} \left({z}−\frac{\mathrm{1}}{\sqrt{\alpha}}\:{e}^{\frac{{i}\pi}{\mathrm{4}}} \right)\left({z}+\frac{\mathrm{1}}{\sqrt{\alpha}}\:{e}^{\frac{{i}\pi}{\mathrm{4}}} \right)\left({z}−\frac{\mathrm{1}}{\sqrt{\alpha}}\:{e}^{−\frac{{i}\pi}{\mathrm{4}}} \right)\left({z}+\frac{\mathrm{1}}{\sqrt{\alpha}}{e}^{−\frac{{i}\pi}{\mathrm{4}}} \right) \\ $$$${so}\:{the}\:{poles}\:{of}\:\varphi\:{are}\: \\ $$$$\overset{−} {+}\frac{\mathrm{1}}{\sqrt{\alpha}}\:{e}^{\frac{{i}\pi}{\mathrm{4}}} \:\:{and}\:\:\overset{−} {+}\:\frac{\mathrm{1}}{\sqrt{\alpha}}\:{e}^{−\frac{{i}\pi}{\mathrm{4}}} \:\:{also}\:{tbe}\:{complex} \\ $$$${z}_{{k}} \:{with}\:{k}\:\in\left\{\mathrm{1},\mathrm{2},\mathrm{4},\mathrm{5},\mathrm{7},\mathrm{8},\mathrm{10},\mathrm{11}\right\}\:{after}\:{we}\:{use} \\ $$$${Residus}\:{theorem} \\ $$$$\int_{−\infty} ^{+\infty} \:\varphi\left({z}\right){dz}\:=\mathrm{2}{i}\pi\:\sum_{{im}\left({z}_{{k}} \right)>\mathrm{0}} {Res}\left(\varphi\:,{z}_{{k}} \right) \\ $$$$...{be}\:{continued}\:... \\ $$$$ \\ $$
Commented by Tawa1 last updated on 07/Jan/19
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}.\:\:\mathrm{waiting}\:... \\ $$
Commented by Abdo msup. last updated on 07/Jan/19
$${thanks}\:{sir}\:{this}\:{is}\:{the}\:{way}\:{for}\:{this}\:{integral}.... \\ $$
Answered by MJS last updated on 07/Jan/19

$$\approx.\mathrm{269949} \\ $$
