Math Question and Answers


Question and Answers Forum

All Questions Topic List
Mechanics Questions
Previous in All Question Next in All Question
Previous in Mechanics Next in Mechanics
Question Number 52825 by ajfour last updated on 13/Jan/19

Commented by ajfour last updated on 13/Jan/19

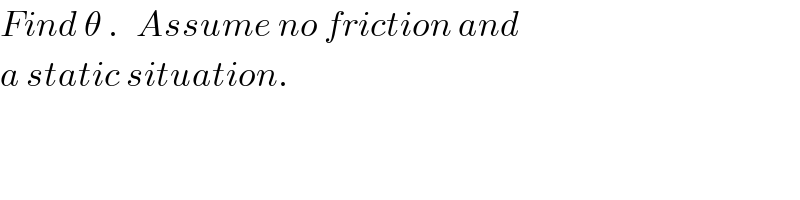
$F i n d θ . A s s u m e n o f r i c t i o n a n d$ $a s t a t i c s i t u a t i o n .$
Commented by tanmay.chaudhury50@gmail.com last updated on 13/Jan/19

Commented by ajfour last updated on 14/Jan/19

Commented by mr W last updated on 14/Jan/19

$p e r f e c t l y i l l u s t r a t e d! t h a n k s a l o t s i r!$
	Answered by mr W last updated on 14/Jan/19
	$let η=(m/M), λ=(L/h) α=angle between string and vertical a=distance from ring to lower end of rod N=contact force between ring and rod ((MgL cos θ)/2)=Na ((Mg)/(sin (π−θ)))=((mg)/(sin (θ−α)))=(N/(sin α)) sin (θ−α)=(m/M) sin θ=η sin θ ⇒θ−α=sin^(−1) (η sin θ) ⇒α=θ−sin^(−1) (η sin θ) N=((Mg sin α)/(sin θ)) a=((h sin α)/(sin ((π/2)+θ−α)))=((h sin α)/(cos (θ−α)))=((h sin α)/(√(1−η^2 sin^2 θ))) ⇒((MgL cos θ)/2)=((Mg sin α)/(sin θ))×((h sin α)/(√(1−η^2 sin^2 θ))) ⇒((cos θ)/2)×(L/h)=((sin^2 α)/(sin θ (√(1−η^2 sin^2 θ)))) ⇒((λ sin θ cos θ (√(1−η^2 sin^2 θ)))/2)=sin^2 α ⇒λ sin 2θ (√(1−η^2 sin^2 θ))=2(1−cos 2α) ⇒λ sin 2θ (√(1−η^2 sin^2 θ))=2[1−cos 2{θ−sin^(−1) (η sin θ)}] examples: λ=(L/h)=2, η=(m/M)=0.2⇒θ=52.7639° λ=(L/h)=2, η=(m/M)=0⇒θ=45°$
	$l e t η = \frac{m}{M}, λ = \frac{L}{h}$ $α = a n g l e b e t w e e n s t r i n g a n d v e r t i c a l$ $a = d i s t a n c e f r o m r i n g t o l o w e r e n d o f r o d$ $N = c o n t a c t f o r c e b e t w e e n r i n g a n d r o d$ $\frac{M g L \cos θ}{2} = N a$ $\frac{M g}{\sin (π - θ)} = \frac{m g}{\sin (θ - α)} = \frac{N}{\sin α}$ $\sin (θ - α) = \frac{m}{M} \sin θ = η \sin θ$ $\Rightarrow θ - α = \sin^{- 1} (η \sin θ)$ $\Rightarrow α = θ - \sin^{- 1} (η \sin θ)$ $N = \frac{M g \sin α}{\sin θ}$ $a = \frac{h \sin α}{\sin (\frac{π}{2} + θ - α)} = \frac{h \sin α}{\cos (θ - α)} = \frac{h \sin α}{\sqrt{1 - η^{2} \sin^{2} θ}}$ $\Rightarrow \frac{M g L \cos θ}{2} = \frac{M g \sin α}{\sin θ} \times \frac{h \sin α}{\sqrt{1 - η^{2} \sin^{2} θ}}$ $\Rightarrow \frac{\cos θ}{2} \times \frac{L}{h} = \frac{\sin^{2} α}{\sin θ \sqrt{1 - η^{2} \sin^{2} θ}}$ $\Rightarrow \frac{λ \sin θ \cos θ \sqrt{1 - η^{2} \sin^{2} θ}}{2} = \sin^{2} α$ $\Rightarrow λ \sin 2 θ \sqrt{1 - η^{2} \sin^{2} θ} = 2 (1 - \cos 2 α)$ $\Rightarrow λ \sin 2 θ \sqrt{1 - η^{2} \sin^{2} θ} = 2 [1 - \cos 2 {θ - \sin^{- 1} (η \sin θ)}]$ $e x a m p l e s :$ $λ = \frac{L}{h} = 2, η = \frac{m}{M} = 0.2 \Rightarrow θ = 52.7639 °$ $λ = \frac{L}{h} = 2, η = \frac{m}{M} = 0 \Rightarrow θ = 45 °$
	Commented by mr W last updated on 13/Jan/19

	Commented by ajfour last updated on 14/Jan/19

	$A l r i g h t a l l - a l o n g S i r . N o t s o e a s y .$ $G o d b l e s s y o u!$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum