
Question and Answers Forum
Question Number 52945 by Tawa1 last updated on 15/Jan/19

Commented by maxmathsup by imad last updated on 15/Jan/19
![3) let A =∫_0 ^∞ ((x ln^2 (x))/(e^x −1)) dx ⇒A =∫_0 ^∞ ((x e^(−x) ln^2 (x))/(1−e^(−x) )) dx =∫_0 ^∞ x e^(−x) ln^2 (x)(Σ_(n=0) ^∞ e^(−nx) )dx =Σ_(n=0) ^∞ ∫_0 ^∞ x e^(−(n+1)x) ln^2 x dx =Σ_(n=0) ^∞ A_n with A_n =∫_0 ^∞ x e^(−(n+1)x) ln^2 (x)dx A_n =_((n+1)x=t) ∫_0 ^∞ (t/(n+1)) e^(−t) ln^2 ((t/(n+1)))(dt/(n+1)) =(1/((n+1)^2 ))∫_0 ^∞ t e^(−t) (ln(t)−ln(n+1))^2 dt ⇒(n+1)^2 A_n =∫_0 ^∞ t e^(−t) (ln^2 (t)−2ln(n+1)ln(t) +ln^2 (n+1))dt =∫_0 ^∞ t e^(−t) ln^2 (t)dt−2ln(n+1)∫_0 ^∞ t e^(−t) ln(t)dt +ln^2 (n+1)∫_0 ^∞ t e^(−t) dt but ∫_0 ^∞ t e^(−t) dt =[−t e^(−t) ]_0 ^(+∞) +∫_0 ^∞ e^(−t) dt =[−e^(−t) ]_0 ^(+∞) =1 by part u^′ =t e^(−t) and v=ln(t) ⇒u =∫ t e^(−t) dt =−t e^(−t) +∫ e^(−t) dt =−t e^(−t) −e^(−t) =−(t+1)e^(−t) +1 ⇒∫_0 ^∞ t e^(−t) ln(t)dt =[(−(t+1)e^(−t) +1)ln(t)]_0 ^(+∞) −∫_0 ^∞ t e^(−t) (dt/t) =−∫_0 ^∞ e^(−t) dt =[e^(−t) ]_0 ^(+∞) =−1 let find ∫_0 ^∞ t e^(−t) ln^2 (t)dt by parts u^′ =t e^(−t) and v=ln^2 (t) ⇒ u =∫ t e^(−t) dt =1−(t+1)e^(−t) ⇒∫_0 ^∞ t e^(−t) ln^2 (t)dt =[(1−(t+1)e^(−t) ln^2 t]_0 ^(+∞) −∫_0 ^∞ t e^(−t) ((2ln(t))/t)dt =−2 ∫_0 ^∞ e^(−t) ln(t)dt =−2(−γ)=2γ ( this result is proved )⇒ (n+1)^2 A_n =2γ +2ln(n+1) +ln^2 (n+1) ⇒ A_n =((2γ)/((n+1)^2 )) + 2((ln(n+1))/((n+1)^2 )) +((ln^2 (n+1))/((n+1)^2 )) ⇒ A =2γ Σ_(n=0) ^∞ (1/((n+1)^2 )) +2Σ_(n=0) ^∞ ((ln(n+1))/((n+1)^2 )) +Σ_(n=0) ^∞ ((ln^2 (n+1))/((n+1)^2 )) Σ_(n=0) ^∞ (1/((n+1)^2 )) =ξ(2)=(π^2 /6) ....becontinued...](Q52981.png)
Commented by Tawa1 last updated on 15/Jan/19
Answered by tanmay.chaudhury50@gmail.com last updated on 15/Jan/19
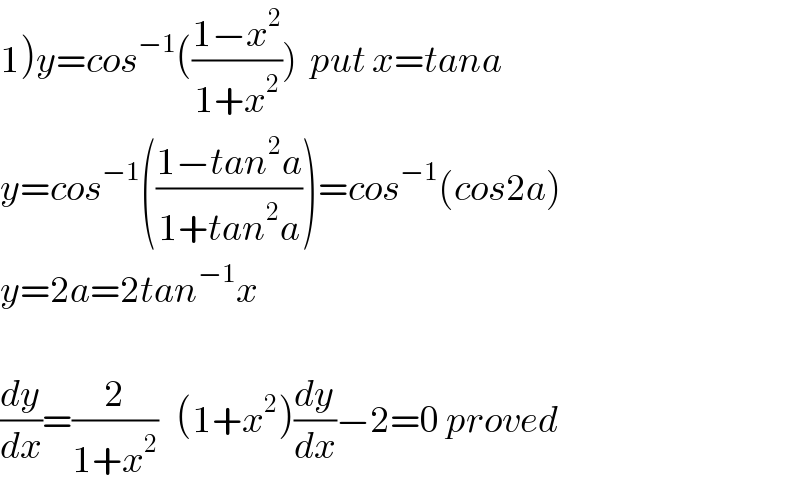
Commented by Tawa1 last updated on 15/Jan/19

Commented by Tawa1 last updated on 15/Jan/19

Answered by tanmay.chaudhury50@gmail.com last updated on 15/Jan/19
![2)cos^(−1) x=a cosa=x sin[cot^(−1) {tana)}] =sin[cot^(−1) {cot((π/2)−a)}] =sin((π/2)−a) =cosa=x proved](Q52962.png)
Commented by Tawa1 last updated on 15/Jan/19

Answered by tanmay.chaudhury50@gmail.com last updated on 15/Jan/19
Commented by Tawa1 last updated on 15/Jan/19

