Question and Answers Forum

All Questions      Topic List

UNKNOWN Questions

Previous in All Question      Next in All Question      

Previous in UNKNOWN      Next in UNKNOWN      

Question Number 53384 by gunawan last updated on 21/Jan/19

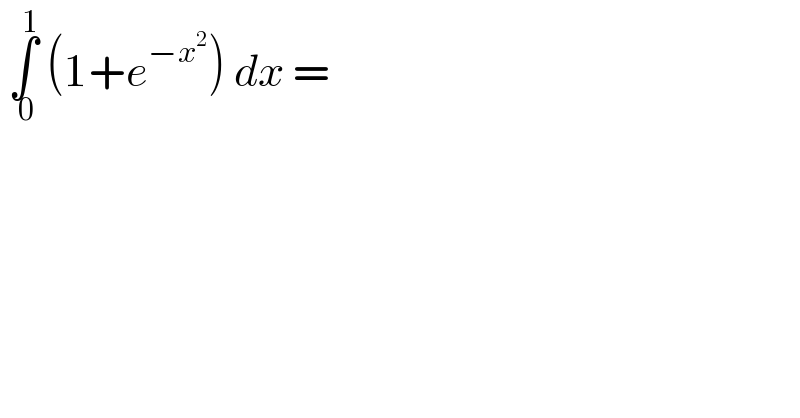
∫_( 0) ^1 (1+e^(−x^2 ) ) dx =

$$\:\underset{\:\mathrm{0}} {\overset{\mathrm{1}} {\int}}\:\left(\mathrm{1}+{e}^{−{x}^{\mathrm{2}} } \right)\:{dx}\:= \\ $$

Commented by Abdo msup. last updated on 21/Jan/19

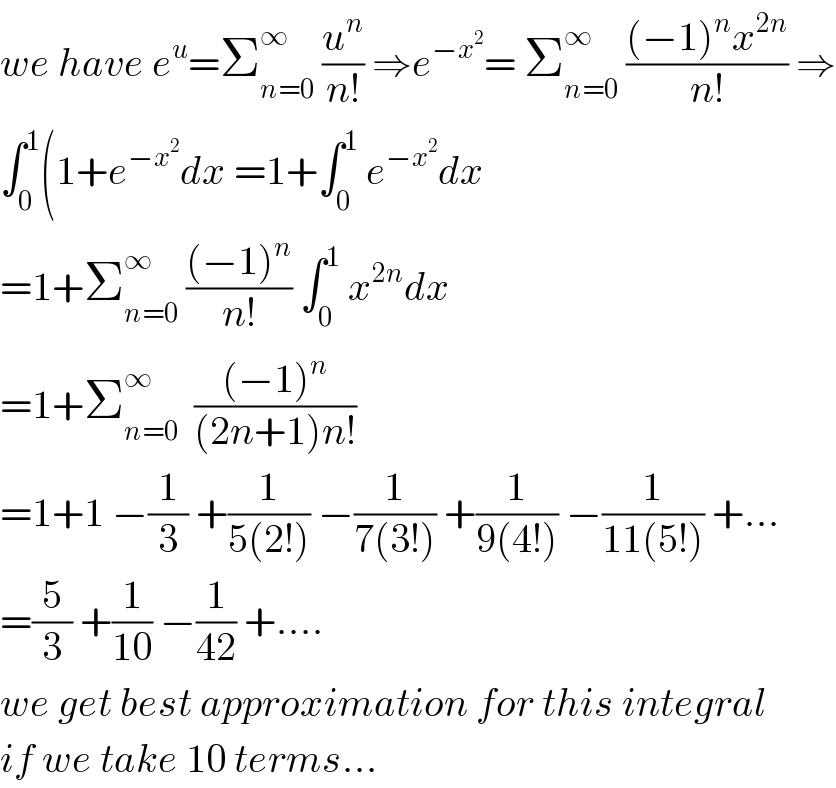
we have e^u =Σ_(n=0) ^∞ (u^n /(n!)) ⇒e^(−x^2 ) = Σ_(n=0) ^∞ (((−1)^n x^(2n) )/(n!)) ⇒ ∫_0 ^1 (1+e^(−x^2 ) dx =1+∫_0 ^1 e^(−x^2 ) dx =1+Σ_(n=0) ^∞ (((−1)^n )/(n!)) ∫_0 ^1 x^(2n) dx =1+Σ_(n=0) ^∞ (((−1)^n )/((2n+1)n!)) =1+1 −(1/3) +(1/(5(2!))) −(1/(7(3!))) +(1/(9(4!))) −(1/(11(5!))) +... =(5/3) +(1/(10)) −(1/(42)) +.... we get best approximation for this integral if we take 10 terms...

$${we}\:{have}\:{e}^{{u}} =\sum_{{n}=\mathrm{0}} ^{\infty} \:\frac{{u}^{{n}} }{{n}!}\:\Rightarrow{e}^{−{x}^{\mathrm{2}} } =\:\sum_{{n}=\mathrm{0}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{{n}} {x}^{\mathrm{2}{n}} }{{n}!}\:\Rightarrow \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \left(\mathrm{1}+{e}^{−{x}^{\mathrm{2}} } {dx}\:=\mathrm{1}+\int_{\mathrm{0}} ^{\mathrm{1}} \:{e}^{−{x}^{\mathrm{2}} } {dx}\right. \\ $$$$=\mathrm{1}+\sum_{{n}=\mathrm{0}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{{n}} }{{n}!}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:{x}^{\mathrm{2}{n}} {dx} \\ $$$$=\mathrm{1}+\sum_{{n}=\mathrm{0}} ^{\infty} \:\:\frac{\left(−\mathrm{1}\right)^{{n}} }{\left(\mathrm{2}{n}+\mathrm{1}\right){n}!} \\ $$$$=\mathrm{1}+\mathrm{1}\:−\frac{\mathrm{1}}{\mathrm{3}}\:+\frac{\mathrm{1}}{\mathrm{5}\left(\mathrm{2}!\right)}\:−\frac{\mathrm{1}}{\mathrm{7}\left(\mathrm{3}!\right)}\:+\frac{\mathrm{1}}{\mathrm{9}\left(\mathrm{4}!\right)}\:−\frac{\mathrm{1}}{\mathrm{11}\left(\mathrm{5}!\right)}\:+... \\ $$$$=\frac{\mathrm{5}}{\mathrm{3}}\:+\frac{\mathrm{1}}{\mathrm{10}}\:−\frac{\mathrm{1}}{\mathrm{42}}\:+.... \\ $$$${we}\:{get}\:{best}\:{approximation}\:{for}\:{this}\:{integral} \\ $$$${if}\:{we}\:{take}\:\mathrm{10}\:{terms}... \\ $$

Answered by tanmay.chaudhury50@gmail.com last updated on 21/Jan/19

∫_0 ^1 (1+(1/e^x^2 ))dx this intrgal can be solved withthe help of graph f(x)=1+(1/e^x^2 ) f(0)=2 f(1)=1+e^(−1) =0<∫_0 ^1 (1+(1/e^x^2 ))<(1/2){f(0)+f(1)}×1 0<I<(1/2)(2+1+e^(−1) ) 0<I<(1/2)(3+(1/e)) 0<I<1.68 but from attached graph the area is A+B but we found A=(1/2)(3+(1/e)) so actual value of ∫_0 ^1 (1+(1/e^x^2 ))dx=A+B

$$\int_{\mathrm{0}} ^{\mathrm{1}} \left(\mathrm{1}+\frac{\mathrm{1}}{{e}^{{x}^{\mathrm{2}} } }\right){dx} \\ $$$${this}\:{intrgal}\:{can}\:{be}\:{solved}\:{withthe}\:{help}\:{of}\:{graph} \\ $$$${f}\left({x}\right)=\mathrm{1}+\frac{\mathrm{1}}{{e}^{{x}^{\mathrm{2}} } } \\ $$$${f}\left(\mathrm{0}\right)=\mathrm{2} \\ $$$${f}\left(\mathrm{1}\right)=\mathrm{1}+{e}^{−\mathrm{1}} \\ $$$$=\mathrm{0}<\int_{\mathrm{0}} ^{\mathrm{1}} \left(\mathrm{1}+\frac{\mathrm{1}}{{e}^{{x}^{\mathrm{2}} } }\right)<\frac{\mathrm{1}}{\mathrm{2}}\left\{{f}\left(\mathrm{0}\right)+{f}\left(\mathrm{1}\right)\right\}×\mathrm{1} \\ $$$$\:\:\mathrm{0}<{I}<\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{2}+\mathrm{1}+{e}^{−\mathrm{1}} \right) \\ $$$$\mathrm{0}<{I}<\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{3}+\frac{\mathrm{1}}{{e}}\right) \\ $$$$\mathrm{0}<{I}<\mathrm{1}.\mathrm{68} \\ $$$${but}\:{from}\:{attached}\:{graph}\:{the}\:{area}\:{is}\:{A}+{B} \\ $$$${but}\:{we}\:{found}\:{A}=\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{3}+\frac{\mathrm{1}}{{e}}\right) \\ $$$${so}\:{actual}\:{value}\:{of}\:\int_{\mathrm{0}} ^{\mathrm{1}} \left(\mathrm{1}+\frac{\mathrm{1}}{{e}^{{x}^{\mathrm{2}} } }\right){dx}={A}+{B} \\ $$

Commented by tanmay.chaudhury50@gmail.com last updated on 21/Jan/19

Terms of Service

Privacy Policy

Contact: info@tinkutara.com