Math Question and Answers


Question and Answers Forum

All Questions Topic List
Geometry Questions
Previous in All Question Next in All Question
Previous in Geometry Next in Geometry
Question Number 53570 by ajfour last updated on 23/Jan/19

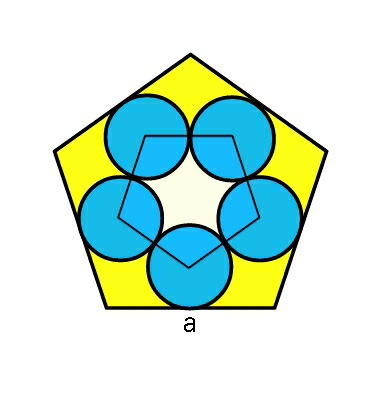
Commented by ajfour last updated on 23/Jan/19

$R e g u l a r p e n t a g o n s i d e a . F i n d t h e$ $c e n t r a l u n c o l o u r e d a r e a .$
Commented by tanmay.chaudhury50@gmail.com last updated on 23/Jan/19

$n o c o m m e n t s r e c e i v e d . . h e n c e d e l e t e d$
Commented by tanmay.chaudhury50@gmail.com last updated on 23/Jan/19

Commented by ajfour last updated on 23/Jan/19

$a s s o o n a s i s a w i h a d c o m m e n t e d,$ $t h a n k s s i r, i r e m e m b e r y o u r a n s w e r$ $e v e n .$ $A_{w h i t e} = \frac{a^{2}}{4} \tan^{2} 27 ° (5 \cot 36 ° - \frac{3 π}{2})$ $i t m a t c h e d w i t h w h a t i c o u l d o b t a i n .$
Commented by ajfour last updated on 23/Jan/19

$T a n m a y S i r, w h y y o u d e l e t e d y o u r$ $p o s t e d s o l u t i o n ?$
Commented by tanmay.chaudhury50@gmail.com last updated on 23/Jan/19

$t a n α = \frac{r}{\frac{a}{2}} r = \frac{a}{2} t a n α . . . . (1)$ $5 θ = 2 π θ = \frac{2 π}{5} . . . . . (2)$ $2 β + θ = π β = \frac{π - \frac{2 π}{5}}{2} = \frac{3 π}{10} . . . . (3)$ $n o w a r e a o f s m a l l p e n t a g o n . . .$ $5 \times \frac{1}{2} \times 2 r \times h$ $t a n \frac{θ}{2} = \frac{r}{h} s o h = r c o t \frac{θ}{2}$ $s o a r e a o f s m a l l p e n t a g o n = 5 \times \frac{1}{2} \times 2 r \times r c o t (\frac{θ}{2})$ $a r e a o f f i v e s e c t o r o f c i r c l e = 5 \times \frac{π r^{2}}{2 π} \times 2 β$ $= 5 r^{2} β$ $s o a r e a o f w h i t e c e n t r e s t a r$ $= a r e a o f s m a l l p e n t a g o n - f i v e s e c t o r a r e a$ $= 5 r^{2} c o t (\frac{θ}{2}) - 5 r^{2} β$ $= 5 r^{2} [c o t (\frac{θ}{2}) - β]$ $= 5 \times {(\frac{a}{2} t a n α)}^{2} [c o t (\frac{θ}{2}) - β]$ $n o w θ = \frac{2 π}{5} β = \frac{3 π}{10}$ $t o f i n d α . . .$ $u s i n g f o r m u l a . . .$ $n u m b e r o f s i d e = \frac{360}{e x t e r n a l a n g k e}$ $e x t e r n a l a n g k e = \frac{360}{5} = 72^{o}$ $i n t e r n a l + e x t e r n a l a n g l d = 180^{o}$ $i n y e r n s l a n g l d = 180^{o} - 72 = 108^{o}$ $4 α = 108 = s o α = 27^{o}$ $h d n c e r e q u i r e d a n s w e r i s$ $= ★ ★ 5 \times {(\frac{a}{2} t a n α)}^{2} [c o t (\frac{θ}{2}) - β] ★ ★$ $= 5 \times {(\frac{a}{2} t a n 27^{o})}^{2} [c o t (\frac{2 π}{5 \times 2}) - \frac{3 π}{10}]$ $= \frac{a^{2}}{4} {t a n}^{2} 27^{o} \times [5 c o t 36^{o} - \frac{3 π}{2}]$ $n o w p l s c h e c k . . .$
Commented by tanmay.chaudhury50@gmail.com last updated on 23/Jan/19

$t h a n k y o u . .$
	Answered by mr W last updated on 23/Jan/19

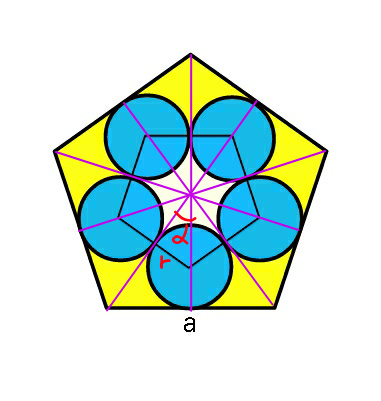
	Commented by mr W last updated on 23/Jan/19

	$α = \frac{2 π}{10} = \frac{π}{5}$ $(r + \frac{r}{\sin α}) \tan α = \frac{a}{2}$ $r = \frac{a \cos α}{2 (1 + \sin α)}$ $A = 10 [\frac{r^{2}}{2 \tan α} - \frac{r^{2}}{2} (\frac{π}{2} - α)]$ $A = 5 r^{2} [α + \frac{1}{\tan α} - \frac{π}{2}]$ $A = \frac{5 a^{2} \cos^{2} α}{4 {(1 + \sin α)}^{2}} [\frac{π}{5} + \frac{1}{\tan α} - \frac{π}{2}]$ $\cos α = \frac{\sqrt{5} + 1}{4}$ $\sin α = \frac{\sqrt{2 (5 - \sqrt{5})}}{4}$ $\tan α = \sqrt{5 - 2 \sqrt{5}}$ $A = \frac{a^{2} \cos^{2} α}{8 {(1 + \sin α)}^{2}} [\frac{10}{\tan α} - 3 π]$ $A = \frac{a^{2} {(\sqrt{5} + 1)}^{2}}{8 {(4 + \sqrt{2 (5 - \sqrt{5}})}^{2}} [\frac{10 \sqrt{5 + 2 \sqrt{5}}}{\sqrt{5}} - 3 π]$ $\Rightarrow A = \frac{a^{2} (3 + \sqrt{5}) [2 \sqrt{5 (5 + 2 \sqrt{5}}) - 3 π]}{8 [13 - \sqrt{5} + 4 \sqrt{2 (5 - \sqrt{5})}]} \approx 0.14081 a^{2}$
	Commented by ajfour last updated on 23/Jan/19

	$T h a n k s, b e a u t i f u l w a y s i r .$
	Commented by mr W last updated on 23/Jan/19

	$t h a n k s s i r!$
	Commented by Otchere Abdullai last updated on 24/Jan/19

	$m y p r o f$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum