Question and Answers Forum

All Questions      Topic List

Number Theory Questions

Previous in All Question      Next in All Question      

Previous in Number Theory      Next in Number Theory      

Question Number 53618 by kaivan.ahmadi last updated on 23/Jan/19

Let x,y∈Z if x^2 +y^2 divide xy+1 then prove ((x^2 +y^2 )/(xy+1)) is square of integer number

$$\mathrm{Let}\:\mathrm{x},\mathrm{y}\in\mathbb{Z} \\ $$$$\mathrm{if}\:\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} \:\mathrm{divide}\:\mathrm{xy}+\mathrm{1}\:\mathrm{then}\:\mathrm{prove} \\ $$$$\frac{\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} }{\mathrm{xy}+\mathrm{1}}\:\mathrm{is}\:\mathrm{square}\:\mathrm{of}\:\mathrm{integer}\:\mathrm{number} \\ $$

Commented by mr W last updated on 24/Jan/19

i think there is only one possibility: x=y=1 or −1

$${i}\:{think}\:{there}\:{is}\:{only}\:{one}\:{possibility}: \\ $$$${x}={y}=\mathrm{1}\:{or}\:−\mathrm{1} \\ $$

Commented by kaivan.ahmadi last updated on 24/Jan/19

thank you, chek the proof....

$$\mathrm{thank}\:\mathrm{you},\:\mathrm{chek}\:\mathrm{the}\:\mathrm{proof}.... \\ $$

Commented by Kunal12588 last updated on 28/Mar/19

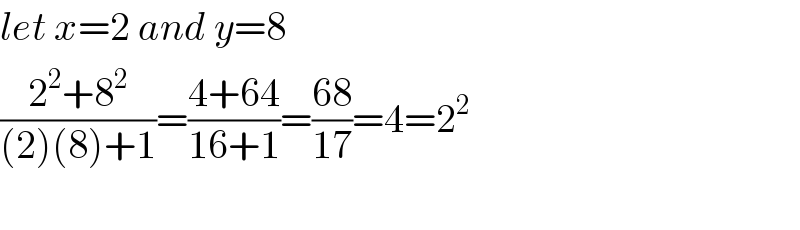
let x=2 and y=8 ((2^2 +8^2 )/((2)(8)+1))=((4+64)/(16+1))=((68)/(17))=4=2^2

$${let}\:{x}=\mathrm{2}\:{and}\:{y}=\mathrm{8} \\ $$$$\frac{\mathrm{2}^{\mathrm{2}} +\mathrm{8}^{\mathrm{2}} }{\left(\mathrm{2}\right)\left(\mathrm{8}\right)+\mathrm{1}}=\frac{\mathrm{4}+\mathrm{64}}{\mathrm{16}+\mathrm{1}}=\frac{\mathrm{68}}{\mathrm{17}}=\mathrm{4}=\mathrm{2}^{\mathrm{2}} \\ $$$$ \\ $$

Answered by kaivan.ahmadi last updated on 24/Jan/19

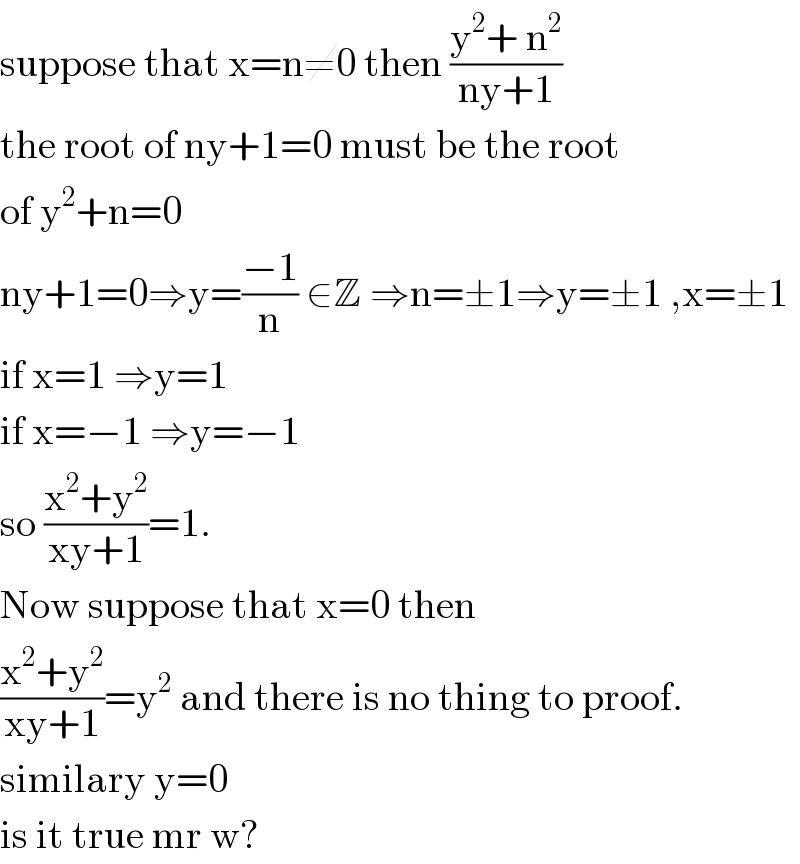
suppose that x=n≠0 then ((y^2 + n^2 )/(ny+1)) the root of ny+1=0 must be the root of y^2 +n=0 ny+1=0⇒y=((−1)/n) ∈Z ⇒n=±1⇒y=±1 ,x=±1 if x=1 ⇒y=1 if x=−1 ⇒y=−1 so ((x^2 +y^2 )/(xy+1))=1. Now suppose that x=0 then ((x^2 +y^2 )/(xy+1))=y^2 and there is no thing to proof. similary y=0 is it true mr w?

$$\mathrm{suppose}\:\mathrm{that}\:\mathrm{x}=\mathrm{n}\neq\mathrm{0}\:\mathrm{then}\:\frac{\mathrm{y}^{\mathrm{2}} +\:\mathrm{n}^{\mathrm{2}} }{\mathrm{ny}+\mathrm{1}} \\ $$$$\mathrm{the}\:\mathrm{root}\:\mathrm{of}\:\mathrm{ny}+\mathrm{1}=\mathrm{0}\:\mathrm{must}\:\mathrm{be}\:\mathrm{the}\:\mathrm{root} \\ $$$$\mathrm{of}\:\mathrm{y}^{\mathrm{2}} +\mathrm{n}=\mathrm{0} \\ $$$$\mathrm{ny}+\mathrm{1}=\mathrm{0}\Rightarrow\mathrm{y}=\frac{−\mathrm{1}}{\mathrm{n}}\:\in\mathbb{Z}\:\Rightarrow\mathrm{n}=\pm\mathrm{1}\Rightarrow\mathrm{y}=\pm\mathrm{1}\:,\mathrm{x}=\pm\mathrm{1} \\ $$$$\mathrm{if}\:\mathrm{x}=\mathrm{1}\:\Rightarrow\mathrm{y}=\mathrm{1} \\ $$$$\mathrm{if}\:\mathrm{x}=−\mathrm{1}\:\Rightarrow\mathrm{y}=−\mathrm{1} \\ $$$$\mathrm{so}\:\frac{\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} }{\mathrm{xy}+\mathrm{1}}=\mathrm{1}. \\ $$$$\mathrm{Now}\:\mathrm{suppose}\:\mathrm{that}\:\mathrm{x}=\mathrm{0}\:\mathrm{then} \\ $$$$\frac{\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} }{\mathrm{xy}+\mathrm{1}}=\mathrm{y}^{\mathrm{2}} \:\mathrm{and}\:\mathrm{there}\:\mathrm{is}\:\mathrm{no}\:\mathrm{thing}\:\mathrm{to}\:\mathrm{proof}. \\ $$$$\mathrm{similary}\:\mathrm{y}=\mathrm{0} \\ $$$$\mathrm{is}\:\mathrm{it}\:\mathrm{true}\:\mathrm{mr}\:\mathrm{w}? \\ $$

Commented by mr W last updated on 24/Jan/19

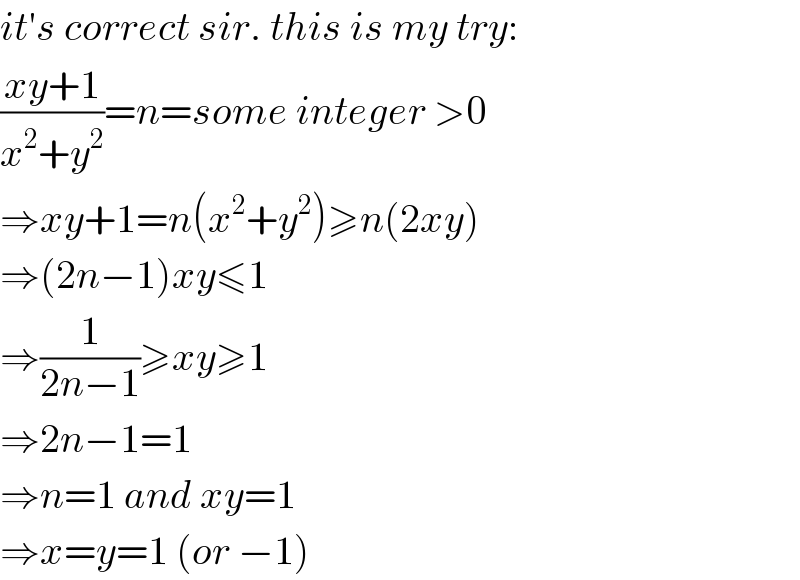
it′s correct sir. this is my try: ((xy+1)/(x^2 +y^2 ))=n=some integer >0 ⇒xy+1=n(x^2 +y^2 )≥n(2xy) ⇒(2n−1)xy≤1 ⇒(1/(2n−1))≥xy≥1 ⇒2n−1=1 ⇒n=1 and xy=1 ⇒x=y=1 (or −1)

$${it}'{s}\:{correct}\:{sir}.\:{this}\:{is}\:{my}\:{try}: \\ $$$$\frac{{xy}+\mathrm{1}}{{x}^{\mathrm{2}} +{y}^{\mathrm{2}} }={n}={some}\:{integer}\:>\mathrm{0} \\ $$$$\Rightarrow{xy}+\mathrm{1}={n}\left({x}^{\mathrm{2}} +{y}^{\mathrm{2}} \right)\geqslant{n}\left(\mathrm{2}{xy}\right) \\ $$$$\Rightarrow\left(\mathrm{2}{n}−\mathrm{1}\right){xy}\leqslant\mathrm{1} \\ $$$$\Rightarrow\frac{\mathrm{1}}{\mathrm{2}{n}−\mathrm{1}}\geqslant{xy}\geqslant\mathrm{1} \\ $$$$\Rightarrow\mathrm{2}{n}−\mathrm{1}=\mathrm{1} \\ $$$$\Rightarrow{n}=\mathrm{1}\:{and}\:{xy}=\mathrm{1} \\ $$$$\Rightarrow{x}={y}=\mathrm{1}\:\left({or}\:−\mathrm{1}\right) \\ $$

Commented by kaivan.ahmadi last updated on 24/Jan/19

very nice.attaching x=0 or y=0 complete the proof. thank you sir.

$$\mathrm{very}\:\mathrm{nice}.\mathrm{attaching}\:\:\mathrm{x}=\mathrm{0}\:\mathrm{or}\:\mathrm{y}=\mathrm{0}\:\mathrm{complete}\:\mathrm{the}\:\mathrm{proof}. \\ $$$$\mathrm{thank}\:\mathrm{you}\:\mathrm{sir}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com