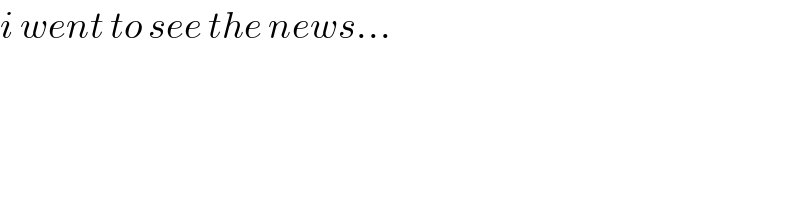Question and Answers Forum
Question Number 53732 by ajfour last updated on 25/Jan/19

Commented by mr W last updated on 25/Jan/19
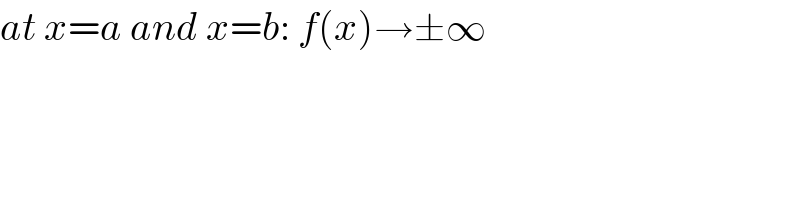
Commented by ajfour last updated on 25/Jan/19

Commented by mr W last updated on 25/Jan/19

Commented by ajfour last updated on 25/Jan/19

Commented by mr W last updated on 25/Jan/19
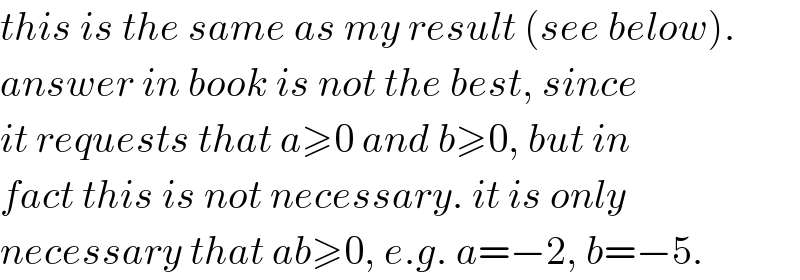
Commented by mr W last updated on 25/Jan/19

Commented by ajfour last updated on 25/Jan/19
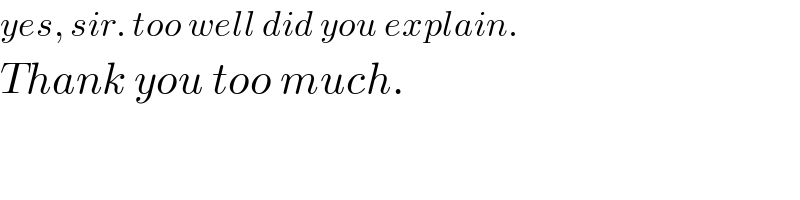
Answered by tanmay.chaudhury50@gmail.com last updated on 25/Jan/19
![y=(((x+a)(x+b))/((x−a)(x−b))) lny=ln(x+a)+ln(x+b)−ln(x−a)−ln(x−b) (1/y)(dy/dx)=(1/(x+a))+(1/(x+b))−(1/(x−a))−(1/(x−b)) for max/min (dy/dx)=0 (1/(x+a))−(1/(x−b))+(1/(x+b))−(1/(x−a))=0 ((x−b−x−a)/((x+a)(x−b)))+((x−a−x−b)/((x+b)(x−a)))=0 ((−(a+b))/((x+a)(x−b)))+((−(a+b))/((x+b)(x−a)))=0 (1/((x+a)(x−b)))+(1/((x+b)(x−a)))=0 x^2 −ax+bx−ab+x^2 −bx+ax−ab=0 2x^2 −2ab=0 x=±(√(ab)) (1/y)(dy/dx)=(1/(x+a))+(1/(x+b))−(1/(x−a))−(1/(x−b)) (dy/dx)=(((x+a)(x+b))/((x−a)(x−b)))[(1/(x+a))+(1/(x+b))−(1/(x−a))−(1/(x−b))] ((wait...)/)wl now i am going to find change of sign using first derivativd method to find max/min ((dy/dx))=(((x+a)(x+b))/(x^2 −x(a+b)+ab))×[(1/(x+a))−(1/(x−b))+(1/(x+b))−(1/(x−a))] =((x^2 +x(a+b)+ab)/(x^2 −x(a+b)+ab))×[((x−b−x−a)/(x^2 −xb+ax−ab))+((x−a−x−b)/(x^2 −ax+bx−ab))] =(N/D)×[((−(a+b))/(x^2 −ab+x(a−b)))+((−(a+b))/(x^2 −ab−x(a−b)))] =(N/D)×−(a+b)×[((x^2 −ab−x(a−b)+x^2 −ab+x(a−b))/((x^2 −ab)^2 −x^2 (a−b)^2 ))] nowD<N so(N/D)>1 (N/D)×−(a+b)=−ve [((2(x^2 −ab))/((x^2 −ab)^2 −x^2 {(a+b)^2 −4ab))] when x>(√(ab)) x^2 =h+ab =(N/D)×−(a+b)×[((2(x^2 −ab))/((x^2 −ab)^2 −x^2 {(a+b)^2 −4ab))] =(−ve)×[((2h)/(h^2 −(h+ab)(a−b)^2 ))]=(−ve)×((+ve)/(−ve)) when x>(√(ab)) sign change from −ve to +ve so at x=(√(ab)) f(x) min y_ =(((x+a)(x+b))/((x−a)(x−b))) (at x=(√(ab)) ) =(((√a) ((√b) +(√a) )×(√b) ((√a) +(√b) ))/((√a) ((√b) −(√a) )×(√b) ((√a) −(√b) ))) =(−)((((√a) +(√b) )^2 )/(((√(b−))(√a) )^2 )) →min value so at x=(√(ab)) min value at x=−(√(ab)) max value y=(((−(√(ab)) +a)(−(√(ab)) +b))/((−(√(ab)) −a)(−(√(ab)) −b))) =(((√a) ((√a) −(√b) )×−(√b) ((√a) −(√b) ))/(−(√a) ((√a) +(√b) )×−(√b) ((√a) +(√b) ))) =(−1)×((((√a) −(√b) )^2 )/(((√a) +(√b) )))←max vzlud...](Q53742.png)
Commented by ajfour last updated on 25/Jan/19

Commented by ajfour last updated on 25/Jan/19
Commented by tanmay.chaudhury50@gmail.com last updated on 25/Jan/19