
Question Number 54074 by rahul 19 last updated on 28/Jan/19
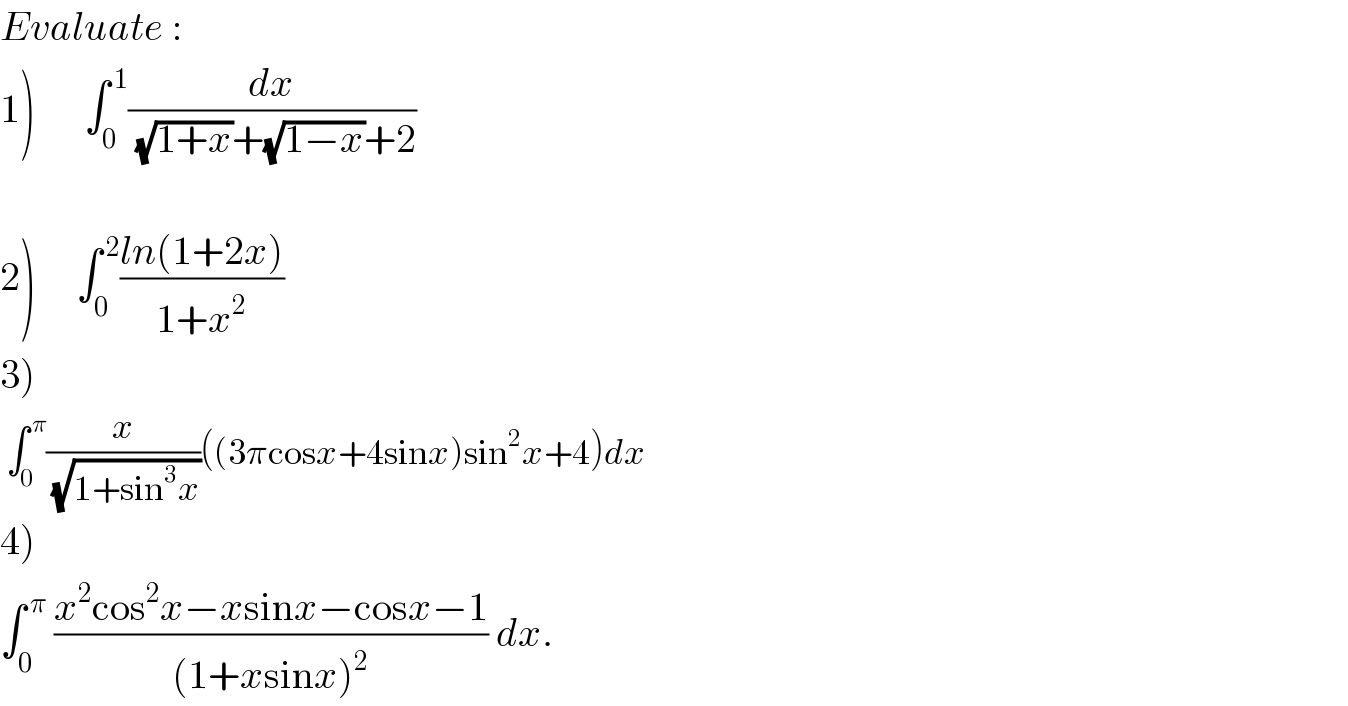
$${Evaluate}\:: \\ $$$$\left.\mathrm{1}\right)\:\:\:\:\:\:\int_{\mathrm{0}} ^{\:\mathrm{1}} \frac{{dx}}{\sqrt{\mathrm{1}+{x}}+\sqrt{\mathrm{1}−{x}}+\mathrm{2}}\: \\ $$$$ \\ $$$$\left.\mathrm{2}\right)\:\:\:\:\:\int_{\mathrm{0}} ^{\:\mathrm{2}} \frac{{ln}\left(\mathrm{1}+\mathrm{2}{x}\right)}{\mathrm{1}+{x}^{\mathrm{2}} } \\ $$$$\left.\mathrm{3}\right) \\ $$$$\:\int_{\mathrm{0}} ^{\:\pi} \frac{{x}}{\sqrt{\mathrm{1}+\mathrm{sin}^{\mathrm{3}} {x}}}\left(\left(\mathrm{3}\pi\mathrm{cos}{x}+\mathrm{4sin}{x}\right)\mathrm{sin}^{\mathrm{2}} {x}+\mathrm{4}\right){dx} \\ $$$$\left.\mathrm{4}\right) \\ $$$$\int_{\mathrm{0}} ^{\:\pi} \:\frac{{x}^{\mathrm{2}} \mathrm{cos}^{\mathrm{2}} {x}−{x}\mathrm{sin}{x}−\mathrm{cos}{x}−\mathrm{1}}{\left(\mathrm{1}+{x}\mathrm{sin}{x}\right)^{\mathrm{2}} }\:{dx}. \\ $$
Commented by maxmathsup by imad last updated on 28/Jan/19

$$\left.\mathrm{1}\right)\:{let}\:{I}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\:\frac{{dx}}{\sqrt{\mathrm{1}+{x}}\:+\sqrt{\mathrm{1}−{x}}\:+\mathrm{2}}\:\:{changement}\:{x}={cos}\:\theta\:\:{give}\: \\ $$$${I}\:=\:−\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\:\frac{−{sin}\theta\:{d}\theta}{\sqrt{\mathrm{2}}{cos}\left(\frac{\theta}{\mathrm{2}}\right)+\sqrt{\mathrm{2}}{sin}\left(\frac{\theta}{\mathrm{2}}\right)\:+\mathrm{2}}\:=\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\frac{{sin}\theta}{{cos}\left(\frac{\theta}{\mathrm{2}}\right)\:+{sin}\left(\frac{\theta}{\mathrm{2}}\right)+\sqrt{\mathrm{2}}}{d}\theta \\ $$$$=_{\frac{\theta}{\mathrm{2}}={t}} \:\:\:\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\:\frac{{sin}\left(\mathrm{2}{t}\right)}{{cost}\:+{sint}\:+\sqrt{\mathrm{2}}}\:\left(\mathrm{2}\right){dt}\:=\sqrt{\mathrm{2}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\frac{{sin}\left(\mathrm{2}{t}\right)}{{cost}\:+{sint}\:+\sqrt{\mathrm{2}}}\:{dt} \\ $$$$=\mathrm{2}\sqrt{\mathrm{2}}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\frac{{sint}\:{cost}}{{cost}\:+{sint}\:+\sqrt{\mathrm{2}}}\:{dt}\:=_{{tan}\left(\frac{{t}}{\mathrm{2}}\right)\:={x}} \:\:\:\:\mathrm{2}\sqrt{\mathrm{2}}\int_{\mathrm{0}} ^{\sqrt{\mathrm{2}}−\mathrm{1}} \:\:\:\:\frac{\frac{\mathrm{2}{x}\left(\mathrm{1}−{x}^{\mathrm{2}} \right)}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{\mathrm{2}} }}{\frac{\mathrm{1}−{x}^{\mathrm{2}} }{\mathrm{1}+{x}^{\mathrm{2}} }\:+\frac{\mathrm{2}{x}}{\mathrm{1}+{x}^{\mathrm{2}} }\:+\sqrt{\mathrm{2}}}\:\frac{\mathrm{2}{dx}}{\mathrm{1}+{x}^{\mathrm{2}} } \\ $$$$=\mathrm{8}\sqrt{\mathrm{2}}\int_{\mathrm{0}} ^{\sqrt{\mathrm{2}}−\mathrm{1}} \:\:\:\:\:\:\frac{{x}\left(\mathrm{1}−{x}^{\mathrm{2}} \right)}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{\mathrm{2}} \left(\mathrm{1}−{x}^{\mathrm{2}} \:+\mathrm{2}{x}+\sqrt{\mathrm{2}}\left(\mathrm{1}+{x}^{\mathrm{2}} \right)\right)}{dx} \\ $$$$=\mathrm{8}\sqrt{\mathrm{2}}\int_{\mathrm{0}} ^{\sqrt{\mathrm{2}}−\mathrm{1}} \:\:\:\frac{{x}\left(\mathrm{1}−{x}^{\mathrm{2}} \right)}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{\mathrm{2}} \left(\left(\sqrt{\mathrm{2}}−\mathrm{1}\right){x}^{\mathrm{2}} \:+\mathrm{2}{x}\:+\mathrm{1}+\sqrt{\mathrm{2}}\right)}{dx}\:{let}\:{decompose} \\ $$$${F}\left({x}\right)\:=\frac{{x}\left(\mathrm{1}−{x}^{\mathrm{2}} \right)}{\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{2}} \left\{\:\left(\sqrt{\mathrm{2}}−\mathrm{1}\right){x}^{\mathrm{2}} \:+\mathrm{2}{x}\:+\mathrm{1}+\sqrt{\mathrm{2}}\right\}} \\ $$$${roots}\:{of}\:\left(\sqrt{\mathrm{2}}−\mathrm{1}\right){x}^{\mathrm{2}} \:+\mathrm{2}{x}\:+\mathrm{1}+\sqrt{\mathrm{2}} \\ $$$$\Delta\:=\mathrm{4}−\mathrm{4}\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)\left(\sqrt{\mathrm{2}}+\mathrm{1}\right)\:=\mathrm{0}\:\Rightarrow{one}\:{root}\:{x}_{\mathrm{0}} =−\frac{{b}}{\mathrm{2}{a}}\:=−\frac{\mathrm{2}}{\mathrm{2}\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)}\:=\frac{−\mathrm{1}}{\sqrt{\mathrm{2}}−\mathrm{1}}\:\Rightarrow \\ $$$$\left(\sqrt{\mathrm{2}}−\mathrm{1}\right){x}^{\mathrm{2}} \:+\mathrm{2}{x}\:+\mathrm{1}+\sqrt{\mathrm{2}}=\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)\left({x}−{x}_{\mathrm{0}} \right)^{\mathrm{2}} \:=\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)\left({x}+\frac{\mathrm{1}}{\sqrt{\mathrm{2}}−\mathrm{1}}\right)^{\mathrm{2}} \\ $$$$=\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)\left({x}\:+\sqrt{\mathrm{2}}+\mathrm{1}\right)^{\mathrm{2}} \:\Rightarrow \\ $$$${F}\left({x}\right)\:=\frac{{a}}{{x}+\sqrt{\mathrm{2}}\:+\mathrm{1}}\:+\frac{{b}}{\left({x}+\sqrt{\mathrm{2}}\:+\mathrm{1}\right)^{\mathrm{2}} }\:+\frac{{cx}\:+{d}}{{x}^{\mathrm{2}} \:+\mathrm{1}}\:+\:\frac{{ex}\:+{f}}{\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{2}} } \\ $$$${b}={lim}_{{x}\rightarrow−\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)} \:\:\:\:\left({x}+\sqrt{\mathrm{2}}+\mathrm{1}\right)^{\mathrm{2}} {F}\left({x}\right)....{after}\:{finding}\:{the}\:{coefficients} \\ $$$${we}\:{get}\:\int\:{F}\left({x}\right){dx}\:={aln}\mid{x}+\sqrt{\mathrm{2}}+\mathrm{1}\mid−\frac{{b}}{\left({x}+\sqrt{\mathrm{2}}\:+\mathrm{1}\right)}\:+\int\:\:\frac{{cx}+{d}}{{x}^{\mathrm{2}} \:+\mathrm{1}}{dx}\:+\int\:\:\frac{{ex}\:+{f}}{\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{2}} }{dx} \\ $$$$...{be}\:{continued}... \\ $$
Commented by maxmathsup by imad last updated on 28/Jan/19
![2) let f(t) =∫_0 ^2 ((ln(1+tx))/(1+x^2 )) dx with t>0 ⇒ f^′ (t) =∫_0 ^2 (x/((1+tx)(1+x^2 )))dx =_(tx =u) ∫_0 ^(2t) (u/(t(1+u)(1+(u^2 /t^2 )))) (du/t) =∫_0 ^(2t) ((udu)/((u+1)(u^2 +t^2 ))) let decompose F(u) = (u/((u+1)(u^2 +t^2 ))) ⇒ F(u) =(a/(u+1)) +((bu +c)/(u^2 +t^2 )) a =lim_(u→−1) (u+1)F(u) =−(1/(t^2 +1)) lim_(u→+∞) u F(u) =0 =a+b ⇒b =(1/(t^2 +1)) F(0) =0 =a +(c/t^2 ) ⇒c =−at^2 =(t^2 /(t^(2 ) +1)) ⇒ F(u) =−(1/((t^2 +1)(u+1))) +(((1/(t^2 +1 ))u +(t^2 /(t^2 +1)))/(u^2 +t^2 )) =(1/(t^2 +1)){ −(1/(u+1)) +((u +t^2 )/(u^2 +t^2 ))} ⇒∫_0 ^(2t) F(u)du =(1/(t^2 +1)) {−∫_0 ^(2t) (du/(u+1)) +∫_0 ^(2t) ((u+t^2 )/(u^2 +t^2 )) du} =(1/(t^2 +1)){−[ln∣u+1∣]_0 ^(2t) + (1/2)[ln(u^2 +t^2 )]_0 ^(2t) +2t^2 ∫_0 ^(2t) (du/(u^2 +t^2 ))} =(1/(t^2 +1)){ −ln(1+2t) +(1/2)( ln(5t^2 )−ln(t^2 )) +2t^2 ∫_0 ^(2t) (du/(u^2 +t^2 ))} =−((ln(1+2t))/(t^2 +1)) +((ln(5))/(2(t^2 +1))) +((2t^2 )/(t^2 +1)) ∫_0 ^(2t) (du/(u^2 +t^2 )) but ∫_0 ^(2t) (du/(u^2 +t^2 )) =_(u =tα) ∫_0 ^2 ((tdα)/(t^2 (1+α^2 ))) =(1/t) ∫_0 ^2 (dα/(1+α^2 )) =((arctan(2))/t) ⇒ f^′ (t) =−((ln(1+2t))/(t^2 +1)) +((ln(5))/(2(t^2 +1))) +((2arctan(2)t)/(t^2 +1)) ⇒ f(t) =−∫_0 ^t ((ln(1+2x))/(1+x^2 ))dx +((ln(5))/2) ∫_0 ^t (dx/(1+x^2 )) + arctan(2) ∫_0 ^t ((2x)/(1+x^2 ))dx +c f(t) =−∫_0 ^t ((ln(1+2x))/(1+x^2 ))dx +((ln(5))/2) arctan(t) +arctan(2)ln(1+t^2 ) +c f(0)=0 ⇒c =0 ⇒ f(t) =−∫_0 ^t ((ln(1+2x))/(1+x^2 ))dx +((ln(5))/2) arctan(t) +arctan(2)ln(1+t^2 ) =∫_0 ^2 ((ln(1+tx))/(1+x^2 ))dx we have f(2) =−∫_0 ^2 ((ln(1+2x))/(1+x^2 )) dx +((ln(5))/2) arctan(2) +ln(5)arctan(2) =∫_0 ^2 ((ln(1+2x))/(1+x^2 ))dx ⇒ 2 ∫_0 ^2 ((ln(1+2x))/(1+x^2 ))dx =(3/2)ln(5) arctan(2) ⇒ ∫_0 ^2 ((ln(1+2x))/(1+x^2 ))dx =(3/4)ln(5)arctan(2) .i](Q54091.png)
$$\left.\mathrm{2}\right)\:{let}\:{f}\left({t}\right)\:=\int_{\mathrm{0}} ^{\mathrm{2}} \:\frac{{ln}\left(\mathrm{1}+{tx}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }\:{dx}\:\:{with}\:{t}>\mathrm{0}\:\Rightarrow \\ $$$${f}^{'} \left({t}\right)\:=\int_{\mathrm{0}} ^{\mathrm{2}} \:\:\frac{{x}}{\left(\mathrm{1}+{tx}\right)\left(\mathrm{1}+{x}^{\mathrm{2}} \right)}{dx}\:=_{{tx}\:={u}} \:\:\int_{\mathrm{0}} ^{\mathrm{2}{t}} \:\:\:\frac{{u}}{{t}\left(\mathrm{1}+{u}\right)\left(\mathrm{1}+\frac{{u}^{\mathrm{2}} }{{t}^{\mathrm{2}} }\right)}\:\frac{{du}}{{t}} \\ $$$$=\int_{\mathrm{0}} ^{\mathrm{2}{t}} \:\:\:\frac{{udu}}{\left({u}+\mathrm{1}\right)\left({u}^{\mathrm{2}} \:+{t}^{\mathrm{2}} \right)}\:\:{let}\:{decompose}\:{F}\left({u}\right)\:=\:\frac{{u}}{\left({u}+\mathrm{1}\right)\left({u}^{\mathrm{2}} \:+{t}^{\mathrm{2}} \right)}\:\Rightarrow \\ $$$${F}\left({u}\right)\:=\frac{{a}}{{u}+\mathrm{1}}\:+\frac{{bu}\:+{c}}{{u}^{\mathrm{2}} \:+{t}^{\mathrm{2}} } \\ $$$${a}\:={lim}_{{u}\rightarrow−\mathrm{1}} \left({u}+\mathrm{1}\right){F}\left({u}\right)\:=−\frac{\mathrm{1}}{{t}^{\mathrm{2}} \:+\mathrm{1}} \\ $$$${lim}_{{u}\rightarrow+\infty} {u}\:{F}\left({u}\right)\:=\mathrm{0}\:={a}+{b}\:\Rightarrow{b}\:=\frac{\mathrm{1}}{{t}^{\mathrm{2}} \:+\mathrm{1}} \\ $$$${F}\left(\mathrm{0}\right)\:=\mathrm{0}\:={a}\:+\frac{{c}}{{t}^{\mathrm{2}} }\:\Rightarrow{c}\:=−{at}^{\mathrm{2}} \:=\frac{{t}^{\mathrm{2}} }{{t}^{\mathrm{2}\:} \:+\mathrm{1}}\:\Rightarrow \\ $$$${F}\left({u}\right)\:=−\frac{\mathrm{1}}{\left({t}^{\mathrm{2}} \:+\mathrm{1}\right)\left({u}+\mathrm{1}\right)}\:+\frac{\frac{\mathrm{1}}{{t}^{\mathrm{2}} \:+\mathrm{1}\:}{u}\:+\frac{{t}^{\mathrm{2}} }{{t}^{\mathrm{2}} \:+\mathrm{1}}}{{u}^{\mathrm{2}} \:+{t}^{\mathrm{2}} } \\ $$$$=\frac{\mathrm{1}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\left\{\:−\frac{\mathrm{1}}{{u}+\mathrm{1}}\:+\frac{{u}\:+{t}^{\mathrm{2}} }{{u}^{\mathrm{2}} \:+{t}^{\mathrm{2}} }\right\}\:\Rightarrow\int_{\mathrm{0}} ^{\mathrm{2}{t}} \:\:{F}\left({u}\right){du} \\ $$$$=\frac{\mathrm{1}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:\left\{−\int_{\mathrm{0}} ^{\mathrm{2}{t}} \:\frac{{du}}{{u}+\mathrm{1}}\:+\int_{\mathrm{0}} ^{\mathrm{2}{t}} \:\:\frac{{u}+{t}^{\mathrm{2}} }{{u}^{\mathrm{2}} \:+{t}^{\mathrm{2}} }\:{du}\right\} \\ $$$$=\frac{\mathrm{1}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\left\{−\left[{ln}\mid{u}+\mathrm{1}\mid\right]_{\mathrm{0}} ^{\mathrm{2}{t}} \:+\:\frac{\mathrm{1}}{\mathrm{2}}\left[{ln}\left({u}^{\mathrm{2}} \:+{t}^{\mathrm{2}} \right)\right]_{\mathrm{0}} ^{\mathrm{2}{t}} \:\:+\mathrm{2}{t}^{\mathrm{2}} \:\int_{\mathrm{0}} ^{\mathrm{2}{t}} \:\:\:\frac{{du}}{{u}^{\mathrm{2}} \:+{t}^{\mathrm{2}} }\right\} \\ $$$$=\frac{\mathrm{1}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\left\{\:−{ln}\left(\mathrm{1}+\mathrm{2}{t}\right)\:+\frac{\mathrm{1}}{\mathrm{2}}\left(\:{ln}\left(\mathrm{5}{t}^{\mathrm{2}} \right)−{ln}\left({t}^{\mathrm{2}} \right)\right)\:+\mathrm{2}{t}^{\mathrm{2}} \:\int_{\mathrm{0}} ^{\mathrm{2}{t}} \:\:\frac{{du}}{{u}^{\mathrm{2}} \:+{t}^{\mathrm{2}} }\right\} \\ $$$$=−\frac{{ln}\left(\mathrm{1}+\mathrm{2}{t}\right)}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:+\frac{{ln}\left(\mathrm{5}\right)}{\mathrm{2}\left({t}^{\mathrm{2}} \:+\mathrm{1}\right)}\:+\frac{\mathrm{2}{t}^{\mathrm{2}} }{{t}^{\mathrm{2}} \:+\mathrm{1}}\:\int_{\mathrm{0}} ^{\mathrm{2}{t}} \:\:\frac{{du}}{{u}^{\mathrm{2}} \:+{t}^{\mathrm{2}} }\:{but} \\ $$$$\int_{\mathrm{0}} ^{\mathrm{2}{t}} \:\:\:\frac{{du}}{{u}^{\mathrm{2}} \:+{t}^{\mathrm{2}} }\:=_{{u}\:={t}\alpha} \:\:\:\int_{\mathrm{0}} ^{\mathrm{2}} \:\:\:\frac{{td}\alpha}{{t}^{\mathrm{2}} \left(\mathrm{1}+\alpha^{\mathrm{2}} \right)}\:=\frac{\mathrm{1}}{{t}}\:\int_{\mathrm{0}} ^{\mathrm{2}} \:\:\frac{{d}\alpha}{\mathrm{1}+\alpha^{\mathrm{2}} }\:=\frac{{arctan}\left(\mathrm{2}\right)}{{t}}\:\Rightarrow \\ $$$${f}^{'} \left({t}\right)\:=−\frac{{ln}\left(\mathrm{1}+\mathrm{2}{t}\right)}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:+\frac{{ln}\left(\mathrm{5}\right)}{\mathrm{2}\left({t}^{\mathrm{2}} \:+\mathrm{1}\right)}\:+\frac{\mathrm{2}{arctan}\left(\mathrm{2}\right){t}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:\Rightarrow \\ $$$${f}\left({t}\right)\:=−\int_{\mathrm{0}} ^{{t}} \:\:\frac{{ln}\left(\mathrm{1}+\mathrm{2}{x}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}\:+\frac{{ln}\left(\mathrm{5}\right)}{\mathrm{2}}\:\int_{\mathrm{0}} ^{{t}} \:\frac{{dx}}{\mathrm{1}+{x}^{\mathrm{2}} }\:+\:{arctan}\left(\mathrm{2}\right)\:\int_{\mathrm{0}} ^{{t}} \:\:\frac{\mathrm{2}{x}}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}\:+{c}\: \\ $$$${f}\left({t}\right)\:=−\int_{\mathrm{0}} ^{{t}} \:\:\frac{{ln}\left(\mathrm{1}+\mathrm{2}{x}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}\:+\frac{{ln}\left(\mathrm{5}\right)}{\mathrm{2}}\:{arctan}\left({t}\right)\:+{arctan}\left(\mathrm{2}\right){ln}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)\:+{c} \\ $$$${f}\left(\mathrm{0}\right)=\mathrm{0}\:\Rightarrow{c}\:=\mathrm{0}\:\Rightarrow \\ $$$${f}\left({t}\right)\:=−\int_{\mathrm{0}} ^{{t}} \:\:\frac{{ln}\left(\mathrm{1}+\mathrm{2}{x}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}\:+\frac{{ln}\left(\mathrm{5}\right)}{\mathrm{2}}\:{arctan}\left({t}\right)\:\:+{arctan}\left(\mathrm{2}\right){ln}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)\:=\int_{\mathrm{0}} ^{\mathrm{2}} \:\frac{{ln}\left(\mathrm{1}+{tx}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx} \\ $$$${we}\:{have}\:{f}\left(\mathrm{2}\right)\:=−\int_{\mathrm{0}} ^{\mathrm{2}} \:\:\frac{{ln}\left(\mathrm{1}+\mathrm{2}{x}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }\:{dx}\:+\frac{{ln}\left(\mathrm{5}\right)}{\mathrm{2}}\:{arctan}\left(\mathrm{2}\right)\:+{ln}\left(\mathrm{5}\right){arctan}\left(\mathrm{2}\right) \\ $$$$=\int_{\mathrm{0}} ^{\mathrm{2}} \:\frac{{ln}\left(\mathrm{1}+\mathrm{2}{x}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}\:\Rightarrow\:\mathrm{2}\:\int_{\mathrm{0}} ^{\mathrm{2}} \:\:\frac{{ln}\left(\mathrm{1}+\mathrm{2}{x}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}\:=\frac{\mathrm{3}}{\mathrm{2}}{ln}\left(\mathrm{5}\right)\:{arctan}\left(\mathrm{2}\right)\:\:\Rightarrow \\ $$$$\int_{\mathrm{0}} ^{\mathrm{2}} \:\:\:\frac{{ln}\left(\mathrm{1}+\mathrm{2}{x}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}\:=\frac{\mathrm{3}}{\mathrm{4}}{ln}\left(\mathrm{5}\right){arctan}\left(\mathrm{2}\right)\:.{i} \\ $$
Commented by rahul 19 last updated on 29/Jan/19
$${thank}\:{you}\:{prof}\:{Abdo}. \\ $$
Commented by rahul 19 last updated on 29/Jan/19
$${Everyone}\:{pls}\:{try}\:{Q}.\mathrm{3},\mathrm{4}\:{also}. \\ $$
Commented by maxmathsup by imad last updated on 29/Jan/19
$${you}\:{are}\:{welcome}\:{sir}. \\ $$
Answered by tanmay.chaudhury50@gmail.com last updated on 29/Jan/19

$$\left.\mathrm{4}\right)\int_{\mathrm{0}} ^{\pi} \frac{\left(\mathrm{1}+{xsinx}\right)\frac{{du}}{{dx}}−{u}\frac{{d}}{{dx}}\left(\mathrm{1}+{xsinx}\right)}{\left(\mathrm{1}+{xsinx}\right)^{\mathrm{2}} }{dx} \\ $$$$\int_{\mathrm{0}} ^{\pi} \frac{{d}}{{dx}}\left(\frac{{u}}{\mathrm{1}+{xsinx}}\right){dx} \\ $$$$\int_{\mathrm{0}} ^{\pi} {d}\left(\frac{{u}}{\mathrm{1}+{xsinx}}\right) \\ $$$$\mid\frac{{u}}{\mathrm{1}+{xsinx}}\mid_{\mathrm{0}} ^{\pi} \:\:{u}={g}\left({x}\right){say} \\ $$$$=\frac{{g}\left(\pi\right)}{\mathrm{1}+\pi{sin}\pi}−\frac{{g}\left(\mathrm{0}\right)}{\mathrm{1}+\mathrm{0}×{sin}\mathrm{0}} \\ $$$$={g}\left(\pi\right)−{g}\left(\mathrm{0}\right) \\ $$$${wait}\:{i}\:{have}\:{to}\:{find}\:{g}\left({x}\right)={u}... \\ $$
