
Question and Answers Forum
Question Number 54536 by 951172235v last updated on 05/Feb/19
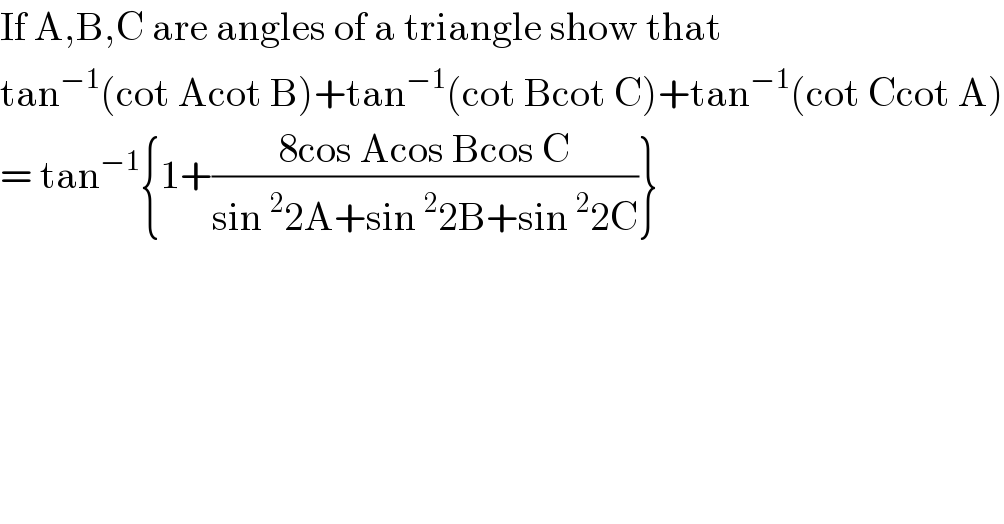
Answered by 951172235v last updated on 08/Feb/19
![A+B+C =Λ^− tan A+tan B+tan C = tan Atan Btan tan C tan α =cot Acot B tan β =cot Bcot C tan γ =cot Ccot A tan α+tan β+tan γ =1 tan (α+β+γ) =((Σtan α−tan αtan βtan γ)/(1−Σtan αtan β)) = ((1−(cot Acot Bcot C)^2 )/(1−cot Acot Bcot C(cot A+cot B+cot C))) =((tan Atan Btan C−cot Acot Bcot C)/(Σtan A−Σcot A)) = ((tan Atan Btan C−cot Acot Bcot C)/(Σ(((−cos 2A)/(sin 2A))))) = ((8(sin Asin Bsin C)^2 −8(cos Acos Bcos C)^2 )/(−Σsin 2A(sin 2Bcos 2C+sin 2Ccos 2B))) = (((1/2){(Σsin 2A)^2 −[1+Σcos 2A]^2 })/(Σsin^2 2A)) =(((1/2){2Σsin^2 A −4Σcos 2A−4})/(Σsin^2 2A)) = ((Σsin^2 2A+8cos Acos Bcos C)/(Σsin^2 2A)) =1+ ((8cos Acos Bcos C)/(Σsin^2 2A)) α+β+γ =tan^(−1) {1+((8cos Acos Bcos C)/(Σsin^2 2A))} ans.](Q54651.png)
| ||
Question and Answers Forum | ||
Question Number 54536 by 951172235v last updated on 05/Feb/19 | ||
 | ||
Answered by 951172235v last updated on 08/Feb/19 | ||
![A+B+C =Λ^− tan A+tan B+tan C = tan Atan Btan tan C tan α =cot Acot B tan β =cot Bcot C tan γ =cot Ccot A tan α+tan β+tan γ =1 tan (α+β+γ) =((Σtan α−tan αtan βtan γ)/(1−Σtan αtan β)) = ((1−(cot Acot Bcot C)^2 )/(1−cot Acot Bcot C(cot A+cot B+cot C))) =((tan Atan Btan C−cot Acot Bcot C)/(Σtan A−Σcot A)) = ((tan Atan Btan C−cot Acot Bcot C)/(Σ(((−cos 2A)/(sin 2A))))) = ((8(sin Asin Bsin C)^2 −8(cos Acos Bcos C)^2 )/(−Σsin 2A(sin 2Bcos 2C+sin 2Ccos 2B))) = (((1/2){(Σsin 2A)^2 −[1+Σcos 2A]^2 })/(Σsin^2 2A)) =(((1/2){2Σsin^2 A −4Σcos 2A−4})/(Σsin^2 2A)) = ((Σsin^2 2A+8cos Acos Bcos C)/(Σsin^2 2A)) =1+ ((8cos Acos Bcos C)/(Σsin^2 2A)) α+β+γ =tan^(−1) {1+((8cos Acos Bcos C)/(Σsin^2 2A))} ans.](Q54651.png) | ||
| ||