solve for: x 1) (√(3−x))+(√(x+1))>(1/2) 2) cos^2 x+cos^2 2x+cos^2 3x=1 3)(√(x^2 −p))+2(√(x^2 −1))=x [p∈R]


Question and Answers Forum

All Questions Topic List
Algebra Questions
Previous in All Question Next in All Question
Previous in Algebra Next in Algebra
Question Number 54600 by behi83417@gmail.com last updated on 07/Feb/19

$solve for : x$ $1) \sqrt{3 - x} + \sqrt{x + 1} > \frac{1}{2}$ $2) \cos^{2} x + \cos^{2} 2 x + \cos^{2} 3 x = 1$ $3) \sqrt{x^{2} - p} + 2 \sqrt{x^{2} - 1} = x [p \in R]$
Commented bymaxmathsup by imad last updated on 07/Feb/19

$1) w e m u s t h a v e f i r s t 3 - x ⩾ 0 a n d x + 1 ⩾ 0 \Leftrightarrow - 1 ⩽ x ⩽ 3$ $(e) \Leftrightarrow 4 + 2 \sqrt{3 - x} \sqrt{x + 1} ⩾ \frac{1}{4} \Rightarrow 2 \sqrt{3 - x} \sqrt{x + 1} ⩾ \frac{1}{4} - 4 \Rightarrow 2 \sqrt{3 - x} \sqrt{x + 1} ⩾ \frac{- 7}{4}$ $\Rightarrow \sqrt{3 - x} \sqrt{x + 1} ⩾ - \frac{7}{8} b u t t h i s i n e q u a l i t y i s t r u e f o r a l l x \in [- 1, 3] \Rightarrow$ $S = [- 1, 3] .$
Commented bymaxmathsup by imad last updated on 07/Feb/19

$2) (e) \Leftrightarrow \frac{1 + c o s (2 x)}{2} + \frac{1 + c o s (4 x)}{2} + \frac{1 + c o s (6 x)}{2} = 1 \Leftrightarrow$ $3 + c o s (2 x) + c o s (4 x) + c o s (6 x) = 2 \Rightarrow c o s (2 x) + c o s (4 x) + c o s (6 x) = - 1 \Rightarrow$ $b u t c o s a + c o s b = 2 c o s (\frac{a + b}{2}) c o s (\frac{a - b}{2}) \Rightarrow c o s (2 x) + c o s (6 x)$ $= 2 c o s (4 x) c o s (2 x) \Rightarrow 2 c o s (4 x) c o s (2 x) + c o s (4 x) = - 1 \Rightarrow$ $c o s (4 x) (2 c o s (2 x) + 1) = - 1 \Rightarrow (2 {c o s}^{2} (2 x) - 1) (2 c o s (2 x) + 1) + 1 = 0$ $\Rightarrow (2 t^{2} - 1) (2 t + 1) + 1 = 0 w i t h t = c o s (2 x) \Rightarrow$ $4 t^{3} + 2 t^{2} - 2 t - 1 + 1 = 0 \Rightarrow t (4 t^{2} + 2 t - 2) = 0 \Rightarrow 2 t (2 t^{2} + t - 1) = 0 \Rightarrow t = 0 o r$ $2 t^{2} + t - 1 = 0$ $Δ = 1 - 4 (2) (- 1) = 9 \Rightarrow t_{1} = \frac{- 1 + 3}{4} = \frac{1}{2} a n d t_{2} = - 1$ $t = 0 \Rightarrow c o s (2 x) = 0 \Rightarrow 2 x = \frac{π}{2} + k π \Rightarrow x = \frac{π}{4} + \frac{k π}{2} (k \in Z)$ $t = \frac{1}{2} \Rightarrow c o s (2 x) = c o s (\frac{π}{3}) \Rightarrow 2 x = \frac{π}{3} + 2 k π o r x = - \frac{π}{3} + 2 k π \Rightarrow$ $x = \frac{π}{6} + k π o r x = - \frac{π}{6} + k π (k f r o m Z)$ $t = - 1 \Rightarrow c o s (2 x) = c o s π \Rightarrow 2 x = (2 k + 1) π o r 2 x = (2 k - 1) π \Rightarrow$ $x = \frac{(2 k + 1) π}{2} o r x = \frac{(2 k - 1) π}{2} .$
	Answered by MJS last updated on 07/Feb/19
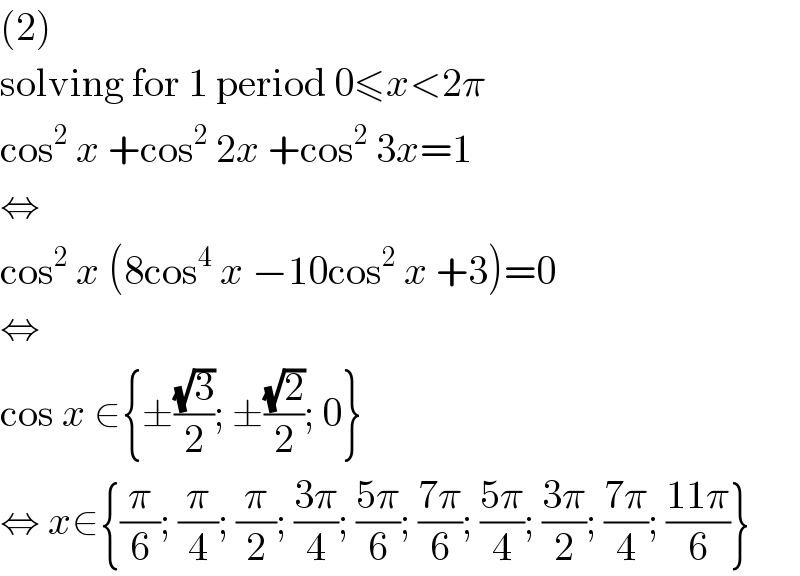
	$(2) solving for 1 period 0≤x<2π cos^2 x +cos^2 2x +cos^2 3x=1 ⇔ cos^2 x (8cos^4 x −10cos^2 x +3)=0 ⇔ cos x ∈{±((√3)/2); ±((√2)/2); 0} ⇔ x∈{(π/6); (π/4); (π/2); ((3π)/4); ((5π)/6); ((7π)/6); ((5π)/4); ((3π)/2); ((7π)/4); ((11π)/6)}$
	$(2)$ $solving for 1 period 0 ⩽ x < 2 π$ $\cos^{2} x + \cos^{2} 2 x + \cos^{2} 3 x = 1$ $\Leftrightarrow$ $\cos^{2} x ({8 \cos}^{4} x - {10 \cos}^{2} x + 3) = 0$ $\Leftrightarrow$ $\cos x \in {\pm \frac{\sqrt{3}}{2}; \pm \frac{\sqrt{2}}{2}; 0}$ $\Leftrightarrow x \in {\frac{π}{6}; \frac{π}{4}; \frac{π}{2}; \frac{3 π}{4}; \frac{5 π}{6}; \frac{7 π}{6}; \frac{5 π}{4}; \frac{3 π}{2}; \frac{7 π}{4}; \frac{11 π}{6}}$
	Answered by MJS last updated on 07/Feb/19
	$(3) squaring 4(√(x^2 −1))(√(x^2 −p))+5x^2 −p−4=x^2 4(√(x^2 −1))(√(x^2 −p))=−4x^2 +p+4 squaring again and transforming x^2 =(((p−4)^2 )/(8(2−p))) ≥0 ⇒ p∈]−∞; 2[∪{4} x=±(((p−4)(√2))/(4(√(2−p)))) but only one solution solves the original eq trying we find x=(((4−p)(√2))/(4(√(2−p)))) but only if 0≤p≤(4/3)$
	$(3)$ $squaring$ $4 \sqrt{x^{2} - 1} \sqrt{x^{2} - p} + 5 x^{2} - p - 4 = x^{2}$ $4 \sqrt{x^{2} - 1} \sqrt{x^{2} - p} = - 4 x^{2} + p + 4$ $squaring again and transforming$ $x^{2} = \frac{{(p - 4)}^{2}}{8 (2 - p)} ⩾ 0 \Rightarrow p \in] - \infty; 2 [\cup {4}$ $x = \pm \frac{(p - 4) \sqrt{2}}{4 \sqrt{2 - p}}$ $but only one solution solves the original eq$ $trying we find$ $x = \frac{(4 - p) \sqrt{2}}{4 \sqrt{2 - p}} but only if 0 ⩽ p ⩽ \frac{4}{3}$
	Commented bybehi83417@gmail.com last updated on 08/Feb/19

	$t h a n k y o u v e r y m u c h s i r A b d o a n d s i r$ $M J S . i c a n t r e c i v e n o t i f i c a t i o n s f r o m$ $a p p a n y m o r e .$
	Commented byMJS last updated on 08/Feb/19

	$me neither . at one point I received about 60$ $messages at once but now again nothing$
	Commented byrahul 19 last updated on 08/Feb/19
	$me neither :\$
	$m e n e i t h e r : ∖$
	Commented bymr W last updated on 08/Feb/19

	$m e t o o o o o!$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum