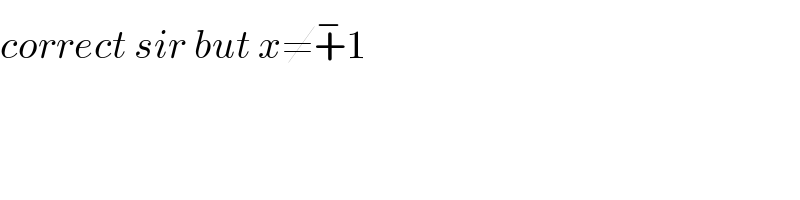let p(x)=(1+x^2 )(1+x^4 )...(1+x^2^n ) with n integr natural 1) find a simple form of p(x) 2) find roots of p(x)and decompose p(x) inside C[x] 3)calculate ∫_0 ^1 p(x)dx 4) decompose the fraction F(x)=(1/(p(x))) .


Question and Answers Forum

All Questions Topic List
Algebra Questions
Previous in All Question Next in All Question
Previous in Algebra Next in Algebra
Question Number 54808 by turbo msup by abdo last updated on 11/Feb/19
$let p(x)=(1+x^2 )(1+x^4 )...(1+x^2^n ) with n integr natural 1) find a simple form of p(x) 2) find roots of p(x)and decompose p(x) inside C[x] 3)calculate ∫_0 ^1 p(x)dx 4) decompose the fraction F(x)=(1/(p(x))) .$
$l e t p (x) = (1 + x^{2}) (1 + x^{4}) . . . (1 + x^{2^{n}})$ $w i t h n i n t e g r n a t u r a l$ $1) f i n d a s i m p l e f o r m o f p (x)$ $2) f i n d r o o t s o f p (x) a n d d e c o m p o s e$ $p (x) i n s i d e C [x]$ $3) c a l c u l a t e \int_{0}^{1} p (x) d x$ $4) d e c o m p o s e t h e f r a c t i o n$ $F (x) = \frac{1}{p (x)} .$
Commented by maxmathsup by imad last updated on 13/Feb/19

$1) w e c a n u s e r e c u r r e n c e t o p r o v e t h a t f o r x \neq \bar{+} 1$ $P (x) = \frac{1 - x^{2^{n + 1}}}{1 - x^{2}}$ $2) P (z) = 0 \Leftrightarrow 1 - z^{2^{n + 1}} = 0 \Leftrightarrow 1 - z^{q} = 0 w i t h m = 2^{n + 1} t h e r o o t s o f$ $z^{m} = 1 a r e z_{k} = e^{\frac{i 2 k π}{m}} w i t h k \in [[0, m - 1]] \Rightarrow z_{k} = e^{\frac{i 2 k π}{2^{n + 1}}} = e^{\frac{i k π}{2^{n}}}$ $w i t h k \in [[0, 2^{n + 1} - 1]] b u t b u t e l i m i n a t e v a l u e s o f k / z_{k}^{2} = 1 .$
Commented by maxmathsup by imad last updated on 13/Feb/19

$P (x) = \prod_{k = 0_{z_{k} \neq \overset{- 1}{+}}}^{2^{n + 1} - 1} (x - z_{k})$
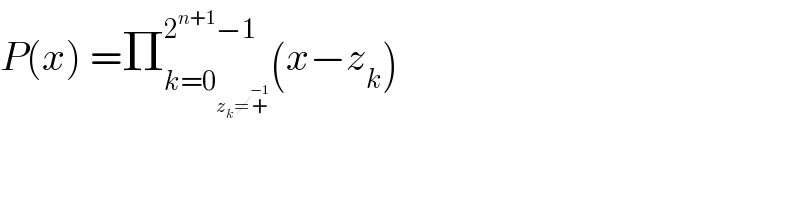
	Answered by mr W last updated on 12/Feb/19

	$1)$ $p (x) = (1 + x^{2}) (1 + x^{4}) . . . (1 + x^{2^{n}})$ $(1 - x^{2}) p (x) = (1 - x^{2}) (1 + x^{2}) (1 + x^{4}) . . . (1 + x^{2^{n}})$ $(1 - x^{2}) p (x) = (1 - x^{4}) (1 + x^{4}) . . . (1 + x^{2^{n}})$ $(1 - x^{2}) p (x) = (1 - x^{8}) . . . (1 + x^{2^{n}})$ $. . . .$ $(1 - x^{2}) p (x) = (1 - x^{2^{n}}) . . . (1 + x^{2^{n}})$ $(1 - x^{2}) p (x) = (1 - x^{2^{n + 1}})$ $\Rightarrow p (x) = \frac{1 - x^{2^{n + 1}}}{1 - x^{2}}$
	Commented by maxmathsup by imad last updated on 12/Feb/19

	$c o r r e c t s i r b u t x \neq \bar{+} 1$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum