
Question and Answers Forum
Question Number 54975 by naka3546 last updated on 15/Feb/19

Answered by mr W last updated on 15/Feb/19
![y=x^3 −2x+4 x^3 −2x+4−y=0 (u+v)^3 −3uv(u+v)−(u^3 +v^3 )=0 ley x=u+v ⇒u^3 v^3 =(8/(27)) ⇒u^3 +v^3 =y−4 z^2 −(y−4)z+(8/(27))=0 z=(1/2)[y−4±(√(y^2 −8y+((400)/(27))))] u=(((y−4+(√(y^2 −8y+((400)/(27))))))^(1/3) /(2)^(1/3) ) u=(((y−4−(√(y^2 −8y+((400)/(27))))))^(1/3) /(2)^(1/3) ) ⇒x=u+v=((((y−4+(√(y^2 −8y+((400)/(27))))))^(1/3) +((y−4−(√(y^2 −8y+((400)/(27))))))^(1/3) )/(2)^(1/3) ) ⇒f^(−1) (x)=((((x−4+(√(x^2 −8x+((400)/(27))))))^(1/3) +((x−4−(√(x^2 −8x+((400)/(27))))))^(1/3) )/(2)^(1/3) )](Q54984.png)
Answered by MJS last updated on 15/Feb/19
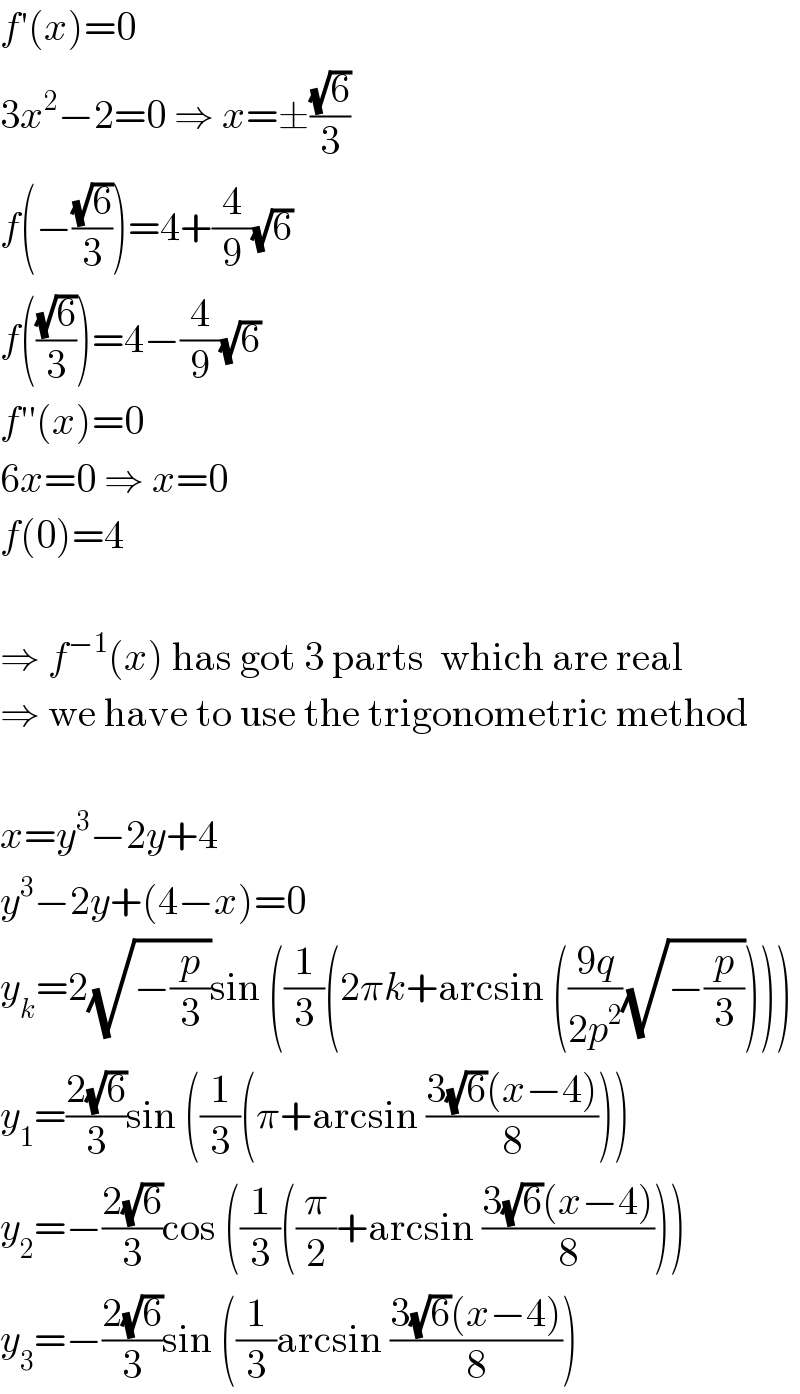
Answered by Rio Mike last updated on 16/Feb/19
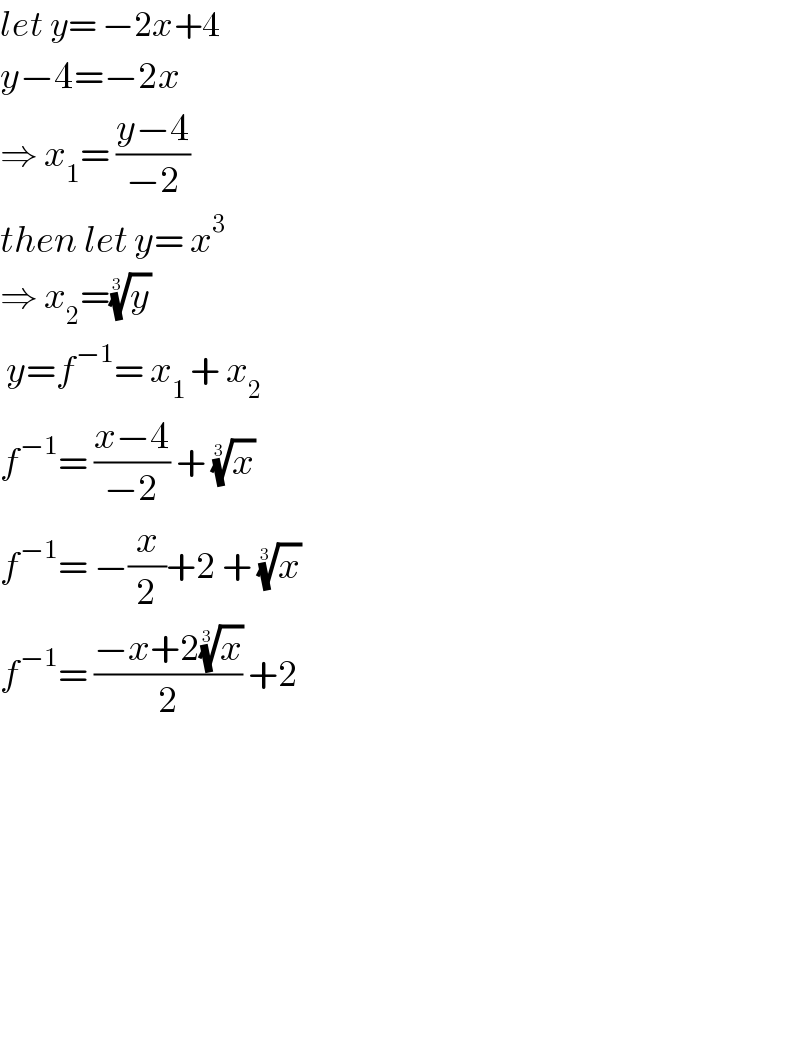
| ||
Question and Answers Forum | ||
Question Number 54975 by naka3546 last updated on 15/Feb/19 | ||
 | ||
Answered by mr W last updated on 15/Feb/19 | ||
![y=x^3 −2x+4 x^3 −2x+4−y=0 (u+v)^3 −3uv(u+v)−(u^3 +v^3 )=0 ley x=u+v ⇒u^3 v^3 =(8/(27)) ⇒u^3 +v^3 =y−4 z^2 −(y−4)z+(8/(27))=0 z=(1/2)[y−4±(√(y^2 −8y+((400)/(27))))] u=(((y−4+(√(y^2 −8y+((400)/(27))))))^(1/3) /(2)^(1/3) ) u=(((y−4−(√(y^2 −8y+((400)/(27))))))^(1/3) /(2)^(1/3) ) ⇒x=u+v=((((y−4+(√(y^2 −8y+((400)/(27))))))^(1/3) +((y−4−(√(y^2 −8y+((400)/(27))))))^(1/3) )/(2)^(1/3) ) ⇒f^(−1) (x)=((((x−4+(√(x^2 −8x+((400)/(27))))))^(1/3) +((x−4−(√(x^2 −8x+((400)/(27))))))^(1/3) )/(2)^(1/3) )](Q54984.png) | ||
| ||
Answered by MJS last updated on 15/Feb/19 | ||
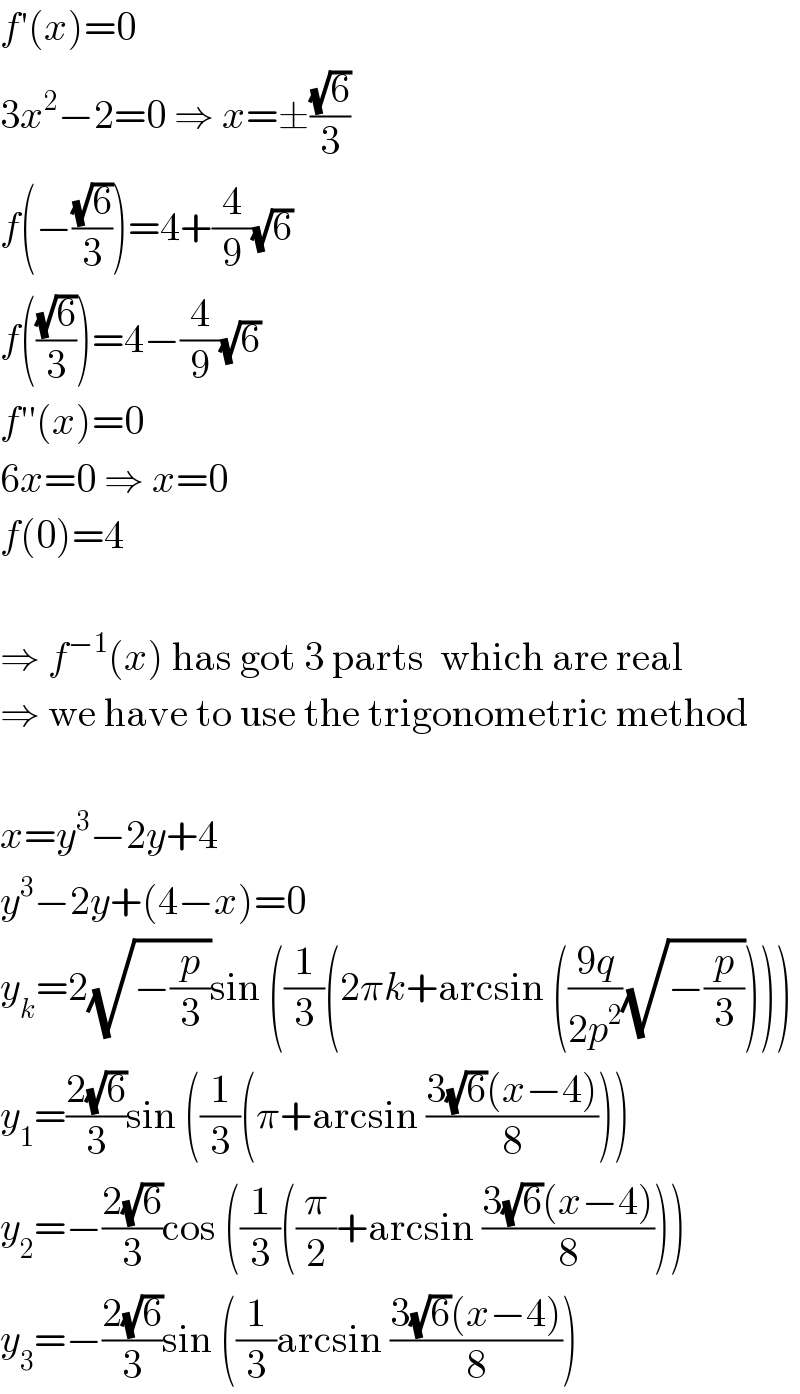 | ||
| ||
Answered by Rio Mike last updated on 16/Feb/19 | ||
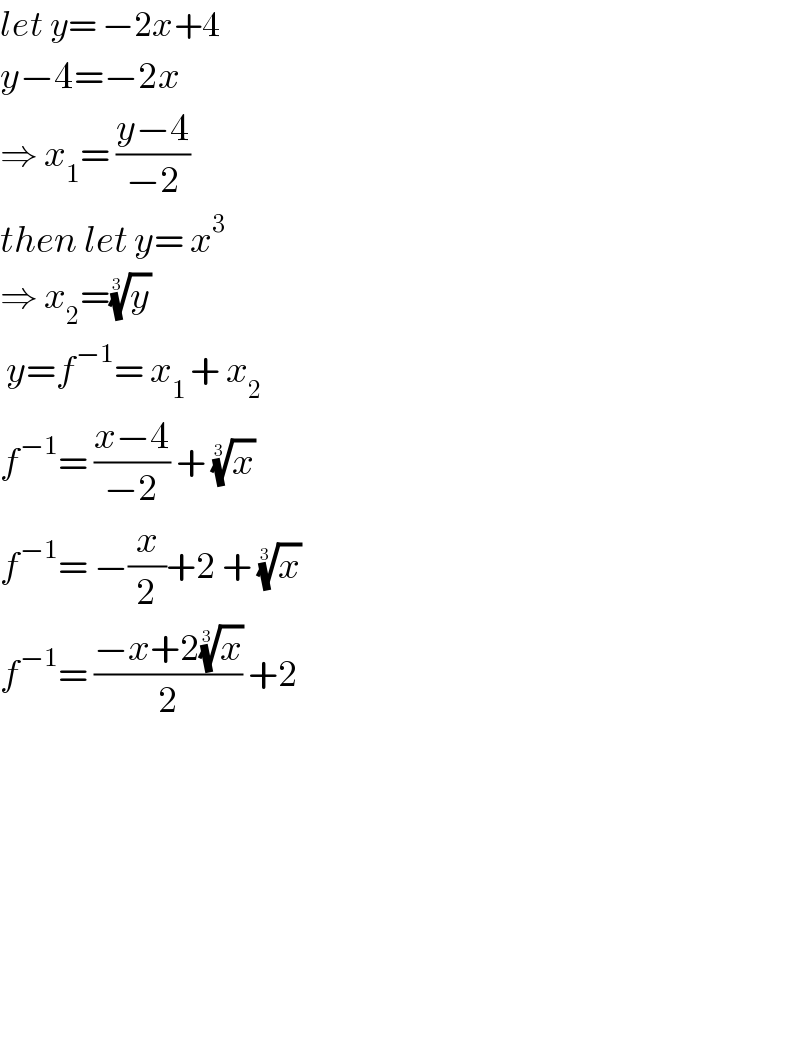 | ||
| ||