Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 55520 by Rdk96 last updated on 26/Feb/19

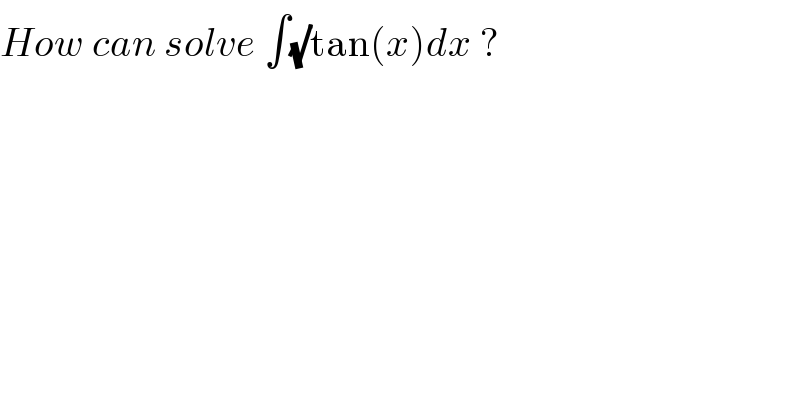
How can solve ∫(√)tan(x)dx ?

$${How}\:{can}\:{solve}\:\int\sqrt{}\mathrm{tan}\left({x}\right){dx}\:? \\ $$

Commented by maxmathsup by imad last updated on 26/Feb/19

let A =∫ (√(tanx))dx changement (√(tanx))=t give tanx =t^2 ⇒x =arctan(t^2 ) ⇒ A =∫ t ((2t)/(1+t^4 )) dt =2 ∫ (t^2 /(t^4 +1))dt let decompose F(t)=(t^2 /(t^4 +1)) ⇒ F(t)=(t^2 /((t^2 −(√2)t +1)(t^2 +(√2)t +1))) =((at +b)/(t^2 −(√2)t +1)) +((ct +d)/(t^2 +(√2)t +1)) F(−t)=F(t) ⇒((−at +b)/(t^2 +(√2)t +1))+((−ct +d)/(t^2 −(√2)t +1)) =F(t) ⇒c=−a and d=b ⇒ F(t)=((at +b)/(t^2 −(√2)t+1)) +((−at +b)/(t^2 +(√2)t +1)) F(0) =0 =2b ⇒b=0 ⇒F(t)=((at)/(t^2 −(√2)t +1)) −((at)/(t^2 +(√2)t +1)) F(1) =(1/((2−(√2))(2+(√2)))) = (a/(2−(√2))) −(a/(2+(√2))) ⇒(1/2) =(((2+(√2))a−(2−(√2))a)/2) ⇒ 2(√2)a =1 ⇒a =(1/(2(√2))) ⇒F(t)=(1/(2(√2))){ (t/(t^2 −(√2)t +1)) −(t/(t^2 +(√2)t +1))} ⇒ A =(1/(√2)){ ∫ ((tdt)/(t^2 −(√2)t +1)) −∫ ((tdt)/(t^2 +(√2)t +1))} +c but ∫ ((tdt)/(t^2 −(√2)t +1)) =(1/2) ∫ ((2t−(√2)+(√2))/(t^2 −(√2)t +1)) dt =(1/2)ln(t^2 −(√2)t +1)+(1/(√2))∫ (dt/(t^2 −(√2)t +1)) ∫ (dt/(t^2 −(√2)t +1)) =∫ (dt/((t−((√2)/2))^2 +(1/2))) =_(t−(1/(√2))=(u/(√2))) ∫ (du/((√2)(1/2)(1+u^2 ))) =(√2)arctan(t(√2)−1) ⇒∫ ((tdt)/(t^2 −(√2)t +1)) =(1/2)ln(t^2 −(√2)t +1) +arctan(t(√2)−1) also we get ∫ ((tdt)/(t^2 +(√2)t +1)) =(1/2)ln(t^2 +(√2)t +1) +arctan(t(√2)+1) ⇒ (√2)A =(1/2)ln(t^2 −(√2)t +1)+arctan(t(√2)−1)−(1/2)ln(t^2 +(√2)t +1)−arctan(t(√2)+1) +c =ln(√((t^2 −(√2)t+1)/(t^2 +(√2)t +1))) +arctan(t(√2)−1)−arctan(t(√2)+1) +c ⇒ A =(1/(√2)){ ln((√((tanx−(√(2tanx))+1)/(tanx+(√(2tanx))+1)))) +arctan((√(2tanx))−1)−arctan((√(2tanx)) +1)} +c

$${let}\:{A}\:=\int\:\sqrt{{tanx}}{dx}\:\:{changement}\:\sqrt{{tanx}}={t}\:{give}\:{tanx}\:={t}^{\mathrm{2}} \:\Rightarrow{x}\:={arctan}\left({t}^{\mathrm{2}} \right)\:\Rightarrow \\ $$$${A}\:=\int\:{t}\:\frac{\mathrm{2}{t}}{\mathrm{1}+{t}^{\mathrm{4}} }\:{dt}\:=\mathrm{2}\:\int\:\:\frac{{t}^{\mathrm{2}} }{{t}^{\mathrm{4}} +\mathrm{1}}{dt}\:\:{let}\:{decompose}\:{F}\left({t}\right)=\frac{{t}^{\mathrm{2}} }{{t}^{\mathrm{4}} \:+\mathrm{1}}\:\Rightarrow \\ $$$${F}\left({t}\right)=\frac{{t}^{\mathrm{2}} }{\left({t}^{\mathrm{2}} \:−\sqrt{\mathrm{2}}{t}\:+\mathrm{1}\right)\left({t}^{\mathrm{2}} \:+\sqrt{\mathrm{2}}{t}\:+\mathrm{1}\right)}\:=\frac{{at}\:+{b}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}\:+\mathrm{1}}\:+\frac{{ct}\:+{d}}{{t}^{\mathrm{2}} \:+\sqrt{\mathrm{2}}{t}\:+\mathrm{1}} \\ $$$${F}\left(−{t}\right)={F}\left({t}\right)\:\Rightarrow\frac{−{at}\:+{b}}{{t}^{\mathrm{2}} +\sqrt{\mathrm{2}}{t}\:+\mathrm{1}}+\frac{−{ct}\:+{d}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}\:+\mathrm{1}}\:={F}\left({t}\right)\:\Rightarrow{c}=−{a}\:{and}\:{d}={b}\:\Rightarrow \\ $$$${F}\left({t}\right)=\frac{{at}\:+{b}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}+\mathrm{1}}\:+\frac{−{at}\:+{b}}{{t}^{\mathrm{2}} \:+\sqrt{\mathrm{2}}{t}\:+\mathrm{1}} \\ $$$${F}\left(\mathrm{0}\right)\:=\mathrm{0}\:=\mathrm{2}{b}\:\Rightarrow{b}=\mathrm{0}\:\Rightarrow{F}\left({t}\right)=\frac{{at}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}\:+\mathrm{1}}\:−\frac{{at}}{{t}^{\mathrm{2}} +\sqrt{\mathrm{2}}{t}\:+\mathrm{1}} \\ $$$${F}\left(\mathrm{1}\right)\:=\frac{\mathrm{1}}{\left(\mathrm{2}−\sqrt{\mathrm{2}}\right)\left(\mathrm{2}+\sqrt{\mathrm{2}}\right)}\:=\:\frac{{a}}{\mathrm{2}−\sqrt{\mathrm{2}}}\:−\frac{{a}}{\mathrm{2}+\sqrt{\mathrm{2}}}\:\Rightarrow\frac{\mathrm{1}}{\mathrm{2}}\:=\frac{\left(\mathrm{2}+\sqrt{\mathrm{2}}\right){a}−\left(\mathrm{2}−\sqrt{\mathrm{2}}\right){a}}{\mathrm{2}}\:\Rightarrow \\ $$$$\mathrm{2}\sqrt{\mathrm{2}}{a}\:=\mathrm{1}\:\Rightarrow{a}\:=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}\:\Rightarrow{F}\left({t}\right)=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}\left\{\:\frac{{t}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}\:+\mathrm{1}}\:−\frac{{t}}{{t}^{\mathrm{2}} \:+\sqrt{\mathrm{2}}{t}\:+\mathrm{1}}\right\}\:\Rightarrow \\ $$$${A}\:=\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\left\{\:\int\:\:\:\frac{{tdt}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}\:+\mathrm{1}}\:−\int\:\:\:\frac{{tdt}}{{t}^{\mathrm{2}} +\sqrt{\mathrm{2}}{t}\:+\mathrm{1}}\right\}\:+{c}\:\:{but}\: \\ $$$$\int\:\:\:\frac{{tdt}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}\:+\mathrm{1}}\:=\frac{\mathrm{1}}{\mathrm{2}}\:\int\:\:\frac{\mathrm{2}{t}−\sqrt{\mathrm{2}}+\sqrt{\mathrm{2}}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}\:+\mathrm{1}}\:{dt}\:=\frac{\mathrm{1}}{\mathrm{2}}{ln}\left({t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}\:+\mathrm{1}\right)+\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\int\:\:\frac{{dt}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}\:+\mathrm{1}} \\ $$$$\int\:\:\frac{{dt}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}\:+\mathrm{1}}\:=\int\:\:\frac{{dt}}{\left({t}−\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\right)^{\mathrm{2}} \:+\frac{\mathrm{1}}{\mathrm{2}}}\:=_{{t}−\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}=\frac{{u}}{\sqrt{\mathrm{2}}}} \:\:\:\:\int\:\:\:\:\:\frac{{du}}{\sqrt{\mathrm{2}}\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{1}+{u}^{\mathrm{2}} \right)} \\ $$$$=\sqrt{\mathrm{2}}{arctan}\left({t}\sqrt{\mathrm{2}}−\mathrm{1}\right)\:\Rightarrow\int\:\:\frac{{tdt}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}\:+\mathrm{1}}\:=\frac{\mathrm{1}}{\mathrm{2}}{ln}\left({t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}\:+\mathrm{1}\right)\:+{arctan}\left({t}\sqrt{\mathrm{2}}−\mathrm{1}\right) \\ $$$${also}\:{we}\:{get}\:\:\int\:\:\frac{{tdt}}{{t}^{\mathrm{2}} +\sqrt{\mathrm{2}}{t}\:+\mathrm{1}}\:=\frac{\mathrm{1}}{\mathrm{2}}{ln}\left({t}^{\mathrm{2}} \:+\sqrt{\mathrm{2}}{t}\:+\mathrm{1}\right)\:+{arctan}\left({t}\sqrt{\mathrm{2}}+\mathrm{1}\right)\:\Rightarrow \\ $$$$\sqrt{\mathrm{2}}{A}\:=\frac{\mathrm{1}}{\mathrm{2}}{ln}\left({t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}\:+\mathrm{1}\right)+{arctan}\left({t}\sqrt{\mathrm{2}}−\mathrm{1}\right)−\frac{\mathrm{1}}{\mathrm{2}}{ln}\left({t}^{\mathrm{2}} \:+\sqrt{\mathrm{2}}{t}\:+\mathrm{1}\right)−{arctan}\left({t}\sqrt{\mathrm{2}}+\mathrm{1}\right)\:+{c} \\ $$$$={ln}\sqrt{\frac{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}+\mathrm{1}}{{t}^{\mathrm{2}} \:+\sqrt{\mathrm{2}}{t}\:+\mathrm{1}}}\:\:+{arctan}\left({t}\sqrt{\mathrm{2}}−\mathrm{1}\right)−{arctan}\left({t}\sqrt{\mathrm{2}}+\mathrm{1}\right)\:+{c}\:\Rightarrow \\ $$$${A}\:=\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\left\{\:{ln}\left(\sqrt{\frac{{tanx}−\sqrt{\mathrm{2}{tanx}}+\mathrm{1}}{{tanx}+\sqrt{\mathrm{2}{tanx}}+\mathrm{1}}}\right)\:+{arctan}\left(\sqrt{\mathrm{2}{tanx}}−\mathrm{1}\right)−{arctan}\left(\sqrt{\mathrm{2}{tanx}}\:+\mathrm{1}\right)\right\}\:+{c} \\ $$$$ \\ $$$$ \\ $$

Commented by maxmathsup by imad last updated on 26/Feb/19

∫ ((tdt)/(t^2 +(√2)t +1)) =_(t=−u) ∫ (((−u)(−du))/(u^2 −(√2)u +1)) =∫ ((udu)/(u^2 −(√2)u +1)) =(1/2)ln(u^2 −(√2)u +1) +arctan(u(√2)−1) =(1/2)ln(t^2 +(√2)t +1)−arctan(t(√2)+1) ⇒ (√2)A =(1/2)ln(t^2 −(√2)t +1)+arctan(t(√2)−1)−(1/2)ln(t^2 +(√2)t +1) +arctan(t(√2)+1) A =(1/(√2)){ ln((√((tanx−2(√(tanx))+1)/(tanx +(√(2tanx))+1)))) +arctan(t(√2)−1) +arctan(t(√2)+1)} +c .

$$\int\:\:\frac{{tdt}}{{t}^{\mathrm{2}} +\sqrt{\mathrm{2}}{t}\:+\mathrm{1}}\:=_{{t}=−{u}} \:\:\:\int\:\:\frac{\left(−{u}\right)\left(−{du}\right)}{{u}^{\mathrm{2}} −\sqrt{\mathrm{2}}{u}\:+\mathrm{1}}\:=\int\:\frac{{udu}}{{u}^{\mathrm{2}} −\sqrt{\mathrm{2}}{u}\:+\mathrm{1}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}{ln}\left({u}^{\mathrm{2}} −\sqrt{\mathrm{2}}{u}\:+\mathrm{1}\right)\:+{arctan}\left({u}\sqrt{\mathrm{2}}−\mathrm{1}\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}{ln}\left({t}^{\mathrm{2}} +\sqrt{\mathrm{2}}{t}\:+\mathrm{1}\right)−{arctan}\left({t}\sqrt{\mathrm{2}}+\mathrm{1}\right)\:\Rightarrow \\ $$$$\sqrt{\mathrm{2}}{A}\:=\frac{\mathrm{1}}{\mathrm{2}}{ln}\left({t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}\:+\mathrm{1}\right)+{arctan}\left({t}\sqrt{\mathrm{2}}−\mathrm{1}\right)−\frac{\mathrm{1}}{\mathrm{2}}{ln}\left({t}^{\mathrm{2}} \:+\sqrt{\mathrm{2}}{t}\:+\mathrm{1}\right)\:+{arctan}\left({t}\sqrt{\mathrm{2}}+\mathrm{1}\right) \\ $$$${A}\:=\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\left\{\:{ln}\left(\sqrt{\frac{{tanx}−\mathrm{2}\sqrt{{tanx}}+\mathrm{1}}{{tanx}\:+\sqrt{\mathrm{2}{tanx}}+\mathrm{1}}}\right)\:+{arctan}\left({t}\sqrt{\mathrm{2}}−\mathrm{1}\right)\:+{arctan}\left({t}\sqrt{\mathrm{2}}+\mathrm{1}\right)\right\}\:+{c}\:. \\ $$

Answered by tanmay.chaudhury50@gmail.com last updated on 26/Feb/19

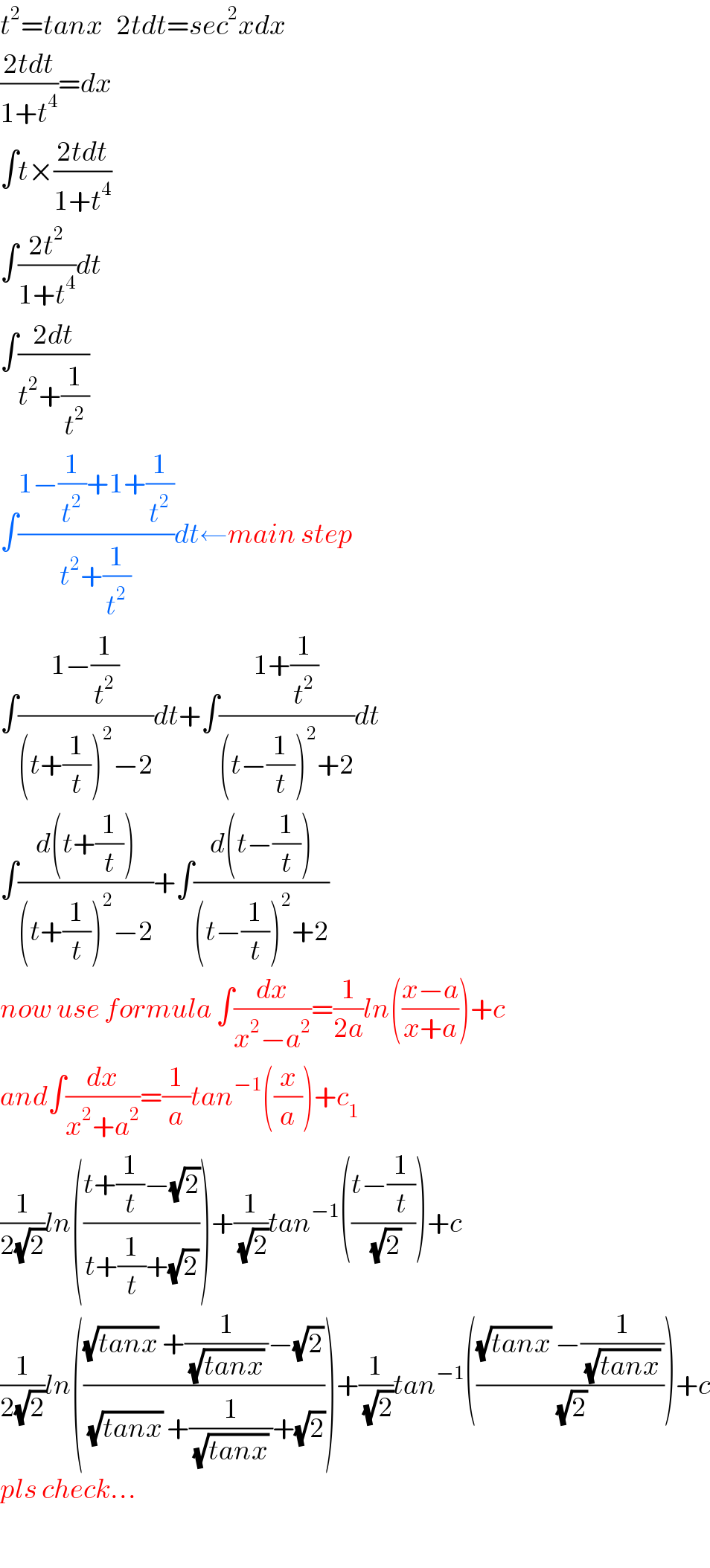
t^2 =tanx 2tdt=sec^2 xdx ((2tdt)/(1+t^4 ))=dx ∫t×((2tdt)/(1+t^4 )) ∫((2t^2 )/(1+t^4 ))dt ∫((2dt)/(t^2 +(1/t^2 ))) ∫((1−(1/t^2 )+1+(1/t^2 ))/(t^2 +(1/t^2 )))dt←main step ∫((1−(1/t^2 ))/((t+(1/t))^2 −2))dt+∫((1+(1/t^2 ))/((t−(1/t))^2 +2))dt ∫((d(t+(1/t)))/((t+(1/t))^2 −2))+∫((d(t−(1/t)))/((t−(1/t))^2 +2)) now use formula ∫(dx/(x^2 −a^2 ))=(1/(2a))ln(((x−a)/(x+a)))+c and∫(dx/(x^2 +a^2 ))=(1/a)tan^(−1) ((x/a))+c_1 (1/(2(√2)))ln(((t+(1/t)−(√2))/(t+(1/t)+(√2))))+(1/(√2))tan^(−1) (((t−(1/t))/(√2)))+c (1/(2(√2)))ln((((√(tanx)) +(1/((√(tanx)) ))−(√2))/((√(tanx)) +(1/((√(tanx)) ))+(√2))))+(1/(√2))tan^(−1) ((((√(tanx)) −(1/((√(tanx)) )))/(√2)))+c pls check...

$${t}^{\mathrm{2}} ={tanx}\:\:\:\mathrm{2}{tdt}={sec}^{\mathrm{2}} {xdx} \\ $$$$\frac{\mathrm{2}{tdt}}{\mathrm{1}+{t}^{\mathrm{4}} }={dx} \\ $$$$\int{t}×\frac{\mathrm{2}{tdt}}{\mathrm{1}+{t}^{\mathrm{4}} } \\ $$$$\int\frac{\mathrm{2}{t}^{\mathrm{2}} }{\mathrm{1}+{t}^{\mathrm{4}} }{dt} \\ $$$$\int\frac{\mathrm{2}{dt}}{{t}^{\mathrm{2}} +\frac{\mathrm{1}}{{t}^{\mathrm{2}} }} \\ $$$$\int\frac{\mathrm{1}−\frac{\mathrm{1}}{{t}^{\mathrm{2}} }+\mathrm{1}+\frac{\mathrm{1}}{{t}^{\mathrm{2}} }}{{t}^{\mathrm{2}} +\frac{\mathrm{1}}{{t}^{\mathrm{2}} }}{dt}\leftarrow{main}\:{step} \\ $$$$\int\frac{\mathrm{1}−\frac{\mathrm{1}}{{t}^{\mathrm{2}} }}{\left({t}+\frac{\mathrm{1}}{{t}}\right)^{\mathrm{2}} −\mathrm{2}}{dt}+\int\frac{\mathrm{1}+\frac{\mathrm{1}}{{t}^{\mathrm{2}} }}{\left({t}−\frac{\mathrm{1}}{{t}}\right)^{\mathrm{2}} +\mathrm{2}}{dt} \\ $$$$\int\frac{{d}\left({t}+\frac{\mathrm{1}}{{t}}\right)}{\left({t}+\frac{\mathrm{1}}{{t}}\right)^{\mathrm{2}} −\mathrm{2}}+\int\frac{{d}\left({t}−\frac{\mathrm{1}}{{t}}\right)}{\left({t}−\frac{\mathrm{1}}{{t}}\right)^{\mathrm{2}} +\mathrm{2}} \\ $$$${now}\:{use}\:{formula}\:\int\frac{{dx}}{{x}^{\mathrm{2}} −{a}^{\mathrm{2}} }=\frac{\mathrm{1}}{\mathrm{2}{a}}{ln}\left(\frac{{x}−{a}}{{x}+{a}}\right)+{c} \\ $$$${and}\int\frac{{dx}}{{x}^{\mathrm{2}} +{a}^{\mathrm{2}} }=\frac{\mathrm{1}}{{a}}{tan}^{−\mathrm{1}} \left(\frac{{x}}{{a}}\right)+{c}_{\mathrm{1}} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}{ln}\left(\frac{{t}+\frac{\mathrm{1}}{{t}}−\sqrt{\mathrm{2}}}{{t}+\frac{\mathrm{1}}{{t}}+\sqrt{\mathrm{2}}}\right)+\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}{tan}^{−\mathrm{1}} \left(\frac{{t}−\frac{\mathrm{1}}{{t}}}{\sqrt{\mathrm{2}}}\right)+{c} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}{ln}\left(\frac{\sqrt{{tanx}}\:+\frac{\mathrm{1}}{\sqrt{{tanx}}\:}−\sqrt{\mathrm{2}}}{\sqrt{{tanx}}\:+\frac{\mathrm{1}}{\sqrt{{tanx}}\:}+\sqrt{\mathrm{2}}}\right)+\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}{tan}^{−\mathrm{1}} \left(\frac{\sqrt{{tanx}}\:−\frac{\mathrm{1}}{\sqrt{{tanx}}\:}}{\sqrt{\mathrm{2}}}\right)+{c} \\ $$$${pls}\:{check}... \\ $$$$ \\ $$

Commented by Rdk96 last updated on 26/Feb/19

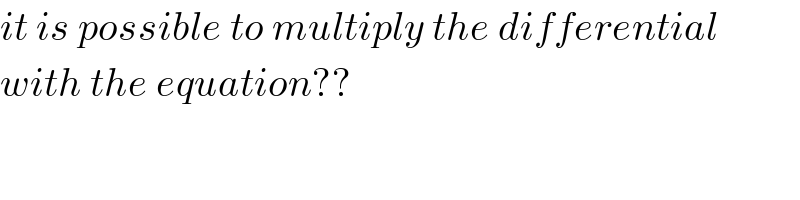
it is possible to multiply the differential with the equation??

$${it}\:{is}\:{possible}\:{to}\:{multiply}\:{the}\:{differential}\: \\ $$$${with}\:{the}\:{equation}?? \\ $$$$ \\ $$

Commented by tanmay.chaudhury50@gmail.com last updated on 26/Feb/19

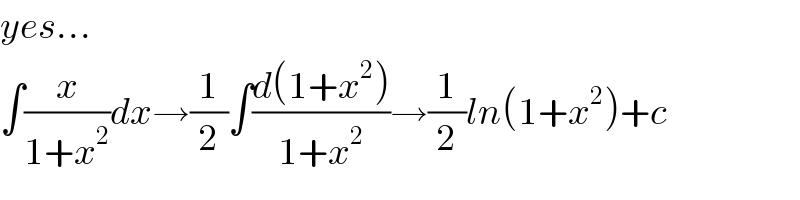
yes... ∫(x/(1+x^2 ))dx→(1/2)∫((d(1+x^2 ))/(1+x^2 ))→(1/2)ln(1+x^2 )+c

$${yes}... \\ $$$$\int\frac{{x}}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}\rightarrow\frac{\mathrm{1}}{\mathrm{2}}\int\frac{{d}\left(\mathrm{1}+{x}^{\mathrm{2}} \right)}{\mathrm{1}+{x}^{\mathrm{2}} }\rightarrow\frac{\mathrm{1}}{\mathrm{2}}{ln}\left(\mathrm{1}+{x}^{\mathrm{2}} \right)+{c} \\ $$

Commented by Rdk96 last updated on 26/Feb/19

correct.

$${correct}. \\ $$

Answered by tanmay.chaudhury50@gmail.com last updated on 26/Feb/19

or method... p=∫(√(tanx)) +(√(cotx)) dx q=(√(tanx)) −(√(cotx)) dx ∫(√(tanx)) dx=((p+q)/2) p=∫(√((sinx)/(cosx))) +(√((cosx)/(sinx))) dx p=∫((sinx+cosx)/(√(sinxcosx)))dx p=(√2)∫((d(sinx−cosx))/(√(1−1+2sinxcosx)))dx =(√2) ∫((d(sinx−cosx))/((√(1−(sinx−cosx)^2 )) )) =(√2) sin^(−1) (sinx−cosx) +c_1 q=∫((√((sinx)/(cosx))) −(√((cosx)/(sinx))) ) dx q=∫((sinx−cosx)/(√(sinxcosx)))dx→−∫((cosx−sinx)/(√(sinxcosx)))dx q=(−(√2) )∫((d(sinx+cosx))/(√(1+2sinxcosx−1))) =(−(√2) )∫((d(sinx+cosx))/(√((sinx+cosx)^2 −1))) =(−(√2) )ln{(sinx+cosx)+(√((sinx+cosx)^2 −1)) } so ∫(√(tanx)) dx ((√2)/2)[sin^(−1) (sinx−cosx)−ln{(sinx+cosx)+(√((sinx+cosx)^2 −1)) }]+c pls check...

$${or}\:{method}... \\ $$$${p}=\int\sqrt{{tanx}}\:+\sqrt{{cotx}}\:{dx} \\ $$$${q}=\sqrt{{tanx}}\:−\sqrt{{cotx}}\:{dx} \\ $$$$\int\sqrt{{tanx}}\:{dx}=\frac{{p}+{q}}{\mathrm{2}} \\ $$$${p}=\int\sqrt{\frac{{sinx}}{{cosx}}}\:+\sqrt{\frac{{cosx}}{{sinx}}}\:{dx} \\ $$$${p}=\int\frac{{sinx}+{cosx}}{\sqrt{{sinxcosx}}}{dx} \\ $$$${p}=\sqrt{\mathrm{2}}\int\frac{{d}\left({sinx}−{cosx}\right)}{\sqrt{\mathrm{1}−\mathrm{1}+\mathrm{2}{sinxcosx}}}{dx} \\ $$$$=\sqrt{\mathrm{2}}\:\int\frac{{d}\left({sinx}−{cosx}\right)}{\sqrt{\mathrm{1}−\left({sinx}−{cosx}\right)^{\mathrm{2}} }\:} \\ $$$$=\sqrt{\mathrm{2}}\:{sin}^{−\mathrm{1}} \left({sinx}−{cosx}\right)\:+{c}_{\mathrm{1}} \\ $$$${q}=\int\left(\sqrt{\frac{{sinx}}{{cosx}}}\:−\sqrt{\frac{{cosx}}{{sinx}}}\:\right)\:{dx} \\ $$$${q}=\int\frac{{sinx}−{cosx}}{\sqrt{{sinxcosx}}}{dx}\rightarrow−\int\frac{{cosx}−{sinx}}{\sqrt{{sinxcosx}}}{dx} \\ $$$${q}=\left(−\sqrt{\mathrm{2}}\:\right)\int\frac{{d}\left({sinx}+{cosx}\right)}{\sqrt{\mathrm{1}+\mathrm{2}{sinxcosx}−\mathrm{1}}} \\ $$$$=\left(−\sqrt{\mathrm{2}}\:\right)\int\frac{{d}\left({sinx}+{cosx}\right)}{\sqrt{\left({sinx}+{cosx}\right)^{\mathrm{2}} −\mathrm{1}}} \\ $$$$=\left(−\sqrt{\mathrm{2}}\:\right){ln}\left\{\left({sinx}+{cosx}\right)+\sqrt{\left({sinx}+{cosx}\right)^{\mathrm{2}} −\mathrm{1}}\:\right\} \\ $$$$ \\ $$$${so}\:\int\sqrt{{tanx}}\:{dx} \\ $$$$\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\left[{sin}^{−\mathrm{1}} \left({sinx}−{cosx}\right)−{ln}\left\{\left({sinx}+{cosx}\right)+\sqrt{\left({sinx}+{cosx}\right)^{\mathrm{2}} −\mathrm{1}}\:\right\}\right]+{c} \\ $$$${pls}\:{check}... \\ $$$$ \\ $$

Answered by MJS last updated on 26/Feb/19

∫(√(tan x))dx= [t=(√(tan x)) → dx=2cos^2 x (√(tan x))dt=((2tdt)/(t^4 +1))] =2∫(t^2 /(t^4 +1))dt=2∫(t^2 /((t^2 −(√2)t+1)(t^2 +(√2)t+1)))dt= =2∫((((√2)t)/(4(t^2 −(√2)t+1)))−(((√2)t)/(4(t^2 +(√2)t+1))))dt= =(1/2)∫((((√2)t−1)/(t^2 −(√2)t+1))+(1/(t^2 −(√2)t+1))−(((√2)t+1)/(t^2 +(√2)t+1))+(1/(t^2 +(√2)t+1)))dt= =((√2)/4)∫(((2t−(√2))/(t^2 −(√2)t+1))−((2t+(√2))/(t^2 +(√2)t+1)))dt+(1/2)∫((1/(t^2 −(√2)t+1))+(1/(t^2 +(√2)t+1)))dt= =((√2)/4)(ln (t^2 −(√2)t+1) −ln (t^2 +(√2)t+1))+((√2)/2)(arctan ((√2)t−1) +arctan ((√2)t+1))= =((√2)/4)(ln ∣((tan x −(√(2tan x))+1)/(tan x +(√(2tan x))+1))∣ +2(arctan ((√(2tan x))−1) +arctan ((√(2tan x))+1)))+C

$$\int\sqrt{\mathrm{tan}\:{x}}{dx}= \\ $$$$\:\:\:\:\:\left[{t}=\sqrt{\mathrm{tan}\:{x}}\:\rightarrow\:{dx}=\mathrm{2cos}^{\mathrm{2}} \:{x}\:\sqrt{\mathrm{tan}\:{x}}{dt}=\frac{\mathrm{2}{tdt}}{{t}^{\mathrm{4}} +\mathrm{1}}\right] \\ $$$$=\mathrm{2}\int\frac{{t}^{\mathrm{2}} }{{t}^{\mathrm{4}} +\mathrm{1}}{dt}=\mathrm{2}\int\frac{{t}^{\mathrm{2}} }{\left({t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}+\mathrm{1}\right)\left({t}^{\mathrm{2}} +\sqrt{\mathrm{2}}{t}+\mathrm{1}\right)}{dt}= \\ $$$$=\mathrm{2}\int\left(\frac{\sqrt{\mathrm{2}}{t}}{\mathrm{4}\left({t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}+\mathrm{1}\right)}−\frac{\sqrt{\mathrm{2}}{t}}{\mathrm{4}\left({t}^{\mathrm{2}} +\sqrt{\mathrm{2}}{t}+\mathrm{1}\right)}\right){dt}= \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int\left(\frac{\sqrt{\mathrm{2}}{t}−\mathrm{1}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}+\mathrm{1}}+\frac{\mathrm{1}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}+\mathrm{1}}−\frac{\sqrt{\mathrm{2}}{t}+\mathrm{1}}{{t}^{\mathrm{2}} +\sqrt{\mathrm{2}}{t}+\mathrm{1}}+\frac{\mathrm{1}}{{t}^{\mathrm{2}} +\sqrt{\mathrm{2}}{t}+\mathrm{1}}\right){dt}= \\ $$$$=\frac{\sqrt{\mathrm{2}}}{\mathrm{4}}\int\left(\frac{\mathrm{2}{t}−\sqrt{\mathrm{2}}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}+\mathrm{1}}−\frac{\mathrm{2}{t}+\sqrt{\mathrm{2}}}{{t}^{\mathrm{2}} +\sqrt{\mathrm{2}}{t}+\mathrm{1}}\right){dt}+\frac{\mathrm{1}}{\mathrm{2}}\int\left(\frac{\mathrm{1}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}+\mathrm{1}}+\frac{\mathrm{1}}{{t}^{\mathrm{2}} +\sqrt{\mathrm{2}}{t}+\mathrm{1}}\right){dt}= \\ $$$$=\frac{\sqrt{\mathrm{2}}}{\mathrm{4}}\left(\mathrm{ln}\:\left({t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}+\mathrm{1}\right)\:−\mathrm{ln}\:\left({t}^{\mathrm{2}} +\sqrt{\mathrm{2}}{t}+\mathrm{1}\right)\right)+\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\left(\mathrm{arctan}\:\left(\sqrt{\mathrm{2}}{t}−\mathrm{1}\right)\:+\mathrm{arctan}\:\left(\sqrt{\mathrm{2}}{t}+\mathrm{1}\right)\right)= \\ $$$$=\frac{\sqrt{\mathrm{2}}}{\mathrm{4}}\left(\mathrm{ln}\:\mid\frac{\mathrm{tan}\:{x}\:−\sqrt{\mathrm{2tan}\:{x}}+\mathrm{1}}{\mathrm{tan}\:{x}\:+\sqrt{\mathrm{2tan}\:{x}}+\mathrm{1}}\mid\:+\mathrm{2}\left(\mathrm{arctan}\:\left(\sqrt{\mathrm{2tan}\:{x}}−\mathrm{1}\right)\:+\mathrm{arctan}\:\left(\sqrt{\mathrm{2tan}\:{x}}+\mathrm{1}\right)\right)\right)+{C} \\ $$

Commented by behi83417@gmail.com last updated on 26/Feb/19

arctg((√(2tgx))−1)+arctg((√(2tgx))+1)= arctg((2(√(2tgx)))/(2(1−tgx)))=arctg(((√(2tgx))/(1−tgx)))= arccos∣cosx−sinx∣ ln((tgx−(√(2tgx))+1)/(tgx+(√(2tgx))+1))=ln(((tgx+1−(√(2tgx)))^2 )/(tg^2 x+1))= =2ln[cosx.(tgx+1−(√(2tgx))]= =2ln[sinx+cosx−(√(sin2x))] ⇒I=((√2)/2).ln∣cosx+sinx−(√(sin2x))∣+2cos^(−1) ∣cosx−sinx∣+C

$${arctg}\left(\sqrt{\mathrm{2}{tgx}}−\mathrm{1}\right)+{arctg}\left(\sqrt{\mathrm{2}{tgx}}+\mathrm{1}\right)= \\ $$$${arctg}\frac{\mathrm{2}\sqrt{\mathrm{2}{tgx}}}{\mathrm{2}\left(\mathrm{1}−{tgx}\right)}={arctg}\left(\frac{\sqrt{\mathrm{2}{tgx}}}{\mathrm{1}−{tgx}}\right)= \\ $$$${arc}\mathrm{cos}\mid{cosx}−{sinx}\mid \\ $$$${ln}\frac{{tgx}−\sqrt{\mathrm{2}{tgx}}+\mathrm{1}}{{tgx}+\sqrt{\mathrm{2}{tgx}}+\mathrm{1}}={ln}\frac{\left({tgx}+\mathrm{1}−\sqrt{\mathrm{2}{tgx}}\right)^{\mathrm{2}} }{{tg}^{\mathrm{2}} {x}+\mathrm{1}}= \\ $$$$=\mathrm{2}{ln}\left[{cosx}.\left({tgx}+\mathrm{1}−\sqrt{\mathrm{2}{tgx}}\right]=\right. \\ $$$$=\mathrm{2}{ln}\left[{sinx}+{cosx}−\sqrt{{sin}\mathrm{2}{x}}\right] \\ $$$$\Rightarrow{I}=\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}.\boldsymbol{\mathrm{ln}}\mid\boldsymbol{\mathrm{cosx}}+\boldsymbol{\mathrm{sinx}}−\sqrt{\boldsymbol{\mathrm{sin}}\mathrm{2}\boldsymbol{\mathrm{x}}}\mid+\mathrm{2}\boldsymbol{\mathrm{cos}}^{−\mathrm{1}} \mid\boldsymbol{\mathrm{cosx}}−\boldsymbol{\mathrm{sinx}}\mid+\boldsymbol{{C}} \\ $$

Commented by MJS last updated on 26/Feb/19

thank you!

$$\mathrm{thank}\:\mathrm{you}! \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com