Question and Answers Forum

All Questions      Topic List

UNKNOWN Questions

Previous in All Question      Next in All Question      

Previous in UNKNOWN      Next in UNKNOWN      

Question Number 55787 by gunawan last updated on 04/Mar/19

$$\mathrm{The}\mathrm{remainder}\mathrm{when}{5}^{99}\mathrm{is}\mathrm{divided}\mathrm{by}13\mathrm{is}$$

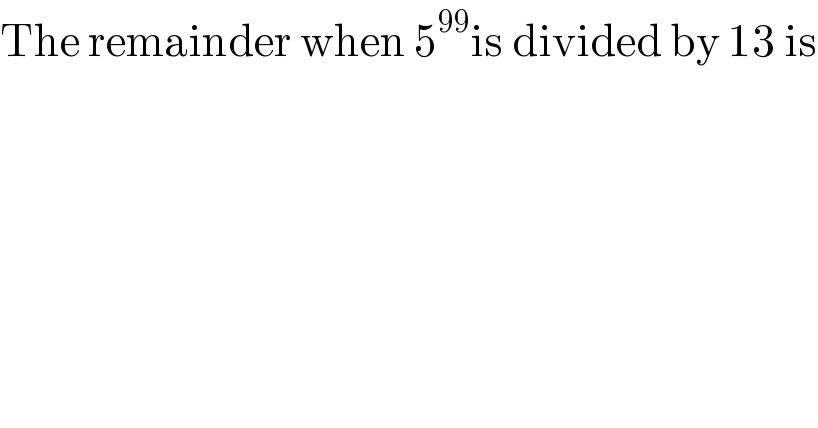
Answered by MJS last updated on 04/Mar/19

$$\mathrm{remainders}\mathrm{of}\frac{5^n}{13}$$$$n=1+4k:5$$$$n=2+4k:12$$$$n=3+4k:8$$$$n=4+4k:3$$$$99=3+4\times 24\Rightarrow \mathrm{remainder}\mathrm{is}8$$

Answered by tanmay.chaudhury50@gmail.com last updated on 04/Mar/19

$$tryingotherway$$$$5^{99}$$$$={\left(5^3\right)}^{33}$$$$={\left(125\right)}^{33}$$$$={(9\times 13+8)}^{33}$$$$={\left(9\times 13\right)}^{33}+33c_1{\left(9\times 13\right)}^{32}\times 8+...+8^{33}$$$$=f\left(13\right)+{\left(512\right)}^{11}$$$$=f\left(13\right)+{(13\times 39+5)}^{11}$$$$=f\left(13\right)+g\left(13\right)+5^{11}$$$$=f(13+g\left(13\right)+5\times {\left(5^2\right)}^5$$$$=f\left(13\right)+g\left(13\right)+5\times {(13+12)}^5$$$$=f\left(13\right)+g\left(13\right)+5\times [{13}^5+5c_1{13}^4+...+{12}^5]$$$$=f\left(13\right)+g\left(13\right)+5\times h\left(13\right)+5\times {12}^5$$$$=f\left(13\right)+g\left(13\right)+5\times h\left(13\right)+5\times {\left(144\right)}^3$$$$=f\left(13\right)+g\left(13\right)+5\times h\left(13\right)+5\times {(13\times 11+1)}^3$$$$=f\left(13\right)+g\left(13\right)+5\times h\left(13\right)+5\times [{\left(13\times 11\right)}^3+3\times {\left(13\times 11\right)}^2+3\times \left(13\times 11\right)\times 1^2+1^3]$$$$=f\left(13\right)+g\left(13\right)+5\times h\left(13\right)+5\times \gamma \left(13\right)+5\times 1^3$$$$soinmycalculationremaideris5$$

Commented by mr W last updated on 05/Mar/19

$$hereiswrongsir!$$$$=f\left(13\right)+g\left(13\right)+5\times h\left(13\right)+5\times {12}^5$$$$=f\left(13\right)+g\left(13\right)+5\times h\left(13\right)+5\times {\left(144\right)}^3$$$$itshouldbe:$$$$=f\left(13\right)+g\left(13\right)+5\times h\left(13\right)+5\times 12\times {\left(144\right)}^2$$$$=f\left(13\right)+g\left(13\right)+5\times h\left(13\right)+60\times {(13\times 11+1)}^2$$$$=......+60$$$$=......+13\times 4+8$$$$=......+8$$$$\Rightarrow remainderis8.$$

Commented by tanmay.chaudhury50@gmail.com last updated on 05/Mar/19

$$thankyousir..manymanythanks...$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com