The sum of the last eight coefficients in the expansion of (1+x)^(16) is 2^(15) .


Question and Answers Forum

All Questions Topic List
UNKNOWN Questions
Previous in All Question Next in All Question
Previous in UNKNOWN Next in UNKNOWN
Question Number 55788 by gunawan last updated on 04/Mar/19

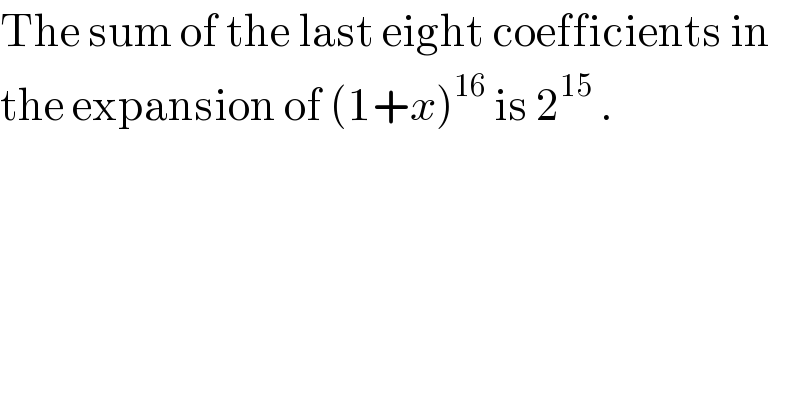
$The sum of the last eight coefficients in$ $the expansion of {(1 + x)}^{16} is 2^{15} .$
Commented by gunawan last updated on 04/Mar/19

$True or false$
Commented by maxmathsup by imad last updated on 04/Mar/19

$w e h a v e {(x + 1)}^{16} = \sum_{k = 0}^{16} C_{16}^{k} x^{k} = \sum_{k = 0}^{16} a_{k} x^{k} \Rightarrow$ $a_{0} + a_{1} + . . . . + a_{7} = \sum_{k = 0}^{7} C_{16}^{k} b u t i f p (x) = a_{0} + a_{1} x + a_{2} x^{2} + . . . + a_{n} x^{n} \Rightarrow$ $a_{o} + a_{1} + . . . . + a_{n} = p (1) a n d a_{o} + a_{1} + . . . + a_{p} = p (1) - a_{p + 1} - a_{p + 2} - . . . - a_{n} i n t h i s c a s e$ $p (x) = {(x + 1)}^{16} \Rightarrow a_{0} + a_{1} + . . . + a_{7} = p (1) - a_{8} - a_{9} - . . . . - a_{16}$ $b u t a_{8} = C_{16}^{8} a_{9} = C_{16}^{9} = C_{16}^{7} = a_{7}, a_{10} = C_{16}^{10} = C_{16}^{6} = a_{6}, a_{16} = a_{0}$ $2 (a_{0} + a_{1} + . . . . + a_{7}) = p (1) - a_{8} = 2^{16} - C_{16}^{8} = 2^{16} - \frac{16!}{8! 8!}$ $= 2^{16} - \frac{16!}{{(8!)}^{2}}$
Commented by maxmathsup by imad last updated on 04/Mar/19

$\Rightarrow a_{0} + a_{1} + . . . . + a_{7} = 2^{15} - \frac{1}{2} \frac{16!}{{(8!)}^{2}}$
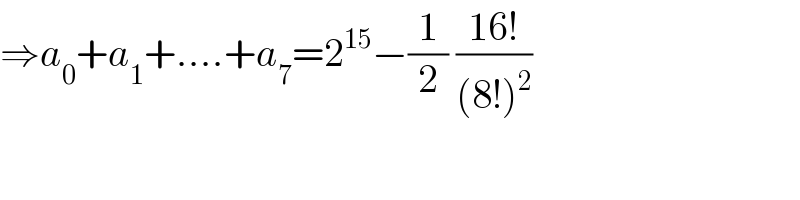
	Answered by tanmay.chaudhury50@gmail.com last updated on 04/Mar/19

	$t o t a l t e r m s = 16 + 1 = 17$ $s u m o f c o e f f u c i e n t s$ ${(1 + x)}^{16} = 1 + 16 c_{1} x + 16 c_{2} x^{2} + 16 c_{3} x^{3} + . . + 16 c_{16} x^{16}$ $2^{16} = 16 c_{0} + 16 c_{1} + . . . + 16 c_{8} + 16 c_{9} + 16 c_{10} + . . + 16 c_{16}$ $w e h a v e t i f i n d t h e v a l u e o f$ $16 c_{16} + 16 c_{15} + 16 c_{14} + 16 c_{13} + 16 c_{12} + 16 c_{11} + 16 c_{10} + 16 c_{9} = k$ $a g a i n$ $16 c_{0} + 16 c_{1} + 16 c_{2} + 16 c_{3} + 16 c_{4} + 16 c_{5} + 16 c_{6} + 16 c_{7} = k$ $[s i n c e {n c}_{r} = {n c}_{n - r}]$ $n o w$ $n i n t h t e r m i s 16 c_{8} x^{8} \to c o e e f i c i e n t = 16 c_{8}$ $s o 2 k + 16 c_{8} = 2^{16}$ $2 k = 2^{16} - \frac{16!}{8! 8!}$ $k = 2^{15} - \frac{1}{2} (\frac{16!}{8! 8!})$ $r e q u i r e d a n s i s 2^{15} - \frac{1}{2} (\frac{16!}{8! 8!})$ $p l s c h e c k . . .$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum