Question Number 56214 by necx1 last updated on 12/Mar/19
$${x}^{{x}} =\mathrm{4} \\ $$$$ \\ $$$${find}\:{x} \\ $$
Answered by tanmay.chaudhury50@gmail.com last updated on 12/Mar/19
$${x}=\mathrm{2}\:{only}\:{one}\:{solution}... \\ $$
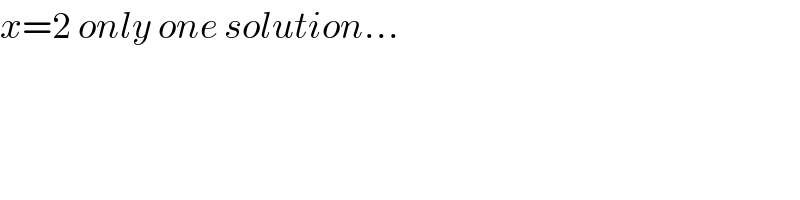
Commented by tanmay.chaudhury50@gmail.com last updated on 12/Mar/19
Commented by necx1 last updated on 12/Mar/19
$${solution}\:{please}\:{sir} \\ $$
Commented by necx1 last updated on 12/Mar/19
$${thanks}.....{Any}\:{analytical}\:{approach} \\ $$
Answered by mr W last updated on 13/Mar/19
$${general}: \\ $$$${x}^{{x}} ={a}\:\:{with}\:{a}\geqslant\frac{\mathrm{1}}{\sqrt[{{e}}]{{e}}}\approx\mathrm{0}.\mathrm{6922} \\ $$$$\Rightarrow{x}={a}^{\frac{\mathrm{1}}{{x}}} ={e}^{\frac{\mathrm{ln}\:{a}}{{x}}} \\ $$$$\Rightarrow\frac{\mathrm{1}}{{x}}{e}^{\frac{\mathrm{ln}\:{a}}{{x}}} =\mathrm{1} \\ $$$$\Rightarrow\frac{\mathrm{ln}\:{a}}{{x}}{e}^{\frac{\mathrm{ln}\:{a}}{{x}}} =\mathrm{ln}\:{a} \\ $$$$\Rightarrow\frac{\mathrm{ln}\:{a}}{{x}}=\mathbb{W}\left(\mathrm{ln}\:{a}\right)\:\:\:\leftarrow\:\:{Lambert}\:{function} \\ $$$$\Rightarrow{x}=\frac{\mathrm{ln}\:{a}}{\mathbb{W}\left(\mathrm{ln}\:{a}\right)} \\ $$$${if}\:{a}=\mathrm{4}: \\ $$$${x}=\frac{\mathrm{ln}\:\mathrm{4}}{{W}\left(\mathrm{ln}\:\mathrm{4}\right)}=\frac{\mathrm{ln}\:\mathrm{4}}{\mathrm{0}.\mathrm{6931}}=\mathrm{2} \\ $$$${if}\:{a}=\mathrm{5}: \\ $$$${x}=\frac{\mathrm{ln}\:\mathrm{5}}{{W}\left(\mathrm{ln}\:\mathrm{5}\right)}=\frac{\mathrm{ln}\:\mathrm{5}}{\mathrm{0}.\mathrm{7558}}=\mathrm{2}.\mathrm{1294} \\ $$
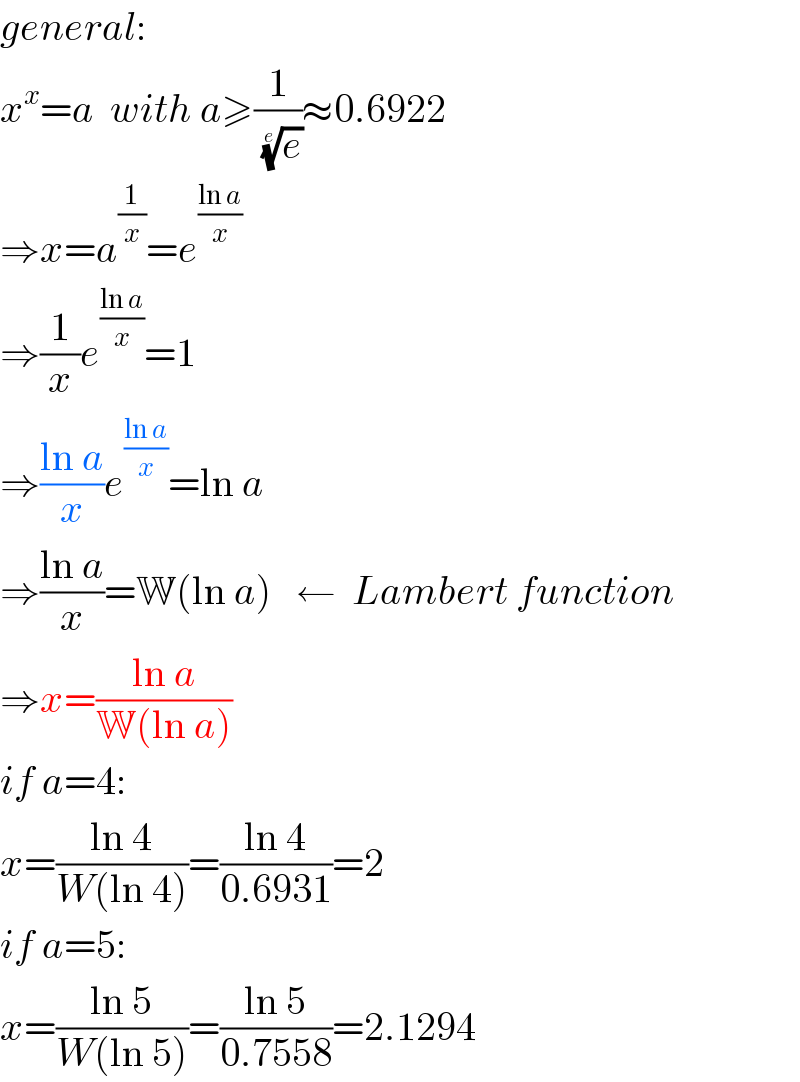
Answered by MJS last updated on 12/Mar/19
![x^x =4 xln x =2ln 2 so x=2 is obvious but is there another solution? x<0 x^x =(−x)^x (cos πx +isin πx) ⇒ sin πx =0 ∧ (−x)^x cos πx =4 sin πx =0 ⇒ x∈Z [(−x)^x cos πx ∣ x={−1, −2, −3, −4, ...}]= ={−1, (1/4), −(1/(27)), (1/(256)), ...} ⇒ no solution for x<0 x=0 0^0 is not defined but lim_(x→0^+ ) x^x =1 ⇒ no solution for x=0 0<x<1 x^x has its minimum at x=(1/e) and is decreasing in ]0; (1/e)[ and increasing in ](1/e); 1[ ⇒ no solution for 0<x<1 x≥1 x^x is increasing ⇒ no other solution than x=2](Q56271.png)
$${x}^{{x}} =\mathrm{4} \\ $$$${x}\mathrm{ln}\:{x}\:=\mathrm{2ln}\:\mathrm{2} \\ $$$$\mathrm{so}\:{x}=\mathrm{2}\:\mathrm{is}\:\mathrm{obvious} \\ $$$$\mathrm{but}\:\mathrm{is}\:\mathrm{there}\:\mathrm{another}\:\mathrm{solution}? \\ $$$${x}<\mathrm{0} \\ $$$${x}^{{x}} =\left(−{x}\right)^{{x}} \left(\mathrm{cos}\:\pi{x}\:+{i}\mathrm{sin}\:\pi{x}\right) \\ $$$$\Rightarrow\:\mathrm{sin}\:\pi{x}\:=\mathrm{0}\:\wedge\:\left(−{x}\right)^{{x}} \mathrm{cos}\:\pi{x}\:=\mathrm{4} \\ $$$$\:\:\:\:\:\:\mathrm{sin}\:\pi{x}\:=\mathrm{0}\:\Rightarrow\:{x}\in\mathbb{Z} \\ $$$$\:\:\:\:\:\:\left[\left(−{x}\right)^{{x}} \mathrm{cos}\:\pi{x}\:\mid\:{x}=\left\{−\mathrm{1},\:−\mathrm{2},\:−\mathrm{3},\:−\mathrm{4},\:...\right\}\right]= \\ $$$$\:\:\:\:\:\:\:\:\:\:\:=\left\{−\mathrm{1},\:\frac{\mathrm{1}}{\mathrm{4}},\:−\frac{\mathrm{1}}{\mathrm{27}},\:\frac{\mathrm{1}}{\mathrm{256}},\:...\right\} \\ $$$$\Rightarrow\:\mathrm{no}\:\mathrm{solution}\:\mathrm{for}\:{x}<\mathrm{0} \\ $$$${x}=\mathrm{0} \\ $$$$\mathrm{0}^{\mathrm{0}} \:\mathrm{is}\:\mathrm{not}\:\mathrm{defined}\:\mathrm{but} \\ $$$$\underset{{x}\rightarrow\mathrm{0}^{+} } {\mathrm{lim}}{x}^{{x}} =\mathrm{1} \\ $$$$\Rightarrow\:\mathrm{no}\:\mathrm{solution}\:\mathrm{for}\:{x}=\mathrm{0} \\ $$$$\mathrm{0}<{x}<\mathrm{1} \\ $$$${x}^{{x}} \:\mathrm{has}\:\mathrm{its}\:\mathrm{minimum}\:\mathrm{at}\:{x}=\frac{\mathrm{1}}{\mathrm{e}}\:\mathrm{and}\:\mathrm{is}\:\mathrm{decreasing} \\ $$$$\left.\:\:\:\:\:\mathrm{in}\:\right]\mathrm{0};\:\frac{\mathrm{1}}{\mathrm{e}}\left[\:\mathrm{and}\:\mathrm{increasing}\:\mathrm{in}\:\right]\frac{\mathrm{1}}{\mathrm{e}};\:\mathrm{1}\left[\right. \\ $$$$\Rightarrow\:\mathrm{no}\:\mathrm{solution}\:\mathrm{for}\:\mathrm{0}<{x}<\mathrm{1} \\ $$$${x}\geqslant\mathrm{1} \\ $$$${x}^{{x}} \:\mathrm{is}\:\mathrm{increasing}\:\Rightarrow\:\mathrm{no}\:\mathrm{other}\:\mathrm{solution}\:\mathrm{than} \\ $$$$\:\:\:\:\:{x}=\mathrm{2} \\ $$