
Question and Answers Forum
Question Number 56829 by maxmathsup by imad last updated on 24/Mar/19
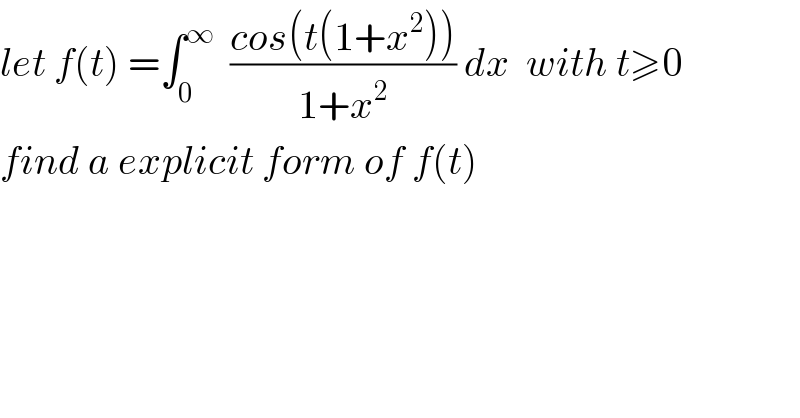
Answered by Smail last updated on 25/Mar/19

Commented by maxmathsup by imad last updated on 26/Mar/19
| ||
Question and Answers Forum | ||
Question Number 56829 by maxmathsup by imad last updated on 24/Mar/19 | ||
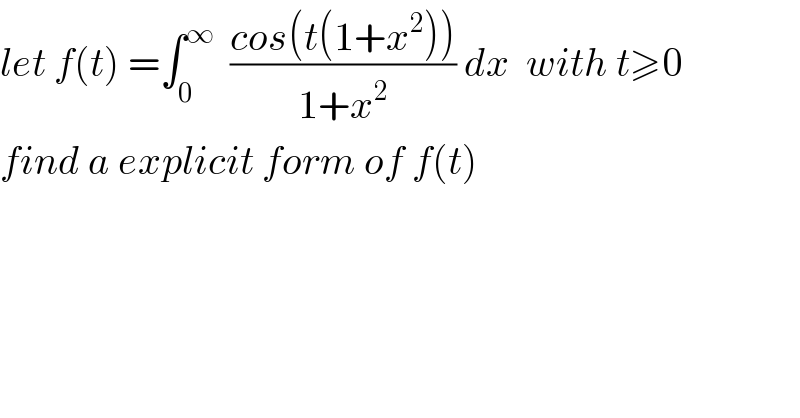 | ||
Answered by Smail last updated on 25/Mar/19 | ||
 | ||
| ||
Commented by maxmathsup by imad last updated on 26/Mar/19 | ||
 | ||