1−2sin(4x)<cos^2 (4x) solve.


Question and Answers Forum

All Questions Topic List
None Questions
Previous in All Question Next in All Question
Previous in None Next in None
Question Number 57357 by ANTARES VY last updated on 03/Apr/19

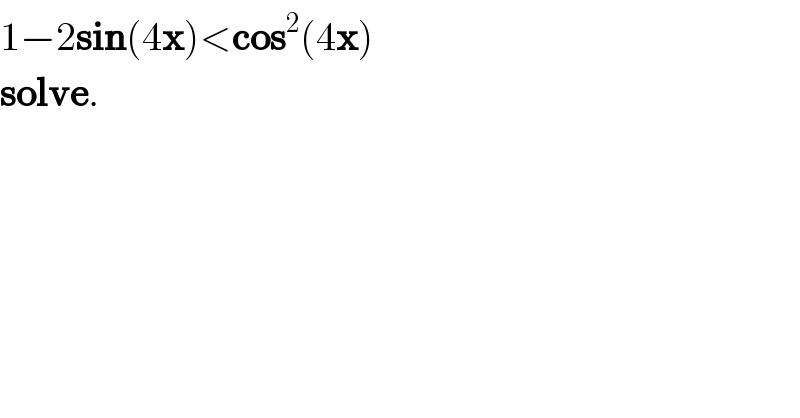
$1 - 2 \sin (4 x) < \cos^{2} (4 x)$ $solve .$
Commented bybshahid010@gmail.com last updated on 03/Apr/19

$1 - 2 \sin (4 x) < \cos^{2} (4 x)$ $1 - 2 \sin (4 x) - \cos^{2} (4 x) < 0$ $1 - 2 \sin (4 x) - 1 + \sin^{2} (4 x) < 0$ $\sin^{2} 4 x - 2 \sin 4 x < 0$ $\sin 4 x (\sin 4 x - 2) < 0$ $\sin 4 x \in (0, 2) so \sin 4 x \in (0, 1)$ $x \in (0, \sin 1)$
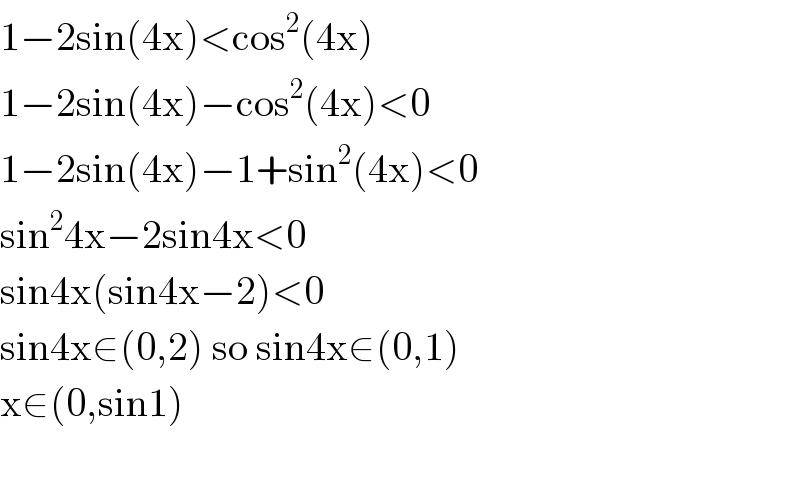
Commented bytanmay.chaudhury50@gmail.com last updated on 03/Apr/19

$m a x v a l u e o f s i n θ = 1$ $s o i t h i n k s i n 4 x \in (0, 1) b u t$ $s i n 4 x \notin (0, 2)$ $l e t o t h e r c o m m e n t . . .$
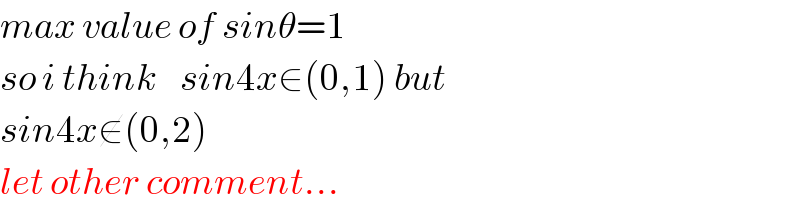
	Answered by tanmay.chaudhury50@gmail.com last updated on 03/Apr/19

	$s i n 4 x = a$ $1 - 2 a < 1 - a^{2}$ $a^{2} - 2 a < 0$ $a (a - 2) < 0$ $a \neq 2 a_{m a x} = 1$ $1 > a > 0 i s t h e r e a u i r e d s o l u t i o n$ $1 > s i n 4 x > 0$ $s i n \frac{π}{2} > s i n 4 x > s i n 0$ $\frac{π}{8} > x > 0$
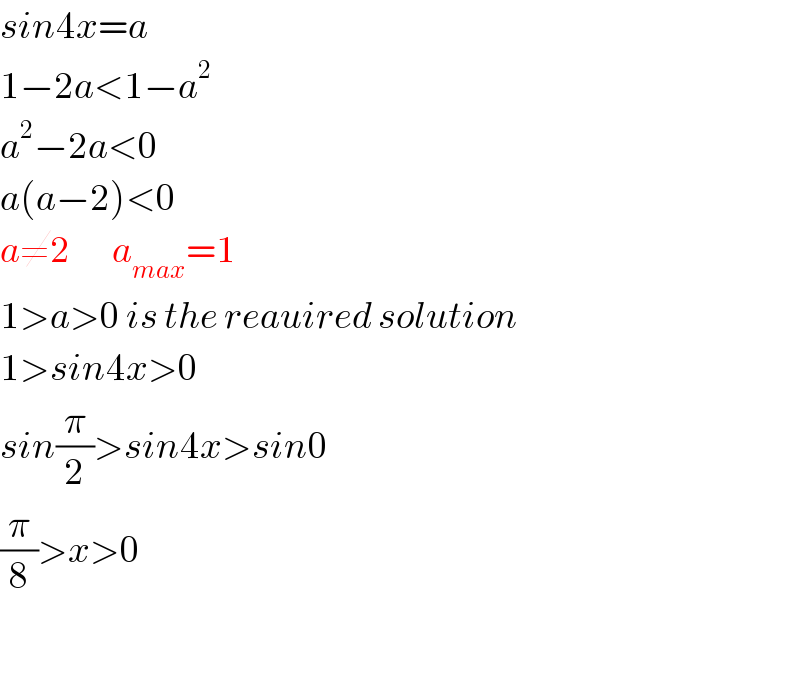
	Commented byANTARES VY last updated on 03/Apr/19

	$? ? ?$
	Answered by einsteindrmaths@hotmail.fr last updated on 03/Apr/19
	$cos^2 (4x)=1−sin^2 (4x)====>1−sin(4x)<1−sin^2 (4x) ==>sin^2 (4x)−sin(4x)<0==>sin(4x)(1−sin(4x))<0==> sin(4x)>0&1−sin(4x)≠0===>x≠{(π/8)+((kπ)/2)/k∈IZ}∪]((kπ)/(2 ));(((2k+1)π)/4)[$
	$c os^{2} (4 x) = 1 - {s i n}^{2} (4 x) ====> 1 - \sin (4 x) < 1 - {s i n}^{2} (4 x)$ $==> {s i n}^{2} (4 x) - s i n (4 x) < 0 ==> s i n (4 x) (1 - s i n (4 x)) < 0 ==>$ $s i n (4 x) > 0 & 1 - s i n (4 x) \neq 0 ===> x \neq {\frac{π}{8} + \frac{k π}{2} / k \in I Z} \cup] \frac{k π}{2}; \frac{(2 k + 1) π}{4} [$
	Answered by mr W last updated on 03/Apr/19
	$1−2 sin (4x)<1−sin^2 (4x) sin (4x){sin (4x)−2}<0 since sin (4x)−2<0 ⇒sin (4x)>0 or ⇒0<sin (4x)≤1 ⇒4x∈(2nπ,2nπ+π) ⇒x∈(((nπ)/2),((nπ)/2)+(π/4)) with n∈W$
	$1 - 2 \sin (4 x) < 1 - \sin^{2} (4 x)$ $\sin (4 x) {\sin (4 x) - 2} < 0$ $s i n c e \sin (4 x) - 2 < 0$ $\Rightarrow \sin (4 x) > 0$ $o r$ $\Rightarrow 0 < \sin (4 x) ⩽ 1$ $\Rightarrow 4 x \in (2 n π, 2 n π + π)$ $\Rightarrow x \in (\frac{n π}{2}, \frac{n π}{2} + \frac{π}{4}) w i t h n \in W$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum