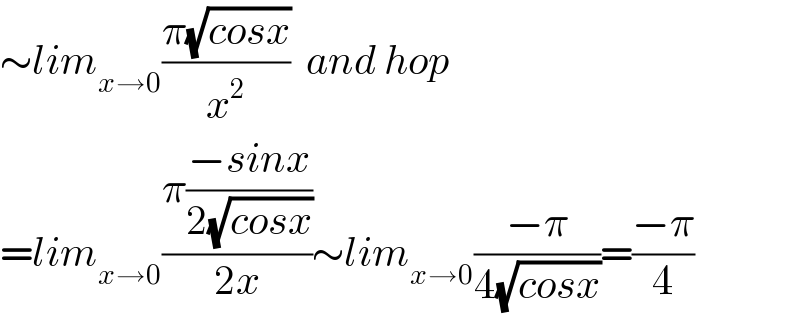Question and Answers Forum
Question Number 57404 by turbo msup by abdo last updated on 03/Apr/19

Commented by Smail last updated on 04/Apr/19
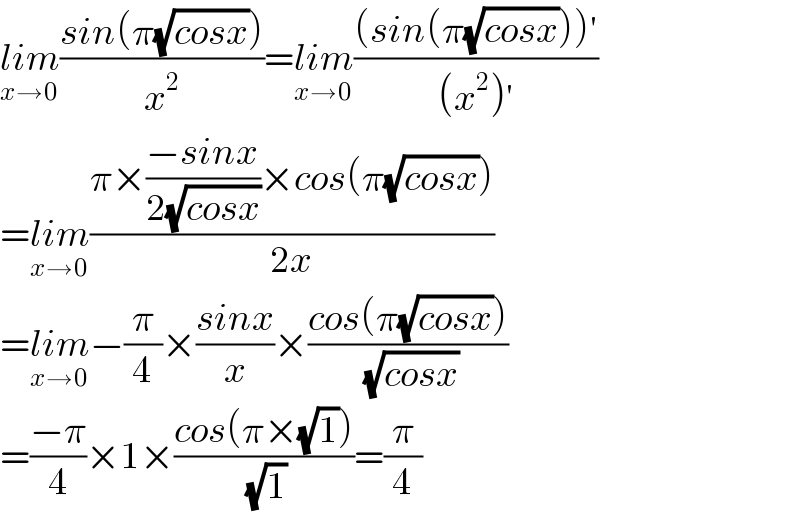
Commented by maxmathsup by imad last updated on 04/Apr/19
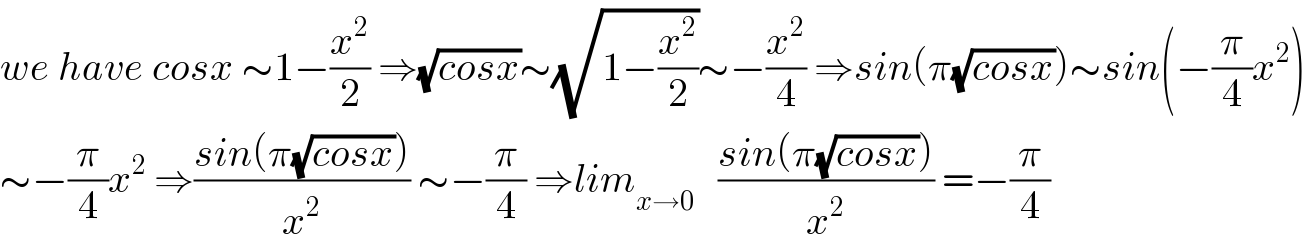
Commented by Smail last updated on 04/Apr/19
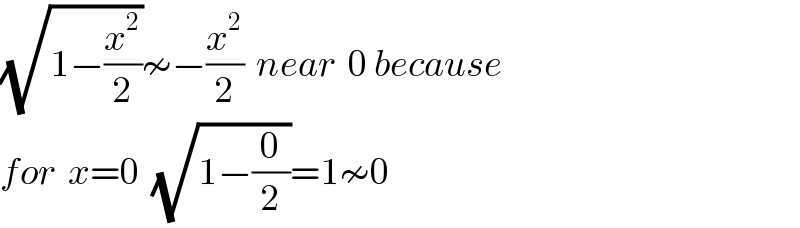
Commented by Smail last updated on 04/Apr/19

Commented by maxmathsup by imad last updated on 04/Apr/19
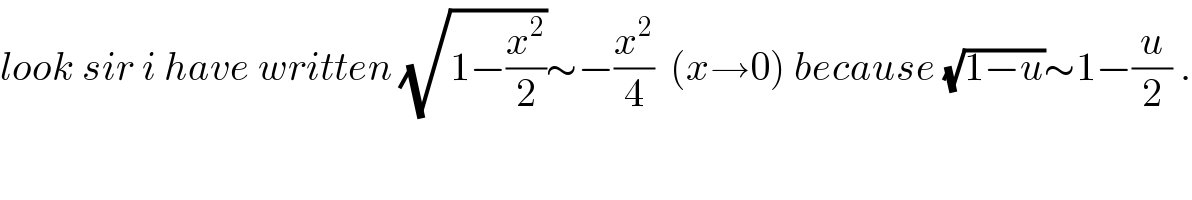
Answered by kaivan.ahmadi last updated on 04/Apr/19