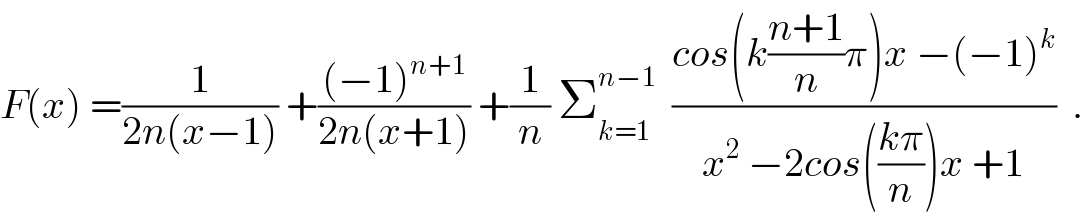decompose the fraction F(x) =(x^n /(x^(2n) −1)) inside C(x) and R(x)


Question and Answers Forum

All Questions Topic List
Relation and Functions Questions
Previous in All Question Next in All Question
Previous in Relation and Functions Next in Relation and Functions
Question Number 57923 by maxmathsup by imad last updated on 14/Apr/19
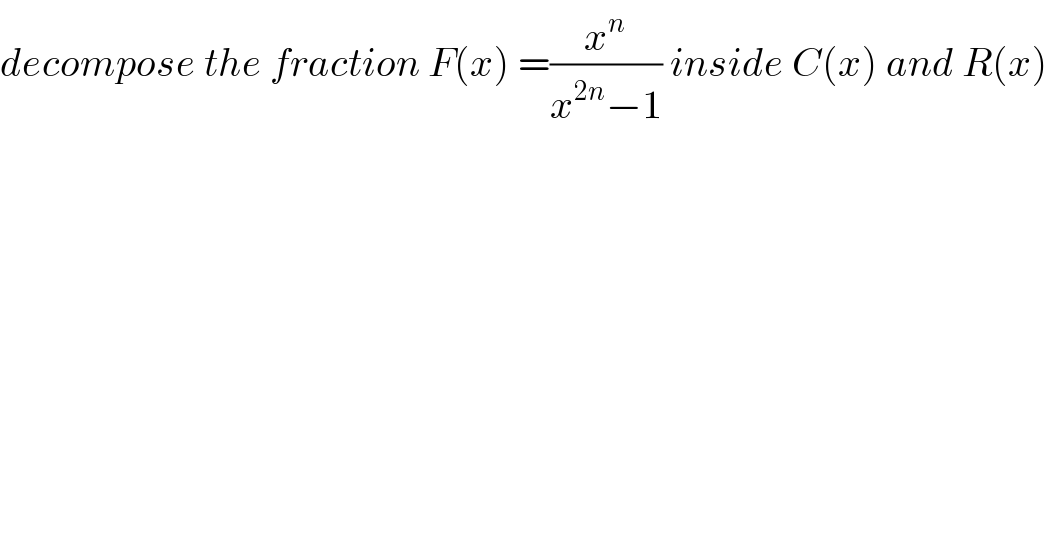
$decompose the fraction F(x) =(x^n /(x^(2n) −1)) inside C(x) and R(x)$
$d e c o m p o s e t h e f r a c t i o n F (x) = \frac{x^{n}}{x^{2 n} - 1} i n s i d e C (x) a n d R (x)$
Commented by maxmathsup by imad last updated on 28/Apr/19

$r o o t s o f z^{2 n} - 1 = 0 l e t z = {r e}^{i θ} s o z^{2 n} = 1 \Rightarrow r^{n} = 1 a n d 2 n θ = 2 k π \Rightarrow$ $θ_{k} = \frac{k π}{n} \Rightarrow t h e r o o t s {a r e Z}_{k} = e^{\frac{i k}{n}} a n d k \in [[0, 2 n - 1]] \Rightarrow$ $F (x) = \frac{x^{n}}{\prod_{k = 0}^{2 n - 1} (x - Z_{k})} = \sum_{k = 0}^{2 n - 1} \frac{λ_{k}}{x - Z_{k}} a n d λ_{k} = \frac{Z_{k}^{n}}{2 n Z_{k}^{2 n - 1}} = \frac{1}{2 n} Z_{k}^{n + 1} \Rightarrow$ $F (x) = \frac{1}{2 n} \sum_{k = 0}^{2 n - 1} \frac{Z_{k}^{n + 1}}{x - Z_{k}} i s t h e d e c o m p o s i t i o n i n s i d e C (x) .$
Commented by maxmathsup by imad last updated on 28/Apr/19
$we have Z_k =e^((ikπ)/n) with k∈[[0,2n−1]] Z_0 =1 , Z_1 =e^((iπ)/n) , Z_(2 ) = e^((i2π)/n) , Z_(n−1) =e^(i(((n−1)π)/n)) ,Z_n =−1 ,Z_(n+1) =e^(i(((n+1)π)/n)) , ....Z_(2n−1) =e^(i(((2n−1)π)/n)) ⇒Z_(2n−1) =Z_1 ^− ,Z_(2n−2) =Z_2 ^− .... ⇒ F(x) =(1/(2n)) { (1/(Z−1)) +(((−1)^(n+1) )/(x+1)) +Σ_(k=1) ^(n−1) ( (Z_k ^(n+1) /(x−Z_k )) +(((Z_k ^− )^(n+1) )/(x−Z_k ^− )))} ⇒ F(x)= (1/(2n(x−1))) +(((−1)^(n+1) )/(2n(x+1))) +(1/(2n)) +Σ_(k=0) ^(n−1) ((Z_k ^(n+1) (x−Z_k ^− )+(x−Z_k )(Z_k ^− )^(n+1) )/(x^2 −2xRe(Z_k )+1)) F(x) =(1/(2n(x−1))) +(((−1)^(n+1) )/(2n(x+1))) +Σ_(k=0) ^(n−1) (((Z_k ^(n+1) +(Z_k ^− )^(n+1) )x −Z_k ^(n+1) Z_k ^− −Z_k (Z_k ^− )^(n+1) )/(x^2 −2cos(((kπ)/n))x +1)) is the decomposition inside R(x) with Z_k ^(n+1) +(Z_k ^− )^(n+1) = 2 Re(Z_k ^(n+1) ) =2cos(k(((n+1)π)/n)) −Z_k ^(n+1) Z_k ^− −Z_k (Z_k ^− )^(n+1) =−( Z_k ^n +(Z_k ^− )^n ) =−2cos(kπ) =−2(−1)^k .$
$w e h a v e Z_{k} = e^{\frac{i k π}{n}} w i t h k \in [[0, 2 n - 1]]$ $Z_{0} = 1, Z_{1} = e^{\frac{i π}{n}}, Z_{2} = e^{\frac{i 2 π}{n}}, Z_{n - 1} = e^{i \frac{(n - 1) π}{n}}, Z_{n} = - 1, Z_{n + 1} = e^{i \frac{(n + 1) π}{n}},$ $. . . . Z_{2 n - 1} = e^{i \frac{(2 n - 1) π}{n}} \Rightarrow Z_{2 n - 1} = {\bar{Z}}_{1}, Z_{2 n - 2} = {\bar{Z}}_{2} . . . . \Rightarrow$ $F (x) = \frac{1}{2 n} {\frac{1}{Z - 1} + \frac{{(- 1)}^{n + 1}}{x + 1} + \sum_{k = 1}^{n - 1} (\frac{Z_{k}^{n + 1}}{x - Z_{k}} + \frac{{({\bar{Z}}_{k})}^{n + 1}}{x - {\bar{Z}}_{k}})} \Rightarrow$ $F (x) = \frac{1}{2 n (x - 1)} + \frac{{(- 1)}^{n + 1}}{2 n (x + 1)} + \frac{1}{2 n} + \sum_{k = 0}^{n - 1} \frac{Z_{k}^{n + 1} (x - {\bar{Z}}_{k}) + (x - Z_{k}) {({\bar{Z}}_{k})}^{n + 1}}{x^{2} - 2 x R e (Z_{k}) + 1}$ $F (x) = \frac{1}{2 n (x - 1)} + \frac{{(- 1)}^{n + 1}}{2 n (x + 1)} + \sum_{k = 0}^{n - 1} \frac{(Z_{k}^{n + 1} + {({\bar{Z}}_{k})}^{n + 1}) x - Z_{k}^{n + 1} {\bar{Z}}_{k} - Z_{k} {({\bar{Z}}_{k})}^{n + 1}}{x^{2} - 2 c o s (\frac{k π}{n}) x + 1} i s t h e d e c o m p o s i t i o n i n s i d e R (x)$ $w i t h Z_{k}^{n + 1} + {({\bar{Z}}_{k})}^{n + 1} = 2 R e (Z_{k}^{n + 1}) = 2 c o s (k \frac{(n + 1) π}{n})$ $- Z_{k}^{n + 1} {\bar{Z}}_{k} - Z_{k} {({\bar{Z}}_{k})}^{n + 1} = - (Z_{k}^{n} + {({\bar{Z}}_{k})}^{n}) = - 2 c o s (k π) = - 2 {(- 1)}^{k} .$
Commented by maxmathsup by imad last updated on 29/Apr/19

$F (x) = \frac{1}{2 n (x - 1)} + \frac{{(- 1)}^{n + 1}}{2 n (x + 1)} + \frac{1}{n} \sum_{k = 1}^{n - 1} \frac{c o s (k \frac{n + 1}{n} π) x - {(- 1)}^{k}}{x^{2} - 2 c o s (\frac{k π}{n}) x + 1} .$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum