Question and Answers Forum

All Questions      Topic List

Differentiation Questions

Previous in All Question      Next in All Question      

Previous in Differentiation      Next in Differentiation      

Question Number 58097 by rahul 19 last updated on 17/Apr/19

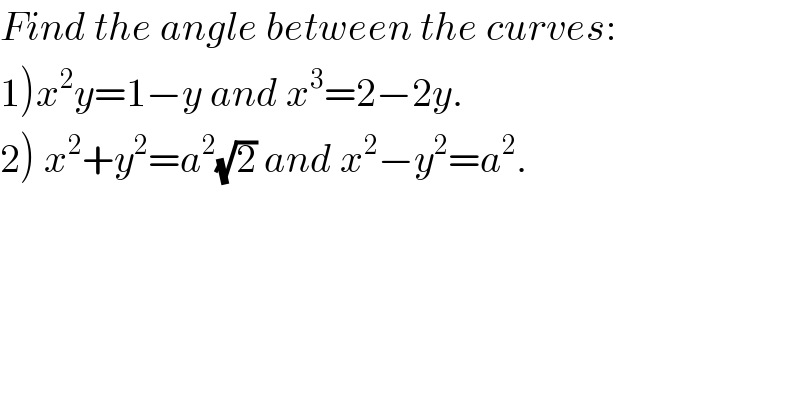
Find the angle between the curves: 1)x^2 y=1−y and x^3 =2−2y. 2) x^2 +y^2 =a^2 (√2) and x^2 −y^2 =a^2 .

$${Find}\:{the}\:{angle}\:{between}\:{the}\:{curves}: \\ $$$$\left.\mathrm{1}\right){x}^{\mathrm{2}} {y}=\mathrm{1}−{y}\:{and}\:{x}^{\mathrm{3}} =\mathrm{2}−\mathrm{2}{y}. \\ $$$$\left.\mathrm{2}\right)\:{x}^{\mathrm{2}} +{y}^{\mathrm{2}} ={a}^{\mathrm{2}} \sqrt{\mathrm{2}}\:{and}\:{x}^{\mathrm{2}} −{y}^{\mathrm{2}} ={a}^{\mathrm{2}} . \\ $$

Answered by tanmay last updated on 17/Apr/19

1)x^2 y=1−y y=(1/(1+x^2 )) x^3 =2−2y x^3 =2−2((1/(1+x^2 ))) x^3 (1+x^2 )=2(1+x^2 )−2 x^3 +x^5 =2x^2 x^2 (x+x^3 −2)=0 x^2 {(x−1)+x^3 −1}=0 x^2 (x−1)(1+x^2 +x+1)=0 x^2 (x−1)(x^2 +x+2)=0 x=0 x=1 x=((−1±(√(1−4×1×2)))/(2×1)) x=((−1±(√7) i)/2) ←not feasible first curve y=(1/(1+x^2 )) (dy/dx)=((−1)/((1+x^2 )^2 ))×2x m_1 =((−2×1)/((1+1)^2 ))=((−1)/2) 2y=2−x^3 y=1−(x^3 /2) →(dy/dx)=((−3)/2)×x^2 =((−3x^2 )/2) when point (x=1) m_2 =((−3)/2) [point x=1] tanθ_2 =((((−1)/2)+(3/2))/(1+(((−1)/2))(((−3)/2)))) =((2/2)/(1+(3/4)))=(4/7)→θ_2 =tan^(−1) ((4/7)) discussion... let two curve intesect at point(x_1 ,y_1 ) then angle between two tangents at that point(x_1 ,y_1 ) is the angle between two curves

$$\left.\mathrm{1}\right){x}^{\mathrm{2}} {y}=\mathrm{1}−{y} \\ $$$${y}=\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{2}} } \\ $$$${x}^{\mathrm{3}} =\mathrm{2}−\mathrm{2}{y} \\ $$$${x}^{\mathrm{3}} =\mathrm{2}−\mathrm{2}\left(\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{2}} }\right) \\ $$$${x}^{\mathrm{3}} \left(\mathrm{1}+{x}^{\mathrm{2}} \right)=\mathrm{2}\left(\mathrm{1}+{x}^{\mathrm{2}} \right)−\mathrm{2} \\ $$$${x}^{\mathrm{3}} +{x}^{\mathrm{5}} =\mathrm{2}{x}^{\mathrm{2}} \\ $$$${x}^{\mathrm{2}} \left({x}+{x}^{\mathrm{3}} −\mathrm{2}\right)=\mathrm{0} \\ $$$${x}^{\mathrm{2}} \left\{\left({x}−\mathrm{1}\right)+{x}^{\mathrm{3}} −\mathrm{1}\right\}=\mathrm{0} \\ $$$${x}^{\mathrm{2}} \left({x}−\mathrm{1}\right)\left(\mathrm{1}+{x}^{\mathrm{2}} +{x}+\mathrm{1}\right)=\mathrm{0} \\ $$$${x}^{\mathrm{2}} \left({x}−\mathrm{1}\right)\left({x}^{\mathrm{2}} +{x}+\mathrm{2}\right)=\mathrm{0} \\ $$$${x}=\mathrm{0} \\ $$$${x}=\mathrm{1} \\ $$$${x}=\frac{−\mathrm{1}\pm\sqrt{\mathrm{1}−\mathrm{4}×\mathrm{1}×\mathrm{2}}}{\mathrm{2}×\mathrm{1}} \\ $$$${x}=\frac{−\mathrm{1}\pm\sqrt{\mathrm{7}}\:{i}}{\mathrm{2}}\:\leftarrow{not}\:{feasible} \\ $$$$ \\ $$$${first}\:{curve}\:{y}=\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{2}} } \\ $$$$\frac{{dy}}{{dx}}=\frac{−\mathrm{1}}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{\mathrm{2}} }×\mathrm{2}{x}\: \\ $$$${m}_{\mathrm{1}} =\frac{−\mathrm{2}×\mathrm{1}}{\left(\mathrm{1}+\mathrm{1}\right)^{\mathrm{2}} }=\frac{−\mathrm{1}}{\mathrm{2}} \\ $$$$\mathrm{2}{y}=\mathrm{2}−{x}^{\mathrm{3}} \\ $$$${y}=\mathrm{1}−\frac{{x}^{\mathrm{3}} }{\mathrm{2}}\:\:\rightarrow\frac{{dy}}{{dx}}=\frac{−\mathrm{3}}{\mathrm{2}}×{x}^{\mathrm{2}} =\frac{−\mathrm{3}{x}^{\mathrm{2}} }{\mathrm{2}} \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$${when}\:{point}\:\left({x}=\mathrm{1}\right) \\ $$$$ \\ $$$${m}_{\mathrm{2}} =\frac{−\mathrm{3}}{\mathrm{2}}\:\left[{point}\:{x}=\mathrm{1}\right] \\ $$$${tan}\theta_{\mathrm{2}} =\frac{\frac{−\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{3}}{\mathrm{2}}}{\mathrm{1}+\left(\frac{−\mathrm{1}}{\mathrm{2}}\right)\left(\frac{−\mathrm{3}}{\mathrm{2}}\right)} \\ $$$$\:\:=\frac{\frac{\mathrm{2}}{\mathrm{2}}}{\mathrm{1}+\frac{\mathrm{3}}{\mathrm{4}}}=\frac{\mathrm{4}}{\mathrm{7}}\rightarrow\theta_{\mathrm{2}} ={tan}^{−\mathrm{1}} \left(\frac{\mathrm{4}}{\mathrm{7}}\right) \\ $$$${discussion}... \\ $$$${let}\:{two}\:{curve}\:{intesect}\:{at}\:{point}\left({x}_{\mathrm{1}} ,{y}_{\mathrm{1}} \right) \\ $$$${then}\:{angle}\:{between}\:{two}\:{tangents}\:{at}\:{that} \\ $$$${point}\left({x}_{\mathrm{1}} ,{y}_{\mathrm{1}} \right)\:{is}\:{the}\:{angle}\:{between}\:{two}\:{curves} \\ $$

Commented by rahul 19 last updated on 17/Apr/19

Thank u sir!

$${Thank}\:{u}\:{sir}! \\ $$

Commented by tanmay last updated on 18/Apr/19

most welcome rahul...

$${most}\:{welcome}\:{rahul}... \\ $$

Answered by tanmay last updated on 17/Apr/19

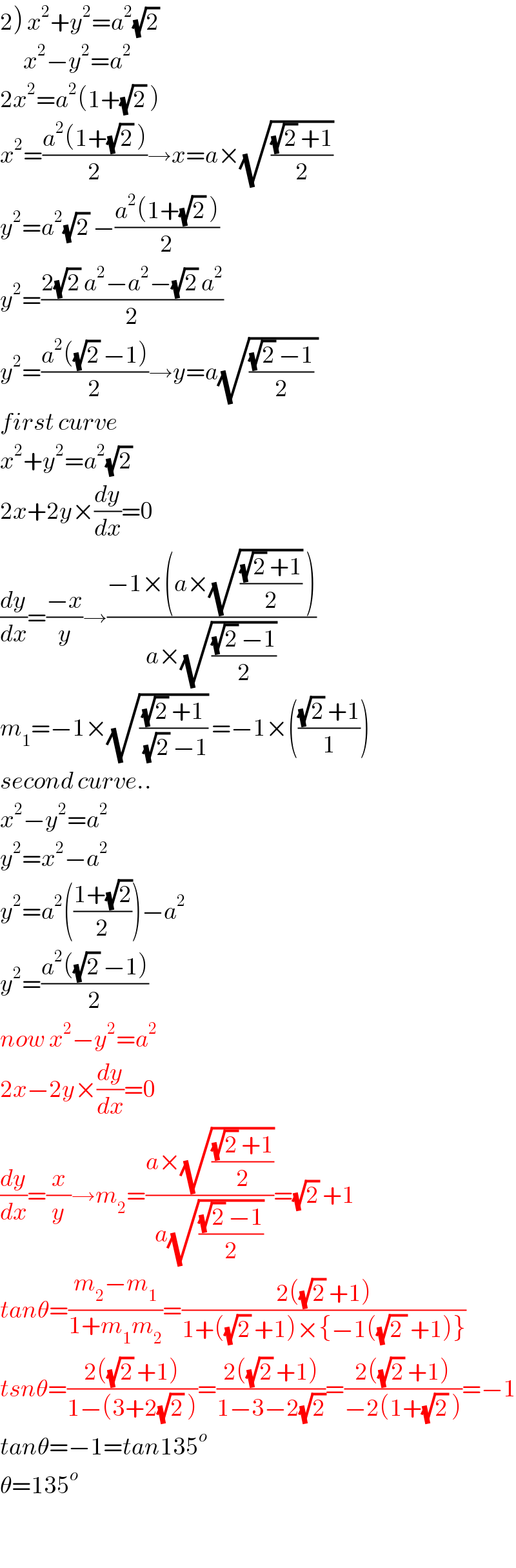
2) x^2 +y^2 =a^2 (√2) x^2 −y^2 =a^2 2x^2 =a^2 (1+(√2) ) x^2 =((a^2 (1+(√2) ))/2)→x=a×(√(((√2) +1)/2)) y^2 =a^2 (√2) −((a^2 (1+(√2) ))/2) y^2 =((2(√2) a^2 −a^2 −(√2) a^2 )/2) y^2 =((a^2 ((√2) −1))/2)→y=a(√((((√2) −1)/2) )) first curve x^2 +y^2 =a^2 (√2) 2x+2y×(dy/dx)=0 (dy/dx)=((−x)/y)→((−1×(a×(√(((√2) +1)/2)) ))/(a×(√(((√2) −1)/2)))) m_1 =−1×(√(((√2) +1)/((√2) −1))) =−1×((((√2) +1)/1)) second curve.. x^2 −y^2 =a^2 y^2 =x^2 −a^2 y^2 =a^2 (((1+(√2))/2))−a^2 y^2 =((a^2 ((√2) −1))/2) now x^2 −y^2 =a^2 2x−2y×(dy/dx)=0 (dy/dx)=(x/y)→m_2 =((a×(√(((√2) +1)/2)))/(a(√(((√2) −1)/2))))=(√2) +1 tanθ=((m_2 −m_1 )/(1+m_1 m_2 ))=((2((√2) +1))/(1+((√2) +1)×{−1((√(2 )) +1)})) tsnθ=((2((√2) +1))/(1−(3+2(√2) )))=((2((√2) +1))/(1−3−2(√2)))=((2((√2) +1))/(−2(1+(√2) )))=−1 tanθ=−1=tan135^o θ=135^o

$$\left.\mathrm{2}\right)\:{x}^{\mathrm{2}} +{y}^{\mathrm{2}} ={a}^{\mathrm{2}} \sqrt{\mathrm{2}} \\ $$$$\:\:\:\:\:\:{x}^{\mathrm{2}} −{y}^{\mathrm{2}} ={a}^{\mathrm{2}} \\ $$$$\mathrm{2}{x}^{\mathrm{2}} ={a}^{\mathrm{2}} \left(\mathrm{1}+\sqrt{\mathrm{2}}\:\right) \\ $$$${x}^{\mathrm{2}} =\frac{{a}^{\mathrm{2}} \left(\mathrm{1}+\sqrt{\mathrm{2}}\:\right)}{\mathrm{2}}\rightarrow{x}={a}×\sqrt{\frac{\sqrt{\mathrm{2}}\:+\mathrm{1}}{\mathrm{2}}} \\ $$$${y}^{\mathrm{2}} ={a}^{\mathrm{2}} \sqrt{\mathrm{2}}\:−\frac{{a}^{\mathrm{2}} \left(\mathrm{1}+\sqrt{\mathrm{2}}\:\right)}{\mathrm{2}} \\ $$$${y}^{\mathrm{2}} =\frac{\mathrm{2}\sqrt{\mathrm{2}}\:{a}^{\mathrm{2}} −{a}^{\mathrm{2}} −\sqrt{\mathrm{2}}\:{a}^{\mathrm{2}} }{\mathrm{2}} \\ $$$${y}^{\mathrm{2}} =\frac{{a}^{\mathrm{2}} \left(\sqrt{\mathrm{2}}\:−\mathrm{1}\right)}{\mathrm{2}}\rightarrow{y}={a}\sqrt{\frac{\sqrt{\mathrm{2}}\:−\mathrm{1}}{\mathrm{2}}\:} \\ $$$${first}\:{curve} \\ $$$${x}^{\mathrm{2}} +{y}^{\mathrm{2}} ={a}^{\mathrm{2}} \sqrt{\mathrm{2}}\: \\ $$$$\mathrm{2}{x}+\mathrm{2}{y}×\frac{{dy}}{{dx}}=\mathrm{0} \\ $$$$\frac{{dy}}{{dx}}=\frac{−{x}}{{y}}\rightarrow\frac{−\mathrm{1}×\left({a}×\sqrt{\frac{\sqrt{\mathrm{2}}\:+\mathrm{1}}{\mathrm{2}}}\:\right)}{{a}×\sqrt{\frac{\sqrt{\mathrm{2}}\:−\mathrm{1}}{\mathrm{2}}}} \\ $$$${m}_{\mathrm{1}} =−\mathrm{1}×\sqrt{\frac{\sqrt{\mathrm{2}}\:+\mathrm{1}}{\sqrt{\mathrm{2}}\:−\mathrm{1}}}\:=−\mathrm{1}×\left(\frac{\sqrt{\mathrm{2}}\:+\mathrm{1}}{\mathrm{1}}\right) \\ $$$${second}\:{curve}.. \\ $$$${x}^{\mathrm{2}} −{y}^{\mathrm{2}} ={a}^{\mathrm{2}} \\ $$$${y}^{\mathrm{2}} ={x}^{\mathrm{2}} −{a}^{\mathrm{2}} \\ $$$${y}^{\mathrm{2}} ={a}^{\mathrm{2}} \left(\frac{\mathrm{1}+\sqrt{\mathrm{2}}}{\mathrm{2}}\right)−{a}^{\mathrm{2}} \\ $$$${y}^{\mathrm{2}} =\frac{{a}^{\mathrm{2}} \left(\sqrt{\mathrm{2}}\:−\mathrm{1}\right)}{\mathrm{2}} \\ $$$${now}\:{x}^{\mathrm{2}} −{y}^{\mathrm{2}} ={a}^{\mathrm{2}} \\ $$$$\mathrm{2}{x}−\mathrm{2}{y}×\frac{{dy}}{{dx}}=\mathrm{0} \\ $$$$\frac{{dy}}{{dx}}=\frac{{x}}{{y}}\rightarrow{m}_{\mathrm{2}} =\frac{{a}×\sqrt{\frac{\sqrt{\mathrm{2}}\:+\mathrm{1}}{\mathrm{2}}}}{{a}\sqrt{\frac{\sqrt{\mathrm{2}}\:−\mathrm{1}}{\mathrm{2}}}}=\sqrt{\mathrm{2}}\:+\mathrm{1} \\ $$$${tan}\theta=\frac{{m}_{\mathrm{2}} −{m}_{\mathrm{1}} }{\mathrm{1}+{m}_{\mathrm{1}} {m}_{\mathrm{2}} }=\frac{\mathrm{2}\left(\sqrt{\mathrm{2}}\:+\mathrm{1}\right)}{\mathrm{1}+\left(\sqrt{\mathrm{2}}\:+\mathrm{1}\right)×\left\{−\mathrm{1}\left(\sqrt{\mathrm{2}\:}\:+\mathrm{1}\right)\right\}} \\ $$$${tsn}\theta=\frac{\mathrm{2}\left(\sqrt{\mathrm{2}}\:+\mathrm{1}\right)}{\mathrm{1}−\left(\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}\:\right)}=\frac{\mathrm{2}\left(\sqrt{\mathrm{2}}\:+\mathrm{1}\right)}{\mathrm{1}−\mathrm{3}−\mathrm{2}\sqrt{\mathrm{2}}}=\frac{\mathrm{2}\left(\sqrt{\mathrm{2}}\:+\mathrm{1}\right)}{−\mathrm{2}\left(\mathrm{1}+\sqrt{\mathrm{2}}\:\right)}=−\mathrm{1} \\ $$$${tan}\theta=−\mathrm{1}={tan}\mathrm{135}^{{o}} \\ $$$$\theta=\mathrm{135}^{{o}} \\ $$$$ \\ $$

Commented by rahul 19 last updated on 17/Apr/19

Ans given is 45°.

Commented by MJS last updated on 17/Apr/19

without going through above workings: the angle between 2 curves = the angle between their tangents. the angle between 2 straight lines is not unique, it′s either α or 180°−α ⇒ 45° is ok and 135° is ok too

$$\mathrm{without}\:\mathrm{going}\:\mathrm{through}\:\mathrm{above}\:\mathrm{workings}: \\ $$$$\mathrm{the}\:\mathrm{angle}\:\mathrm{between}\:\mathrm{2}\:\mathrm{curves}\:=\:\mathrm{the}\:\mathrm{angle} \\ $$$$\mathrm{between}\:\mathrm{their}\:\mathrm{tangents}.\:\mathrm{the}\:\mathrm{angle}\:\mathrm{between} \\ $$$$\mathrm{2}\:\mathrm{straight}\:\mathrm{lines}\:\mathrm{is}\:\mathrm{not}\:\mathrm{unique},\:\mathrm{it}'\mathrm{s}\:\mathrm{either} \\ $$$$\alpha\:\mathrm{or}\:\mathrm{180}°−\alpha\:\Rightarrow\:\mathrm{45}°\:\mathrm{is}\:\mathrm{ok}\:\mathrm{and}\:\mathrm{135}°\:\mathrm{is}\:\mathrm{ok}\:\mathrm{too} \\ $$

Commented by tanmay last updated on 18/Apr/19

thank you sir...

$${thank}\:{you}\:{sir}... \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com