Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 58770 by Mr X pcx last updated on 29/Apr/19

find the value of integrals I =∫_0 ^∞ (dx/((x^2 +1)^3 )) , J =∫_0 ^∞ (dx/((x^2 +1)^5 ))

$${find}\:{the}\:{value}\:{of}\:{integrals} \\ $$$$\:{I}\:=\int_{\mathrm{0}} ^{\infty} \:\:\:\:\frac{{dx}}{\left({x}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{3}} }\:\:\:,\:{J}\:=\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{dx}}{\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{5}} } \\ $$

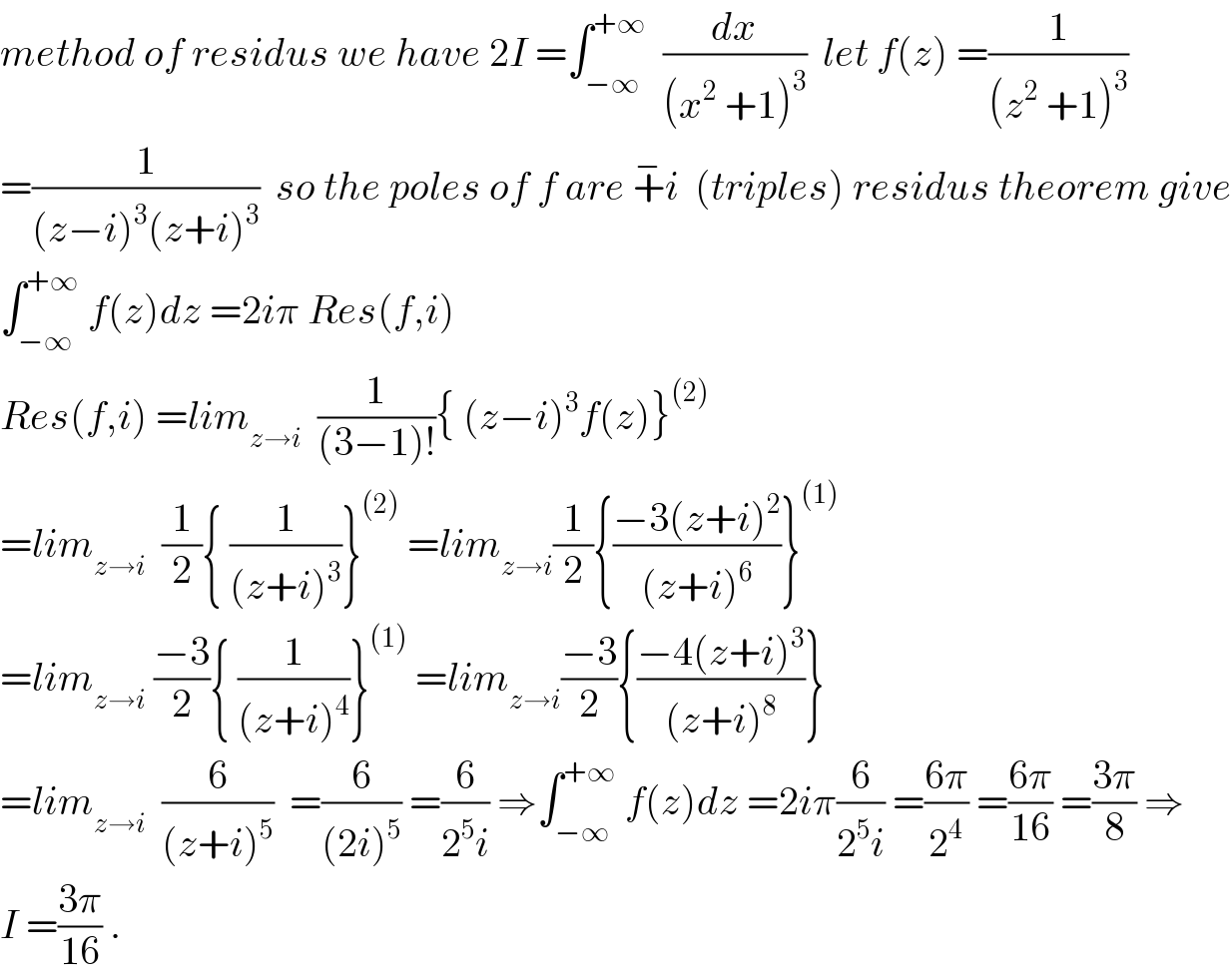
Commented by maxmathsup by imad last updated on 15/May/19

method of residus we have 2I =∫_(−∞) ^(+∞) (dx/((x^2 +1)^3 )) let f(z) =(1/((z^2 +1)^3 )) =(1/((z−i)^3 (z+i)^3 )) so the poles of f are +^− i (triples) residus theorem give ∫_(−∞) ^(+∞) f(z)dz =2iπ Res(f,i) Res(f,i) =lim_(z→i) (1/((3−1)!)){ (z−i)^3 f(z)}^((2)) =lim_(z→i) (1/2){ (1/((z+i)^3 ))}^((2)) =lim_(z→i) (1/2){((−3(z+i)^2 )/((z+i)^6 ))}^((1)) =lim_(z→i) ((−3)/2){ (1/((z+i)^4 ))}^((1)) =lim_(z→i) ((−3)/2){((−4(z+i)^3 )/((z+i)^8 ))} =lim_(z→i) (6/((z+i)^5 )) =(6/((2i)^5 )) =(6/(2^5 i)) ⇒∫_(−∞) ^(+∞) f(z)dz =2iπ(6/(2^5 i)) =((6π)/2^4 ) =((6π)/(16)) =((3π)/8) ⇒ I =((3π)/(16)) .

$${method}\:{of}\:{residus}\:{we}\:{have}\:\mathrm{2}{I}\:=\int_{−\infty} ^{+\infty} \:\:\frac{{dx}}{\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{3}} }\:\:{let}\:{f}\left({z}\right)\:=\frac{\mathrm{1}}{\left({z}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{3}} } \\ $$$$=\frac{\mathrm{1}}{\left({z}−{i}\right)^{\mathrm{3}} \left({z}+{i}\right)^{\mathrm{3}} }\:\:{so}\:{the}\:{poles}\:{of}\:{f}\:{are}\:\overset{−} {+}{i}\:\:\left({triples}\right)\:{residus}\:{theorem}\:{give} \\ $$$$\int_{−\infty} ^{+\infty} \:{f}\left({z}\right){dz}\:=\mathrm{2}{i}\pi\:{Res}\left({f},{i}\right) \\ $$$${Res}\left({f},{i}\right)\:={lim}_{{z}\rightarrow{i}} \:\:\frac{\mathrm{1}}{\left(\mathrm{3}−\mathrm{1}\right)!}\left\{\:\left({z}−{i}\right)^{\mathrm{3}} {f}\left({z}\right)\right\}^{\left(\mathrm{2}\right)} \\ $$$$={lim}_{{z}\rightarrow{i}} \:\:\frac{\mathrm{1}}{\mathrm{2}}\left\{\:\frac{\mathrm{1}}{\left({z}+{i}\right)^{\mathrm{3}} }\right\}^{\left(\mathrm{2}\right)} \:={lim}_{{z}\rightarrow{i}} \frac{\mathrm{1}}{\mathrm{2}}\left\{\frac{−\mathrm{3}\left({z}+{i}\right)^{\mathrm{2}} }{\left({z}+{i}\right)^{\mathrm{6}} }\right\}^{\left(\mathrm{1}\right)} \\ $$$$={lim}_{{z}\rightarrow{i}} \:\frac{−\mathrm{3}}{\mathrm{2}}\left\{\:\frac{\mathrm{1}}{\left({z}+{i}\right)^{\mathrm{4}} }\right\}^{\left(\mathrm{1}\right)} \:={lim}_{{z}\rightarrow{i}} \frac{−\mathrm{3}}{\mathrm{2}}\left\{\frac{−\mathrm{4}\left({z}+{i}\right)^{\mathrm{3}} }{\left({z}+{i}\right)^{\mathrm{8}} }\right\} \\ $$$$={lim}_{{z}\rightarrow{i}} \:\:\frac{\mathrm{6}}{\left({z}+{i}\right)^{\mathrm{5}} }\:\:=\frac{\mathrm{6}}{\left(\mathrm{2}{i}\right)^{\mathrm{5}} }\:=\frac{\mathrm{6}}{\mathrm{2}^{\mathrm{5}} {i}}\:\Rightarrow\int_{−\infty} ^{+\infty} \:{f}\left({z}\right){dz}\:=\mathrm{2}{i}\pi\frac{\mathrm{6}}{\mathrm{2}^{\mathrm{5}} {i}}\:=\frac{\mathrm{6}\pi}{\mathrm{2}^{\mathrm{4}} }\:=\frac{\mathrm{6}\pi}{\mathrm{16}}\:=\frac{\mathrm{3}\pi}{\mathrm{8}}\:\Rightarrow \\ $$$${I}\:=\frac{\mathrm{3}\pi}{\mathrm{16}}\:. \\ $$

Answered by tanmay last updated on 30/Apr/19

I=∫_0 ^∞ (dx/((x^2 +1)^3 )) x=tana dx=sec^2 ada ∫_0 ^(π/2) ((sec^2 ada)/(sec^6 a)) ∫_0 ^(π/2) cos^4 ada ∫_0 ^(π/2) (sina)^(2×(1/2)−1) ×(cosa)^(2×(5/2)−1) da =((⌈((1/2))⌈((5/2)))/(2⌈((1/2)+(5/2)))) now ⌈((1/2))=(√π) ⌈((5/2))=⌈((3/2)+1) =(3/2)⌈((3/2)) =(3/2)×⌈((1/2)+1) =(3/2)×(1/2)×(√π) ⌈((1/2)+(5/2)) =⌈(2+1) =2⌈(1+1) =2×1 =2 so answer is =(((√π) ×(3/4)×(√π) )/(2×2))=(3/(16))π J=∫_0 ^∞ (dx/((x^2 +1)^5 )) =∫_0 ^(π/2) ((sec^2 ada)/(sec^(10) a)) =∫_0 ^(π/2) cos^8 ada =∫_0 ^(π/2) (sina)^(2×(1/2)−1) (cosa)^(2×(9/2)−1) da =((⌈((1/2))⌈((9/2)))/(2⌈(((1+9)/2)))) =(((√π) ×(7/2)×(5/2)×(3/2)×(1/2)×(√π))/(2×4×3×2)) [⌈5)=⌈(4+1)=4! =((π×((7×5×3)/(2×2×2×2)))/(2×4×3×2)) =π×((7×5×3)/(16×4×3×2×2)) =((35π)/(128))×(1/2)=((35π)/(256)) pls check ∫_0 ^(π/2) sin^(2p−1) a×cos_ ^(2q−1) ada=((⌈(p)⌈q))/(2⌈(p+q))) i forgot to put 2 of denominator...later corrected

$${I}=\int_{\mathrm{0}} ^{\infty} \frac{{dx}}{\left({x}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{3}} } \\ $$$${x}={tana}\:{dx}={sec}^{\mathrm{2}} {ada} \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{{sec}^{\mathrm{2}} {ada}}{{sec}^{\mathrm{6}} {a}} \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {cos}^{\mathrm{4}} {ada} \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \left({sina}\right)^{\mathrm{2}×\frac{\mathrm{1}}{\mathrm{2}}−\mathrm{1}} ×\left({cosa}\right)^{\mathrm{2}×\frac{\mathrm{5}}{\mathrm{2}}−\mathrm{1}} {da} \\ $$$$=\frac{\lceil\left(\frac{\mathrm{1}}{\mathrm{2}}\right)\lceil\left(\frac{\mathrm{5}}{\mathrm{2}}\right)}{\mathrm{2}\lceil\left(\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{5}}{\mathrm{2}}\right)} \\ $$$${now}\:\lceil\left(\frac{\mathrm{1}}{\mathrm{2}}\right)=\sqrt{\pi}\: \\ $$$$\lceil\left(\frac{\mathrm{5}}{\mathrm{2}}\right)=\lceil\left(\frac{\mathrm{3}}{\mathrm{2}}+\mathrm{1}\right) \\ $$$$=\frac{\mathrm{3}}{\mathrm{2}}\lceil\left(\frac{\mathrm{3}}{\mathrm{2}}\right) \\ $$$$=\frac{\mathrm{3}}{\mathrm{2}}×\lceil\left(\frac{\mathrm{1}}{\mathrm{2}}+\mathrm{1}\right) \\ $$$$=\frac{\mathrm{3}}{\mathrm{2}}×\frac{\mathrm{1}}{\mathrm{2}}×\sqrt{\pi}\: \\ $$$$\lceil\left(\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{5}}{\mathrm{2}}\right) \\ $$$$=\lceil\left(\mathrm{2}+\mathrm{1}\right) \\ $$$$=\mathrm{2}\lceil\left(\mathrm{1}+\mathrm{1}\right) \\ $$$$=\mathrm{2}×\mathrm{1} \\ $$$$=\mathrm{2} \\ $$$${so}\:{answer}\:{is}\:=\frac{\sqrt{\pi}\:×\frac{\mathrm{3}}{\mathrm{4}}×\sqrt{\pi}\:}{\mathrm{2}×\mathrm{2}}=\frac{\mathrm{3}}{\mathrm{16}}\pi \\ $$$${J}=\int_{\mathrm{0}} ^{\infty} \frac{{dx}}{\left({x}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{5}} } \\ $$$$=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{{sec}^{\mathrm{2}} {ada}}{{sec}^{\mathrm{10}} {a}} \\ $$$$=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {cos}^{\mathrm{8}} {ada} \\ $$$$=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \left({sina}\right)^{\mathrm{2}×\frac{\mathrm{1}}{\mathrm{2}}−\mathrm{1}} \left({cosa}\right)^{\mathrm{2}×\frac{\mathrm{9}}{\mathrm{2}}−\mathrm{1}} {da} \\ $$$$=\frac{\lceil\left(\frac{\mathrm{1}}{\mathrm{2}}\right)\lceil\left(\frac{\mathrm{9}}{\mathrm{2}}\right)}{\mathrm{2}\lceil\left(\frac{\mathrm{1}+\mathrm{9}}{\mathrm{2}}\right)} \\ $$$$=\frac{\sqrt{\pi}\:×\frac{\mathrm{7}}{\mathrm{2}}×\frac{\mathrm{5}}{\mathrm{2}}×\frac{\mathrm{3}}{\mathrm{2}}×\frac{\mathrm{1}}{\mathrm{2}}×\sqrt{\pi}}{\mathrm{2}×\mathrm{4}×\mathrm{3}×\mathrm{2}}\:\:\:\:\left[\lceil\mathrm{5}\right)=\lceil\left(\mathrm{4}+\mathrm{1}\right)=\mathrm{4}! \\ $$$$=\frac{\pi×\frac{\mathrm{7}×\mathrm{5}×\mathrm{3}}{\mathrm{2}×\mathrm{2}×\mathrm{2}×\mathrm{2}}}{\mathrm{2}×\mathrm{4}×\mathrm{3}×\mathrm{2}} \\ $$$$=\pi×\frac{\mathrm{7}×\mathrm{5}×\mathrm{3}}{\mathrm{16}×\mathrm{4}×\mathrm{3}×\mathrm{2}×\mathrm{2}} \\ $$$$=\frac{\mathrm{35}\pi}{\mathrm{128}}×\frac{\mathrm{1}}{\mathrm{2}}=\frac{\mathrm{35}\pi}{\mathrm{256}} \\ $$$${pls}\:{check} \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {sin}^{\mathrm{2}{p}−\mathrm{1}} {a}×{cos}_{} ^{\mathrm{2}{q}−\mathrm{1}} {ada}=\frac{\left.\lceil\left({p}\right)\lceil{q}\right)}{\mathrm{2}\lceil\left({p}+{q}\right)} \\ $$$${i}\:{forgot}\:{to}\:{put}\:\mathrm{2}\:{of}\:{denominator}...{later}\:{corrected} \\ $$$$ \\ $$

Commented by Mr X pcx last updated on 30/Apr/19

thanks sir

$${thanks}\:{sir} \\ $$

Commented by tanmay last updated on 30/Apr/19

most welcome sir...

$${most}\:{welcome}\:{sir}... \\ $$

Answered by Smail last updated on 30/Apr/19

A_n =∫_0 ^∞ (dx/((x^2 +1)^n )) let x=tant⇒dx=(1+tan^2 t)dt A_n =∫_0 ^(π/2) ((1+tan^2 t)/((1+tan^2 t)^n ))dt=∫_0 ^(π/2) (dt/((1+tan^2 t)^(n−1) )) =∫_0 ^(π/2) cos^(2(n−1)) tdt=∫_0 ^(π/2) (1−sin^2 t)cos^(2n−4) tdt =∫_0 ^(π/2) cos^(2(n−2)) tdt−∫_0 ^(π/2) sintsintcos^(2n−4) tdt =A_(n−1) −∫_0 ^(π/2) sint×((d(−cos^(2n−3) t))/((2n−3))) =A_(n−1) +[(1/(2n−3))sint×cos^(2n−3) t]_0 ^(π/2) −∫_0 ^(π/2) cost×((cos^(2n−3) t)/(2n−3))dt =A_(n−1) −(A_n /(2n−3)) A_n =((2n−3)/(2(n−1)))A_(n−1) =((2n−3)/(2(n−1)))(((2n−5)/(2(n−2)))A_(n−2) ) ... A_n =(((2(n−1)−1)(2(n−2)−1)...(2(n−(n−1))−1))/(2^(n−1) (n−1)(n−2)...(n−(n−1))))A_(n−(n−1)) =(((2n−3)(2n−4)(2n−5)(2n−6)...1)/(2^(n−1) (n−1)!×(2n−4)(2n−6)...2))A_1 =(((2n−3)!)/(2^(n−1) (n−1)!×2^(n−2) (n−2)!))∫_0 ^(π/2) dt =(((2n−3)!)/(2^(2n−3) (n−1)((n−2)!)^2 ))×(π/2) A_n =((π(n−1)(2n−3)!)/(2^(2n−2) ((n−1)!)^2 )) For n=3 A_3 =I=∫_0 ^∞ (dx/((x^2 +1)^3 ))=((π(3−1)(6−3)!)/(2^(6−2) ((3−1)!)^2 ))=((3π)/(16)) For n=5 A_5 =J=∫_0 ^∞ (dx/((x^2 +1)^5 ))=((π(5−1)(10−3)!)/(2^(10−2) ((5−1)!)^2 )) =((7!π)/(2^(12) ×3^2 ))=((7×5π)/2^8 )=((35π)/(256))

$${A}_{{n}} =\int_{\mathrm{0}} ^{\infty} \frac{{dx}}{\left({x}^{\mathrm{2}} +\mathrm{1}\right)^{{n}} } \\ $$$${let}\:{x}={tant}\Rightarrow{dx}=\left(\mathrm{1}+{tan}^{\mathrm{2}} {t}\right){dt} \\ $$$${A}_{{n}} =\int_{\mathrm{0}} ^{\pi/\mathrm{2}} \frac{\mathrm{1}+{tan}^{\mathrm{2}} {t}}{\left(\mathrm{1}+{tan}^{\mathrm{2}} {t}\right)^{{n}} }{dt}=\int_{\mathrm{0}} ^{\pi/\mathrm{2}} \frac{{dt}}{\left(\mathrm{1}+{tan}^{\mathrm{2}} {t}\right)^{{n}−\mathrm{1}} } \\ $$$$=\int_{\mathrm{0}} ^{\pi/\mathrm{2}} {cos}^{\mathrm{2}\left({n}−\mathrm{1}\right)} {tdt}=\int_{\mathrm{0}} ^{\pi/\mathrm{2}} \left(\mathrm{1}−{sin}^{\mathrm{2}} {t}\right){cos}^{\mathrm{2}{n}−\mathrm{4}} {tdt} \\ $$$$=\int_{\mathrm{0}} ^{\pi/\mathrm{2}} {cos}^{\mathrm{2}\left({n}−\mathrm{2}\right)} {tdt}−\int_{\mathrm{0}} ^{\pi/\mathrm{2}} {sintsintcos}^{\mathrm{2}{n}−\mathrm{4}} {tdt} \\ $$$$={A}_{{n}−\mathrm{1}} −\int_{\mathrm{0}} ^{\pi/\mathrm{2}} {sint}×\frac{{d}\left(−{cos}^{\mathrm{2}{n}−\mathrm{3}} {t}\right)}{\left(\mathrm{2}{n}−\mathrm{3}\right)} \\ $$$$={A}_{{n}−\mathrm{1}} +\left[\frac{\mathrm{1}}{\mathrm{2}{n}−\mathrm{3}}{sint}×{cos}^{\mathrm{2}{n}−\mathrm{3}} {t}\right]_{\mathrm{0}} ^{\pi/\mathrm{2}} −\int_{\mathrm{0}} ^{\pi/\mathrm{2}} {cost}×\frac{{cos}^{\mathrm{2}{n}−\mathrm{3}} {t}}{\mathrm{2}{n}−\mathrm{3}}{dt} \\ $$$$={A}_{{n}−\mathrm{1}} −\frac{{A}_{{n}} }{\mathrm{2}{n}−\mathrm{3}} \\ $$$${A}_{{n}} =\frac{\mathrm{2}{n}−\mathrm{3}}{\mathrm{2}\left({n}−\mathrm{1}\right)}{A}_{{n}−\mathrm{1}} =\frac{\mathrm{2}{n}−\mathrm{3}}{\mathrm{2}\left({n}−\mathrm{1}\right)}\left(\frac{\mathrm{2}{n}−\mathrm{5}}{\mathrm{2}\left({n}−\mathrm{2}\right)}{A}_{{n}−\mathrm{2}} \right) \\ $$$$... \\ $$$${A}_{{n}} =\frac{\left(\mathrm{2}\left({n}−\mathrm{1}\right)−\mathrm{1}\right)\left(\mathrm{2}\left({n}−\mathrm{2}\right)−\mathrm{1}\right)...\left(\mathrm{2}\left({n}−\left({n}−\mathrm{1}\right)\right)−\mathrm{1}\right)}{\mathrm{2}^{{n}−\mathrm{1}} \left({n}−\mathrm{1}\right)\left({n}−\mathrm{2}\right)...\left({n}−\left({n}−\mathrm{1}\right)\right)}{A}_{{n}−\left({n}−\mathrm{1}\right)} \\ $$$$=\frac{\left(\mathrm{2}{n}−\mathrm{3}\right)\left(\mathrm{2}{n}−\mathrm{4}\right)\left(\mathrm{2}{n}−\mathrm{5}\right)\left(\mathrm{2}{n}−\mathrm{6}\right)...\mathrm{1}}{\mathrm{2}^{{n}−\mathrm{1}} \left({n}−\mathrm{1}\right)!×\left(\mathrm{2}{n}−\mathrm{4}\right)\left(\mathrm{2}{n}−\mathrm{6}\right)...\mathrm{2}}{A}_{\mathrm{1}} \\ $$$$=\frac{\left(\mathrm{2}{n}−\mathrm{3}\right)!}{\mathrm{2}^{{n}−\mathrm{1}} \left({n}−\mathrm{1}\right)!×\mathrm{2}^{{n}−\mathrm{2}} \left({n}−\mathrm{2}\right)!}\int_{\mathrm{0}} ^{\pi/\mathrm{2}} {dt} \\ $$$$=\frac{\left(\mathrm{2}{n}−\mathrm{3}\right)!}{\mathrm{2}^{\mathrm{2}{n}−\mathrm{3}} \left({n}−\mathrm{1}\right)\left(\left({n}−\mathrm{2}\right)!\right)^{\mathrm{2}} }×\frac{\pi}{\mathrm{2}} \\ $$$${A}_{{n}} =\frac{\pi\left({n}−\mathrm{1}\right)\left(\mathrm{2}{n}−\mathrm{3}\right)!}{\mathrm{2}^{\mathrm{2}{n}−\mathrm{2}} \left(\left({n}−\mathrm{1}\right)!\right)^{\mathrm{2}} } \\ $$$${For}\:{n}=\mathrm{3} \\ $$$${A}_{\mathrm{3}} ={I}=\int_{\mathrm{0}} ^{\infty} \frac{{dx}}{\left({x}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{3}} }=\frac{\pi\left(\mathrm{3}−\mathrm{1}\right)\left(\mathrm{6}−\mathrm{3}\right)!}{\mathrm{2}^{\mathrm{6}−\mathrm{2}} \left(\left(\mathrm{3}−\mathrm{1}\right)!\right)^{\mathrm{2}} }=\frac{\mathrm{3}\pi}{\mathrm{16}} \\ $$$${For}\:{n}=\mathrm{5} \\ $$$${A}_{\mathrm{5}} ={J}=\int_{\mathrm{0}} ^{\infty} \frac{{dx}}{\left({x}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{5}} }=\frac{\pi\left(\mathrm{5}−\mathrm{1}\right)\left(\mathrm{10}−\mathrm{3}\right)!}{\mathrm{2}^{\mathrm{10}−\mathrm{2}} \left(\left(\mathrm{5}−\mathrm{1}\right)!\right)^{\mathrm{2}} } \\ $$$$=\frac{\mathrm{7}!\pi}{\mathrm{2}^{\mathrm{12}} ×\mathrm{3}^{\mathrm{2}} }=\frac{\mathrm{7}×\mathrm{5}\pi}{\mathrm{2}^{\mathrm{8}} }=\frac{\mathrm{35}\pi}{\mathrm{256}} \\ $$$$ \\ $$

Commented by Mr X pcx last updated on 30/Apr/19

thanks sir

$${thanks}\:{sir} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com