Question Number 58774 by maxmathsup by imad last updated on 29/Apr/19
$${let}\:{f}\left({x}\right)\:=\int_{\frac{\pi}{\mathrm{3}}} ^{\frac{\pi}{\mathrm{2}}} \:\:\frac{{d}\theta}{\mathrm{1}+{xtan}\theta}\:\:\:{with}\:{x}\:{real} \\ $$$$\left.\mathrm{1}\right)\:{find}\:{a}\:{explicit}\:{form}\:{for}\:{f}\left({x}\right) \\ $$$$\left.\mathrm{2}\right)\:{determine}\:{also}\:{g}\left({x}\right)\:=\int_{\frac{\pi}{\mathrm{3}}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\frac{{tan}\theta}{\left(\mathrm{1}+{xtan}\theta\right)^{\mathrm{2}} }\:{d}\theta \\ $$$$\left.\mathrm{3}\right)\:{let}\:{U}_{{n}} \left({x}\right)\:={f}^{\left({n}\right)} \left({x}\right)\:\:{give}\:{U}_{{n}} \left({x}\right)\:{at}\:{form}\:{of}\:{integral}. \\ $$$$\left.\mathrm{4}\right)\:{calculate}\:\int_{\frac{\pi}{\mathrm{3}}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\frac{{d}\theta}{\mathrm{1}+\mathrm{2}{tan}\theta}\:\:{and}\:\:\int_{\frac{\pi}{\mathrm{3}}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\frac{{tan}\theta\:{d}\theta}{\left(\mathrm{1}+\mathrm{2}{tan}\theta\right)^{\mathrm{2}} } \\ $$
Commented by maxmathsup by imad last updated on 01/May/19
![1) we have f(x) =∫_(π/3) ^(π/2) (dθ/(1+x ((2tan((θ/2)))/(1−tan^2 ((θ/2)))))) =_(tan((θ/2))=t) ∫_(1/(√3)) ^1 (1/(1+((2xt)/(1−t^2 )))) ((2dt)/(1+t^2 )) = ∫_(1/(√3)) ^1 ((2(1−t^2 )dt)/((1−t^2 +2xt)(1+t^2 ))) =∫_(1/(√3)) ^1 ((2(t^2 −1))/((t^2 −2xt−1)(t^2 +1))) dt roots of t^2 −2xt −1 →Δ^′ =x^2 +1⇒ t_1 =x+(√(1+x^2 )) and t_2 =x−(√(1+x^2 )) let decompose F(t) =((2(t^2 −1))/((t^2 −2xt −1)(t^2 +1))) ⇒F(t) =(a/(t−t_1 )) +(b/(t−t_2 )) +((ct +d)/(t^2 +1)) =((2(t^2 −1))/((t−t_1 )(t−t_2 )(t^2 +1))) a =lim_(t→t_1 ) (t−t_1 )F(t) =((2(t_1 ^2 −1))/((t_1 −t_2 )(t_1 ^2 +1))) =((2(t_1 ^2 −1))/(2(√(1+x^2 ))(t_1 ^2 +1))) b =lim_(t→t_2 ) (t−t_2 )F(t) =((2(t_2 ^2 −1))/(−2(√(1+x^2 ))(t_2 ^2 +1))) lim_(t→+∞) tF(t) =0 =a+b +c ⇒c =−a−b ⇒F(t) =(a/(t−t_1 )) +(b/(t−t_2 )) +(((−a−b)t +d)/(t^2 +1)) F(0) =2 =−(a/t_1 ) −(b/t_2 ) +d ⇒d =(a/t_1 ) +(b/t_2 ) +2 ⇒ ∫_(1/(√3)) ^1 F(t)dt =a∫_(1/(√3)) ^1 (dt/(t−t_1 )) +b ∫_(1/(√3)) ^1 (dt/(t−t_2 )) −((a+b)/2) ∫_(1/(√3)) ^1 ((2dt)/(t^2 +1)) +d ∫_(1/(√3)) ^1 (dt/(t^2 +1)) =[aln∣t−t_1 ∣ +bln∣t−t_2 ∣]_(1/(√3)) ^1 −((a+b)/2)[ln(t^2 +1)]_(1/(√3)) ^1 + d [arctan(t)]_(1/(√3)) ^1 =aln∣1−t_1 ∣+bln∣1−t_2 ∣−aln∣(1/(√3)) −t_1 ∣−bln∣(1/(√3))−t_2 ∣−((a+b)/2){ln(2)−ln((4/3))} +d {(π/4) −(π/6)}=aln∣((1−t_1 )/((1/(√3))−t_1 ))∣ +bln∣((1−t_2 )/((1/(√3))−t_2 ))∣ −((a+b)/2)(ln(3)−ln(2)) +((πd)/(12)) a =((2( (x+(√(1+x^2 )))^2 −1))/(2(√(1+x^2 ))( (x+(√(1+x^2 )))^2 +1))) =(({ x^2 +2x(√(1+x^2 )) + x^2 })/((√(1+x^2 ))(x^2 +2x(√(1+x^2 )) +x^2 +2))) =(({x^2 +x(√(1+x^2 ))})/((√(1+x^2 )){x^2 +x(√(1+x^2 +1})))) b =((2( (x−(√(1+x^2 )))^2 −1))/(−2(√(1+x^2 ))( (x−(√(1+x^2 )))^2 +1))) =− ((x^2 −2x(√(1+x^2 )) +x^2 )/((√(1+x^2 ))(x^2 −2x(√(1+x^2 )) +x^2 +2))) =−(((x^2 −x(√(1+x^2 ))))/((√(1+x^2 ))( x^2 −x(√(1+x^2 +1))+1))) ....be continued....](Q58883.png)
$$\left.\mathrm{1}\right)\:{we}\:{have}\:{f}\left({x}\right)\:=\int_{\frac{\pi}{\mathrm{3}}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\frac{{d}\theta}{\mathrm{1}+{x}\:\frac{\mathrm{2}{tan}\left(\frac{\theta}{\mathrm{2}}\right)}{\mathrm{1}−{tan}^{\mathrm{2}} \left(\frac{\theta}{\mathrm{2}}\right)}}\:=_{{tan}\left(\frac{\theta}{\mathrm{2}}\right)={t}} \:\:\:\:\:\int_{\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}} ^{\mathrm{1}} \:\:\:\:\frac{\mathrm{1}}{\mathrm{1}+\frac{\mathrm{2}{xt}}{\mathrm{1}−{t}^{\mathrm{2}} }}\:\frac{\mathrm{2}{dt}}{\mathrm{1}+{t}^{\mathrm{2}} } \\ $$$$=\:\int_{\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}} ^{\mathrm{1}} \:\:\:\:\:\frac{\mathrm{2}\left(\mathrm{1}−{t}^{\mathrm{2}} \right){dt}}{\left(\mathrm{1}−{t}^{\mathrm{2}} \:+\mathrm{2}{xt}\right)\left(\mathrm{1}+{t}^{\mathrm{2}} \right)}\:=\int_{\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}} ^{\mathrm{1}} \:\:\:\frac{\mathrm{2}\left({t}^{\mathrm{2}} −\mathrm{1}\right)}{\left({t}^{\mathrm{2}} −\mathrm{2}{xt}−\mathrm{1}\right)\left({t}^{\mathrm{2}} \:+\mathrm{1}\right)}\:{dt}\:\:{roots}\:{of} \\ $$$${t}^{\mathrm{2}} −\mathrm{2}{xt}\:−\mathrm{1}\:\rightarrow\Delta^{'} ={x}^{\mathrm{2}} \:+\mathrm{1}\Rightarrow\:{t}_{\mathrm{1}} ={x}+\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }\:\:{and}\:{t}_{\mathrm{2}} ={x}−\sqrt{\mathrm{1}+{x}^{\mathrm{2}} \:\:}\:{let}\:{decompose} \\ $$$${F}\left({t}\right)\:=\frac{\mathrm{2}\left({t}^{\mathrm{2}} −\mathrm{1}\right)}{\left({t}^{\mathrm{2}} −\mathrm{2}{xt}\:−\mathrm{1}\right)\left({t}^{\mathrm{2}} +\mathrm{1}\right)}\:\Rightarrow{F}\left({t}\right)\:=\frac{{a}}{{t}−{t}_{\mathrm{1}} }\:+\frac{{b}}{{t}−{t}_{\mathrm{2}} }\:+\frac{{ct}\:+{d}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:=\frac{\mathrm{2}\left({t}^{\mathrm{2}} −\mathrm{1}\right)}{\left({t}−{t}_{\mathrm{1}} \right)\left({t}−{t}_{\mathrm{2}} \right)\left({t}^{\mathrm{2}} \:+\mathrm{1}\right)} \\ $$$${a}\:={lim}_{{t}\rightarrow{t}_{\mathrm{1}} } \left({t}−{t}_{\mathrm{1}} \right){F}\left({t}\right)\:=\frac{\mathrm{2}\left({t}_{\mathrm{1}} ^{\mathrm{2}} −\mathrm{1}\right)}{\left({t}_{\mathrm{1}} −{t}_{\mathrm{2}} \right)\left({t}_{\mathrm{1}} ^{\mathrm{2}} \:+\mathrm{1}\right)}\:=\frac{\mathrm{2}\left({t}_{\mathrm{1}} ^{\mathrm{2}} −\mathrm{1}\right)}{\mathrm{2}\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }\left({t}_{\mathrm{1}} ^{\mathrm{2}} \:+\mathrm{1}\right)} \\ $$$${b}\:={lim}_{{t}\rightarrow{t}_{\mathrm{2}} } \left({t}−{t}_{\mathrm{2}} \right){F}\left({t}\right)\:=\frac{\mathrm{2}\left({t}_{\mathrm{2}} ^{\mathrm{2}} −\mathrm{1}\right)}{−\mathrm{2}\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }\left({t}_{\mathrm{2}} ^{\mathrm{2}} \:+\mathrm{1}\right)} \\ $$$${lim}_{{t}\rightarrow+\infty} {tF}\left({t}\right)\:=\mathrm{0}\:={a}+{b}\:+{c}\:\Rightarrow{c}\:=−{a}−{b}\:\Rightarrow{F}\left({t}\right)\:=\frac{{a}}{{t}−{t}_{\mathrm{1}} }\:+\frac{{b}}{{t}−{t}_{\mathrm{2}} }\:+\frac{\left(−{a}−{b}\right){t}\:+{d}}{{t}^{\mathrm{2}} \:+\mathrm{1}} \\ $$$${F}\left(\mathrm{0}\right)\:=\mathrm{2}\:=−\frac{{a}}{{t}_{\mathrm{1}} }\:−\frac{{b}}{{t}_{\mathrm{2}} }\:+{d}\:\Rightarrow{d}\:=\frac{{a}}{{t}_{\mathrm{1}} }\:+\frac{{b}}{{t}_{\mathrm{2}} }\:+\mathrm{2}\:\Rightarrow \\ $$$$\int_{\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}} ^{\mathrm{1}} \:{F}\left({t}\right){dt}\:={a}\int_{\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}} ^{\mathrm{1}} \:\:\frac{{dt}}{{t}−{t}_{\mathrm{1}} }\:+{b}\:\int_{\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}} ^{\mathrm{1}} \:\:\frac{{dt}}{{t}−{t}_{\mathrm{2}} }\:−\frac{{a}+{b}}{\mathrm{2}}\:\int_{\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}} ^{\mathrm{1}} \:\:\frac{\mathrm{2}{dt}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:+{d}\:\int_{\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}} ^{\mathrm{1}} \:\:\frac{{dt}}{{t}^{\mathrm{2}} \:+\mathrm{1}} \\ $$$$=\left[{aln}\mid{t}−{t}_{\mathrm{1}} \mid\:+{bln}\mid{t}−{t}_{\mathrm{2}} \mid\right]_{\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}} ^{\mathrm{1}} \:−\frac{{a}+{b}}{\mathrm{2}}\left[{ln}\left({t}^{\mathrm{2}} \:+\mathrm{1}\right)\right]_{\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}} ^{\mathrm{1}} \:\:+\:{d}\:\left[{arctan}\left({t}\right)\right]_{\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}} ^{\mathrm{1}} \\ $$$$={aln}\mid\mathrm{1}−{t}_{\mathrm{1}} \mid+{bln}\mid\mathrm{1}−{t}_{\mathrm{2}} \mid−{aln}\mid\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}\:−{t}_{\mathrm{1}} \mid−{bln}\mid\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}−{t}_{\mathrm{2}} \mid−\frac{{a}+{b}}{\mathrm{2}}\left\{{ln}\left(\mathrm{2}\right)−{ln}\left(\frac{\mathrm{4}}{\mathrm{3}}\right)\right\} \\ $$$$+{d}\:\left\{\frac{\pi}{\mathrm{4}}\:−\frac{\pi}{\mathrm{6}}\right\}={aln}\mid\frac{\mathrm{1}−{t}_{\mathrm{1}} }{\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}−{t}_{\mathrm{1}} }\mid\:+{bln}\mid\frac{\mathrm{1}−{t}_{\mathrm{2}} }{\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}−{t}_{\mathrm{2}} }\mid\:−\frac{{a}+{b}}{\mathrm{2}}\left({ln}\left(\mathrm{3}\right)−{ln}\left(\mathrm{2}\right)\right)\:+\frac{\pi{d}}{\mathrm{12}} \\ $$$${a}\:=\frac{\mathrm{2}\left(\:\left({x}+\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }\right)^{\mathrm{2}} −\mathrm{1}\right)}{\mathrm{2}\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }\left(\:\left({x}+\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }\right)^{\mathrm{2}} \:+\mathrm{1}\right)}\:=\frac{\left\{\:{x}^{\mathrm{2}} \:+\mathrm{2}{x}\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }\:+\:{x}^{\mathrm{2}} \right\}}{\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }\left({x}^{\mathrm{2}} \:+\mathrm{2}{x}\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }\:+{x}^{\mathrm{2}} \:+\mathrm{2}\right)} \\ $$$$=\frac{\left\{{x}^{\mathrm{2}} \:+{x}\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }\right\}}{\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }\left\{{x}^{\mathrm{2}} \:+{x}\sqrt{\left.\mathrm{1}+{x}^{\mathrm{2}} \:+\mathrm{1}\right\}}\right.} \\ $$$${b}\:=\frac{\mathrm{2}\left(\:\left({x}−\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }\right)^{\mathrm{2}} −\mathrm{1}\right)}{−\mathrm{2}\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }\left(\:\left({x}−\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }\right)^{\mathrm{2}} \:+\mathrm{1}\right)}\:=−\:\frac{{x}^{\mathrm{2}} −\mathrm{2}{x}\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }\:+{x}^{\mathrm{2}} }{\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }\left({x}^{\mathrm{2}} −\mathrm{2}{x}\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }\:+{x}^{\mathrm{2}} \:+\mathrm{2}\right)} \\ $$$$=−\frac{\left({x}^{\mathrm{2}} −{x}\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }\right)}{\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }\left(\:{x}^{\mathrm{2}} −{x}\sqrt{\mathrm{1}+{x}^{\mathrm{2}} \:+\mathrm{1}}+\mathrm{1}\right)}\:\:\:....{be}\:{continued}.... \\ $$
Commented by maxmathsup by imad last updated on 01/May/19
$$\left.\mathrm{2}\right)\:{we}\:{have}\:{f}^{'} \left({x}\right)\:=−\int_{\frac{\pi}{\mathrm{3}}} ^{\frac{\pi}{\mathrm{2}}} \:\:\frac{{tan}\theta\:{d}\theta}{\left(\mathrm{1}+{xtan}\theta\right)^{\mathrm{2}} }\:=−{g}\left({x}\right)\:\Rightarrow{g}\left({x}\right)=−{f}^{'} \left({x}\right)\:\:{rest}\:{to}\:{calculate} \\ $$$${f}^{'} \left({x}\right) \\ $$$$\left.\mathrm{3}\right)\:{we}\:{have}\:{f}^{\left(\mathrm{1}\right)} \left({x}\right)\:=\left(−\mathrm{1}\right)\:\int_{\frac{\pi}{\mathrm{3}}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\frac{{tan}\theta}{\left(\mathrm{1}+{xtan}\theta\right)^{\mathrm{2}} }\:{d}\theta\:\Rightarrow{f}^{\left(\mathrm{2}\right)} \left({x}\right)\:=\left(−\mathrm{1}\right)^{\mathrm{2}} \:\int_{\frac{\pi}{\mathrm{3}}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{2}\left(\mathrm{1}+{xtan}\theta\right)}{\left(\mathrm{1}+{xtan}\theta\right)^{\mathrm{4}} }\:{tan}^{\mathrm{2}} \theta\:{d}\theta \\ $$$$=\mathrm{2}.\left(−\mathrm{1}\right)^{\mathrm{2}} \:\int_{\frac{\pi}{\mathrm{3}}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\:\frac{{tan}^{\mathrm{2}} \theta}{\left(\mathrm{1}+{xtan}\theta\right)^{\mathrm{3}} }\:{d}\theta\:\:\:{let}\:{suppose}\:{f}^{\left({n}\right)} \left({x}\right)\:=\left(−\mathrm{1}\right)^{{n}} {n}!\:\int_{\frac{\pi}{\mathrm{3}}} ^{\frac{\pi}{\mathrm{2}}} \:\:\frac{{tan}^{{n}} \theta}{\left(\mathrm{1}+{xtan}\theta\right)^{{n}+\mathrm{1}} }\:{d}\theta \\ $$$$\Rightarrow{f}^{\left({n}+\mathrm{1}\right)} \left({x}\right)\:=\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} {n}!\:\int_{\frac{\pi}{\mathrm{3}}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\frac{\left({n}+\mathrm{1}\right){tan}\theta\:\left(\mathrm{1}+{xtan}\theta\right)^{{n}} {tan}^{{n}} \theta}{\left(\mathrm{1}+{xtan}\theta\right)^{\mathrm{2}{n}+\mathrm{2}} }\:{d}\theta \\ $$$$=\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} \left({n}+\mathrm{1}\right)!\:\int_{\frac{\pi}{\mathrm{3}}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\:\frac{{tan}^{{n}+\mathrm{1}} \theta}{\left(\mathrm{1}+{xtan}\theta\right)^{{n}+\mathrm{2}} }\:{d}\theta\:\:\:\:{so}\:{the}\:{result}\:{is}\:{proved}\:. \\ $$
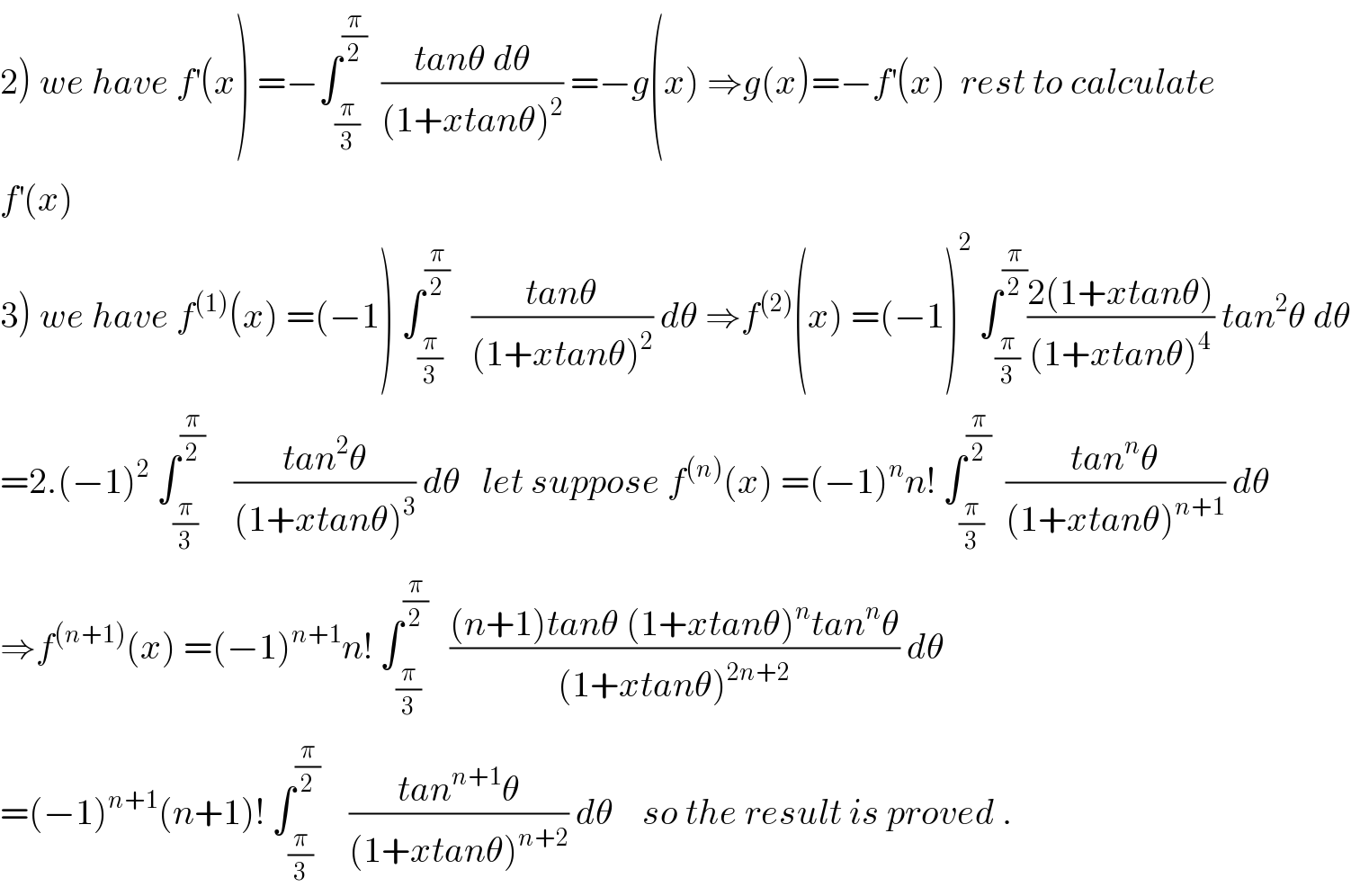
Commented by maxmathsup by imad last updated on 01/May/19
![4) let A =∫_(π/3) ^(π/2) (dθ/(1+2tanθ)) ⇒ A =∫_(π/3) ^(π/2) (dθ/(1+2 ((2tan((θ/2)))/(1−tan^2 ((θ/2)))))) A =_(tan((θ/2)) =t) ∫_(1/(√3)) ^1 (1/(1+((4t)/(1−t^2 )))) ((2dt)/(1+t^2 )) =∫_(1/(√3)) ^1 ((2(1−t^2 ))/((1−t^2 +4t)(1+t^2 ))) dt =∫_(1/(√3)) ^1 ((2(t^2 −1))/((t^2 −4t−1)(t^2 +1))) dt let decompose F(t) =((2(t^2 −1))/((t^2 −4t−1)(t^2 +1))) rootf t^2 −4t−1 →Δ^′ =4+1 =5 ⇒t_1 =2+(√5) and t_2 =2−(√5) ⇒ F(t) = (a/(t−t_1 )) +(b/(t−t_2 )) +((ct +d)/(t^2 +1)) =((2(t^2 −1))/((t−t_1 )(t−t_2 )(t^2 +1))) a =lim_(t→t_1 ) (t−t_1 )F(t) = ((2(t_1 ^2 −1))/(2(√5)(t_1 ^2 +1))) =(((2+(√5))^2 −1)/((√5)( (2+(√5))^2 +1))) =((4 +4(√5)+5−1)/((√5)( 4 +4(√5) +5+1))) =((8 +4(√5))/((√5)(10 +4(√5)))) =((4 +2(√5))/((√5)( 5 +2(√5)))) b =lim_(t→t_2 ) (t−t_2 )F(t) =((2(t_2 ^2 −1))/(−2(√5)( t_2 ^2 +1))) =−(((2−(√5))^2 −1)/((√5)((2−(√5))^2 +1))) =−((4−4(√5)+5 −1)/((√5)( 4−4(√5) +5 +1))) =−((8−4(√5))/((√5)(10−4(√5)))) =−((4−2(√5))/((√5)(5 −2(√5)))) =((−4 +2(√5))/((√5)( 5−2(√5)))) lim_(t→+∞) tF(t) =0 =a+b +c ⇒c =−(a+b) F(0) =−(a/t_1 ) −(b/t_2 ) +d =2 ⇒d =2 +(a/t_1 ) +(b/t_2 ) ⇒ ∫_(1/(√3)) ^1 F(t)dt =a ∫_(1/(√3)) ^1 (dt/(t−t_1 )) +b ∫_(1/(√3)) ^1 (dt/(t−t_2 )) +(c/2) ∫_(1/(√3)) ^1 ((2t)/(t^2 +1)) dt +d ∫_(1/(√3)) ^1 (dt/(1+t^2 )) =[aln∣t−t_1 ∣ +bln∣t−t_2 ∣ +(c/2)ln(t^2 +1) +darctan(t)]_(1/(√3)) ^1 =a ln∣1−t_1 ∣ +bln∣1−t_2 ∣ +(c/2)ln(2) + (dπ/4) −aln∣(1/(√3)) −t_1 ∣−b ln∣(1/(√3)) −t_2 ∣ −(c/2) ln((4/3))−(dπ/6) ....](Q58951.png)
$$\left.\mathrm{4}\right)\:{let}\:{A}\:=\int_{\frac{\pi}{\mathrm{3}}} ^{\frac{\pi}{\mathrm{2}}} \:\:\frac{{d}\theta}{\mathrm{1}+\mathrm{2}{tan}\theta}\:\Rightarrow\:{A}\:\:=\int_{\frac{\pi}{\mathrm{3}}} ^{\frac{\pi}{\mathrm{2}}} \:\:\frac{{d}\theta}{\mathrm{1}+\mathrm{2}\:\frac{\mathrm{2}{tan}\left(\frac{\theta}{\mathrm{2}}\right)}{\mathrm{1}−{tan}^{\mathrm{2}} \left(\frac{\theta}{\mathrm{2}}\right)}} \\ $$$${A}\:=_{{tan}\left(\frac{\theta}{\mathrm{2}}\right)\:={t}} \:\:\:\:\:\:\:\int_{\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}} ^{\mathrm{1}} \:\:\:\:\frac{\mathrm{1}}{\mathrm{1}+\frac{\mathrm{4}{t}}{\mathrm{1}−{t}^{\mathrm{2}} }}\:\frac{\mathrm{2}{dt}}{\mathrm{1}+{t}^{\mathrm{2}} }\:=\int_{\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}} ^{\mathrm{1}} \:\:\frac{\mathrm{2}\left(\mathrm{1}−{t}^{\mathrm{2}} \right)}{\left(\mathrm{1}−{t}^{\mathrm{2}} \:+\mathrm{4}{t}\right)\left(\mathrm{1}+{t}^{\mathrm{2}} \right)}\:{dt} \\ $$$$=\int_{\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}} ^{\mathrm{1}} \:\:\:\frac{\mathrm{2}\left({t}^{\mathrm{2}} −\mathrm{1}\right)}{\left({t}^{\mathrm{2}} −\mathrm{4}{t}−\mathrm{1}\right)\left({t}^{\mathrm{2}} \:+\mathrm{1}\right)}\:{dt}\:\:\:{let}\:{decompose}\:{F}\left({t}\right)\:=\frac{\mathrm{2}\left({t}^{\mathrm{2}} −\mathrm{1}\right)}{\left({t}^{\mathrm{2}} −\mathrm{4}{t}−\mathrm{1}\right)\left({t}^{\mathrm{2}} \:+\mathrm{1}\right)} \\ $$$${rootf}\:{t}^{\mathrm{2}} −\mathrm{4}{t}−\mathrm{1}\:\rightarrow\Delta^{'} =\mathrm{4}+\mathrm{1}\:=\mathrm{5}\:\Rightarrow{t}_{\mathrm{1}} =\mathrm{2}+\sqrt{\mathrm{5}}\:\:{and}\:{t}_{\mathrm{2}} =\mathrm{2}−\sqrt{\mathrm{5}}\:\:\Rightarrow \\ $$$${F}\left({t}\right)\:=\:\frac{{a}}{{t}−{t}_{\mathrm{1}} }\:+\frac{{b}}{{t}−{t}_{\mathrm{2}} }\:+\frac{{ct}\:+{d}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:=\frac{\mathrm{2}\left({t}^{\mathrm{2}} −\mathrm{1}\right)}{\left({t}−{t}_{\mathrm{1}} \right)\left({t}−{t}_{\mathrm{2}} \right)\left({t}^{\mathrm{2}} \:+\mathrm{1}\right)} \\ $$$${a}\:={lim}_{{t}\rightarrow{t}_{\mathrm{1}} } \left({t}−{t}_{\mathrm{1}} \right){F}\left({t}\right)\:=\:\frac{\mathrm{2}\left({t}_{\mathrm{1}} ^{\mathrm{2}} −\mathrm{1}\right)}{\mathrm{2}\sqrt{\mathrm{5}}\left({t}_{\mathrm{1}} ^{\mathrm{2}} \:+\mathrm{1}\right)}\:=\frac{\left(\mathrm{2}+\sqrt{\mathrm{5}}\right)^{\mathrm{2}} −\mathrm{1}}{\sqrt{\mathrm{5}}\left(\:\left(\mathrm{2}+\sqrt{\mathrm{5}}\right)^{\mathrm{2}} \:+\mathrm{1}\right)} \\ $$$$=\frac{\mathrm{4}\:+\mathrm{4}\sqrt{\mathrm{5}}+\mathrm{5}−\mathrm{1}}{\sqrt{\mathrm{5}}\left(\:\mathrm{4}\:+\mathrm{4}\sqrt{\mathrm{5}}\:+\mathrm{5}+\mathrm{1}\right)}\:=\frac{\mathrm{8}\:+\mathrm{4}\sqrt{\mathrm{5}}}{\sqrt{\mathrm{5}}\left(\mathrm{10}\:+\mathrm{4}\sqrt{\mathrm{5}}\right)}\:=\frac{\mathrm{4}\:+\mathrm{2}\sqrt{\mathrm{5}}}{\sqrt{\mathrm{5}}\left(\:\mathrm{5}\:+\mathrm{2}\sqrt{\mathrm{5}}\right)} \\ $$$${b}\:={lim}_{{t}\rightarrow{t}_{\mathrm{2}} } \:\left({t}−{t}_{\mathrm{2}} \right){F}\left({t}\right)\:=\frac{\mathrm{2}\left({t}_{\mathrm{2}} ^{\mathrm{2}} −\mathrm{1}\right)}{−\mathrm{2}\sqrt{\mathrm{5}}\left(\:{t}_{\mathrm{2}} ^{\mathrm{2}} \:+\mathrm{1}\right)}\:=−\frac{\left(\mathrm{2}−\sqrt{\mathrm{5}}\right)^{\mathrm{2}} −\mathrm{1}}{\sqrt{\mathrm{5}}\left(\left(\mathrm{2}−\sqrt{\mathrm{5}}\right)^{\mathrm{2}} \:+\mathrm{1}\right)} \\ $$$$=−\frac{\mathrm{4}−\mathrm{4}\sqrt{\mathrm{5}}+\mathrm{5}\:−\mathrm{1}}{\sqrt{\mathrm{5}}\left(\:\mathrm{4}−\mathrm{4}\sqrt{\mathrm{5}}\:+\mathrm{5}\:+\mathrm{1}\right)}\:=−\frac{\mathrm{8}−\mathrm{4}\sqrt{\mathrm{5}}}{\sqrt{\mathrm{5}}\left(\mathrm{10}−\mathrm{4}\sqrt{\mathrm{5}}\right)}\:=−\frac{\mathrm{4}−\mathrm{2}\sqrt{\mathrm{5}}}{\sqrt{\mathrm{5}}\left(\mathrm{5}\:−\mathrm{2}\sqrt{\mathrm{5}}\right)}\:=\frac{−\mathrm{4}\:+\mathrm{2}\sqrt{\mathrm{5}}}{\sqrt{\mathrm{5}}\left(\:\mathrm{5}−\mathrm{2}\sqrt{\mathrm{5}}\right)} \\ $$$${lim}_{{t}\rightarrow+\infty} \:\:{tF}\left({t}\right)\:=\mathrm{0}\:={a}+{b}\:+{c}\:\Rightarrow{c}\:=−\left({a}+{b}\right) \\ $$$${F}\left(\mathrm{0}\right)\:=−\frac{{a}}{{t}_{\mathrm{1}} }\:−\frac{{b}}{{t}_{\mathrm{2}} }\:+{d}\:=\mathrm{2}\:\Rightarrow{d}\:=\mathrm{2}\:+\frac{{a}}{{t}_{\mathrm{1}} }\:+\frac{{b}}{{t}_{\mathrm{2}} }\:\Rightarrow \\ $$$$\int_{\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}} ^{\mathrm{1}} \:\:{F}\left({t}\right){dt}\:={a}\:\int_{\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}} ^{\mathrm{1}} \:\:\frac{{dt}}{{t}−{t}_{\mathrm{1}} }\:+{b}\:\int_{\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}} ^{\mathrm{1}} \:\:\frac{{dt}}{{t}−{t}_{\mathrm{2}} }\:+\frac{{c}}{\mathrm{2}}\:\int_{\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}} ^{\mathrm{1}} \:\frac{\mathrm{2}{t}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:{dt}\:\:+{d}\:\int_{\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}} ^{\mathrm{1}} \:\:\frac{{dt}}{\mathrm{1}+{t}^{\mathrm{2}} } \\ $$$$=\left[{aln}\mid{t}−{t}_{\mathrm{1}} \mid\:+{bln}\mid{t}−{t}_{\mathrm{2}} \mid\:+\frac{{c}}{\mathrm{2}}{ln}\left({t}^{\mathrm{2}} \:+\mathrm{1}\right)\:+{darctan}\left({t}\right)\right]_{\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}} ^{\mathrm{1}} \\ $$$$={a}\:{ln}\mid\mathrm{1}−{t}_{\mathrm{1}} \mid\:+{bln}\mid\mathrm{1}−{t}_{\mathrm{2}} \mid\:+\frac{{c}}{\mathrm{2}}{ln}\left(\mathrm{2}\right)\:+\:\frac{{d}\pi}{\mathrm{4}}\:−{aln}\mid\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}\:−{t}_{\mathrm{1}} \mid−{b}\:{ln}\mid\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}\:−{t}_{\mathrm{2}} \mid \\ $$$$−\frac{{c}}{\mathrm{2}}\:{ln}\left(\frac{\mathrm{4}}{\mathrm{3}}\right)−\frac{{d}\pi}{\mathrm{6}}\:.... \\ $$