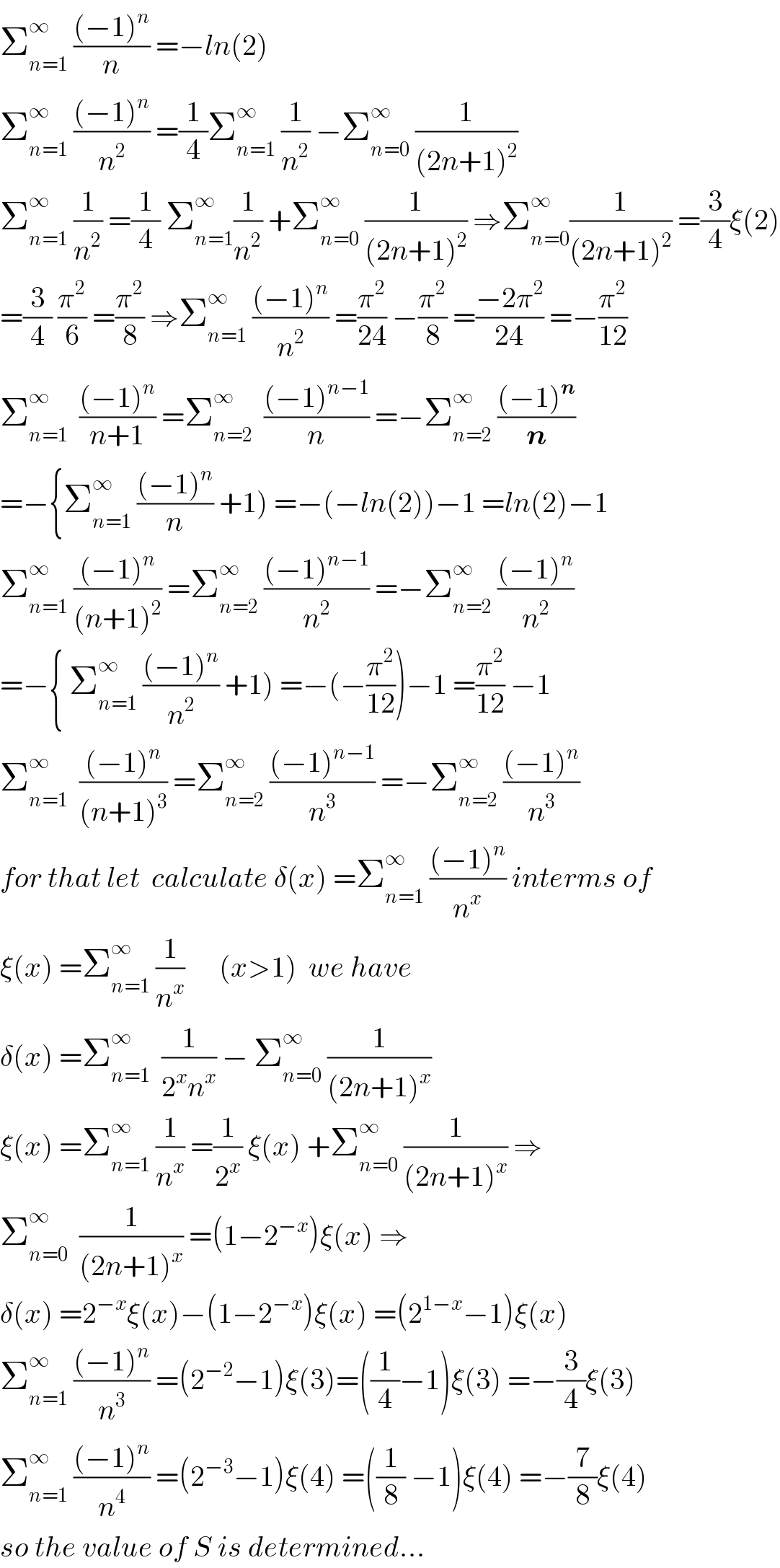Question and Answers Forum
Previous in Relation and Functions Next in Relation and Functions
Question Number 60499 by abdo mathsup 649 cc last updated on 21/May/19
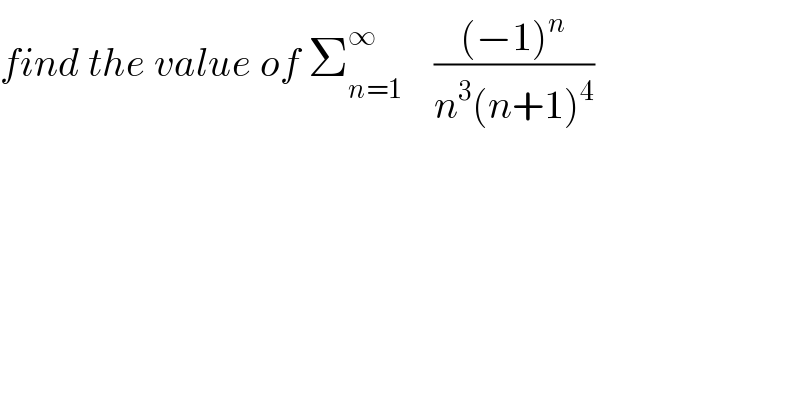
Commented by maxmathsup by imad last updated on 30/May/19

Commented by maxmathsup by imad last updated on 30/May/19