
Question Number 60506 by prof Abdo imad last updated on 21/May/19
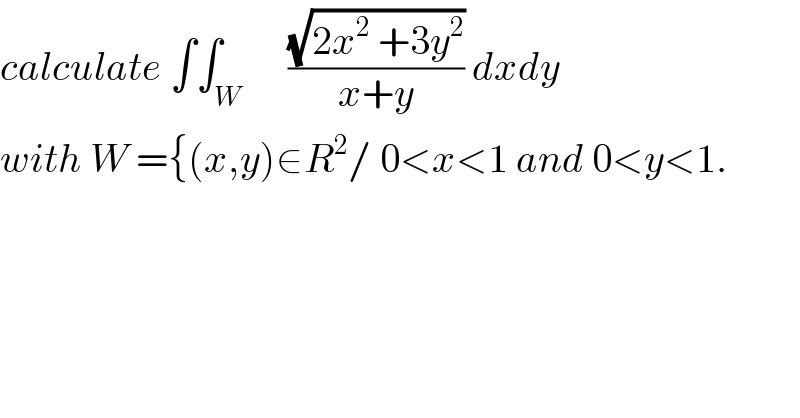
$${calculate}\:\int\int_{{W}} \:\:\:\:\:\frac{\sqrt{\mathrm{2}{x}^{\mathrm{2}} \:+\mathrm{3}{y}^{\mathrm{2}} }}{{x}+{y}}\:{dxdy} \\ $$ $${with}\:{W}\:=\left\{\left({x},{y}\right)\in{R}^{\mathrm{2}} /\:\mathrm{0}<{x}<\mathrm{1}\:{and}\:\mathrm{0}<{y}<\mathrm{1}.\right. \\ $$
Commented byMr X pcx last updated on 23/May/19
![let consider the diffromorphism x=(r/(√2)) cosθ and y =(r/(√3)) sinθ 0<x^2 +y^2 <2 ⇒0<(r^2 /2) +(r^2 /3)<2 ⇒ 0<(5/6) r^2 <2 ⇒0<r^2 <((12)/5) ⇒0<r<((2(√3))/(√5)) M_j = ((( (∂ϕ_1 /∂r) (∂ϕ_1 /∂θ))),(((∂ϕ_2 /∂r) (∂ϕ_2 /∂θ))) ) = ((((1/(√2))cosθ −(r/(√2))sinθ)),(((1/(√3))sinθ (r/(√3))cosθ)) ) det(M_j ) =(r/((√2)(√3))) ⇒ I = ∫∫_(0<r<((2(√3))/(√5)) and 0<θ<(π/2)) (r/((r/(√2))cosθ +(r/(√3))sinθ))(1/(√6)) rdrdθ =∫_0 ^((2(√3))/(√5)) rdr ∫_0 ^(π/2) (dθ/((√3)cosθ +(√2)sinθ)) ∫_0 ^((2(√3))/(√5)) rdr =[(r^2 /2)]_0 ^((2(√3))/(√5)) =(1/2)(((4.3)/5)) =(6/5) ∫_0 ^(π/2) (dθ/((√3)cosθ +(√2)sinθ)) =_(tan((θ/2)) =x) =∫_0 ^1 ((2dx)/((1+x^2 ){ (√3)((1−x^2 )/(1+x^2 )) +(√2) ((2x)/(1+x^2 ))})) =∫_0 ^1 ((2dx)/((√3)−(√3)x^2 +2(√2)x)) =∫_0 ^1 ((−2dx)/((√3)x^2 −2(√2)x −(√3))) let drcompose F(x)=((−2)/((√3)x^2 −2(√2)x −(√3))) Δ^′ =2 +3 =5 ⇒x_1 =(((√2) +(√5))/(√3)) x_2 =(((√2)−(√5))/(√3)) ⇒ F(x) =((−2)/((√3)(x−x_1 )(x−x_2 ))) =((−2)/((√3)(x_1 −x_2 ))){ (1/(x−x_1 )) −(1/(x−x_2 ))} =((−2)/((√3)(((2(√5))/(√3))))){ (1/(x−x_1 )) −(1/(x−x_2 ))} =−(1/(√5)){ ....} ⇒ ∫_0 ^1 F(x)dx?=(1/(√5))[ln∣x−x_2 ∣−ln∣x−x_1 ∣] =(1/(√5))[ln∣((x−x_2 )/(x−x_1 ))∣]_0 ^1 =(1/(√5))( ln(((1−(((√2)−(√5))/(√3)))/(1−(((√2) +(√5))/(√3)))))−ln∣(((√2)−(√5))/((√2) +(√5)))∣) =(1/(√5))( ln∣(((√3)−(√2)+(√5))/((√3)−(√2) +(√5)))∣ −ln((((√5)−(√2))/((√5) +(√2)))) so the vslue of I is known.](Q60657.png)
$${let}\:{consider}\:{the}\:{diffromorphism} \\ $$ $${x}=\frac{{r}}{\sqrt{\mathrm{2}}}\:{cos}\theta\:\:{and}\:{y}\:=\frac{{r}}{\sqrt{\mathrm{3}}}\:{sin}\theta \\ $$ $$\mathrm{0}<{x}^{\mathrm{2}} \:+{y}^{\mathrm{2}} <\mathrm{2}\:\Rightarrow\mathrm{0}<\frac{{r}^{\mathrm{2}} }{\mathrm{2}}\:+\frac{{r}^{\mathrm{2}} }{\mathrm{3}}<\mathrm{2}\:\Rightarrow \\ $$ $$\mathrm{0}<\frac{\mathrm{5}}{\mathrm{6}}\:{r}^{\mathrm{2}} <\mathrm{2}\:\Rightarrow\mathrm{0}<{r}^{\mathrm{2}} <\frac{\mathrm{12}}{\mathrm{5}}\:\Rightarrow\mathrm{0}<{r}<\frac{\mathrm{2}\sqrt{\mathrm{3}}}{\sqrt{\mathrm{5}}} \\ $$ $${M}_{{j}} =\begin{pmatrix}{\:\:\frac{\partial\varphi_{\mathrm{1}} }{\partial{r}}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\frac{\partial\varphi_{\mathrm{1}} }{\partial\theta}}\\{\frac{\partial\varphi_{\mathrm{2}} }{\partial{r}}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\frac{\partial\varphi_{\mathrm{2}} }{\partial\theta}}\end{pmatrix} \\ $$ $$=\:\begin{pmatrix}{\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}{cos}\theta\:\:\:\:\:\:\:\:\:\:\:−\frac{{r}}{\sqrt{\mathrm{2}}}{sin}\theta}\\{\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}{sin}\theta\:\:\:\:\:\:\:\:\:\:\:\:\:\:\frac{{r}}{\sqrt{\mathrm{3}}}{cos}\theta}\end{pmatrix} \\ $$ $${det}\left({M}_{{j}} \right)\:=\frac{{r}}{\sqrt{\mathrm{2}}\sqrt{\mathrm{3}}}\:\:\:\Rightarrow \\ $$ $${I}\:=\:\int\int_{\mathrm{0}<{r}<\frac{\mathrm{2}\sqrt{\mathrm{3}}}{\sqrt{\mathrm{5}}}\:\:\:{and}\:\:\mathrm{0}<\theta<\frac{\pi}{\mathrm{2}}} \:\:\frac{{r}}{\frac{{r}}{\sqrt{\mathrm{2}}}{cos}\theta\:+\frac{{r}}{\sqrt{\mathrm{3}}}{sin}\theta}\frac{\mathrm{1}}{\sqrt{\mathrm{6}}}\:{rdrd}\theta \\ $$ $$=\int_{\mathrm{0}} ^{\frac{\mathrm{2}\sqrt{\mathrm{3}}}{\sqrt{\mathrm{5}}}} \:\:{rdr}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\frac{{d}\theta}{\sqrt{\mathrm{3}}{cos}\theta\:+\sqrt{\mathrm{2}}{sin}\theta} \\ $$ $$\int_{\mathrm{0}} ^{\frac{\mathrm{2}\sqrt{\mathrm{3}}}{\sqrt{\mathrm{5}}}} \:{rdr}\:=\left[\frac{{r}^{\mathrm{2}} }{\mathrm{2}}\right]_{\mathrm{0}} ^{\frac{\mathrm{2}\sqrt{\mathrm{3}}}{\sqrt{\mathrm{5}}}} \:=\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{\mathrm{4}.\mathrm{3}}{\mathrm{5}}\right)\:=\frac{\mathrm{6}}{\mathrm{5}} \\ $$ $$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\:\frac{{d}\theta}{\sqrt{\mathrm{3}}{cos}\theta\:+\sqrt{\mathrm{2}}{sin}\theta}\:=_{{tan}\left(\frac{\theta}{\mathrm{2}}\right)\:={x}} \\ $$ $$=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\frac{\mathrm{2}{dx}}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)\left\{\:\sqrt{\mathrm{3}}\frac{\mathrm{1}−{x}^{\mathrm{2}} }{\mathrm{1}+{x}^{\mathrm{2}} }\:+\sqrt{\mathrm{2}}\:\frac{\mathrm{2}{x}}{\mathrm{1}+{x}^{\mathrm{2}} }\right\}} \\ $$ $$=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{\mathrm{2}{dx}}{\sqrt{\mathrm{3}}−\sqrt{\mathrm{3}}{x}^{\mathrm{2}} \:+\mathrm{2}\sqrt{\mathrm{2}}{x}} \\ $$ $$=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\frac{−\mathrm{2}{dx}}{\sqrt{\mathrm{3}}{x}^{\mathrm{2}} \:−\mathrm{2}\sqrt{\mathrm{2}}{x}\:−\sqrt{\mathrm{3}}} \\ $$ $${let}\:{drcompose}\:{F}\left({x}\right)=\frac{−\mathrm{2}}{\sqrt{\mathrm{3}}{x}^{\mathrm{2}} −\mathrm{2}\sqrt{\mathrm{2}}{x}\:−\sqrt{\mathrm{3}}} \\ $$ $$\Delta^{'} \:=\mathrm{2}\:+\mathrm{3}\:=\mathrm{5}\:\Rightarrow{x}_{\mathrm{1}} =\frac{\sqrt{\mathrm{2}}\:+\sqrt{\mathrm{5}}}{\sqrt{\mathrm{3}}} \\ $$ $$\underset{\mathrm{2}} {{x}}=\frac{\sqrt{\mathrm{2}}−\sqrt{\mathrm{5}}}{\sqrt{\mathrm{3}}}\:\Rightarrow \\ $$ $${F}\left({x}\right)\:=\frac{−\mathrm{2}}{\sqrt{\mathrm{3}}\left({x}−{x}_{\mathrm{1}} \right)\left({x}−{x}_{\mathrm{2}} \right)} \\ $$ $$=\frac{−\mathrm{2}}{\sqrt{\mathrm{3}}\left({x}_{\mathrm{1}} −{x}_{\mathrm{2}} \right)}\left\{\:\frac{\mathrm{1}}{{x}−{x}_{\mathrm{1}} }\:−\frac{\mathrm{1}}{{x}−{x}_{\mathrm{2}} }\right\} \\ $$ $$=\frac{−\mathrm{2}}{\sqrt{\mathrm{3}}\left(\frac{\mathrm{2}\sqrt{\mathrm{5}}}{\sqrt{\mathrm{3}}}\right)}\left\{\:\frac{\mathrm{1}}{{x}−{x}_{\mathrm{1}} }\:−\frac{\mathrm{1}}{{x}−{x}_{\mathrm{2}} }\right\} \\ $$ $$=−\frac{\mathrm{1}}{\sqrt{\mathrm{5}}}\left\{\:....\right\}\:\Rightarrow \\ $$ $$\int_{\mathrm{0}} ^{\mathrm{1}} \:{F}\left({x}\right){dx}?=\frac{\mathrm{1}}{\sqrt{\mathrm{5}}}\left[{ln}\mid{x}−{x}_{\mathrm{2}} \mid−{ln}\mid{x}−{x}_{\mathrm{1}} \mid\right] \\ $$ $$=\frac{\mathrm{1}}{\sqrt{\mathrm{5}}}\left[{ln}\mid\frac{{x}−{x}_{\mathrm{2}} }{{x}−{x}_{\mathrm{1}} }\mid\right]_{\mathrm{0}} ^{\mathrm{1}} \\ $$ $$=\frac{\mathrm{1}}{\sqrt{\mathrm{5}}}\left(\:{ln}\left(\frac{\mathrm{1}−\frac{\sqrt{\mathrm{2}}−\sqrt{\mathrm{5}}}{\sqrt{\mathrm{3}}}}{\mathrm{1}−\frac{\sqrt{\mathrm{2}}\:+\sqrt{\mathrm{5}}}{\sqrt{\mathrm{3}}}}\right)−{ln}\mid\frac{\sqrt{\mathrm{2}}−\sqrt{\mathrm{5}}}{\sqrt{\mathrm{2}}\:+\sqrt{\mathrm{5}}}\mid\right) \\ $$ $$=\frac{\mathrm{1}}{\sqrt{\mathrm{5}}}\left(\:{ln}\mid\frac{\sqrt{\mathrm{3}}−\sqrt{\mathrm{2}}+\sqrt{\mathrm{5}}}{\sqrt{\mathrm{3}}−\sqrt{\mathrm{2}}\:+\sqrt{\mathrm{5}}}\mid\:−{ln}\left(\frac{\sqrt{\mathrm{5}}−\sqrt{\mathrm{2}}}{\sqrt{\mathrm{5}}\:+\sqrt{\mathrm{2}}}\right)\right. \\ $$ $${so}\:{the}\:{vslue}\:{of}\:\:{I}\:{is}\:{known}. \\ $$
