(x^4 −3x^2 +2x+1)/(x−1)


Question and Answers Forum

All Questions Topic List
Algebra Questions
Previous in All Question Next in All Question
Previous in Algebra Next in Algebra
Question Number 60854 by Askash last updated on 26/May/19

$(x^{4} - 3 x^{2} + 2 x + 1) / (x - 1)$
Commented by Askash last updated on 26/May/19

$i w a n t t h e b e s t m e t h o d .$
Commented by maxmathsup by imad last updated on 26/May/19

$l e t p (x) = x^{4} - 3 x^{2} + 2 x + 1 t h e b e s t m e t h o d i s t o d i v i d e p (x) b y x - 1$ $(u s i n g p p o p e r a t i o n) l e t u s e a n o t h e r m e t h o d w e h a v e d e g (x - 1) = 1 \Rightarrow$ $p (x) = (x - 1) (x^{3} + {a x}^{2} + b x + c) + d d = p (1) = 1 - 3 + 2 + 1 = 1 \Rightarrow$ $p (x) = (x - 1) (x^{3} + {a x}^{2} + b x + c) + 1 \Rightarrow$ $x^{4} + {a x}^{3} + {b x}^{2} + c x - x^{3} - {a x}^{2} - b x - c + 1 = x^{4} - 3 x^{2} + 2 x + 1 \Rightarrow$ $(a - 1) x^{3} + (b - a) x^{2} + (c - b) x - c = - 3 x^{2} + 2 x \Rightarrow$ $a - 1 = 0 a n d b - a = - 3 a n d c - b = 2 \Rightarrow a = 1, b = - 2, c = 0 \Rightarrow$ $Q (x) = x^{3} + x^{2} - 2 x a n d R = 1$
Commented by Tawa1 last updated on 26/May/19

$let x - 1 = 0, \Rightarrow x = 1$ $So, by remainder theorem$ $f (x) = x^{4} - {3 x}^{2} + 2 x + 1$ $f (1) = 1^{4} - 3 {(1)}^{2} + 2 (1) + 1$ $f (1) = 1 - 3 + 2 + 1$ $f (1) = 1$ $So, the remainder is 1 .$
Commented by Askash last updated on 26/May/19

$a n y e a s y t o f i n d r e m a i n d e r$
	Answered by ajfour last updated on 26/May/19
	$let x−1=t ⇒ x=t+1 ,then {(t+1)^4 −3(t+1)^2 +2(t+1)+1}/t =(t^4 +4t^3 +6t^2 +4t+1−3t^2 −6t−3 +2t+2+1)/t =t^3 +4t^2 +3t+(1/t) = (x−1){(x−1)^2 +4(x−1)+3}+(1/(x−1)) =x(x−1)(x+2)+(1/(x−1)) .$
	$l e t x - 1 = t \Rightarrow x = t + 1, t h e n$ ${{(t + 1)}^{4} - 3 {(t + 1)}^{2} + 2 (t + 1) + 1} / t$ $= (t^{4} + 4 t^{3} + 6 t^{2} + 4 t + 1 - 3 t^{2} - 6 t - 3$ $+ 2 t + 2 + 1) / t$ $= t^{3} + 4 t^{2} + 3 t + \frac{1}{t}$ $= (x - 1) {{(x - 1)}^{2} + 4 (x - 1) + 3} + \frac{1}{x - 1}$ $= x (x - 1) (x + 2) + \frac{1}{x - 1} .$
	Answered by Rasheed.Sindhi last updated on 26/May/19

	$S y n t h e t i c D i v i s i o n i s p e r h a p s t h e$ $e a s i e s t w a y w h e n d i v i s o r i s l i n e a r$ $a s i n t h i s c a s e .$ $P (x) = x^{4} + 0 x^{3} - 3 x^{2} + 2 x + 1$ $D (x) = x - 1$ $Q (x) : Q u o t i e n t \int$ $R : R e m a i n d e r$ $(\begin{matrix} 1) & 1 & 0 & - 3 & 2 & 1 \\ 1 & 1 & - 2 & 0 \\ - & - & - & - & - \\ 1 & 1 & - 2 & 0 & [1 \end{matrix})$ $Q (x) = x^{3} + x^{2} - 2 x, R = 1$
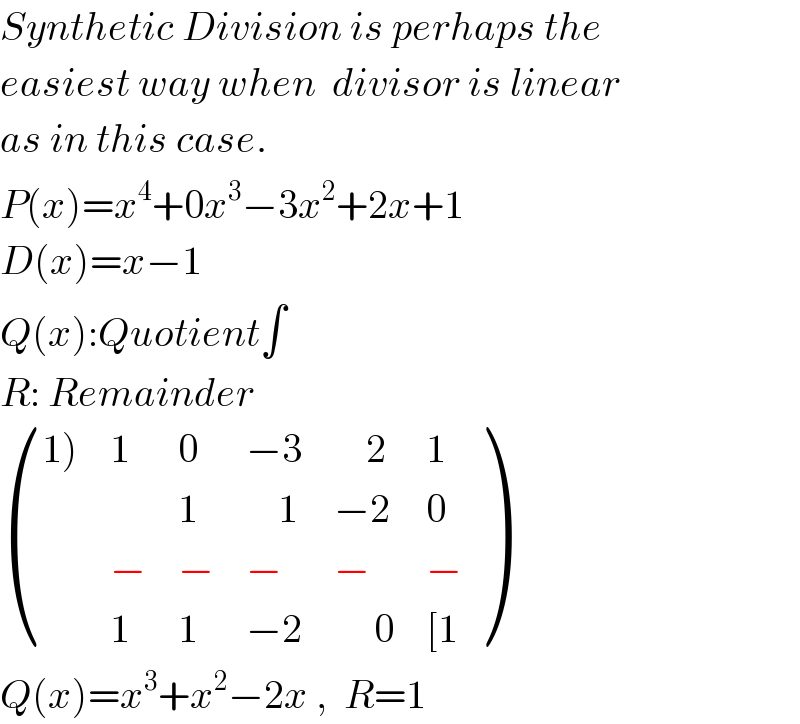
	Commented by malwaan last updated on 26/May/19

	$y e s m r R a s h e e d$ $f a s t a n d e a s y$ $t h a n k y o u$
	Commented by Rasheed.Sindhi last updated on 27/May/19

	${Y o u}^{'} r e w e l c o m e s i r!$
	Commented by Rasheed.Sindhi last updated on 27/May/19

	$T h i s i s a l s o a n e a s y w a y t o e v a l u a t e$ $p o l y n o m i a l P (x) a t x = a i - e P (a) .$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum