Question and Answers Forum

All Questions      Topic List

Mensuration Questions

Previous in All Question      Next in All Question      

Previous in Mensuration      Next in Mensuration      

Question Number 6136 by sanusihammed last updated on 15/Jun/16

Show that of all rectangles inscribed in a given circle the square has a maximum area.

$${Show}\:{that}\:{of}\:{all}\:{rectangles}\:{inscribed}\:{in}\:{a}\:{given}\:{circle}\: \\ $$$${the}\:{square}\:{has}\:{a}\:{maximum}\:{area}. \\ $$

Answered by Rasheed Soomro last updated on 15/Jun/16

All the rectangles inscribed in same circle have equal diagonals and vice versa. Let the measure of diagonal is D If a and b are dimentions of rectangle D=(√(a^2 +b^2 )) If s is measure of the side of square D=(√(s^2 +s^2 ))=(√2) s (√(a^2 +b^2 ))=(√2) s s=(√((a^2 +b^2 )/2)) Area of square=s^2 =((a^2 +b^2 )/2) Area of rectangle=ab Now we have to prove that Area of square>Area of rectangle ((a^2 +b^2 )/2)>ab ∀a,b>0 ∧ a≠b There is a proved relation between arithmatic mean,geometric mean and harmonic mean which will help us to prove the above result. If A, G, H are A.M. ,positive G.M. and H.M. between any two positive and unequal numbers a and b then A>G>H ((a+b)/2)>ab>((2ab)/(a+b)) ((a+b)/2)>((2ab)/(a+b)) (( (a+b)^2 )/2)>2ab (( a^2 +2ab+b^2 )/2)>2ab (a^2 /2)+ab+(b^2 /2)>2ab ((a^2 +b^2 )/2)>ab Area of square_() >Area of rectangle provided that they have same diagonals.I-E they are inscribed in same circle

$${All}\:{the}\:{rectangles}\:{inscribed}\:{in}\:{same}\:{circle} \\ $$$${have}\:{equal}\:{diagonals}\:{and}\:{vice}\:{versa}. \\ $$$${Let}\:{the}\:{measure}\:{of}\:{diagonal}\:\:{is}\:{D} \\ $$$${If}\:\boldsymbol{{a}}\:{and}\:\boldsymbol{{b}}\:{are}\:{dimentions}\:{of}\:{rectangle} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{D}=\sqrt{\boldsymbol{{a}}^{\mathrm{2}} +\boldsymbol{{b}}^{\mathrm{2}} } \\ $$$${If}\:\boldsymbol{{s}}\:{is}\:{measure}\:\:{of}\:{the}\:{side}\:{of}\:{square} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{D}=\sqrt{\boldsymbol{{s}}^{\mathrm{2}} +\boldsymbol{{s}}^{\mathrm{2}} }=\sqrt{\mathrm{2}}\:\boldsymbol{{s}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\sqrt{\boldsymbol{{a}}^{\mathrm{2}} +\boldsymbol{{b}}^{\mathrm{2}} }=\sqrt{\mathrm{2}}\:\boldsymbol{{s}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\boldsymbol{{s}}=\sqrt{\frac{\boldsymbol{{a}}^{\mathrm{2}} +\boldsymbol{{b}}^{\mathrm{2}} }{\mathrm{2}}} \\ $$$$\:\:\:{Area}\:{of}\:{square}=\boldsymbol{{s}}^{\mathrm{2}} =\frac{\boldsymbol{{a}}^{\mathrm{2}} +\boldsymbol{{b}}^{\mathrm{2}} }{\mathrm{2}} \\ $$$$\:\:\:{Area}\:{of}\:{rectangle}=\boldsymbol{{ab}} \\ $$$${Now}\:{we}\:{have}\:{to}\:{prove}\:{that} \\ $$$$\:\:\:\:\:\:\:\:\:{Area}\:{of}\:{square}>{Area}\:{of}\:{rectangle} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\frac{\boldsymbol{{a}}^{\mathrm{2}} +\boldsymbol{{b}}^{\mathrm{2}} }{\mathrm{2}}>\boldsymbol{{ab}}\:\:\:\:\:\:\forall\boldsymbol{{a}},\boldsymbol{{b}}>\mathrm{0}\:\wedge\:\boldsymbol{{a}}\neq\boldsymbol{{b}} \\ $$$${There}\:{is}\:{a}\:{proved}\:{relation}\:{between}\:{arithmatic} \\ $$$${mean},{geometric}\:{mean}\:{and}\:{harmonic}\:{mean} \\ $$$${which}\:{will}\:{help}\:{us}\:{to}\:{prove}\:{the}\:{above}\:{result}. \\ $$$${If}\:{A},\:{G},\:{H}\:{are}\:{A}.{M}.\:,{positive}\:{G}.{M}.\:{and} \\ $$$${H}.{M}.\:\:{between}\:{any}\:{two}\:{positive}\:{and}\:{unequal} \\ $$$${numbers}\:\boldsymbol{{a}}\:\:{and}\:\:\boldsymbol{{b}}\:\:{then} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{A}>{G}>{H} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\frac{\boldsymbol{{a}}+\boldsymbol{{b}}}{\mathrm{2}}>\boldsymbol{{ab}}>\frac{\mathrm{2}\boldsymbol{{ab}}}{\boldsymbol{{a}}+\boldsymbol{{b}}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\frac{\boldsymbol{{a}}+\boldsymbol{{b}}}{\mathrm{2}}>\frac{\mathrm{2}\boldsymbol{{ab}}}{\boldsymbol{{a}}+\boldsymbol{{b}}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\frac{\:\left(\boldsymbol{{a}}+\boldsymbol{{b}}\right)^{\mathrm{2}} }{\mathrm{2}}>\mathrm{2}\boldsymbol{{ab}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\frac{\:\boldsymbol{{a}}^{\mathrm{2}} +\mathrm{2}\boldsymbol{{ab}}+\boldsymbol{{b}}^{\mathrm{2}} }{\mathrm{2}}>\mathrm{2}\boldsymbol{{ab}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\frac{\boldsymbol{{a}}^{\mathrm{2}} }{\mathrm{2}}+\boldsymbol{{ab}}+\frac{\boldsymbol{{b}}^{\mathrm{2}} }{\mathrm{2}}>\mathrm{2}\boldsymbol{{ab}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\frac{\boldsymbol{{a}}^{\mathrm{2}} +\boldsymbol{{b}}^{\mathrm{2}} }{\mathrm{2}}>\boldsymbol{{ab}} \\ $$$$\:\:\:\:\:\:\:\:\underset{} {{Area}\:{of}\:{square}}>{Area}\:{of}\:{rectangle} \\ $$$${provided}\:{that}\:{they}\:{have}\:{same}\:{diagonals}.{I}-{E} \\ $$$${they}\:{are}\:{inscribed}\:{in}\:{same}\:{circle} \\ $$$$ \\ $$

Answered by FilupSmith last updated on 15/Jun/16

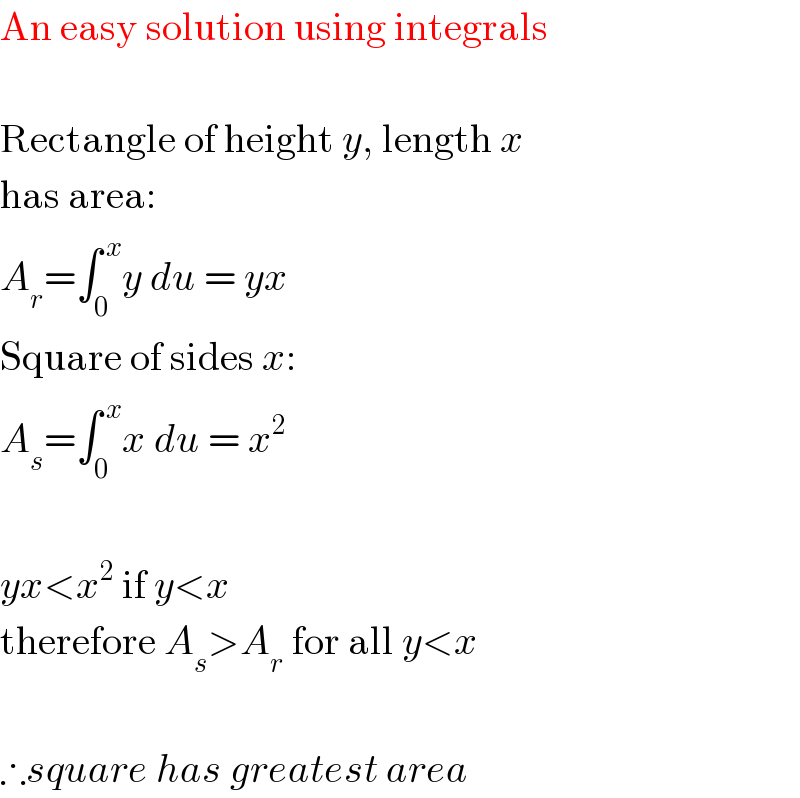
An easy solution using integrals Rectangle of height y, length x has area: A_r =∫_0 ^( x) y du = yx Square of sides x: A_s =∫_0 ^( x) x du = x^2 yx<x^2 if y<x therefore A_s >A_r for all y<x ∴square has greatest area

$$\mathrm{An}\:\mathrm{easy}\:\mathrm{solution}\:\mathrm{using}\:\mathrm{integrals} \\ $$$$\: \\ $$$$\mathrm{Rectangle}\:\mathrm{of}\:\mathrm{height}\:{y},\:\mathrm{length}\:{x} \\ $$$$\mathrm{has}\:\mathrm{area}: \\ $$$${A}_{{r}} =\int_{\mathrm{0}} ^{\:{x}} {y}\:{du}\:=\:{yx} \\ $$$$\mathrm{Square}\:\mathrm{of}\:\mathrm{sides}\:{x}: \\ $$$${A}_{{s}} =\int_{\mathrm{0}} ^{\:{x}} {x}\:{du}\:=\:{x}^{\mathrm{2}} \\ $$$$ \\ $$$${yx}<{x}^{\mathrm{2}} \:\mathrm{if}\:{y}<{x} \\ $$$$\mathrm{therefore}\:{A}_{{s}} >{A}_{{r}} \:\mathrm{for}\:\mathrm{all}\:{y}<{x} \\ $$$$ \\ $$$$\therefore{square}\:{has}\:{greatest}\:{area} \\ $$

Commented by FilupSmith last updated on 15/Jun/16

oh, i didnt read the part about the cirlce sorry, but i misread the question

$$\mathrm{oh},\:\mathrm{i}\:\mathrm{didnt}\:\mathrm{read}\:\mathrm{the}\:\mathrm{part}\:\mathrm{about}\:\mathrm{the}\:\mathrm{cirlce} \\ $$$$\mathrm{sorry},\:\mathrm{but}\:\mathrm{i}\:\mathrm{misread}\:\mathrm{the}\:\mathrm{question} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com