
Question and Answers Forum
Question Number 61966 by aliesam last updated on 12/Jun/19
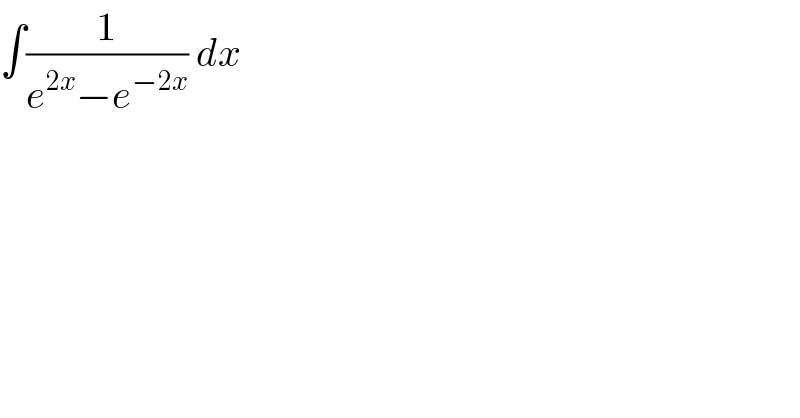
Commented by kaivan.ahmadi last updated on 12/Jun/19

Commented by maxmathsup by imad last updated on 12/Jun/19
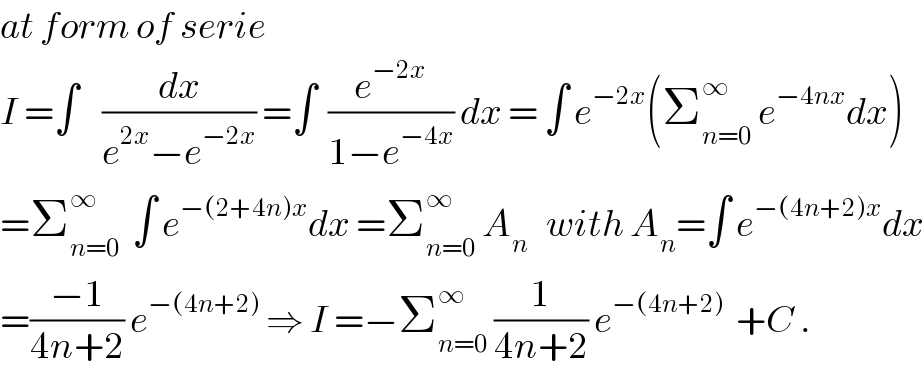
Commented by kaivan.ahmadi last updated on 12/Jun/19
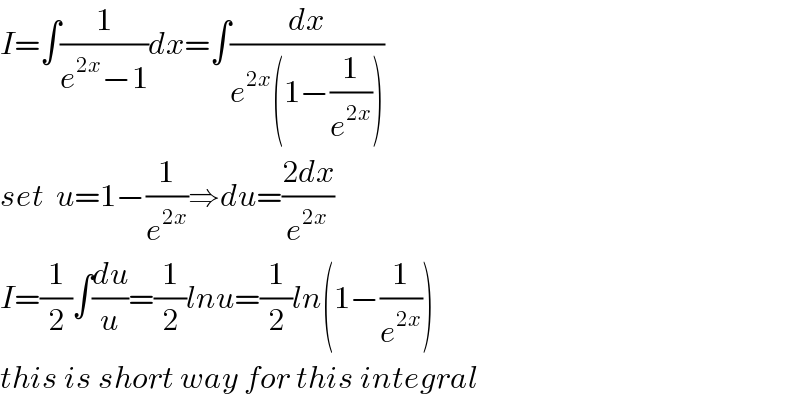
Commented by maxmathsup by imad last updated on 12/Jun/19
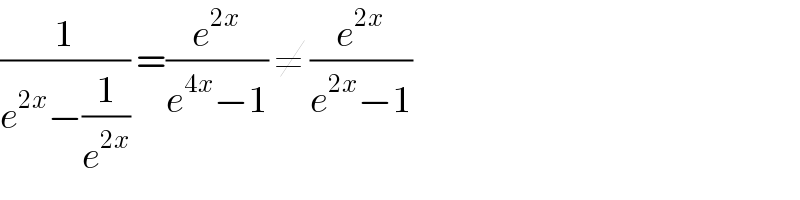
Answered by MJS last updated on 13/Jun/19
![∫(dx/(e^(2x) −e^(−2x) ))= [t=e^(2x) → dx=(dt/(2t))] =∫(dt/(2(t^2 −1)))=∫((1/(4(t−1)))−(1/(4(t+1))))dt=(1/4)ln ∣((t−1)/(t+1))∣ = =(1/4)ln ((e^(2x) −1)/(e^(2x) +1)) +C=(1/4)ln tanh x +C btw. ∫(dx/(e^(2x) −e^(−2x) ))=∫(dx/(2sinh 2x))](Q61988.png)
Answered by mr W last updated on 13/Jun/19
![∫(1/(e^(2x) −e^(−2x) )) dx =(1/2)∫(1/(e^(2x) −e^(−2x) )) d(2x) =(1/2)∫(1/(e^t −e^(−t) )) dt =(1/2)∫(e^t /((e^t )^2 −1)) dt =(1/2)∫(1/((e^t )^2 −1)) d(e^t ) =(1/2)∫(1/(s^2 −1)) ds =(1/4)∫[(1/(s−1))−(1/(s+1))] ds =(1/4)ln ∣((s−1)/(s+1))∣+C =(1/4)ln ∣((e^(2x) −1)/(e^(2x) +1))∣+C](Q61984.png)
