
Question and Answers Forum
Question Number 62086 by Cypher1207 last updated on 15/Jun/19
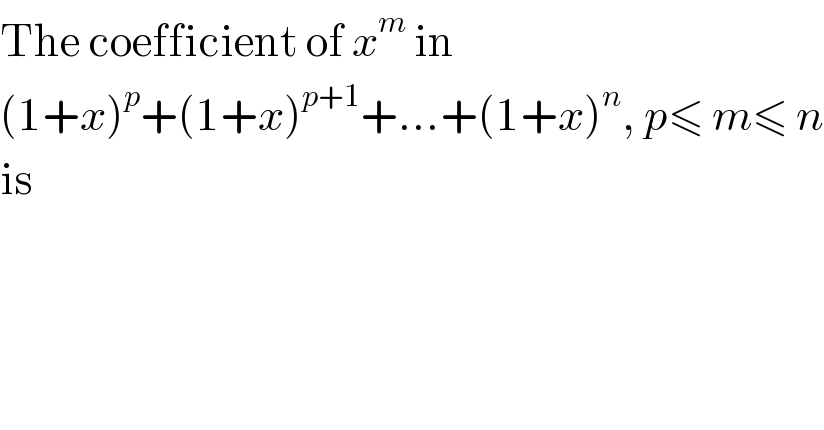
Answered by mr W last updated on 15/Jun/19
![(1+x)^p +(1+x)^(p+1) +...+(1+x)^n ← GP =(((1+x)^p [(1+x)^(n−p+1) −1])/((1+x)−1)) =(((1+x)^(n+1) −(1+x)^p )/x) =(1/x)[Σ_(k=0) ^(n+1) C_k ^(n+1) x^k −Σ_(k=0) ^p C_k ^p x^k ] =(1/x)[Σ_(k=0) ^p C_k ^(n+1) x^k −Σ_(k=0) ^p C_k ^p x^k +Σ_(k=p+1) ^(n+1) C_k ^(n+1) x^k ] =(1/x)[Σ_(k=0) ^p (C_k ^(n+1) −C_k ^p )x^k +Σ_(k=p+1) ^(n+1) C_k ^(n+1) x^k ] =Σ_(k=0) ^p (C_k ^(n+1) −C_k ^p )x^(k−1) +Σ_(k=p+1) ^(n+1) C_k ^(n+1) x^(k−1) =Σ_(k=0) ^(p−1) (C_(k+1) ^(n+1) −C_(k+1) ^p )x^k +Σ_(k=p) ^n C_(k+1) ^(n+1) x^k =Σ_(k=0) ^n a_k x^k with a_k = { ((C_(k+1) ^(n+1) −C_(k+1) ^p for 0≤k≤p−1)),((C_(k+1) ^(n+1) for p≤k≤n)) :} for p≤m≤n, coef. of x^m is C_(m+1) ^(n+1) .](Q62095.png)
| ||
Question and Answers Forum | ||
Question Number 62086 by Cypher1207 last updated on 15/Jun/19 | ||
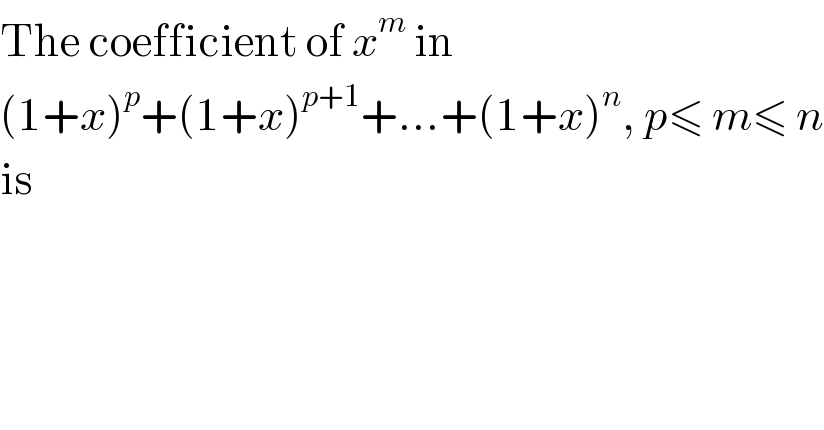 | ||
Answered by mr W last updated on 15/Jun/19 | ||
![(1+x)^p +(1+x)^(p+1) +...+(1+x)^n ← GP =(((1+x)^p [(1+x)^(n−p+1) −1])/((1+x)−1)) =(((1+x)^(n+1) −(1+x)^p )/x) =(1/x)[Σ_(k=0) ^(n+1) C_k ^(n+1) x^k −Σ_(k=0) ^p C_k ^p x^k ] =(1/x)[Σ_(k=0) ^p C_k ^(n+1) x^k −Σ_(k=0) ^p C_k ^p x^k +Σ_(k=p+1) ^(n+1) C_k ^(n+1) x^k ] =(1/x)[Σ_(k=0) ^p (C_k ^(n+1) −C_k ^p )x^k +Σ_(k=p+1) ^(n+1) C_k ^(n+1) x^k ] =Σ_(k=0) ^p (C_k ^(n+1) −C_k ^p )x^(k−1) +Σ_(k=p+1) ^(n+1) C_k ^(n+1) x^(k−1) =Σ_(k=0) ^(p−1) (C_(k+1) ^(n+1) −C_(k+1) ^p )x^k +Σ_(k=p) ^n C_(k+1) ^(n+1) x^k =Σ_(k=0) ^n a_k x^k with a_k = { ((C_(k+1) ^(n+1) −C_(k+1) ^p for 0≤k≤p−1)),((C_(k+1) ^(n+1) for p≤k≤n)) :} for p≤m≤n, coef. of x^m is C_(m+1) ^(n+1) .](Q62095.png) | ||
| ||