Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 62207 by maxmathsup by imad last updated on 17/Jun/19

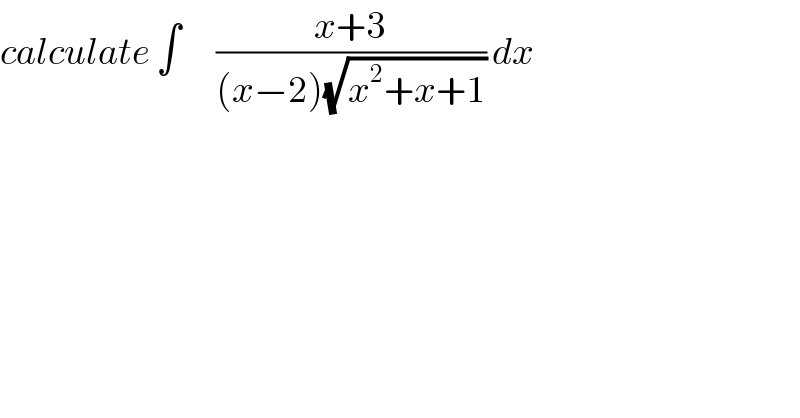
calculate ∫ ((x+3)/((x−2)(√(x^2 +x+1)))) dx

$${calculate}\:\int\:\:\:\:\:\:\frac{{x}+\mathrm{3}}{\left({x}−\mathrm{2}\right)\sqrt{{x}^{\mathrm{2}} +{x}+\mathrm{1}}}\:{dx} \\ $$

Commented by maxmathsup by imad last updated on 18/Jun/19

I =∫ ((x−2 +5)/((x−2)(√(x^2 +x+1))))dx =∫ (dx/(√(x^2 +x+1))) +5∫ (dx/((x−2)(√(x^2 +x+1)))) =H +5K we have x^2 +x+1 =(x+(1/2))^2 +(3/4) so we use the chang.x+(1/2) =((√3)/2)t ⇒ H =∫ ((√3)/(2((√3)/2)(√(1+t^2 )))) dt =∫ (dt/(√(1+t^2 )))=ln(t+(√(1+t^2 ))) +c_1 = H =ln(((2x+1)/(√3)) +(√(1+(((2x+1)/(√3)))^2 ))) +c_1 K =∫ (1/(((((√3)t)/2)−(1/2)−2)((√3)/2)(√(1+t^2 )))) ((√3)/2)dt = ∫ (dt/((((√3)/2)t−(5/2))(√(1+t^2 ))))dt =2 ∫ (dt/(((√3) t−5)(√(1+t^2 )))) =_(t =sh(u)) 2 ∫ ((ch(u)du)/(((√3)sh(u)−5)ch(u))) =2 ∫ (du/((√3)sh(u)−5)) =2 ∫ (du/((√3)((e^u −e^(−u) )/2)−5)) =4 ∫ (du/((√3)(e^u −e^(−u) )−10)) =_(e^u =α) 4 ∫ (1/((√3)α −(√3)α^(−1) −10)) (dα/α) =4 ∫ (dα/((√3)α^2 −(√3)−10 α)) =4 ∫ (dα/((√3)α^2 −10α−(√3))) Δ^′ =(−5)^2 +3 =25 +3 =28 ⇒α_1 =((5+(√(28)))/(√3)) and α_2 =((5−(√(28)))/(√3)) ⇒ ∫ (dα/((√3)α^2 −10α −(√3))) =∫ (dα/((√3)(α−α_1 )(α−α_2 ))) =(1/((√3)(2((√(28))/(√3))))) ∫ ((1/(α−α_1 )) −(1/(α−α_2 )))dα =(1/(4(√7)))ln∣((α−α_1 )/(α−α_2 ))∣ +c_2 ⇒ K =(1/(√7))ln∣((e^u −α_1 )/(e^u −α_2 ))∣ +c_2 but u =argsh(t) =ln(t+(√(1+t^2 ))) ⇒e^u =t+(√(1+t^2 )) K =(1/(√7))ln∣((t+(√(1+t^2 ))−α_1 )/(t+(√(1+t^2 −α_2 ))))∣ +c_2 but t =((2x+1)/(√3)) ⇒ K =(1/(√7))ln∣((((2x+1)/(√3))+(√(1+(((2x+1)/(√3)))^2 ))−α_1 )/(((2x+1)/(√3)) +(√(1+(((2x+1)/(√3)))^2 ))−α_2 ))∣ +c_2 I =H +5K is nown .

$${I}\:=\int\:\frac{{x}−\mathrm{2}\:+\mathrm{5}}{\left({x}−\mathrm{2}\right)\sqrt{{x}^{\mathrm{2}} +{x}+\mathrm{1}}}{dx}\:=\int\:\frac{{dx}}{\sqrt{{x}^{\mathrm{2}} +{x}+\mathrm{1}}}\:+\mathrm{5}\int\:\frac{{dx}}{\left({x}−\mathrm{2}\right)\sqrt{{x}^{\mathrm{2}} +{x}+\mathrm{1}}}\:={H}\:+\mathrm{5}{K} \\ $$$${we}\:{have}\:{x}^{\mathrm{2}} +{x}+\mathrm{1}\:=\left({x}+\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} \:+\frac{\mathrm{3}}{\mathrm{4}}\:{so}\:{we}\:{use}\:{the}\:{chang}.{x}+\frac{\mathrm{1}}{\mathrm{2}}\:=\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}{t}\:\Rightarrow \\ $$$${H}\:=\int\:\:\:\:\:\frac{\sqrt{\mathrm{3}}}{\mathrm{2}\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }}\:{dt}\:=\int\:\:\frac{{dt}}{\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }}={ln}\left({t}+\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }\right)\:+{c}_{\mathrm{1}} \:= \\ $$$${H}\:={ln}\left(\frac{\mathrm{2}{x}+\mathrm{1}}{\sqrt{\mathrm{3}}}\:+\sqrt{\mathrm{1}+\left(\frac{\mathrm{2}{x}+\mathrm{1}}{\sqrt{\mathrm{3}}}\right)^{\mathrm{2}} }\right)\:+{c}_{\mathrm{1}} \\ $$$${K}\:=\int\:\:\:\frac{\mathrm{1}}{\left(\frac{\sqrt{\mathrm{3}}{t}}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{2}}−\mathrm{2}\right)\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }}\:\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}{dt}\:=\:\int\:\:\:\:\:\:\frac{{dt}}{\left(\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}{t}−\frac{\mathrm{5}}{\mathrm{2}}\right)\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }}{dt} \\ $$$$=\mathrm{2}\:\int\:\:\:\frac{{dt}}{\left(\sqrt{\mathrm{3}}\:{t}−\mathrm{5}\right)\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }}\:=_{{t}\:={sh}\left({u}\right)} \:\:\:\mathrm{2}\:\int\:\:\:\frac{{ch}\left({u}\right){du}}{\left(\sqrt{\mathrm{3}}{sh}\left({u}\right)−\mathrm{5}\right){ch}\left({u}\right)} \\ $$$$=\mathrm{2}\:\int\:\:\frac{{du}}{\sqrt{\mathrm{3}}{sh}\left({u}\right)−\mathrm{5}}\:=\mathrm{2}\:\int\:\:\frac{{du}}{\sqrt{\mathrm{3}}\frac{{e}^{{u}} −{e}^{−{u}} }{\mathrm{2}}−\mathrm{5}}\:=\mathrm{4}\:\int\:\:\frac{{du}}{\sqrt{\mathrm{3}}\left({e}^{{u}} −{e}^{−{u}} \right)−\mathrm{10}} \\ $$$$=_{{e}^{{u}} \:=\alpha} \:\:\:\:\:\mathrm{4}\:\int\:\:\:\:\frac{\mathrm{1}}{\sqrt{\mathrm{3}}\alpha\:−\sqrt{\mathrm{3}}\alpha^{−\mathrm{1}} −\mathrm{10}}\:\frac{{d}\alpha}{\alpha}\:=\mathrm{4}\:\int\:\:\:\frac{{d}\alpha}{\sqrt{\mathrm{3}}\alpha^{\mathrm{2}} −\sqrt{\mathrm{3}}−\mathrm{10}\:\alpha}\:=\mathrm{4}\:\int\:\:\frac{{d}\alpha}{\sqrt{\mathrm{3}}\alpha^{\mathrm{2}} −\mathrm{10}\alpha−\sqrt{\mathrm{3}}} \\ $$$$\Delta^{'} \:=\left(−\mathrm{5}\right)^{\mathrm{2}} +\mathrm{3}\:=\mathrm{25}\:+\mathrm{3}\:=\mathrm{28}\:\Rightarrow\alpha_{\mathrm{1}} =\frac{\mathrm{5}+\sqrt{\mathrm{28}}}{\sqrt{\mathrm{3}}}\:{and}\:\alpha_{\mathrm{2}} =\frac{\mathrm{5}−\sqrt{\mathrm{28}}}{\sqrt{\mathrm{3}}}\:\Rightarrow \\ $$$$\int\:\:\frac{{d}\alpha}{\sqrt{\mathrm{3}}\alpha^{\mathrm{2}} −\mathrm{10}\alpha\:−\sqrt{\mathrm{3}}}\:=\int\:\:\frac{{d}\alpha}{\sqrt{\mathrm{3}}\left(\alpha−\alpha_{\mathrm{1}} \right)\left(\alpha−\alpha_{\mathrm{2}} \right)} \\ $$$$=\frac{\mathrm{1}}{\sqrt{\mathrm{3}}\left(\mathrm{2}\frac{\sqrt{\mathrm{28}}}{\sqrt{\mathrm{3}}}\right)}\:\int\:\left(\frac{\mathrm{1}}{\alpha−\alpha_{\mathrm{1}} }\:−\frac{\mathrm{1}}{\alpha−\alpha_{\mathrm{2}} }\right){d}\alpha\:=\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{7}}}{ln}\mid\frac{\alpha−\alpha_{\mathrm{1}} }{\alpha−\alpha_{\mathrm{2}} }\mid\:+{c}_{\mathrm{2}} \:\Rightarrow \\ $$$${K}\:=\frac{\mathrm{1}}{\sqrt{\mathrm{7}}}{ln}\mid\frac{{e}^{{u}} −\alpha_{\mathrm{1}} }{{e}^{{u}} −\alpha_{\mathrm{2}} }\mid\:+{c}_{\mathrm{2}} \:\:\:{but}\:\:{u}\:={argsh}\left({t}\right)\:={ln}\left({t}+\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }\right)\:\Rightarrow{e}^{{u}} \:={t}+\sqrt{\mathrm{1}+{t}^{\mathrm{2}} } \\ $$$${K}\:=\frac{\mathrm{1}}{\sqrt{\mathrm{7}}}{ln}\mid\frac{{t}+\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }−\alpha_{\mathrm{1}} }{{t}+\sqrt{\mathrm{1}+{t}^{\mathrm{2}} \:−\alpha_{\mathrm{2}} }}\mid\:+{c}_{\mathrm{2}} \:\:{but}\:{t}\:=\frac{\mathrm{2}{x}+\mathrm{1}}{\sqrt{\mathrm{3}}}\:\Rightarrow \\ $$$${K}\:=\frac{\mathrm{1}}{\sqrt{\mathrm{7}}}{ln}\mid\frac{\frac{\mathrm{2}{x}+\mathrm{1}}{\sqrt{\mathrm{3}}}+\sqrt{\mathrm{1}+\left(\frac{\mathrm{2}{x}+\mathrm{1}}{\sqrt{\mathrm{3}}}\right)^{\mathrm{2}} }−\alpha_{\mathrm{1}} }{\frac{\mathrm{2}{x}+\mathrm{1}}{\sqrt{\mathrm{3}}}\:+\sqrt{\mathrm{1}+\left(\frac{\mathrm{2}{x}+\mathrm{1}}{\sqrt{\mathrm{3}}}\right)^{\mathrm{2}} }−\alpha_{\mathrm{2}} }\mid\:+{c}_{\mathrm{2}} \\ $$$${I}\:={H}\:+\mathrm{5}{K}\:\:{is}\:{nown}\:. \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$

Answered by tanmay last updated on 17/Jun/19

∫((x−2+5)/((x−2)(√(x^2 +x+1))))dx ∫(dx/(√(x^2 +x+1)))+5∫(dx/((x−2)(√(x^2 +x+1)))) I_1 +5I_2 I_1 =∫(dx/(√(x^2 +2×x×(1/2)+(1/4)+(3/4)))) =∫(dx/(√((x+(1/2))^2 +(((√3)/2))^2 ))) =ln[(x+(1/2))+(√((x+(1/2))^2 +(((√3)/2))^2 )) ] +c_1 I_2 =∫(dx/((x−2)(√(x^2 +x+1)))) x−2=(1/t)→dx=((−dt)/t^2 ) ∫((−dt)/(t^2 ×(1/t)×(√(((1/t)+2)^2 +((1/t)+2)+1)))) ∫((−dt)/(t×(√((1/t^2 )+(4/t)+4+(1/t)+3)))) ∫((−dt)/(t×(√((1+4t+4t^2 +t+3t^2 )/t^2 )))) ∫((−dt)/(√(7t^2 +5t+1))) (1/(√7))∫((−dt)/(√(t^2 +2×t×(5/(14))+((5/(14)))^2 +(1/7)−((25)/(196))))) (1/(√7))∫((−dt)/(√((t+(5/(14)))^2 +((28−25)/(196))))) (1/(√7))∫((−dt)/(√((t+(5/(14)))^2 +(3/(196))))) ((−1)/(√7))×ln[(t+(5/(14)))+(√((t+(5/(14)))^2 +(3/(196)))) ] =((−1)/(√7))×ln[((1/(x−2))+(5/(14)))+(√(((1/(x−2))+(5/(14)))^2 +(3/(196)))) )] now pls caculate I_1 +5I_2

$$\int\frac{{x}−\mathrm{2}+\mathrm{5}}{\left({x}−\mathrm{2}\right)\sqrt{{x}^{\mathrm{2}} +{x}+\mathrm{1}}}{dx} \\ $$$$\int\frac{{dx}}{\sqrt{{x}^{\mathrm{2}} +{x}+\mathrm{1}}}+\mathrm{5}\int\frac{{dx}}{\left({x}−\mathrm{2}\right)\sqrt{{x}^{\mathrm{2}} +{x}+\mathrm{1}}} \\ $$$$\boldsymbol{{I}}_{\mathrm{1}} +\mathrm{5}{I}_{\mathrm{2}} \\ $$$${I}_{\mathrm{1}} =\int\frac{{dx}}{\sqrt{{x}^{\mathrm{2}} +\mathrm{2}×{x}×\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{4}}+\frac{\mathrm{3}}{\mathrm{4}}}} \\ $$$$=\int\frac{{dx}}{\sqrt{\left({x}+\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} +\left(\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\right)^{\mathrm{2}} \:}} \\ $$$$={ln}\left[\left({x}+\frac{\mathrm{1}}{\mathrm{2}}\right)+\sqrt{\left({x}+\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} +\left(\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\right)^{\mathrm{2}} }\:\:\right]\:+{c}_{\mathrm{1}} \\ $$$${I}_{\mathrm{2}} =\int\frac{{dx}}{\left({x}−\mathrm{2}\right)\sqrt{{x}^{\mathrm{2}} +{x}+\mathrm{1}}} \\ $$$${x}−\mathrm{2}=\frac{\mathrm{1}}{{t}}\rightarrow{dx}=\frac{−{dt}}{{t}^{\mathrm{2}} } \\ $$$$\int\frac{−{dt}}{{t}^{\mathrm{2}} ×\frac{\mathrm{1}}{{t}}×\sqrt{\left(\frac{\mathrm{1}}{{t}}+\mathrm{2}\right)^{\mathrm{2}} +\left(\frac{\mathrm{1}}{{t}}+\mathrm{2}\right)+\mathrm{1}}} \\ $$$$\int\frac{−{dt}}{{t}×\sqrt{\frac{\mathrm{1}}{{t}^{\mathrm{2}} }+\frac{\mathrm{4}}{{t}}+\mathrm{4}+\frac{\mathrm{1}}{{t}}+\mathrm{3}}} \\ $$$$\int\frac{−{dt}}{{t}×\sqrt{\frac{\mathrm{1}+\mathrm{4}{t}+\mathrm{4}{t}^{\mathrm{2}} +{t}+\mathrm{3}{t}^{\mathrm{2}} }{{t}^{\mathrm{2}} }}} \\ $$$$\int\frac{−{dt}}{\sqrt{\mathrm{7}{t}^{\mathrm{2}} +\mathrm{5}{t}+\mathrm{1}}} \\ $$$$\frac{\mathrm{1}}{\sqrt{\mathrm{7}}}\int\frac{−{dt}}{\sqrt{{t}^{\mathrm{2}} +\mathrm{2}×{t}×\frac{\mathrm{5}}{\mathrm{14}}+\left(\frac{\mathrm{5}}{\mathrm{14}}\right)^{\mathrm{2}} +\frac{\mathrm{1}}{\mathrm{7}}−\frac{\mathrm{25}}{\mathrm{196}}}} \\ $$$$\frac{\mathrm{1}}{\sqrt{\mathrm{7}}}\int\frac{−{dt}}{\sqrt{\left({t}+\frac{\mathrm{5}}{\mathrm{14}}\right)^{\mathrm{2}} +\frac{\mathrm{28}−\mathrm{25}}{\mathrm{196}}}} \\ $$$$\frac{\mathrm{1}}{\sqrt{\mathrm{7}}}\int\frac{−{dt}}{\sqrt{\left({t}+\frac{\mathrm{5}}{\mathrm{14}}\right)^{\mathrm{2}} +\frac{\mathrm{3}}{\mathrm{196}}}} \\ $$$$\frac{−\mathrm{1}}{\sqrt{\mathrm{7}}}×{ln}\left[\left({t}+\frac{\mathrm{5}}{\mathrm{14}}\right)+\sqrt{\left({t}+\frac{\mathrm{5}}{\mathrm{14}}\right)^{\mathrm{2}} +\frac{\mathrm{3}}{\mathrm{196}}}\:\right] \\ $$$$\left.=\frac{−\mathrm{1}}{\sqrt{\mathrm{7}}}×{ln}\left[\left(\frac{\mathrm{1}}{{x}−\mathrm{2}}+\frac{\mathrm{5}}{\mathrm{14}}\right)+\sqrt{\left(\frac{\mathrm{1}}{{x}−\mathrm{2}}+\frac{\mathrm{5}}{\mathrm{14}}\right)^{\mathrm{2}} +\frac{\mathrm{3}}{\mathrm{196}}}\:\right)\right] \\ $$$${now}\:{pls}\:{caculate}\:{I}_{\mathrm{1}} +\mathrm{5}{I}_{\mathrm{2}} \\ $$$$ \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com